Hostname: page-component-586b7cd67f-t8hqh Total loading time: 0 Render date: 2024-12-02T21:19:26.638Z Has data issue: false hasContentIssue false
  • English
  • Français

Investigation of tone generation in ideally expanded supersonic planar impinging jets using large-eddy simulation

Published online by Cambridge University Press:  26 October 2016

Romain Gojon Open the ORCID record for Romain Gojon [Opens in a new window] ,
Christophe Bogey  and
Olivier Marsden
Romain Gojon*
Affiliation:
Laboratoire de Mécanique des Fluides et d’Acoustique, UMR CNRS 5509, Ecole Centrale de Lyon, Université de Lyon, 69134 Ecully CEDEX, France
Christophe Bogey
Affiliation:
Laboratoire de Mécanique des Fluides et d’Acoustique, UMR CNRS 5509, Ecole Centrale de Lyon, Université de Lyon, 69134 Ecully CEDEX, France
Olivier Marsden
Affiliation:
Laboratoire de Mécanique des Fluides et d’Acoustique, UMR CNRS 5509, Ecole Centrale de Lyon, Université de Lyon, 69134 Ecully CEDEX, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The generation of tones in a supersonic planar jet impinging on a flat plate normally has been investigated by performing compressible large-eddy simulations using low-dissipation and low-dispersion finite differences. At the exit of a straight nozzle of height $h$, the jet is ideally expanded, and has a Mach number of 1.28 and a Reynolds number of 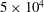$5\times 10^{4}$. Four distances between the nozzle and the plate between 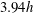$3.94h$ and 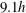$9.1h$ have been considered. Flow snapshots and mean velocity fields are first presented. The variations of turbulence intensities and of the convection velocity in the jet shear layers are then examined. The properties of the jet near fields are subsequently described, in particular by applying Fourier decomposition to the pressure fields. Several coexisting tones appear to be generated by aeroacoustic feedback loops establishing between the nozzle lip and the flat plate, which also lead to the presence of hydrodynamic–acoustic standing waves. The tone frequencies are consistent with those given by the aeroacoustic feedback model and with measurements for high-aspect-ratio rectangular jets. The jet oscillation modes at these frequencies are characterized, and found to agree with experimental data. Their symmetric or antisymmetric natures are shown to be well predicted by a wave analysis carried out using a vortex sheet model of the jet, providing the allowable frequency ranges for the upstream-propagating acoustic waves. Thus, it is possible, for an ideally expanded impinging planar jet to predict both the frequencies of the tones and the symmetric or antisymmetric nature of the corresponding oscillation modes by combining the aeroacoustic feedback model and the wave analysis.

JFM classification

Acoustics: Aeroacoustics Acoustics: Jet noise
Type
Papers
Information
Journal of Fluid Mechanics , Volume 808 , 10 December 2016 , pp. 90 - 115
Check for updates
Copyright
© 2016 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Arthurs, D. & Ziada, S. 2012 Self-excited oscillations of a high-speed impinging planar jet. J. Fluids Struct. 34, 236–258.CrossRefGoogle Scholar
Baars, W. J. & Tinney, C. E.2013 Quantifying crackle-inducing acoustic shock-structures emitted by a fully-expanded mach 3 jet. AIAA Paper 2013-2081.CrossRefGoogle Scholar
Baars, W. J. & Tinney, C. E. 2014 Shock-structures in the acoustic field of a mach 3 jet with crackle. J. Sound Vib. 333 (12), 2539–2553.Google Scholar
Berland, J., Bogey, C. & Bailly, C. 2007a Numerical study of screech generation in a planar supersonic jet. Phys. Fluids 19, 075105.Google Scholar
Berland, J., Bogey, C., Marsden, O. & Bailly, C. 2007b High-order, low dispersive and low dissipative explicit schemes for multiple-scale and boundary problems. J. Comput. Phys. 224 (2), 637–662.CrossRefGoogle Scholar
Berman, C. H. & Williams, J. E. 1970 Instability of a two-dimensional compressible jet. J. Fluid Mech. 42 (1), 151–159.Google Scholar
Bogey, C. & Bailly, C. 2004 A family of low dispersive and low dissipative explicit schemes for flow and noise computations. J. Comput. Phys. 194 (1), 194–214.Google Scholar
Bogey, C. & Bailly, C. 2006 Large eddy simulations of transitional round jets: influence of the Reynolds number on flow development and energy dissipation. Phys. Fluids 18, 065101.CrossRefGoogle Scholar
Bogey, C. & Bailly, C. 2009 Turbulence and energy budget in a self-preserving round jet: direct evaluation using large eddy simulation. J. Fluid Mech. 627, 129–160.Google Scholar
Bogey, C., de Cacqueray, N. & Bailly, C. 2009 A shock-capturing methodology based on adaptative spatial filtering for high-order non-linear computations. J. Comput. Phys. 228 (5), 1447–1465.Google Scholar
Bogey, C. & Marsden, O. 2016 Simulations of initially highly disturbed jets with experiment-like exit boundary layers. AIAA J. 54 (2), 1299–2016.CrossRefGoogle Scholar
Bogey, C., Marsden, O. & Bailly, C. 2011 Large-eddy simulation of the flow and acoustic fields of a Reynolds number 105 subsonic jet with tripped exit boundary layers. Phys. Fluids 23, 035104.Google Scholar
Bogey, C., Marsden, O. & Bailly, C. 2012 Influence of initial turbulence level on the flow and sound fields of a subsonic jet at a diameter-based Reynolds number of 105 . J. Fluid Mech. 701, 352–385.CrossRefGoogle Scholar
Buchmann, N. A., Mitchell, D. M., Ingvorsen, K. M., Honnery, D. R. & Soria, J. 2011 High spatial resolution imaging of a supersonic underexpanded jet impinging on a flat plate. In 6th Australian Conference on Laser Diagnostics in Fluid Mechanics and Combustion, Canberra, Australia, 5–7 December 2011.Google Scholar
de Cacqueray, N. & Bogey, C. 2014 Noise of an overexpanded mach 3.3 jet: non-linear propagation effects and correlations with flow. Intl J. Aeroacoust. 13 (7), 607–632.CrossRefGoogle Scholar
de Cacqueray, N., Bogey, C. & Bailly, C. 2011 Investigation of a high-mach-number overexpanded jet using large-eddy simulation. AIAA J. 49 (10), 2171–2182.Google Scholar
Fauconnier, D., Bogey, C. & Dick, E. 2013 On the performance of relaxation filtering for large-eddy simulation. J. Turbul. 14 (1), 22–49.Google Scholar
Ffowcs-Williams, J. E., Simson, J. & Virchis, V. J. 1975 Crackle: an annoying component of jet noise. J. Fluid Mech. 71 (02), 251–271.Google Scholar
George, W. K., Abrahamsson, H., Eriksson, J., Karlsson, R. I., Löfdahl, L. & Wosnik, M. 2000 A similarity theory for the turbulent plane wall jet without external stream. J. Fluid Mech. 425, 367–411.CrossRefGoogle Scholar
Henderson, B., Bridges, J. & Wernet, M. 2005 An experimental study of the oscillatory flow structure of tone-producing supersonic impinging jets. J. Fluid Mech. 542, 115–137.Google Scholar
Henderson, B. & Powell, A. 1993 Experiments concerning tones produced by an axisymmetric choked jet impinging on flat plates. J. Sound Vib. 168 (2), 307–326.Google Scholar
Ho, C. M. & Nosseir, N. S. 1981 Dynamics of an impinging jet. Part 1. The feedback phenomenon. J. Fluid Mech. 105, 119–142.Google Scholar
Hourigan, K., Rudman, M. & Brocher, E. 1996 The feedback loop in impinging two-dimensional high-subsonic and supersonic jets. Exp. Therm. Fluid Sci. 12 (2), 265–270.Google Scholar
Irwin, H. P. A. H. 1973 Measurements in a self-preserving plane wall jet in a positive pressure gradient. J. Fluid Mech. 61 (1), 33–63.CrossRefGoogle Scholar
Kremer, F. & Bogey, C. 2015 Large-eddy simulation of turbulent channel flow using relaxation filtering: resolution requirement and Reynolds number effect. Comput. Fluids 17 (7), 17–28.CrossRefGoogle Scholar
Krothapalli, A. 1985 Discrete tones generated by an impinging underexpanded rectangular jet. AIAA J. 23 (12), 1910–1915.Google Scholar
Krothapalli, A., Rajkuperan, E., Alvi, F. & Lourenco, L. 1999 Flow field and noise characteristics of a supersonic impinging jet. J. Fluid Mech. 392, 155–181.Google Scholar
Mack, L. M. 1990 On the inviscid acoustic-mode instability of supersonic shear flows. Theor. Comput. Fluid Dyn. 2 (2), 97–123.Google Scholar
Mitchell, D. M., Honnery, D. R. & Soria, J. 2012 The visualization of the acoustic feedback loop in impinging underexpanded supersonic jet flows using ultra-high frame rate schlieren. J. Vis. 15 (4), 333–341.Google Scholar
Norum, T. D. 1991 Supersonic rectangular jet impingement noise experiments. AIAA J. 29 (7), 1051–1057.Google Scholar
Nosseir, N. S. & Ho, C. M. 1982 Dynamics of an impinging jet. Part 2. The noise generation. J. Fluid Mech. 116, 379–391.Google Scholar
Panda, J., Raman, G. & Zaman, K. B. M. Q.1997 Underexpanded screeching jets from circular, rectangular and elliptic nozzles. AIAA Paper 97-1623.Google Scholar
Panda, J. & Seasholtz, R. G. 1999 Measurement of shock structure and shock-vortex interaction in underexpanded jets using rayleigh scattering. Phys. Fluids 11 (12), 3761–3777.Google Scholar
Papamoschou, D. & Roshko, A. 1988 The compressible turbulent shear layer: an experimental study. J. Fluid Mech. 197, 453–477.Google Scholar
Powell, A. 1953 On edge tones and associated phenomena. Acta Acust. 3, 233–243.Google Scholar
Risborg, A. & Soria, J.2009 High-speed optical measurements of an underexpanded supersonic jet impinging on an inclined plate. In 28th International Congress on High-Speed Imaging and Photonics, 7126 (F), International Society for Optics and Photonics.CrossRefGoogle Scholar
Rockwell, D. & Naudascher, E. 1978 Review-self-sustaining oscillations of flow past cavities. Trans. ASME J. Fluids Engng 100 (2), 152–165.CrossRefGoogle Scholar
Sabatini, R. & Bailly, C. 2014 Numerical algorithm for computing acoustic and vortical spatial instability waves. AIAA J. 53 (3), 692–702.Google Scholar
Tam, C. K. W. & Ahuja, K. K. 1990 Theoretical model of discrete tone generation by impinging jets. J. Fluid Mech. 214, 67–87.CrossRefGoogle Scholar
Tam, C. K. W. & Dong, Z. 1994 Wall boundary conditions for high-order finite-difference schemes in computational aeroacoustics. Theor. Comput. Fluid Dyn. 6, 303–322.Google Scholar
Tam, C. K. W. & Hu, F. Q. 1989 On the three families of instability waves of high-speed jets. J. Fluid Mech. 201, 447–483.Google Scholar
Tam, C. K. W. & Norum, T. D. 1992 Impingement tones of large aspect ratio supersonic rectangular jets. AIAA J. 30 (2), 304–311.Google Scholar
Thurow, B., Samimy, M. & Lempert, W.2002 Structure of a supersonic impinging rectangular jet via real-time optical diagnostics. AIAA Paper 2002-2865.Google Scholar

Gojon et al. Movie 1

Animation in the (x,y) plane of the density in the jet and close to the flat plate and of the pressure fluctuations for (a) JetL3.9, (b) JetL5.5, (c) JetL8.3 and (d) JetL9.1. The colour scale ranges from 1 to 2 kg.m-3 for density, from blue to red and from -7500 to 7500 Pa for pressure, from black to white. The nozzle is in black.

Download Gojon et al. Movie 1(Video)
Video 8.6 MB