Hostname: page-component-586b7cd67f-l7hp2 Total loading time: 0 Render date: 2024-11-28T17:37:12.103Z Has data issue: false hasContentIssue false
  • English
  • Français

Interface-resolved numerical simulations of particle-laden turbulent flows in a vertical channel filled with Bingham fluids

Published online by Cambridge University Press:  28 November 2019

Chenlin Zhu ,
Zhaosheng Yu Open the ORCID record for Zhaosheng Yu [Opens in a new window] ,
Xueming Shao  and
Jian Deng
Chenlin Zhu
Affiliation:
State Key Laboratory of Fluid Power and Mechatronic Systems, Department of Mechanics, Zhejiang University, Hangzhou 310027, China China Jiliang University, Hangzhou 310018, China
Zhaosheng Yu*
Affiliation:
State Key Laboratory of Fluid Power and Mechatronic Systems, Department of Mechanics, Zhejiang University, Hangzhou 310027, China
Xueming Shao
Affiliation:
State Key Laboratory of Fluid Power and Mechatronic Systems, Department of Mechanics, Zhejiang University, Hangzhou 310027, China
Jian Deng
Affiliation:
State Key Laboratory of Fluid Power and Mechatronic Systems, Department of Mechanics, Zhejiang University, Hangzhou 310027, China
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

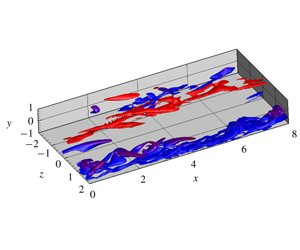
In this paper, a fictitious domain method is used to perform interface-resolved numerical simulations of particle-laden turbulent flows in a vertical channel filled with Bingham fluids. The interactions between the particles and the turbulence are investigated at a bulk Reynolds number of 8000 (based on the mean velocity and the channel width), a particle/channel size ratio of 0.1 and a particle volume fraction of 2 %. The Bingham number based on the mean velocity and the channel half-width is 0.0, 0.54 and 3.0, respectively. Both neutrally buoyant and weak particle settling effects are considered. Our results indicate that the yield stress of Bingham fluids has drag-reduction effects by weakening the turbulence, and the addition of the particles enhances the flow friction. The Saffman lift force is important to the particle concentration distribution across the channel. Particle sedimentation attenuates the fluctuating velocity and Reynolds stress, resulting in a smaller flow friction compared with the neutrally buoyant case. When the fluid plasticity is strong, the single-phase flow has considerable temporal and spatial intermittencies. The vortex structures in one half-channel can be stronger than those in the other half-channel for a long period, resulting in the asymmetry of the statistics for a long time. The vortices are preferentially concentrated in streamwise-extended bands. The vortex bands and the laminar flow regions are largely segregated in the spanwise directions, and the vortex bands in the two half-channels are also typically staggered in the spanwise direction. The addition of the particles suppresses such vortex structures, the enormous intermittency and the asymmetry during the transition in the single-phase flow.

JFM classification

Complex Fluids: Suspensions Multiphase and Particle-laden Flows: Particle/fluid flow Non-Newtonian Flows: Plastic materials
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 883 , 25 January 2020 , A43
Copyright
© 2019 Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Anbarlooei, H. R., Cruz, D. O. A., Ramos, F., Santos, C. M. M. & Freire, A. P. S. 2017 Phenomenological friction equation for turbulent flow of Bingham fluids. Phys. Rev. E 96 (2), 023107.Google ScholarPubMed
Ardekani, M. N., Costa, P., Breugem, W.-P., Picano, F. & Brandt, L. 2017 Drag reduction in turbulent channel flow laden with finite-size oblate spheroids. J. Fluid Mech. 816, 43–70.CrossRefGoogle Scholar
Bird, R. B., Dai, G. C. & Yarusso, B. J. 1983 The rheology and flow of viscoplastic materials. Rev. Chem. Engng 1 (1), 1–70.CrossRefGoogle Scholar
Blackery, J. & Mitsoulis, E. 1997 Creeping motion of a sphere in tubes filled with a Bingham plastic material. J. Non-Newtonian Fluid Mech. 70 (1–2), 59–77.CrossRefGoogle Scholar
Chhabra, R. P. 2006 Bubbles, Drops, and Particles in Non-Newtonian Fluids, 2nd edn. CRC Press.CrossRefGoogle Scholar
Cisse, M., Homann, H. & Bec, J. 2013 Slipping motion of large neutrally buoyant particles in turbulence. J. Fluid Mech. 735, R1.CrossRefGoogle Scholar
Costa, P., Picano, F., Brandt, L. & Breugem, W.-P. 2018 Effects of the finite particle size in turbulent wall-bounded flows of dense suspensions. J. Fluid Mech. 843, 450–478.CrossRefGoogle Scholar
Darby, R. & Melson, J. 1981 How to predict the friction factor for flow of Bingham plastics. Chem. Engng 88 (26), 59–61.Google Scholar
Dean, E. J. & Glowinski, R. 2002 Operator-splitting methods for the simulation of Bingham visco-plastic flow. Chin. Ann. Maths 23 (02), 187–204.CrossRefGoogle Scholar
Dean, R. B. 1978 Reynolds number dependence of skin friction and other bulk flow variables in two-dimensional rectangular duct flow. J. Fluids Engng 100 (2), 215–223.CrossRefGoogle Scholar
Derksen, J. J. 2009 Solid particle mobility in agitated Bingham liquids. Ind. Engng Chem. Res. 48 (4), 2266–2274.CrossRefGoogle Scholar
Escudier, M. P., Poole, R. J., Presti, F., Dales, C., Nouar, C., Desaubry, C., Graham, L. & Pullum, L. 2005 Observations of asymmetrical flow behaviour in transitional pipe flow of yield-stress and other shear-thinning liquids. J. Non-Newtonian Fluid Mech. 127 (2–3), 143–155.CrossRefGoogle Scholar
Esmael, A. & Nouar, C. 2008 Transitional flow of a yield-stress fluid in a pipe: evidence of a robust coherent structure. Phys. Rev. E 77 (5), 057302.Google Scholar
Fornari, W., Formenti, A., Picano, F. & Brandt, L. 2016a The effect of particle density in turbulent channel flow laden with finite size particles in semi-dilute conditions. Phys. Fluids 28 (3), 033301.CrossRefGoogle Scholar
Fornari, W., Kazerooni, H. T., Hussong, J. & Brandt, L. 2018 Suspensions of finite-size neutrally buoyant spheres in turbulent duct flow. J. Fluid Mech. 851, 148–186.CrossRefGoogle Scholar
Fornari, W., Picano, F., Sardina, G. & Brandt, L. 2016b Reduced particle settling speed in turbulence. J. Fluid Mech. 808, 153–167.CrossRefGoogle Scholar
Frigaard, I. & Nouar, C. 2003 On three-dimensional linear stability of Poiseuille flow of Bingham fluids. Phys. Fluids 15 (10), 2843–2851.CrossRefGoogle Scholar
Fukagata, K., Iwamoto, K. & Kasagi, N. 2002 Contribution of Reynolds stress distribution to the skin friction in wall-bounded flows. Phys. Fluids 14 (11), L73–L76.CrossRefGoogle Scholar
Gao, H., Li, H. & Wang, L.-P. 2013 Lattice Boltzmann simulation of turbulent flow laden with finite-size particles. Comput. Maths Appl. 65 (2), 194–210.CrossRefGoogle Scholar
Glowinski, R., Pan, T.-W., Hesla, T. I. & Joseph, D. D. 1999 A distributed Lagrange multiplier/fictitious domain method for particulate flows. Intl J. Multiphase Flow 25 (5), 755–794.CrossRefGoogle Scholar
Güzel, B., Frigaard, I. & Martinez, D. M. 2009 Predicting laminar–turbulent transition in Poiseuille pipe flow for non-Newtonian fluids. Chem. Engng Sci. 64 (2), 254–264.CrossRefGoogle Scholar
He, Y. B., Laskowski, J. S. & Klein, B. 2001 Particle movement in non-Newtonian slurries: the effect of yield stress on dense medium separation. Chem. Engng Sci. 56 (9), 2991–2998.CrossRefGoogle Scholar
Lin, Z., Yu, Z., Shao, X. & Wang, L.-P. 2017a Effects of finite-size neutrally buoyant particles on the turbulent flows in a square duct. Phys. Fluids 29 (10), 103304.CrossRefGoogle Scholar
Lin, Z.-w., Shao, X.-m., Yu, Z.-s. & Wang, L.-p. 2017b Effects of finite-size heavy particles on the turbulent flows in a square duct. J. Hydrodyn. 29 (2), 272–282.CrossRefGoogle Scholar
Lucci, F., Ferrante, A. & Elghobashi, S. 2010 Modulation of isotropic turbulence by particles of Taylor length-scale size. J. Fluid Mech. 650, 5–55.CrossRefGoogle Scholar
Min, T., Choi, H. & Yoo, J. Y. 2003 Maximum drag reduction in a turbulent channel flow by polymer additives. J. Fluid Mech. 492, 91–100.CrossRefGoogle Scholar
Nouar, C., Kabouya, N., Dusek, J. & Mamou, M. 2007 Modal and non-modal linear stability of the plane Bingham Poiseuille flow. J. Fluid Mech. 577 (577), 211–239.CrossRefGoogle Scholar
Ohta, T. & Miyashita, M. 2014 DNS and LES with an extended Smagorinsky model for wall turbulence in non-Newtonian viscous fluids. J. Non-Newtonian Fluid Mech. 206, 29–39.CrossRefGoogle Scholar
Peixinho, J., Nouar, C., Desaubry, C. & Théron, B. 2005 Laminar transitional and turbulent flow of yield stress fluid in a pipe. J. Non-Newtonian Fluid Mech. 128 (2–3), 172–184.CrossRefGoogle Scholar
Peng, C. & Wang, L.-P. 2019 Direct numerical simulations of turbulent pipe flow laden with finite-size neutrally buoyant particles at low flow Reynolds number. Acta Mech. 230 (2), 517–539.CrossRefGoogle Scholar
Peysson, Y. 2004 Solid/liquid dispersions in drilling and production. Oil Gas Sci. Technol. 59 (1), 11–21.CrossRefGoogle Scholar
Picano, F., Breugem, W.-P. & Brandt, L. 2015 Turbulent channel flow of dense suspensions of neutrally buoyant spheres. J. Fluid Mech. 764, 463–487.CrossRefGoogle Scholar
Pope, S. B. 2000 Turbulent Flows. Cambridge University Press.CrossRefGoogle Scholar
Putz, A. & Frigaard, I. A. 2010 Creeping flow around particles in a Bingham fluid. J. Non-Newtonian Fluid Mech. 165 (5–6), 263–280.CrossRefGoogle Scholar
Rosti, M. E., Izbassarov, D., Tammisola, O., Hormozi, S. & Brandt, L. 2018 Turbulent channel flow of an elastoviscoplastic fluid. J. Fluid Mech. 853, 488–514.CrossRefGoogle Scholar
Rouson, D. W. I. & Eaton, J. K. 2001 On the preferential concentration of solid particles in turbulent channel flow. J. Fluid Mech. 428, 149–169.CrossRefGoogle Scholar
Rudman, M., Blackburn, H. M., Graham, L. J. W. & Pullum, L. 2004 Turbulent pipe flow of shear-thinning fluids. J. Non-Newtonian Fluid Mech. 118 (1), 33–48.CrossRefGoogle Scholar
Saramito, P. & Wachs, A. 2017 Progress in numerical simulation of yield stress fluid flows. Rheol. Acta 56 (3), 211–230.CrossRefGoogle Scholar
Shao, X., Wu, T. & Yu, Z. 2012 Fully resolved numerical simulation of particle-laden turbulent flow in a horizontal channel at a low Reynolds number. J. Fluid Mech. 693, 319–344.CrossRefGoogle Scholar
Shokri, R., Ghaemi, S., Nobes, D. S. & Sanders, R. S. 2017 Investigation of particle-laden turbulent pipe flow at high-Reynolds-number using particle image/tracking velocimetry (PIV/PTV). Intl J. Multiphase Flow 89, 136–149.CrossRefGoogle Scholar
Singh, J., Rudman, M. & Blackburn, H. M. 2017 The effect of yield stress on pipe flow turbulence for generalised Newtonian fluids. J. Non-Newtonian Fluid Mech. 249, 53–62.CrossRefGoogle Scholar
Sureshkumar, R., Beris, A. N. & Handler, R. A. 1997 Direct numerical simulation of the turbulent channel flow of a polymer solution. Phys. Fluids 9 (3), 743–755.CrossRefGoogle Scholar
Tsuji, Y., Morikawa, Y. & Shiomi, H. 1984 LDV measurements of an air–solid two-phase flow in a vertical pipe. J. Fluid Mech. 139, 417–434.CrossRefGoogle Scholar
Uhlmann, M. 2008 Interface-resolved direct numerical simulation of vertical particulate channel flow in the turbulent regime. Phys. Fluids 20 (5), 053305.CrossRefGoogle Scholar
Wachs, A. & Frigaard, I. A. 2016 Particle settling in yield stress fluids: limiting time, distance and applications. J. Non-Newtonian Fluid Mech. 238, 189–204.CrossRefGoogle Scholar
Wang, G., Abbas, M. & Climent, É. 2017 Modulation of large-scale structures by neutrally buoyant and inertial finite-size particles in turbulent Couette flow. Phys. Rev. Fluids 2 (8), 084302.CrossRefGoogle Scholar
Wang, L.-P., Peng, C., Guo, Z. & Yu, Z. 2016 Flow modulation by finite-size neutrally buoyant particles in a turbulent channel flow. J. Fluids Engng 138 (4), 041306.Google Scholar
Wu, T.-h., Shao, X.-m. & Yu, Z.-s. 2011 Fully resolved numerical simulation of turbulent pipe flows laden with large neutrally-buoyant particles. J. Hydrodyn. 23 (1), 21–25.CrossRefGoogle Scholar
Yu, W., Vinkovic, I. & Buffat, M. 2016a Finite-size particles in turbulent channel flow: quadrant analysis and acceleration statistics. J. Turbul. 17 (11), 1048–1071.CrossRefGoogle Scholar
Yu, Z., Lin, Z., Shao, X. & Wang, L. P. 2016b A parallel fictitious domain method for the interface-resolved simulation of particle-laden flows and its application to the turbulent channel flow. Engng Appl. Comput. Fluid Mech. 10 (1), 160–170.Google Scholar
Yu, Z., Lin, Z., Shao, X. & Wang, L.-P. 2017 Effects of particle–fluid density ratio on the interactions between the turbulent channel flow and finite-size particles. Phys. Rev. E 96 (3), 033102.Google ScholarPubMed
Yu, Z. & Shao, X. 2007 A direct-forcing fictitious domain method for particulate flows. J. Comput. Phys. 227 (1), 292–314.CrossRefGoogle Scholar
Yu, Z. & Wachs, A. 2007 A fictitious domain method for dynamic simulation of particle sedimentation in Bingham fluids. J. Non-Newtonian Fluid Mech. 145 (2–3), 78–91.CrossRefGoogle Scholar
Zhou, J., Adrian, R. J., Balachandar, S. & Kendall, T. M. 1999 Mechanisms for generating coherent packets of hairpin vortices in channel flow. J. Fluid Mech. 387, 353–396.CrossRefGoogle Scholar