Hostname: page-component-745bb68f8f-5r2nc Total loading time: 0 Render date: 2025-01-25T20:16:42.543Z Has data issue: false hasContentIssue false
  • English
  • Français

Interface models for three-dimensional Rayleigh–Taylor instability

Published online by Cambridge University Press:  16 March 2023

Gavin Pandya Open the ORCID record for Gavin Pandya [Opens in a new window]  and
Steve Shkoller Open the ORCID record for Steve Shkoller [Opens in a new window]
Gavin Pandya*
Affiliation:
Department of Mathematics, University of California, Davis, One Shields Ave, Davis, CA 95616, USA
Steve Shkoller*
Affiliation:
Department of Mathematics, University of California, Davis, One Shields Ave, Davis, CA 95616, USA
*
†Email addresses for correspondence: [email protected], [email protected]
†Email addresses for correspondence: [email protected], [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

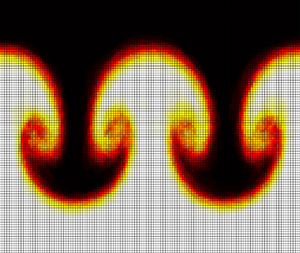
We derive interface models for three-dimensional Rayleigh–Taylor instability (RTI), making use of a novel asymptotic expansion in the non-locality of the fluid flow. These interface models are derived for the purpose of studying universal features associated with RTI such as the Froude number in single-mode RTI, the predicted quadratic growth of the interface amplitude under multi-mode random perturbations, the optimal (viscous) mixing rates induced by the RTI and the self-similarity of horizontally averaged density profiles and the remarkable stabilization of the mixing layer growth rate which arises for the three-fluid two-interface heavy–light–heavy configuration, in which the addition of a third fluid bulk slows the growth of the mixing layer to a linear rate. Our interface models can capture the formation of small-scale structures induced by severe interface roll-up, reproduce experimental data in a number of different regimes and study the effects of multiple interface interactions even as the interface separation distance becomes exceedingly small. Compared with traditional numerical schemes used to study such phenomena, our models provide a computational speed-up of at least two orders of magnitude.

JFM classification

Interfacial Flows (free surface): Interfacial Flows (free surface) Mixing: Mixing Vortex Flows: Vortex Flows
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 959 , 25 March 2023 , A10
Check for updates
Copyright
© The Author(s), 2023. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Albeverio, S. & Cruzeiro, A.B. 1990 Global flows with invariant (Gibbs) measures for Euler and Navier–Stokes two dimensional fluids. Commun. Math. Phys. 129, 431–444.CrossRefGoogle Scholar
Baker, G.R., Meiron, D.I. & Orszag, S.A. 1984 Boundary integral methods for axisymmetric and three-dimensional Rayleigh–Taylor instability problems. Physica D 12 (1–3), 19–31.Google Scholar
Baker, G.R. & Pham, L.D. 2006 A comparison of blob methods for vortex sheet roll-up. J. Fluid Mech. 547, 297–316.CrossRefGoogle Scholar
Beale, J.T. & Majda, A. 1982 Vortex methods. I. Convergence in three dimensions. Maths Comput. 39 (159), 1–27.Google Scholar
Birkhoff, G. 1962 Helmholtz and Taylor instability. Proc. Symp. Appl. maths 13, 55–76.CrossRefGoogle Scholar
Boffetta, G., De Lillo, F. & Musacchio, S. 2010 Nonlinear diffusion model for Rayleigh–Taylor mixing. Phys. Rev. Lett. 104 (3), 034505.Google ScholarPubMed
Cabot, W.H. & Cook, A.W. 2006 Reynolds number effects on Rayleigh–Taylor instability with possible implications for type ia supernovae. Nat. Phys. 2 (8), 562–568.Google Scholar
Canfield, J., Denissen, N., Francois, M., Gore, R., Rauenzahn, R., Reisner, J. & Shkoller, S. 2020 A comparison of interface growth models applied to Rayleigh–Taylor and Richtmyer–Meshkov instabilities. Trans. ASME J. Fluids Engng 142 (12), 121108.CrossRefGoogle Scholar
Chapman, P.R. & Jacobs, J.W. 2006 Experiments on the three-dimensional incompressible Richtmyer–Meshkov instability. Phys. Fluids 18 (7), 074101.CrossRefGoogle Scholar
Cole, R.L. & Tankin, R.S. 1973 Experimental study of Taylor instability. Phys. Fluids 16 (11), 1810–1815.CrossRefGoogle Scholar
Cook, A.W., Cabot, W.H. & Miller, P.L. 2004 The mixing transition in Rayleigh–Taylor instability. J. Fluid Mech. 511, 333–362.CrossRefGoogle Scholar
Daly, B.J. 1967 Numerical study of two fluid Rayleigh–Taylor instability. Phys. Fluids 10 (2), 297–307.CrossRefGoogle Scholar
Emmons, H.W., Chang, C.T. & Watson, B.C. 1960 Taylor instability of finite surface waves. J. Fluid Mech. 7 (2), 177–193.CrossRefGoogle Scholar
Glimm, J., Grove, J.W., Li, X.L., Shyue, K., Zeng, Y. & Zhang, Q. 1998 Three-dimensional front tracking. SIAM J. Sci. Comput. 19 (3), 703–727.CrossRefGoogle Scholar
Goncharov, V.N. 2002 Analytical model of nonlinear, single-mode, classical Rayleigh–Taylor instability at arbitrary Atwood numbers. Phys. Rev. Lett. 88 (13), 134502.CrossRefGoogle ScholarPubMed
Granero-Belinchón, R. & Shkoller, S. 2017 A model for Rayleigh–Taylor mixing and interface turnover. Multiscale Model. Simul. 15 (1), 274–308.Google Scholar
Haan, S.W. 1989 Onset of nonlinear saturation for Rayleigh–Taylor growth in the presence of a full spectrum of modes. Phys. Rev. A 39 (11), 5812.CrossRefGoogle ScholarPubMed
Hirt, C.W. & Nichols, B.D. 1981 Volume of fluid (VoF) method for the dynamics of free boundaries. J. Comput. Phys. 39 (1), 201–225.CrossRefGoogle Scholar
Jacobs, J.W. & Dalziel, S.B. 2005 Rayleigh–Taylor instability in complex stratifications. J. Fluid Mech. 542, 251–279.CrossRefGoogle Scholar
Krasny, R. 1986 a Desingularization of periodic vortex sheet roll-up. J. Comput. Phys. 65 (2), 292–313.CrossRefGoogle Scholar
Krasny, R. 1986 b A study of singularity formation in a vortex sheet by the point-vortex approximation. J. Fluid Mech. 167, 65–93.CrossRefGoogle Scholar
Lin, Z., Thiffeault, J.-L. & Doering, C.R. 2011 Optimal stirring strategies for passive scalar mixing. J. Fluid Mech. 675, 465–476.CrossRefGoogle Scholar
Liska, R. & Wendroff, B. 2003 Comparison of several difference schemes on 1D and 2D test problems for the Euler equations. SIAM J. Sci. Comput. 25 (3), 995–1017.CrossRefGoogle Scholar
Liu, J. & Xin, Z. 1995 Convergence of vortex methods for weak solutions to the 2-D Euler equations with vortex sheet data. Commun. Pure Appl. Maths 48 (6), 611–628.CrossRefGoogle Scholar
Liu, J. & Xin, Z. 2001 Convergence of the point vortex method for 2-D vortex sheet. Maths Comput. 70 (234), 595–606.CrossRefGoogle Scholar
Lopes-Filho, M.C., Lowengrub, J., Lopes, H.J. & Zheng, Y. 2006 Numerical evidence of nonuniqueness in the evolution of vortex sheets. ESAIM: Math. Model. Numer. Anal. 40 (2), 225–237.CrossRefGoogle Scholar
Mathew, G., Mezić, I. & Petzold, L. 2005 A multiscale measure for mixing. Physica D 211 (1–2), 23–46.CrossRefGoogle Scholar
Ramani, R., Reisner, J. & Shkoller, S. 2019 a A space–time smooth artificial viscosity method with wavelet noise indicator and shock collision scheme. Part 1: the 1-$D$ case. J. Comput. Phys. 387, 81–116.CrossRefGoogle Scholar
Ramani, R., Reisner, J. & Shkoller, S. 2019 b A space–time smooth artificial viscosity method with wavelet noise indicator and shock collision scheme. Part 2: the 2-$D$ case. J. Comput. Phys. 387, 45–80.CrossRefGoogle Scholar
Ramani, R. & Shkoller, S. 2020 A multiscale model for Rayleigh–Taylor and Richtmyer–Meshkov instabilities. J. Comput. Phys. 405, 109177.CrossRefGoogle Scholar
Ramaprabhu, P., Dimonte, G., Young, Y.N., Calder, A.C. & Fryxell, B. 2006 Limits of the potential flow approach to the single-mode Rayleigh–Taylor problem. Phys. Rev. E 74 (6), 066308.CrossRefGoogle Scholar
Read, K.I. 1984 Experimental investigation of turbulent mixing by Rayleigh–Taylor instability. Physica D 12 (1–3), 45–58.CrossRefGoogle Scholar
Reisner, J., Serencsa, J. & Shkoller, S. 2013 A space–time smooth artificial viscosity method for nonlinear conservation laws. J. Comput. Phys. 235, 912–933.CrossRefGoogle Scholar
Ristorcelli, J.R. & Clark, T.T. 2004 Rayleigh–Taylor turbulence: self-similar analysis and direct numerical simulations. J. Fluid Mech. 507, 213–253.CrossRefGoogle Scholar
Rollin, B. & Andrews, M.J. 2013 On generating initial conditions for turbulence models: the case of Rayleigh–Taylor instability turbulent mixing. J. Turbul. 14 (3), 77–106.CrossRefGoogle Scholar
Rott, N. 1956 Diffraction of a weak shock with vortex generation. J. Fluid Mech. 1 (1), 111–128.Google Scholar
Sharp, D.H. 1984 An overview of Rayleigh–Taylor instability. Physica D 12 (1–3), 3–18.CrossRefGoogle Scholar
Székelyhidi, L. 2011 Weak solutions to the incompressible Euler equations with vortex sheet initial data. C. R. Math. 349 (19–20), 1063–1066.CrossRefGoogle Scholar
Thiffeault, J.L. 2012 Using multiscale norms to quantify mixing and transport. Nonlinearity 25 (2), R1.CrossRefGoogle Scholar
Unverdi, S.O. & Tryggvason, G. 1992 A front-tracking method for viscous, incompressible, multi-fluid flows. J. Comput. Phys. 100 (1), 25–37.CrossRefGoogle Scholar
Wilkinson, J.P. & Jacobs, J.W. 2007 Experimental study of the single-mode three-dimensional Rayleigh–Taylor instability. Phys. Fluids 19 (12), 124102.Google Scholar
Youngs, D.L. 1984 Numerical simulation of turbulent mixing by Rayleigh–Taylor instability. Physica D 12 (1–3), 32–44.CrossRefGoogle Scholar
Youngs, D.L. 1989 Modelling turbulent mixing by Rayleigh–Taylor instability. Physica D 37 (1–3), 270–287.CrossRefGoogle Scholar
Youngs, D.L. 2013 The density ratio dependence of self-similar Rayleigh–Taylor mixing. Phil. Trans. R. Soc. A 371.CrossRefGoogle ScholarPubMed
Zhou, Y. 2017 a Rayleigh–Taylor and Richtmyer–Meshkov instability induced flow, turbulence, and mixing. II. Phys. Rep. 723, 1–160.Google Scholar
Zhou, Y. 2017 b Rayleigh–Taylor and Richtmyer–Meshkov instability induced flow, turbulence, and mixing. I. Phys. Rep. 720–722, 1–136.Google Scholar
Zhou, Y., et al. 2021 Rayleigh–Taylor and Richtmyer–Meshkov instabilities: a journey through scales. Physica D 423, 132838.CrossRefGoogle Scholar