Hostname: page-component-586b7cd67f-rcrh6 Total loading time: 0 Render date: 2024-11-24T21:34:59.554Z Has data issue: false hasContentIssue false
  • English
  • Français

Interactions of large-scale structures in the near field of round jets at high Reynolds numbers

Published online by Cambridge University Press:  06 February 2020

Jahnavi Kantharaju Open the ORCID record for Jahnavi Kantharaju [Opens in a new window] ,
Romain Courtier ,
Benjamin Leclaire  and
Laurent Jacquin
Jahnavi Kantharaju*
Affiliation:
DAAA, ONERA, Institut Polytechnique de Paris, 92190 Meudon, France
Romain Courtier
Affiliation:
DAAA, ONERA, Institut Polytechnique de Paris, 92190 Meudon, France
Benjamin Leclaire
Affiliation:
DAAA, ONERA, Institut Polytechnique de Paris, 92190 Meudon, France
Laurent Jacquin
Affiliation:
ONERA, Institut Polytechnique de Paris, F-91123Palaiseau, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

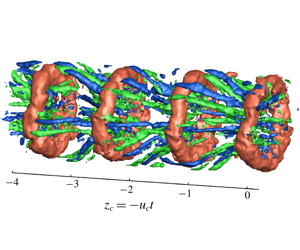
A possible interaction between vortical structures in a round jet shear layer, viz. vortex rings and streamwise vortices, is explored following Davoust et al. (J. Fluid Mech., vol. 709, 2012, pp. 408–444). These authors reported a radial organization of streamwise vorticity in a jet at high diameter-based Reynolds number ($Re$), contrary to the classically observed azimuthal organization. They hypothesized that the observed weaker vortex rings in such jets could be deformed by streamwise vortices and further reoriented and stretched in the streamwise direction. As this hypothesis was based on the observations of one configuration of a jet flow, our study aims at assessing it by varying the key parameter, i.e. the relative strength of the vortex rings and the streamwise vortices, through forcing, along with various jet configurations. We first analyse a low-Mach-number, $Re=1.5\times 10^{5}$ transitional jet, using high-speed stereo particle image velocimetry in a cross-sectional plane at two jet exit diameters from the nozzle exit. The axisymmetric mode is acoustically excited at various amplitudes to increase the strength of the rings relative to streamwise vortices, at a Strouhal number ($St$) of 0.49, the most energetic frequency in the unforced jet. Starting from a radial array in the unexcited jet, a gradual shift towards an azimuthal configuration is obtained with increasing excitation level. Quantification of the relative strengths of the streamwise vortices and vortex rings confirms that a radial array is observed whenever the streamwise vortices are more intense than the rings, and conversely for the azimuthal configuration. We then extend the analysis to other jet cases in terms of $Re$, $St$ and state of the exiting boundary layer. We observe that the correlation between the radial or azimuthal organization of streamwise vortices and the relative strengths of the vortical structures holds systematically, confirming the possibility of the proposed interaction. A detailed analysis of the forced jets also sheds light on some interesting effects of acoustic excitation on the vortical organization in round jets.

JFM classification

Turbulent Flows: Shear layer turbulence Wakes/Jets: Jets Wakes/Jets: Shear layers
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 888 , 10 April 2020 , A8
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Berkooz, G., Holmes, P. & Lumley, J. L. 1993 The proper orthogonal decomposition in the analysis of turbulent flows. Annu. Rev. Fluid Mech. 25 (1), 539–575.CrossRefGoogle Scholar
Bernal, L. P. & Roshko, A. 1986 Streamwise vortex structure in plane mixing layers. J. Fluid Mech. 170, 499–525.CrossRefGoogle Scholar
Bogey, C. & Bailly, C. 2010 Influence of nozzle-exit boundary-layer conditions on the flow and acoustic fields of initially laminar jets. J. Fluid Mech. 663, 507–538.CrossRefGoogle Scholar
Brancher, P., Chomaz, J. M. & Huerre, P. 1994 Direct numerical simulations of round jets: vortex induction and side jets. Phys. Fluids 6 (5), 1768–1774.CrossRefGoogle Scholar
Burattini, P., Antonia, R. A., Rajagopalan, S. & Stephens, M. 2004 Effect of initial conditions on the near-field development of a round jet. Exp. Fluids 37 (1), 56–64.CrossRefGoogle Scholar
Champagnat, F., Plyer, A., Le Besnerais, G., Leclaire, B., Davoust, S. & Le Sant, Y. 2011 Fast and accurate PIV computation using highly parallel iterative correlation maximization. Exp. Fluids 50 (4), 1169.CrossRefGoogle Scholar
Citriniti, J. H. & George, W. K. 2000 Reconstruction of the global velocity field in the axisymmetric mixing layer utilizing the proper orthogonal decomposition. J. Fluid Mech. 418, 137–166.CrossRefGoogle Scholar
Comte, P., Silvestrini, J. H. & Bégou, P. 1998 Streamwise vortices in large-eddy simulations of mixing layers. Eur. J. Mech. (B/Fluids) 17 (4), 615–637.CrossRefGoogle Scholar
Courtier, R.2014 Influence of initial conditions on dynamics of large scales in turbulent jets. PhD thesis, École Polytechnique.Google Scholar
Crow, S. C. J. & Champagne, F. H. 1971 Orderly structure in jet turbulence. J. Fluid Mech. 48 (3), 547–591.CrossRefGoogle Scholar
Davoust, S. & Jacquin, L. 2011 Taylor’s hypothesis convection velocities from mass conservation equation. Phys. Fluids 23 (5), 051701.CrossRefGoogle Scholar
Davoust, S., Jacquin, L. & Leclaire, B. 2012 Dynamics of m = 0 and m = 1 modes and of streamwise vortices in a turbulent axisymmetric mixing layer. J. Fluid Mech. 709, 408–444.CrossRefGoogle Scholar
Glauser, M. N., Leib, S. J. & George, W. K. 1987 Coherent structures in the axisymmetric turbulent jet mixing layer. In Turbulent Shear Flows 5, pp. 134–145. Springer.CrossRefGoogle Scholar
Grinstein, F. F., Gutmark, E. J., Parr, T. P., Hanson-Parr, D. M. & Obeysekare, U. 1996 Streamwise and spanwise vortex interaction in an axisymmetric jet. A computational and experimental study. Phys. Fluids 8 (6), 1515–1524.CrossRefGoogle Scholar
Ho, C. M. & Huerre, P. 1984 Perturbed free shear layers. Annu. Rev. Fluid Mech. 16 (1), 365–422.CrossRefGoogle Scholar
Holmes, P., Lumley, J. L., Berkooz, G. & Rowley, C. W. 2012 Turbulence, Coherent Structures, Dynamical Systems and Symmetry. Cambridge University Press.CrossRefGoogle Scholar
Huang, L. S. & Ho, C. M. 1990 Small-scale transition in a plane mixing layer. J. Fluid Mech. 210, 475–500.CrossRefGoogle Scholar
Hussain, A. K. M. F. & Reynolds, W. C. 1970 The mechanics of an organized wave in turbulent shear flow. J. Fluid Mech. 41 (2), 241–258.CrossRefGoogle Scholar
Hussain, A. K. M. F. & Zaman, K. B. M. Q. 1981 The preferred mode of the axisymmetric jet. J. Fluid Mech. 110, 39–71.CrossRefGoogle Scholar
Hussain, A. K. M. F. & Zedan, M. F. 1978 Effects of the initial condition on the axisymmetric free shear layer: effects of the initial momentum thickness. Phys. Fluids 21 (7), 1100–1112.CrossRefGoogle Scholar
Jung, D., Gamard, S. & George, W. K. 2004 Downstream evolution of the most energetic modes in a turbulent axisymmetric jet at high Reynolds number. Part 1. The near-field region. J. Fluid Mech. 514, 173–204.CrossRefGoogle Scholar
Kovasznay, L. S. G. 1978 Measurement in intermittent and periodic flow. In Proceedings of the Dynamic Flow Conference 1978 on Dynamic Measurements in Unsteady Flows, pp. 133–159. Springer.CrossRefGoogle Scholar
Lasheras, J. C. & Choi, H. 1988 Three-dimensional instability of a plane free shear layer: an experimental study of the formation and evolution of streamwise vortices. J. Fluid Mech. 189, 53–86.CrossRefGoogle Scholar
Liepmann, D. & Gharib, M. 1992 The role of streamwise vorticity in the near-field entrainment of round jets. J. Fluid Mech. 245, 643–668.CrossRefGoogle Scholar
Lin, S. J. & Corcos, G. M. 1984 The mixing layer: deterministic models of a turbulent flow. Part 3. The effect of plane strain on the dynamics of streamwise vortices. J. Fluid Mech. 141, 139–178.CrossRefGoogle Scholar
Martin, J. E. & Meiburg, E. 1991 Numerical investigation of three-dimensionally evolving jets subject to axisymmetric and azimuthal perturbations. J. Fluid Mech. 230, 271–318.CrossRefGoogle Scholar
Michalke, A. 1984 Survey on jet instability theory. Prog. Aerosp. Sci. 21, 159–199.CrossRefGoogle Scholar
Monkewitz, P. A. & Pfizenmaier, E. 1991 Mixing by side jets” in strongly forced and self-excited round jets. Phys. Fluids A 3 (5), 1356–1361.CrossRefGoogle Scholar
Nogueira, P. A. S., Cavalieri, A. V. G., Jordan, P. & Jaunet, V. 2019 Large-scale streaky structures in turbulent jets. J. Fluid Mech. 873, 211–237.CrossRefGoogle Scholar
Pierrehumbert, R. T. & Widnall, S. E. 1982 The two-and three-dimensional instabilities of a spatially periodic shear layer. J. Fluid Mech. 114, 59–82.CrossRefGoogle Scholar
Rogers, M. M. & Moin, P. 1987 The structure of the vorticity field in homogeneous turbulent flows. J. Fluid Mech. 176, 33–66.CrossRefGoogle Scholar
Sadeghi, H. & Pollard, A. 2012 Effects of passive control rings positioned in the shear layer and potential core of a turbulent round jet. Phys. Fluids 24 (11), 115103.CrossRefGoogle Scholar
Samet, M. M. & Petersen, R. A. 1988 Effects of excitation level on the stability of an axisymmetric mixing layer. Phys. Fluids 31 (11), 3246–3252.CrossRefGoogle Scholar
Shaabani-Ardali, L., Sipp, D. & Lesshafft, L. 2019 Vortex pairing in jets as a global floquet instability: modal and transient dynamics. J. Fluid Mech. 862, 951–989.CrossRefGoogle Scholar
Taylor, G. I. 1938 The spectrum of turbulence. Proc. R. Soc. Lond. A 164 (919), 476–490.CrossRefGoogle Scholar
Tinney, C. E., Glauser, M. N. & Ukeiley, L. S. 2008 Low-dimensional characteristics of a transonic jet. Part 1. Proper orthogonal decomposition. J. Fluid Mech. 612, 107–141.CrossRefGoogle Scholar
Towne, A., Schmidt, O. T. & Colonius, T. 2018 Spectral proper orthogonal decomposition and its relationship to dynamic mode decomposition and resolvent analysis. J. Fluid Mech. 847, 821–867.CrossRefGoogle Scholar
Verzicco, R. & Orlandi, P. 1994 Direct simulations of the transitional regime of a circular jet. Phys. Fluids 6 (2), 751–759.CrossRefGoogle Scholar
Widnall, S. E. & Sullivan, J. P. 1973 On the stability of vortex rings. Proc. R. Soc. Lond. A 332 (1590), 335–353.Google Scholar
Zaman, K. B. M. Q. 1985 Far-field noise of a subsonic jet under controlled excitation. J. Fluid Mech. 152, 83–111.CrossRefGoogle Scholar
Zaman, K. B. M. Q. 2012 Effect of initial boundary-layer state on subsonic jet noise. AIAA J. 50 (8), 1784–1795.CrossRefGoogle Scholar
Zaman, K. B. M. Q. & Hussain, A. K. M. F. 1980 Vortex pairing in a circular jet under controlled excitation. Part 1. General jet response. J. Fluid Mech. 101 (03), 449–491.CrossRefGoogle Scholar