Hostname: page-component-586b7cd67f-t7fkt Total loading time: 0 Render date: 2024-12-01T00:47:11.680Z Has data issue: false hasContentIssue false
  • English
  • Français

Insights into the dynamics of spray–swirl interactions

Published online by Cambridge University Press:  24 November 2016

Kuppuraj Rajamanickam  and
Saptarshi Basu
Kuppuraj Rajamanickam
Affiliation:
Department of Mechanical Engineering, Indian Institute of Science, Bangalore-560012, India
Saptarshi Basu*
Affiliation:
Department of Mechanical Engineering, Indian Institute of Science, Bangalore-560012, India
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The near-field breakup and interaction of a hollow-cone liquid sheet with coannular swirling air flow have been examined using high-speed diagnostics. Time-resolved PIV (particle image velocimetry; 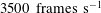$3500~\text{frames}~\text{s}^{-1}$) is employed to capture the spatio-temporal behaviour of the swirling air flow field. The combined liquid–gas phase interaction is visualized with the help of high-speed (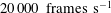$20\,000~\text{frames}~\text{s}^{-1}$) shadowgraphy. In this study, the transition from weak to strong spray–swirl interaction is explained based on the momentum ratio. Proper orthogonal decomposition (POD) is implemented on instantaneous PIV and shadowgraphy images to extract the energetic spatial eigenmodes and characteristic modal frequencies. The POD results suggest the dominance of the KH (Kelvin–Helmholtz) instability mechanism (pure axial shear, axial plus azimuthal shear) in swirl–spray interaction. In addition, linear stability analysis also shows the destabilization of the liquid–air interface caused by KH waves ($\unicode[STIX]{x1D706}_{p}$), which arises from the formation of a vorticity layer of thickness 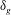$\unicode[STIX]{x1D6FF}_{g}$ near the liquid–air interface. The frequency values obtained from the primary KH wavelength (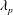$\unicode[STIX]{x1D706}_{p}$) exhibit good agreement with the POD modal frequencies. Scaling laws are proposed to elucidate the relationships between the global length scales (breakup length, spray spread) and the primary wavelength. The breakup length scale and liquid sheet oscillations are meticulously analysed in the time domain to reveal the breakup dynamics of the liquid sheet. Furthermore, the large-scale coherent structures of the swirl flow exhibit different sheet breakup phenomena in the spatial domain. For instance, flapping breakup is induced by the central toroidal recalculation zone in the swirling flow field. Finally, the ligament formation mechanism and its diameter, i.e. the size of first-generation droplets, are measured with phase Doppler interferometry. The measured sizes scale reasonably with KH waves.

JFM classification

Drops and Bubbles: Breakup/coalescence Drops and Bubbles: Drops and Bubbles Multiphase and Particle-laden Flows: Gas/liquid flow
Type
Papers
Information
Journal of Fluid Mechanics , Volume 810 , 10 January 2017 , pp. 82 - 126
Check for updates
Copyright
© 2016 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Albrecht, H.-E., Damaschke, N., Borys, M. & Tropea, C. 2013 Laser Doppler and Phase Doppler Measurement Techniques. Springer.Google Scholar
Anacleto, P., Fernandes, E., Heitor, M. & Shtork, S. 2002 Characterization of a strong swirling flow with precessing vortex core based on measurements of velocity and local pressure fluctuations. In 10th International Symposium on Applications of Laser Techniques to Fluid Mechanics. Springer.Google Scholar
Archer, S. & Gupta, A. K. 2003 Effect of swirl and combustion on flow dynamics in lean direct injection gas turbine combustion. In 41st AIAA Aerospace Sciences Meeting, Reno, NV. AIAA.Google Scholar
Bachalo, W. D. 1980 Method for measuring the size and velocity of spheres by dual-beam light-scatter interferometry. Appl. Opt. 19, 363–370.Google Scholar
Bachalo, W. D. & Houser, M. J. 1984 Phase/Doppler spray analyzer for simultaneous measurements of drop size and velocity distributions. Opt. Engng 23, 235583.CrossRefGoogle Scholar
Beér, J. M. & Chigier, N. A. 1972 Combustion Aerodynamics. Applied Science.Google Scholar
Ben Rayana, F. 2007 Contribution à l’étude des instabilités interfaciales liquide–gaz en atomisation assistée et taille de gouttes. INPG.Google Scholar
Betchov, R. & Szewczyk, A. 1963 Stability of a shear layer between parallel streams. Phys. Fluids 6, 1391–1396.Google Scholar
Billant, P., Chomaz, J. & Huerre, P. 1998 Experimental study of vortex breakdown in swirling jets. J. Fluid Mech. 376, 183–219.Google Scholar
Boeck, T. & Zaleski, S. 2005 Viscous versus inviscid instability of two-phase mixing layers with continuous velocity profile. Phys. Fluids 17, 32106.Google Scholar
Brown, G. L. & Roshko, A. 1974 On density effects and large structure in turbulent mixing layers. J. Fluid Mech. 64, 775–816.CrossRefGoogle Scholar
Castleman, R. A.1931 The mechanism of the atomization of liquids. US Department of Commerce, Bureau of Standards.Google Scholar
Cebeci, T. & Bradshaw, P. 1977 Momentum Transfer in Boundary Layers. Hemisphere.Google Scholar
Champagne, F. H. & Kromat, S. 2000 Experiments on the formation of a recirculation zone in swirling coaxial jets. Exp. Fluids 29, 494–504.Google Scholar
Chandrasekhar, S. 2013 Hydrodynamic and Hydromagnetic Stability. Courier Corporation.Google Scholar
Chigier, N. A. & Chervinsky, A. 1967 Experimental investigation of swirling vortex motion in jets. Trans. ASME J. Appl. Mech. 34, 443–451.CrossRefGoogle Scholar
Coles, D. 1965 Transition in circular Couette flow. J. Fluid Mech. 21, 385–425.CrossRefGoogle Scholar
Dimotakis, P. E. 1986 Two-dimensional shear-layer entrainment. AIAA J. 24, 1791–1796.Google Scholar
Dombrowski, N. & Johns, W. R. 1963 The aerodynamic instability and disintegration of viscous liquid sheets. Chem. Engng Sci. 18, 203–214.CrossRefGoogle Scholar
Duke, D., Honnery, D. & Soria, J. 2010 A cross-correlation velocimetry technique for breakup of an annular liquid sheet. Exp. Fluids 49, 435–445.Google Scholar
Engelbert, C., Hardalupas, Y. & Whitelaw, J. H. 1995 Breakup phenomena in coaxial airblast atomizers. Proc. R. Soc. Lond. A 451, 189–229.Google Scholar
Fraser, R. P., Dombrowski, N. & Routley, J. H. 1963 The atomization of a liquid sheet by an impinging air stream. Chem. Engng Sci. 18, 339–353.Google Scholar
Fuster, D., Matas, J.-P., Marty, S., Popinet, S., Hoepffner, J., Cartellier, A. & Zaleski, S. 2013 Instability regimes in the primary breakup region of planar coflowing sheets. J. Fluid Mech. 736, 150–176.Google Scholar
Gallaire, F. & Chomaz, J.-M. 2003 Instability mechanisms in swirling flows. Phys. Fluids 15, 2622–2639.Google Scholar
Gaster, M. 1962 A note on the relation between temporally-increasing and spatially-increasing disturbances in hydrodynamic stability. J. Fluid Mech. 14, 222–224.Google Scholar
Gu, X., Basu, S. & Kumar, R. 2012 Dispersion and vaporization of biofuels and conventional fuels in a crossflow pre-mixer. Intl J. Heat Mass Transfer 55, 336–346.Google Scholar
Gupta, A. K., Lilley, D. G. & Syred, N. 1984 Swirl Flows, vol. 488, p. 1. Abacus Press.Google Scholar
Hagerty, W. W. & Shea, J. F. 1955 A study of the stability of plane fluid sheets. Trans. ASME J. Appl. Mech. 22, 509–514.Google Scholar
Holmes, P., Lumley, J. L. & Berkooz, G. 1998 Turbulence, Coherent Structures, Dynamical Systems and Symmetry. Cambridge University Press.Google Scholar
Hopfinger, E. J. & Lasheras, J. C. 1996 Explosive breakup of a liquid jet by a swirling coaxial gas jet. Phys. Fluids 8, 1696–1698.Google Scholar
Hoyt, J. W. & Taylor, J. J. 1977 Waves on water jets. J. Fluid Mech. 83, 119–127.Google Scholar
Hussain, A. & Zedan, Mf. 1978 Effects of the initial condition on the axisymmetric free shear layer: effects of the initial momentum thickness. Phys. Fluids 21, 1100–1112.Google Scholar
Ibrahim, A. A. & Jog, M. A. 2006 Effect of liquid and air swirl strength and relative rotational direction on the instability of an annular liquid sheet. Acta Mech. 186, 113–133.CrossRefGoogle Scholar
Jerome, J. J. S., Marty, S., Matas, J.-P., Zaleski, S. & Hoepffner, J. 2013 Vortices catapult droplets in atomization. Phys. Fluids 25, 112109.Google Scholar
Juniper, M. P. & Candel, S. M. 2003 The stability of ducted compound flows and consequences for the geometry of coaxial injectors. J. Fluid Mech. 482, 257–269.CrossRefGoogle Scholar
Kang, D. J. & Lin, S. P. 1989 Breakup of a swirling liquid jet. Intl J. Engng Fluid Mech. 2, 47–62.Google Scholar
Keane, R. D. & Adrian, R. J. 1990 Optimization of particle image velocimeters. I. Double pulsed systems. Meas. Sci. Technol. 1, 1202.CrossRefGoogle Scholar
Lasheras, J. C. & Hopfinger, E. J. 2000 Liquid jet instability and atomization in a coaxial gas stream. Annu. Rev. Fluid Mech. 32, 275–308.CrossRefGoogle Scholar
Lasheras, J. C., Kremer, D. M., Berchielli, A. & Connolly, E. K. 2008 Atomization of viscous and non-Newtonian liquids by a coaxial, high-speed gas jet. Experiments and droplet size modeling. Intl J. Multiphase Flow 34, 161–175.Google Scholar
Lasheras, J. C., Villermaux, E. & Hopfinger, E. J. 1998 Break-up and atomization of a round water jet by a high-speed annular air jet. J. Fluid Mech. 357, 351–379.Google Scholar
Lefebvre, A. H.1966 Fuel injection system for gas turbine engines. US Patent US3283502 A.Google Scholar
Lian, Z. W. & Lin, S. P. 1990 Breakup of a liquid jet in a swirling gas. Phys. Fluids 2, 2134–2139.CrossRefGoogle Scholar
Liang, H. & Maxworthy, T. 2005 An experimental investigation of swirling jets. J. Fluid Mech. 525, 115–159.Google Scholar
Liao, Y., Jeng, S. M., Jog, M. A. & Benjamin, M. A. 2000 The effect of air swirl profile on the instability of a viscous liquid jet. J. Fluid Mech. 424, 1–20.Google Scholar
Lilley, D. G. 1977 Swirl flows in combustion: a review. AIAA J. 15, 1063–1078.Google Scholar
Lin, C. C. 1966 The Theory of Hydrodynamic Stability. Cambridge University Press.Google Scholar
Lozano, A., Barreras, F., Siegler, C. & Löw, D. 2005 The effects of sheet thickness on the oscillation of an air-blasted liquid sheet. Exp. Fluids 39, 127–139.Google Scholar
Lozano, A., Garcıa-Olivares, A. & Dopazo, C. 1998 The instability growth leading to a liquid sheet breakup. Phys. Fluids 10, 2188–2197.Google Scholar
Marek, C. J., Smith, T. D. & Kundu, K. 2005 Low emission hydrogen combustors for gas turbines using lean direct injection. In Proceedings of the 41st Joint Propulsion Conference, Tucson, AZ, July, pp. 10–13. AIAA.Google Scholar
Marmottant, P. & Villermaux, E. 2004 On spray formation. J. Fluid Mech. 498, 73–111.Google Scholar
Matas, J.-P. & Cartellier, A. 2013 Flapping instability of a liquid jet. C. R. Méc. 341, 35–43.Google Scholar
Matas, J.-P., Marty, S. & Cartellier, A. 2011 Experimental and analytical study of the shear instability of a gas–liquid mixing layer. Phys. Fluids 23, 94112.Google Scholar
Mayer, W. O. H. 1994 Coaxial atomization of a round liquid jet in a high speed gas stream: a phenomenological study. Exp. Fluids 16, 401–410.Google Scholar
Metcalfe, R. W., Orszag, S. A., Brachet, M. E., Menon, S. & Riley, J. J. 1987 Secondary instability of a temporally growing mixing layer. J. Fluid Mech. 184, 207–243.Google Scholar
Monkewitz, P. A. & Huerre, P. 1982 Influence of the velocity ratio on the spatial instability of mixing layers. Phys. Fluids 25, 1137–1143.Google Scholar
Mugele, R. A. & Evans, H. D. 1951 Droplet size distribution in sprays. Ind. Engng Chem. 43, 1317–1324.Google Scholar
Panchagnula, M. V., Sojka, P. E. & Santangelo, P. J. 1996 On the three-dimensional instability of a swirling, annular, inviscid liquid sheet subject to unequal gas velocities. Phys. Fluids 8, 3300–3312.Google Scholar
Park, J., Huh, K. Y., Li, X. & Renksizbulut, M. 2004 Experimental investigation on cellular breakup of a planar liquid sheet from an air-blast nozzle. Phys. Fluids 16, 625–632.Google Scholar
Raffel, M., Willert, C. E., Wereley, S. & Kompenhans, J. 2013 Particle Image Velocimetry: A Practical Guide. Springer.Google Scholar
Lord Rayleigh 1878 On the instability of jets. Proc. Lond. Math. Soc. 1, 4–13.Google Scholar
Lord Rayleigh 1880 On the stability, or instability, of certain fluid motions. Proc. Lond. Math. Soc. 11, 57.Google Scholar
Raynal, L., Villermaux, E., Lasheras, J. C. & Hopfinger, E. J. 1997 Primary instability in liquid–gas shear layers. In 11th Symposium on Turbulent Shear Flows, Grenoble, France, pp. 27.1–27.5.Google Scholar
Rehab, H., Villermaux, E. & Hopfinger, E. J. 1997 Flow regimes of large-velocity-ratio coaxial jets. J. Fluid Mech. 345, 357–381.Google Scholar
Saha, A., Lee, J. D., Basu, S. & Kumar, R. 2012 Breakup and coalescence characteristics of a hollow cone swirling spray. Phys. Fluids 24, 124103.CrossRefGoogle Scholar
Santhosh, R. & Basu, S. 2015 Transitions and blowoff of unconfined non-premixed swirling flame. Combust. Flame 164, 35–52.Google Scholar
Santhosh, R., Miglani, A. & Basu, S. 2013 Transition and acoustic response of recirculation structures in an unconfined co-axial isothermal swirling flow. Phys. Fluids 25, 83603.Google Scholar
Schumaker, S. A. & Driscoll, J. F. 2012 Mixing properties of coaxial jets with large velocity ratios and large inverse density ratios. Phys. Fluids 24, 55101.Google Scholar
Shen, J. & Li, X. 1996 Breakup of annular viscous liquid jets in two gas streams. J. Propul. Power 12, 752–759.Google Scholar
Sirovich, L. 1987 Turbulence and the dynamics of coherent structures. Part I. Coherent structures. Q. Appl. Maths 45, 561–571.Google Scholar
Squire, H. B. 1953 Investigation of the instability of a moving liquid film. Br. J. Appl. Phys. 4, 167.CrossRefGoogle Scholar
Syred, N. 2006 A review of oscillation mechanisms and the role of the precessing vortex core (PVC) in swirl combustion systems. Prog. Energy Combust. Sci. 32, 93–161.Google Scholar
Taylor, G. I. 1963 The shape and acceleration of a drop in a high speed air stream. Sci. Pap. GI Taylor 3, 457–464.Google Scholar
Varga, C. M., Lasheras, J. C. & Hopfinger, E. J. 2003 Initial breakup of a small-diameter liquid jet by a high-speed gas stream. J. Fluid Mech. 497, 405–434.CrossRefGoogle Scholar
Villermaux, E. & Hopfinger, E. J. 1994 Periodically arranged co-flowing jets. J. Fluid Mech. 263, 63–92.CrossRefGoogle Scholar
Wahono, S., Honnery, D., Soria, J. & Ghojel, J. 2008 High-speed visualisation of primary break-up of an annular liquid sheet. Exp. Fluids 44, 451–459.CrossRefGoogle Scholar
Wang, S., Yang, V., Hsiao, G., Hsieh, S.-Y. & Mongia, H. C. 2007 Large-eddy simulations of gas-turbine swirl injector flow dynamics. J. Fluid Mech. 583, 1–10.Google Scholar
Weber, C. 1931 Disintegration of liquid jets. Z. Angew Math. Mech. 11, 136–159.Google Scholar
Xianguo, L. & Tankino, R. S. 1987 Droplet size distribution: a derivation of a Nukiyama–Tanasawa type distribution function. Combust. Sci. Technol. 56, 65–76.CrossRefGoogle Scholar
Zhao, H., Liu, H.-F., Tian, X.-S., Xu, J.-L., Li, W.-F. & Lin, K.-F. 2014 Outer ligament-mediated spray formation of annular liquid sheet by an inner round air stream. Exp. Fluids 55, 1–13.Google Scholar

Rajamanickam and Basu movie 1

Instantaneous flow fields for Reg=5089 and 33888

Download Rajamanickam and Basu movie 1(Video)
Video 10.8 MB

Rajamanickam and Basu movie 2

Spray structure for MR=0

Download Rajamanickam and Basu movie 2(Video)
Video 1.2 MB

Rajamanickam and Basu movie 3

Spray structure for MR=338

Download Rajamanickam and Basu movie 3(Video)
Video 1.1 MB

Rajamanickam and Basu movie 4

Spray structure for MR=6100

Download Rajamanickam and Basu movie 4(Video)
Video 1 MB

Rajamanickam and Basu movie 5

Near field Breakup for MR=6100

Download Rajamanickam and Basu movie 5(Video)
Video 1.3 MB