Hostname: page-component-586b7cd67f-rcrh6 Total loading time: 0 Render date: 2024-11-28T06:19:09.009Z Has data issue: false hasContentIssue false
  • English
  • Français

Influence of the velocity field on scalar transport in gaseous transverse jets

Published online by Cambridge University Press:  17 November 2017

L. Gevorkyan ,
T. Shoji ,
W. Y. Peng Open the ORCID record for W. Y. Peng [Opens in a new window]  and
A. R. Karagozian Open the ORCID record for A. R. Karagozian [Opens in a new window]
L. Gevorkyan
Affiliation:
Department of Mechanical and Aerospace Engineering, University of California, Los Angeles, CA 90095-1597, USA
T. Shoji
Affiliation:
Department of Mechanical and Aerospace Engineering, University of California, Los Angeles, CA 90095-1597, USA
W. Y. Peng
Affiliation:
Department of Mechanical and Aerospace Engineering, University of California, Los Angeles, CA 90095-1597, USA
A. R. Karagozian*
Affiliation:
Department of Mechanical and Aerospace Engineering, University of California, Los Angeles, CA 90095-1597, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The present experiments explored the dynamical character of the gaseous jet injected flush into cross-flow for variable jet-to-cross-flow momentum flux ratios 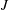$J$ (5, 12 and 41) and density ratios 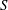$S$ (0.35 and 1.0). Contoured nozzle and straight pipe injectors were studied here, with the jet Reynolds number fixed at 1900 as other flow parameters were varied. Simultaneous acetone planar laser-induced fluorescence (PLIF) imaging and stereo particle image velocimetry (PIV) were used to study the relationships between scalar and velocity/vorticity fields, with a special focus on comparing PLIF-based extraction of scalar dissipation rates and local strain rates with PIV-based local strain rates in the upstream and downstream shear layers of the jet. There was remarkable similarity between the scalar and vorticity fields for the jet in cross-flow, spanning conditions for absolutely unstable upstream jet shear layers at low 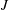$J$ or $S$ values to conditions for convectively unstable shear layers for larger 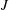$J$, equidensity conditions (Megerian et al., J. Fluid Mech., vol. 593, 2007, pp. 93–129; Getsinger et al., Exp. Fluids, vol. 53, 2012, pp. 783–801). Proper orthogonal decomposition applied to both scalar and velocity fields revealed strengthening instabilities in both the upstream shear layer and in the jet’s wake as 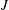$J$ was reduced. The simultaneous measurements allowed PLIF-extracted scalar dissipation rates and strain rates to be determined via a flamelet-like model and compared with PIV-extracted strain rates, each in the diffusion layer-normal direction. There was generally very good qualitative and quantitative agreement for these metrics in both the jet upstream and downstream shear layers for most flow conditions, with excellent correspondence to locations of shear layer vorticity roll up, although downstream shear layer strain rates in some cases showed lesser correspondence between PLIF- and PIV-based data. Such differences are shown to potentially result from diffusion and resolution effects as well as the influence of three-dimensional and transient effects which can be more significant in the lee side of the jet. Nevertheless, the present results reveal interesting dynamics and demonstrate the importance of strain fields in enhanced diffusion and transport phenomena.

JFM classification

Instability: Absolute/convective instability Wakes/Jets: Wakes/Jets
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 834 , 10 January 2018 , pp. 173 - 219
Check for updates
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Adrian, R. J., Durao, D., Durst, F., Heitor, M. V., Maeda, M. & Whitelaw, J. H.(Eds) 2000 Laser Techniques Applied to Fluid Mechanics: Selected Papers from the 9th International Symposium. Springer.Google Scholar
Adrian, R. J. & Westerweel, J. 2011 Particle Image Velocimetry. Cambridge University Press.Google Scholar
Alves, L. S. de B., Kelly, R. E. & Karagozian, A. R. 2008 Transverse-jet shear-layer instabilities. Part 2. Linear analysis for large jet-to-crossflow velocity ratio. J. Fluid Mech. 602, 383–401.Google Scholar
Ashurst, Wm. T., Kerstein, A. R., Kerr, R. M. & Gibson, C. H. 1987 Alignment of vorticity and scalar gradient with strain rate in simulated Navier–Stokes turbulence. Phys. Fluids 30, 2343–2353.Google Scholar
Berkooz, G., Holmes, P. & Lumley, J. L. 1993 The proper orthogonal decomposition in the analysis of turbulent flows. Annu. Rev. Fluid Mech. 25, 539–575.Google Scholar
Bird, R. B., Stewart, W. E. & Lightfoot, E. N. 1960 Transport Phenomena. Wiley.Google Scholar
Bish, E. S. & Dahm, W. J. A. 1995 Strained dissipation and reaction layer analyses of nonequilibrium chemistry in turbulent reacting flows. Combust. Flame 100, 457–464.Google Scholar
Buch, K. A. & Dahm, W. J. A. 1996 Experimental study of the fine-scale structure of conserved scalar mixing in turbulent shear flows. Part 1. Sc ≫ 1. J. Fluid Mech. 317, 21–71.Google Scholar
Buch, K. A. & Dahm, W. J. A. 1998 Experimental study of the fine-scale structure of conserved scalar mixing in turbulent shear flows. Part 2. Sc = 1. J. Fluid Mech. 364, 1–29.Google Scholar
Canzonieri, K.2009 Experimental studies on low density jets in crossflow. Master’s thesis, University of California, Los Angeles.Google Scholar
Chomaz, J.-M. 2005 Global instabilities in spatially developing flows: non-normality and nonlinearity. Annu. Rev. Fluid Mech. 37, 357–392.CrossRefGoogle Scholar
Coriton, B., Steinberg, A. M. & Frank, J. H. 2014 High-speed tomographic PIV and OH PLIF measurements in turbulent reactive flows. Exp. Fluids 55, 1743–1762.Google Scholar
Cortelezzi, L. & Karagozian, A. R. 2001 On the formation of the counter-rotating vortex pair in transverse jets. J. Fluid Mech. 446, 347–373.Google Scholar
Davitian, J., Getsinger, D., Hendrickson, C. & Karagozian, A. R. 2010a Transition to global instability in transverse-jet shear layers. J. Fluid Mech. 661, 294–315.Google Scholar
Davitian, J., Hendrickson, C., Getsinger, D., M’Closkey, R. T. & Karagozian, A. R. 2010b Strategic control of transverse jet shear layer instabilities. AIAA J. 48 (9), 2145–2156.Google Scholar
Dowling, D. R. & Dimotakis, P. E. 1990 Similarity of the concentration field of gas-phase turbulent jets. J. Fluid Mech. 218, 109–141.CrossRefGoogle Scholar
Ekkad, S. V., Ou, S. & Rivir, R. B. 2006 Effect of jet pulsation and duty cycle on film cooling from a single jet on a leading edge model. Trans. ASME J. Turbomach. 128 (3), 564–571.Google Scholar
Fearn, R. & Weston, R. 1974 Vorticity associated with a jet in a crossflow. AIAA J. 12, 1666–1671.Google Scholar
Fric, T. F. & Roshko, A. 1994 Vortical structure in the wake of a transverse jet. J. Fluid Mech. 279, 1–47.Google Scholar
Getsinger, D., Gevorkyan, L., Smith, O. I. & Karagozian, A. R. 2014 Structural and stability characteristics of jets in crossflow. J. Fluid Mech. 760, 342–367.Google Scholar
Getsinger, D. R., Hendrickson, C. & Karagozian, A. R. 2012 Shear layer instabilities in low-density transverse jets. Exp. Fluids 53, 783–801.CrossRefGoogle Scholar
Gevorkyan, L.2015 Structure and mixing characterization of variable density transverse jet flows. PhD thesis, UCLA.Google Scholar
Gevorkyan, L., Shoji, T., Getsinger, D. R., Smith, O. I. & Karagozian, A. R. 2016 Transverse jet mixing characteristics. J. Fluid Mech. 790, 237–274.Google Scholar
Hallberg, M. P. & Strykowski, P. J. 2006 On the universality of global modes in low-density axisymmetric jets. J. Fluid Mech. 569, 493–507.Google Scholar
Hendrickson, C. & M’Closkey, R. 2012 Phase compensation strategies for modulated–demodulated control with application to pulsed jet injection. ASME J. Dyn. Syst. Meas. Control 134, 011024.Google Scholar
Howarth, L. 1948 Concerning the effect of compressibility on laminar boundary layers and their separation. Proc. R. Soc. Lond. A 194 (1036), 16–42.Google Scholar
Huerre, P. & Monkewitz, P. A. 1990 Local and global instabilities in spatially developing flows. Annu. Rev. Fluid Mech. 22, 473–537.Google Scholar
Iyer, P. S. & Mahesh, K. 2016 A numerical study of shear layer characteristics of low-speed transverse jets. J. Fluid Mech. 790, 275–307.Google Scholar
Juniper, M. P., Li, L. K. B. & Nichols, J. W. 2009 Forcing of self-excited round jet diffusion flames. Proc. Combust. Inst. 32, 1191–1198.Google Scholar
Kamotani, Y. & Greber, I. 1972 Experiments on a turbulent jet in a cross flow. AIAA J. 10 (11), 1425–1429.Google Scholar
Karagozian, A. R. 1986 An analytical model for the vorticity associated with a transverse jet. AIAA J. 24, 429–436.Google Scholar
Karagozian, A. R. 2010 Transverse jets and their control. Prog. Energy Combust. Sci. 36, 531–553.Google Scholar
Karagozian, A. R. & Marble, F. E. 1986 Study of a diffusion flame in a stretched vortex. Combust. Sci. Technol. 45, 65–84.Google Scholar
Kelso, R. M., Lim, T. T. & Perry, A. E. 1996 An experimental study of round jets in cross-flow. J. Fluid Mech. 306, 111–144.Google Scholar
Kelso, R. M. & Smits, A. J. 1995 Horseshoe vortex systems resulting from the interaction between a laminar boundary layer and a transverse jet. Phys. Fluids 7, 153–158.Google Scholar
Kerr, R. M. 1985 Higher-order derivative correlations and the alignment of small scale structures in isotropic numerical turbulence. J. Fluid Mech. 153, 31–58.Google Scholar
Kothnur, P. S. & Clemens, N. T. 2005 Effects of unsteady strain rate on scalar dissipation structures in turbulent planar jets. Phys. Fluids 17, 125104.Google Scholar
Krothapalli, A., Lourenco, L. & Buchlin, J. M. 1990 Separated flow upstream of a jet in a crossflow. AIAA J. 28 (3), 414–420.Google Scholar
Kuzo, D. M.1995 An experimental study of the turbulent transverse jet. PhD thesis, California Institute of Technology.Google Scholar
Kyle, D. M. & Sreenivasan, K. R. 1993 The instability and breakdown of a round variable-density jet. J. Fluid Mech. 249, 619–664.Google Scholar
Lozano, A.1992 Laser-excited luminescent tracers for planar concentration measurements in gaseous jets. PhD thesis, Stanford University, Department of Mechanical Engineering.Google Scholar
Lozano, A., Yip, B. & Hanson, R. K. 1992 Acetone: a tracer for concentration measurements in gaseous flows by planar laser-induced fluorescence. Exp. Fluids 13, 369–376.CrossRefGoogle Scholar
Marble, F. E. & Broadwell, J. E.1977 The coherent flame model for turbulent chemical reactions. Project Squid Tech. Rep. TRW-9-PU.CrossRefGoogle Scholar
Margason, R. J.1993 Fifty years of jet in cross flow research. AGARD-CP-534 1, 1–141.Google Scholar
Mathew, G., Mezic, I. & Petzold, L. 2005 A multiscale measure of mixing. Physica D 211 (1), 23–46.Google Scholar
M’Closkey, R. T., King, J., Cortelezzi, L. & Karagozian, A. R. 2002 The actively controlled jet in crossflow. J. Fluid Mech. 452, 325–335.Google Scholar
Megerian, S., Davitian, J., de B. Alves, L. S. & Karagozian, A. R. 2007 Transverse-jet shear-layer instabilities. Part 1. Experimental studies. J. Fluid Mech. 593, 93–129.Google Scholar
Meyer, K. E., Pedersen, J. M. & Özcan, O. 2007 A turbulent jet in crossflow analysed with proper orthogonal decomposition. J. Fluid Mech. 583, 199–227.Google Scholar
Michalke, A. 1984 Survey on jet instability theory. Prog. Aerosp. Sci. 21, 159–199.Google Scholar
Miller, D. N., Yagle, P. J. & Hamstra, J. W.1999 Fluidic throat skewing for thrust vectoring in fixed-geometry nozzles. AIAA P. 99-0365.Google Scholar
Monkewitz, P. A., Lehmann, B., Barsikow, B. & Bechert, D. W. 1989 The spreading of self-excited hot jets by side jets. Phys. Fluids A 1, 446–448.CrossRefGoogle Scholar
Muldoon, F. & Acharya, S. 2010 Direct numerical simulation of pulsed jets in crossflow. Comput. Fluids 39, 1745–1773.Google Scholar
Oh, T. S. & Schetz, J. A. 1990 Finite element simulation of complex jets in a crossflow for v/stol applications. J. Aircraft 27, 389–399.Google Scholar
Peters, N. 1986 Laminar flamelet concepts in turbulent combustion. In Twenty-first Symposium (International) on Combustion, pp. 1231–1250. The Combustion Institute.Google Scholar
Rehm, J. E. & Clemens, N. T.1999 The association of scalar dissipation rate layers and Oh zones with strain, vorticity, and 2D dilatation fields in turbulent non-premixed jets and jet flames. In Paper AIAA-99-0676, 37th Aerospace Sciences Conference, Reno, NV. American Institute of Aeronautics and Astronautics.Google Scholar
Schlatter, P., Bagheri, S. & Henningson, D. S. 2011 Self-sustained global oscillations in a jet in crossflow. Theor. Comput. Fluid Dyn. 25, 29–146.Google Scholar
Shan, J. & Dimotakis, P. 2006 Reynolds-number effects and anisotropy in transverse-jet mixing. J. Fluid Mech. 566, 47–96.Google Scholar
Shapiro, S., King, J., M’Closkey, R. T. & Karagozian, A. R. 2006 Optimization of controlled jets in crossflow. AIAA J. 44, 1292–1298.Google Scholar
Shoji, T.2017 Mixing and structural characteristics of unforced and forced jets in crossflow. PhD thesis, UCLA.Google Scholar
Sirovich, L. 1987 Turbulence and the dynamics of coherent structures. Q. Appl. Maths 45, 561–590.Google Scholar
Smith, S. H. & Mungal, M. G. 1998 Mixing, structure and scaling of the jet in crossflow. J. Fluid Mech. 357, 83–122.Google Scholar
Su, L. K. & Clemens, N. T. 2003 The structure of fine-scale scalar mixing in gas-phase planar turbulent jets. J. Fluid Mech. 488, 1–29.Google Scholar
Su, L. K. & Dahm, W. J. A. 1996 Scalar imaging velocimetry measurements of the velocity gradient tensor field in turbulent flows. II. Experimental results. Phys. Fluids 8, 1883–1906.Google Scholar
Su, L. K. & Mungal, M. G. 2004 Simultaneous measurements of scalar and velocity field evolution in turbulent crossflowing jets. J. Fluid Mech. 513, 1–45.Google Scholar
Sullivan, R., Wilde, B., Noble, D. R., Seitzman, J. M. & Lieuwen, T. C. 2014 Time-averaged characteristics of a reacting fuel jet in vitiated cross-low. Combust. Flame 161, 1792–1803.Google Scholar
Vedula, P., Yeung, P. K. & Fox, R. O. 2001 Dynamics of scalar dissipation in isotropic turbulence: a numerical and modelling study. J. Fluid Mech. 433, 29–60.Google Scholar
Vernet, R., Thomas, L. & David, L. 2009 Analysis and reconstruction of a pulsed jet in crossflow by multi-plane snapshot pod. Exp. Fluids 47, 707–720.Google Scholar
Wagner, J. A., Grib, S. W., Renfro, M. W. & Cetegen, B. M. 2015 Flowfield measurement and flame stabilization of a premixed reacting jet in vitiated crossflow. Combust. Flame 162 (10), 3711–3727.Google Scholar
Wang, G. H. & Clemens, N. T. 2004 Effects of imaging system blur on measurements of flow scalars and scalar gradients. Exp. Fluids 37, 194–205.Google Scholar
Wieneke, B. 2005 Stereo-PIV using self-calibration on particle images. Exp. Fluids 39, 267–280.Google Scholar
Yuan, L. L. & Street, R. L. 1998 Trajectory and entrainment of a round jet in crossflow. Phys. Fluids 10, 2323–2335.Google Scholar