Hostname: page-component-78c5997874-j824f Total loading time: 0 Render date: 2024-11-17T09:20:27.400Z Has data issue: false hasContentIssue false
  • English
  • Français

Influence of surface viscosity on droplets in shear flow

Published online by Cambridge University Press:  22 February 2016

J. Gounley ,
G. Boedec ,
M. Jaeger  and
M. Leonetti
J. Gounley
Affiliation:
Aix-Marseille Université, CNRS, Centrale Marseille, M2P2 UMR 7340, Marseille 13451, France
G. Boedec
Affiliation:
Aix-Marseille Université, CNRS, Centrale Marseille, IRPHE UMR 7342, Marseille 13384, France
M. Jaeger
Affiliation:
Aix-Marseille Université, CNRS, Centrale Marseille, M2P2 UMR 7340, Marseille 13451, France
M. Leonetti*
Affiliation:
Aix-Marseille Université, CNRS, Centrale Marseille, IRPHE UMR 7342, Marseille 13384, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The behaviour of a single droplet in an immiscible external fluid, submitted to shear flow is investigated using numerical simulations. The surface of the droplet is modelled by a Boussinesq–Scriven constitutive law involving the interfacial viscosities and a constant surface tension. A numerical method using Loop subdivision surfaces to represent droplet interface is introduced. This method couples boundary element method for fluid flows and finite element method to take into account the stresses due to the surface dilational and shear viscosities and surface tension. Validation of the numerical scheme with respect to previous analytic and computational work is provided, with particular attention to the viscosity contrast and the shear and dilational viscosities characterized both by a Boussinesq number 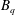$B_{q}$. Then, influence of equal surface viscosities on steady-state characteristics of a droplet in shear flow are studied, considering both small and large deformations and for a large range of bulk viscosity contrast. We find that small deformation analysis is surprisingly predictive at moderate and high surface viscosities. Equal surface viscosities decrease the Taylor deformation parameter and tank-treading angle and also strongly modify the dynamics of the droplet: when the Boussinesq number (surface viscosity) is large relative to the capillary number (surface tension), the droplet displays damped oscillations prior to steady-state tank-treading, reminiscent from the behaviour at large viscosity contrast. In the limit of infinite capillary number 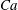$Ca$, such oscillations are permanent. The influence of surface viscosities on breakup is also investigated, and results show that the critical capillary number is increased. A diagram 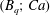$(B_{q};Ca)$ of breakup is established with the same inner and outer bulk viscosities. Additionally, the separate roles of shear and dilational surface viscosity are also elucidated, extending results from small deformation analysis. Indeed, shear (dilational) surface viscosity increases (decreases) the stability of drops to breakup under shear flow. The steady-state deformation (Taylor parameter) varies nonlinearly with each Boussinesq number or a linear combination of both Boussinesq numbers. Finally, the study shows that for certain combinations of shear and dilational viscosities, drop deformation for a given capillary number is the same as in the case of a clean surface while the inclination angle varies.

JFM classification

Biological Fluid Dynamics: Capsule/cell dynamics Complex Fluids: Complex Fluids Drops and Bubbles: Drops and Bubbles
Type
Papers
Information
Journal of Fluid Mechanics , Volume 791 , 25 March 2016 , pp. 464 - 494
Check for updates
Copyright
© 2016 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abreu, D., Levant, M., Steinberg, V. & Seifert, U. 2014 Fluid vesicles in flow. Adv. Colloid Interface Sci. 208, 129–141.Google Scholar
Acrivos, A. 1983 The breakup of small drops and bubbles in shear flows. Ann. N.Y. Acad. Sci. 404, 1–11.Google Scholar
Barthès-Biesel, D. 2009 Capsule motion in flow: deformation and membrane buckling. C. R. Phys. 10 (8), 764–774.Google Scholar
Barthès-Biesel, D. & Acrivos, A. 1973 Deformation and burst of a liquid droplet freely suspended in a linear shear field. J. Fluid Mech. 61 (01), 1–22.CrossRefGoogle Scholar
Barthès-Biesel, D. & Sgaier, H. 1985 Role of membrane viscosity in the orientation and deformation of a spherical capsule suspended in shear flow. J. Fluid Mech. 160, 119–135.CrossRefGoogle Scholar
Boedec, G., Jaeger, M. & Leonetti, M. 2012 Settling of a vesicle in the limit of quasispherical shapes. J. Fluid Mech. 690, 227–261.CrossRefGoogle Scholar
Boedec, G., Jaeger, M. & Leonetti, M. 2014 Pearling instability of a cylindrical vesicle. J. Fluid Mech. 743, 262–279.Google Scholar
Boedec, G., Leonetti, M. & Jaeger, M. 2011 3D vesicle dynamics simulations with a linearly triangulated surface. J. Comput. Phys. 230 (4), 1020–1034.Google Scholar
Boussinesq, M. 1913 Sur l’existence d’une viscosité superficielle, dans la mince couche de transition séparant un liquide d’un autre fluide contigue. Ann. Chim. Phys. 29, 349–357.Google Scholar
Chaffey, C. E. & Brenner, H. 1967 A second-order theory for shear deformation of drops. J. Colloid Interface Sci. 24, 258–269.Google Scholar
Chang, K. & Olbricht, W. 1993 Experimental studies of the deformation and breakup of a synthetic capsule in steady and unsteady simple shear flow. J. Fluid Mech. 250 (1), 609–633.CrossRefGoogle Scholar
Cirak, F., Ortiz, M. & Schroder, P. 2000 Subdivision surfaces: a new paradigm for thin-shell finite-element analysis. Intl J. Numer. Meth. Engng 47 (12), 2039–2072.3.0.CO;2-1>CrossRefGoogle Scholar
Cox, R. 1969 The deformation of a drop in a general time-dependent fluid flow. J. Fluid Mech. 37 (03), 601–623.CrossRefGoogle Scholar
Cristini, V., Guido, S., Alfani, A., Bławzdziewicz, J. & Loewenberg, M. 2003 Drop breakup and fragment size distribution in shear flow. J.  Rheol. 47 (5), 1283–1298.CrossRefGoogle Scholar
Danov, K. D. 2001 On the viscosity of dilute emulsions. J. Colloid Interface Sci. 235 (1), 144–149.Google Scholar
Dickinson, E., Murray, B. S. & Stainsby, G. 1988 Coalescence stability of emulsion-sized droplets at a planar oil–water interface and the relationship to protein film surface rheology. J. Chem. Soc. Faraday Trans. 84 (3), 871–883.Google Scholar
Erni, P. 2011 Deformation modes of complex fluid interfaces. Soft Matt. 7 (17), 7586–7600.Google Scholar
Erni, P., Fischer, P. & Windhab, E. J. 2005 Deformation of single emulsion drops covered with a viscoelastic adsorbed protein layer in simple shear flow. Appl. Phys. Lett. 87 (24), 244104.Google Scholar
Feigl, K., Megias-Alguacil, D., Fischer, P. & Windhab, E. J. 2007 Simulation and experiments of droplet deformation and orientation in simple shear flow with surfactants. Chem. Engng Sci. 62 (12), 3242–3258.Google Scholar
Fischer, P. & Erni, P. 2007 Emulsion drops in external flow fields – the role of liquid interfaces. Curr. Opin. Colloid Interface Sci. 12 (4), 196–205.Google Scholar
Flumerfelt, R. W. 1980 Effects of dynamic interfacial properties on drop deformation and orientation in shear and extensional flow fields. J.  Colloid Interface Sci. 76 (2), 330–349.Google Scholar
Georgieva, D., Schmitt, V., Leal-Calderon, F. & Langevin, D. 2009 On the possible role of surface elasticity in emulsion stability. Langmuir 25 (10), 5565–5573.Google Scholar
Guido, S., Greco, F. & Villone, M. 1999 Experimental determination of drop shape in slow steady shear flow. J. Colloid Interface Sci. 219, 298–309.Google Scholar
Guido, S. & Villone, M. 1998 Three-dimensional shape of a drop under simple shear flow. J. Rheol. 42, 395–415.Google Scholar
Harvey, P., Nguyen, A., Jameson, G. & Evans, G. 2005 Influence of sodium dodecyl sulphate and dowfroth frothers on froth stability. Miner. Engng 18 (3), 311–315.Google Scholar
Huang, W.-X., Chang, C. B. & Sung, H. J. 2012 Three-dimensional simulation of elastic capsules in shear flow by the penalty immersed boundary method. J. Comput. Phys. 231, 3340–3364.Google Scholar
Kennedy, M., Pozrikidis, C. & Skalak, R. 1994 Motion and deformation of liquid drops, and the rheology of dilute emulsions in simple shear flow. Comput. Fluids 23 (2), 251–278.Google Scholar
Kwak, S. & Pozrikidis, C. 1998 Adaptive triangulation of evolving, closed, or open surfaces by the advancing-front method. J. Comput. Phys. 145 (1), 61–88.Google Scholar
Langevin, D. 2000 Influence of interfacial rheology on foam and emulsion properties. Adv. Colloid Interface Sci. 88 (1), 209–222.Google Scholar
Le, D. V. 2010 Effect of bending stiffness on the deformation of liquid capsules enclosed by thin shells in shear flow. Phys. Rev. E 82, 016318.Google ScholarPubMed
LeVan, M. D. 1981 Motion of a droplet with a Newtonian interface. J. Colloid Interface Sci. 83 (1), 11–17.Google Scholar
Li, J., Renardy, Y. Y. & Renardy, M. 2000 Numerical simulation of breakup of a viscous drop in simple shear flow through a volume-of-fluid method. Phys. Fluids 12 (2), 269–282.Google Scholar
Li, X. & Pozrikidis, C. 1997 The effect of surfactants on drop deformation and on the rheology of dilute emulsions in Stokes flow. J. Fluid Mech. 341, 165–194.CrossRefGoogle Scholar
Loop, C. T.1987 Smooth subdivision surfaces based on triangles. Master’s thesis, University of Utah.Google Scholar
de Loubens, C., Deschamps, J., Boedec, G. & Leonetti, M. 2015a Stretching of capsules in an elongation flow, a route to constitutive law. J. Fluid Mech. 767, R3.Google Scholar
de Loubens, C., Deschamps, J., Edwards-Levy, F. & Leonetti, M. 2015b Tank-treading of microcapsules in shear flow. J. Fluid Mech., doi:10.1017/jfm.2015.758.Google Scholar
Manor, O., Lavrenteva, O. & Nir, A. 2008 Effect of non-homogeneous surface viscosity on the marangoni migration of a droplet in viscous fluid. J. Colloid Interface Sci. 321 (1), 142–153.Google Scholar
Miller, R., Ferri, J. K., Javadi, A., Kragel, J., Mucic, N. & Wustneck, R. 2010 Rheology of interfacial layers. Colloid Polym. Sci. 288, 937–950.Google Scholar
Mun, S. & McClements, D. J. 2006 Influence of interfacial characteristics on Ostwald ripening in hydrocarbon oil-in-water emulsions. Langmuir 22 (4), 1551–1554.CrossRefGoogle ScholarPubMed
Narsimhan, V., Spann, A. P. & Shaqfeh, E. S. G. 2015 Pearling, wrinkling buckling of vesicles in elongation flows. J. Fluid Mech. 777, 1–26.Google Scholar
Oldroyd, J. 1955 The effect of interfacial stabilizing films on the elastic and viscous properties of emulsions. Proc. R. Soc. Lond. A 232 (1191), 567–577.Google Scholar
Pawar, Y. & Stebe, K. J. 1996 Marangoni effects on drop deformation in an extensional flow: the role of surfactnt physical chemistry. i. Insoluble surfactants. Phys. Fluids 8 (7), 1738–1751.Google Scholar
Phillips, W. J., Graves, R. W. & Flumerfelt, R. W. 1980 Experimental studies of drop dynamics in shear fields: role of dynamic interfacial effects. J. Colloid Interface Sci. 76 (2), 350–370.Google Scholar
Pozrikidis, C. 1992 Boundary Integral and Singularity Methods for Linearized Viscous Flow. Cambridge University Press.Google Scholar
Pozrikidis, C. 1994 Effects of surface viscosity on the finite deformation of a liquid drop and the rheology of dilute emulsions in simple shearing flow. J. Non-Newtonian Fluid Mech. 51 (2), 161–178.Google Scholar
Rallison, J. 1980 Note on the time-dependent deformation of a viscous drop which is almost spherical. J. Fluid Mech. 98 (03), 625–633.Google Scholar
Rallison, J. 1981 A numerical study of the deformation and burst of a viscous drop in general shear flows. J. Fluid Mech. 109, 465–482.Google Scholar
Rallison, J. & Acrivos, A. 1978 A numerical study of the deformation and burst of a viscous drop in an extensional flow. J. Fluid Mech. 89 (01), 191–200.Google Scholar
Rallison, J. M. 1984 The deformation of small viscous drops and bubbles in shear flows. Annu. Rev. Fluid Mech. 16, 45–66.Google Scholar
Reusken, A. & Zhang, Y. 2013 Numerical simulation of incompressible two-phase flows with a Boussinesq–Scriven interface stress tensor. Intl J. Numer. Meth. Fluids 73 (12), 1042–1058.Google Scholar
Rodrigues, D. S., Ausas, R. F., Mut, F. & Buscaglia, G. C. 2015 A semi-implicit finite element method for viscous lipid membranes. J. Comput. Phys. 298, 565–584.Google Scholar
Rumscheidt, F.-D. & Mason, S. 1961 Particle motions in sheared suspensions XII. Deformation and burst of fluid drops in shear and hyperbolic flow. J. Colloid Sci. 16 (3), 238–261.Google Scholar
Schwalbe, J. T., Phelan, F. R. Jr, Vlahovska, P. M. & Hudson, S. D. 2011 Interfacial effects on droplet dynamics in Poiseuille flow. Soft Matt. 7 (17), 7797–7804.Google Scholar
Scriven, L. 1960 Dynamics of a fluid interface equation of motion for Newtonian surface fluids. Chem. Engng Sci. 12 (2), 98–108.Google Scholar
Secomb, T. & Skalak, R. 1982 Surface flow of viscoelastic membranes in viscous fluids. Q. J. Mech. Appl. Maths 35 (2), 233–247.Google Scholar
Spann, A. P., Zhao, H. & Shaqfeh, E. S. G. 2014 Loop subdivision surface boundary integral method simulations of vesicles at low reduced volume ratio in shear and extensional flow. Phys. Fluids 26, 031902.CrossRefGoogle Scholar
Stone, H. & Leal, L. 1990 The effects of surfactants on drop deformation and breakup. J. Fluid Mech. 220, 161–186.Google Scholar
Stone, H. A. 1994 Dynamics of drop deformation and breakup in viscous fluids. Annu. Rev. Fluid Mech. 26 (1), 65–102.CrossRefGoogle Scholar
Taylor, G. 1934 The formation of emulsions in definable fields of flow. Proc. R. Soc. Lond. A 146 (858), 501–523.Google Scholar
Valkovska, D., Danov, K. & Ivanov, I. 1999 Surfactants role on the deformation of colliding small bubbles. Colloids Surf. A 156 (1), 547–566.CrossRefGoogle Scholar
Vlahovska, P. M., Blawzdziewicz, J. & Loewenberg, M. 2005 Deformation of a surfactant-covered drop in a linear flow. Phys. Fluids 17, 103103.Google Scholar
Vlahovska, P. M., Blawzdziewicz, J. & Loewenberg, M. 2009a Small-deformation theory for a surfactant drop in linear flows. J. Fluid Mech. 624, 293–337.Google Scholar
Vlahovska, P. M., Podgorski, T. & Misbah, C. 2009b Vesicles and red blood cells in flow: from individual dynamics to rheology. C. R. Phys. 10 (8), 775–789.Google Scholar
Yazdani, A. & Bagchi, P. 2013 Influence of membrane viscosity on capsule dynamics in shear flow. J. Fluid Mech. 718, 569–595.Google Scholar
Zell, Z. A., Nowbahar, A., Mansard, V., Leal, L. G., Deshmukh, S. S., Mecca, J. M., Tucker, C. J. & Squires, T. M. 2014 Surface shear inviscidity of soluble surfactants. Proc. Natl Acad. Sci. USA 111 (10), 3677–3682.Google Scholar