Hostname: page-component-745bb68f8f-b95js Total loading time: 0 Render date: 2025-01-25T21:25:56.325Z Has data issue: false hasContentIssue false
  • English
  • Français

Inertial migration of a neutrally buoyant spheroid in plane Poiseuille flow

Published online by Cambridge University Press:  03 November 2023

Prateek Anand Open the ORCID record for Prateek Anand [Opens in a new window]  and
Ganesh Subramanian Open the ORCID record for Ganesh Subramanian [Opens in a new window]
Prateek Anand
Affiliation:
International Centre for Theoretical Sciences, Bengaluru 560089, India
Ganesh Subramanian*
Affiliation:
Engineering Mechanics Unit, Jawaharlal Nehru Centre for Advanced Scientific Research, Bengaluru 560064, India
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

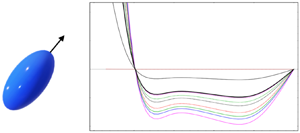
We study the cross-stream inertial migration of a torque-free neutrally buoyant spheroid, of an arbitrary aspect ratio $\kappa$, in wall-bounded plane Poiseuille flow for small particle Reynolds numbers ($Re_p\ll 1$) and confinement ratios ($\lambda \ll 1$), with the channel Reynolds number, $Re_c = Re_p/\lambda ^2$, assumed to be arbitrary; here $\lambda =L/H$, where $L$ is the semi-major axis of the spheroid and $H$ denotes the separation between the channel walls. In the Stokes limit ($Re_p =0)$, and for $\lambda \ll 1$, a spheroid rotates along any of an infinite number of Jeffery orbits parameterized by an orbit constant $C$, while translating with a time-dependent speed along a given ambient streamline. Weak inertial effects stabilize either the spinning ($C=0$) or tumbling orbit ($C=\infty$), or both, depending on $\kappa$. The asymptotic separation of the Jeffery rotation and orbital drift time scales, from that associated with cross-stream migration, implies that migration occurs due to a Jeffery-averaged lift velocity. Although the magnitude of this averaged lift velocity depends on $\kappa$ and $C$, the shape of the lift profiles are identical to those for a sphere, regardless of $Re_c$. In particular, the equilibrium positions for a spheroid remain identical to the classical Segre–Silberberg ones for a sphere, starting off at a distance of about $0.6(H/2)$ from the channel centreline for small $Re_c$, and migrating wallward with increasing $Re_c$. For spheroids with $\kappa \sim O(1)$, the Jeffery-averaged analysis is valid for $Re_p\ll 1$; for extreme aspect ratio spheroids, the regime of validity becomes more restrictive being given by $Re_p \kappa /\ln \kappa \ll 1$ and $Re_p/\kappa \ll 1$ for $\kappa \rightarrow \infty$ (slender fibres) and $\kappa \rightarrow 0$ (flat disks), respectively.

JFM classification

Complex Fluids: Suspensions Low-Reynolds-number flows: Stokesian dynamics Micro-/Nano-fluid dynamics: Microfluidics
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 974 , 10 November 2023 , A39
Check for updates
Copyright
© The Author(s), 2023. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Anand, P. & Subramanian, G. 2023 Inertial migration in pressure-driven channel flow: beyond the Segre–Silberberg pinch. Physical Review Letters (submitted) arXiv:2301.00789.Google Scholar
Aoki, H., Kurosak, Y. & Anzai, H. 1979 Study on the tubular pinch effect in a pipe flow: I. Lateral migration of a single particle in laminar Poiseuille flow. Bull. JSME 22 (164), 206–212.Google Scholar
Arfken, G.B., Weber, H.J. & Harris, F.E. 2011 Mahematical Methods for Physicists: a Comprehensive Guide. Academic.Google Scholar
Asmolov, E.S. 1999 The inertial lift on a spherical particle in a plane Poiseuille flow at large channel Reynolds number. J. Fluid Mech. 381, 63–87.CrossRefGoogle Scholar
Bagge, J., Rosén, T., Lundell, F. & Tornberg, A.-K. 2021 Parabolic velocity profile causes shape-selective drift of inertial ellipsoids. J. Fluid Mech. 926, A24.CrossRefGoogle Scholar
Blake, J.R. 1971 A note on the image system for a Stokeslet in a no-slip boundary. In Mathematical Proceedings of the Cambridge Philosophical Society, vol. 70, pp. 303–310. Cambridge University Press.CrossRefGoogle Scholar
Chen, S.-D., Pan, T.-W. & Chang, C.-C. 2012 The motion of a single and multiple neutrally buoyant elliptical cylinders in plane Poiseuille flow. Phys. Fluids 24 (10), 103302.CrossRefGoogle Scholar
Cherukat, P. & Mclaughlin, J.B. 1994 The inertial lift on a rigid sphere in a linear shear flow field near a flat wall. J. Fluid Mech. 263, 1–18.CrossRefGoogle Scholar
Chun, B. & Ladd, A.J.C. 2006 Inertial migration of neutrally buoyant particles in a square duct: an investigation of multiple equilibrium positions. Phys. Fluids 18 (3), 031704.CrossRefGoogle Scholar
Chung, S.E., Park, W., Shin, S., Lee, S.A. & Kwon, S. 2008 Guided and fluidic self-assembly of microstructures using railed microfluidic channels. Nat. Mater. 7 (7), 581–587.CrossRefGoogle ScholarPubMed
Chwang, A.T. 1975 Hydromechanics of low-Reynolds-number flow. Part 3. Motion of a spheroidal particle in quadratic flows. J. Fluid Mech. 72 (1), 17–34.CrossRefGoogle Scholar
Cox, R.G. & Brenner, H. 1967 Effect of finite boundaries on the Stokes resistance of an arbitrary particle. Part 3. Translation and rotation. J. Fluid Mech. 28 (2), 391–411.CrossRefGoogle Scholar
Cox, R.G. & Brenner, H. 1968 The lateral migration of solid particles in Poiseuille flow—I theory. Chem. Engng Sci. 23 (2), 147–173.CrossRefGoogle Scholar
Cox, R.G. & Hsu, S.K. 1977 The lateral migration of solid particles in a laminar flow near a plane. Intl J. Multiphase Flow 3 (3), 201–222.CrossRefGoogle Scholar
Dabade, V., Marath, N.K. & Subramanian, G. 2015 Effects of inertia and viscoelasticity on sedimenting anisotropic particles. J. Fluid Mech. 778, 133–188.CrossRefGoogle Scholar
Dabade, V., Marath, N.K. & Subramanian, G. 2016 The effect of inertia on the orientation dynamics of anisotropic particles in simple shear flow. J. Fluid Mech. 791, 631–703.CrossRefGoogle Scholar
Di Carlo, D. 2009 Inertial microfluidics. Lab on a Chip 9, 3038–3046.CrossRefGoogle ScholarPubMed
Di Carlo, D., Irimia, D., Tompkins, R.G. & Toner, M. 2007 Continuous inertial focusing, ordering, and separation of particles in microchannels. Proc. Natl Acad. Sci. 104 (48), 18892–18897.CrossRefGoogle ScholarPubMed
Einarsson, J., Candelier, F., Lundell, F., Angilella, J.R. & Mehlig, B. 2015 Rotation of a spheroid in a simple shear at small Reynolds number. Phys. Fluids 27 (6), 063301.CrossRefGoogle Scholar
Goldman, A.J., Cox, R.G. & Brenner, H. 1967 Slow viscous motion of a sphere parallel to a plane wall—II Couette flow. Chem. Engng Sci. 22 (4), 653–660.CrossRefGoogle Scholar
Happel, J. & Brenner, H. 2012 Low Reynolds Number Hydrodynamics: With Special Applications to Particulate Media, vol. 1. Springer.Google Scholar
Ho, B.P. & Leal, L.G. 1974 Inertial migration of rigid spheres in two-dimensional unidirectional flows. J. Fluid Mech. 65 (2), 365–400.CrossRefGoogle Scholar
Hogg, A.J. 1994 The inertial migration of non-neutrally buoyant spherical particles in two-dimensional shear flows. J. Fluid Mech. 272, 285–318.CrossRefGoogle Scholar
Hood, K., Lee, S. & Roper, M. 2015 Inertial migration of a rigid sphere in three-dimensional Poiseuille flow. J. Fluid Mech. 765, 452–479.CrossRefGoogle Scholar
Huang, Y., Marson, R.L. & Larson, R.G. 2019 Inertial migration of neutrally buoyant prolate and oblate spheroids in plane Poiseuille flow using dissipative particle dynamics simulations. Comput. Mater. Sci. 162, 178–185.CrossRefGoogle Scholar
Hur, S.C., Choi, S.-E., Kwon, S. & Carlo, D.D. 2011 Inertial focusing of non-spherical microparticles. Appl. Phys. Lett. 99 (4), 044101.CrossRefGoogle Scholar
Jeffery, G.B. 1922 The motion of ellipsoidal particles immersed in a viscous fluid. In Proceedings of the Royal Society of London. Series A, Containing Papers of a Mathematical and Physical Character. 102 (715), 161–179.Google Scholar
Jeffrey, R.C. & Pearson, J.R.A. 1965 Particle motion in laminar vertical tube flow. J. Fluid Mech. 22 (4), 721–735.CrossRefGoogle Scholar
Karnis, A., Goldsmith, H.L. & Mason, S.G. 1966 The kinetics of flowing dispersions: I. Concentrated suspensions of rigid particles. J. Colloid Interface Sci. 22 (6), 531–553.CrossRefGoogle Scholar
Kim, S. & Karrila, S.J. 1991 Chapter 3 – The Disturbance Field of a Single Particle in a Steady Flow. Butterworth-Heinemann.Google Scholar
Kushch, V.I. & Sangani, A.S. 2003 The complete solutions for Stokes interactions of spheroidal particles by the mutipole expansion method. Intl J. Mutiphase Flow 34, 1353–1366.Google Scholar
Leal, L.G. & Hinch, E.J. 1971 The effect of weak Brownian rotations on particles in shear flow. J. Fluid Mech. 46 (4), 685–703.CrossRefGoogle Scholar
Leighton, D. & Acrivos, A. 1985 The lift on a small sphere touching a plane in the presence of a simple shear flow. Z. Angew. Math. Phys. 36 (1), 174–178.CrossRefGoogle Scholar
Liron, N. & Mochon, S. 1976 Stokes flow for a Stokeslet between two parallel flat plates. J. Engng Maths 10 (4), 287–303.CrossRefGoogle Scholar
Liron, N. & Shahar, R. 1978 Stokes flow due to a Stokeslet in a pipe. J. Fluid Mech. 86 (4), 727–744.CrossRefGoogle Scholar
Marath, N.K., Dwivedi, R. & Subramanian, G. 2017 An orientational order transition in a sheared suspension of anisotropic particles. J. Fluid Mech. 811, R3.CrossRefGoogle Scholar
Marath, N.K. & Subramanian, G. 2017 The effect of inertia on the time period of rotation of an anisotropic particle in simple shear flow. J. Fluid Mech. 830, 165–210.CrossRefGoogle Scholar
Marath, N.K. & Subramanian, G. 2018 The inertial orientation dynamics of anisotropic particles in planar linear flows. J. Fluid Mech. 844, 357–402.CrossRefGoogle Scholar
Masaeli, M., Sollier, E., Amini, H., Mao, W., Camacho, K., Doshi, N., Mitragotri, S., Alexeev, A. & Di Carlo, D. 2012 Continuous inertial focusing and separation of particles by shape. Phys. Rev. X 2 (3), 031017.Google Scholar
Matas, J.-P., Morris, J.F. & Guazzelli, É. 2004 Inertial migration of rigid spherical particles in Poiseuille flow. J. Fluid Mech. 515, 171–195.CrossRefGoogle Scholar
Matas, J.-P., Morris, J.F. & Guazzelli, E. 2009 Lateral force on a rigid sphere in large-inertia laminar pipe flow. J. Fluid Mech. 621, 59–67.CrossRefGoogle Scholar
Mody, N.A. & King, M.R. 2005 Three-dimensional simulations of a platelet-shaped spheroid near a wall in shear flow. Phys. Fluids 17 (11), 113302.CrossRefGoogle Scholar
Morita, Y., Itano, T. & Sugihara-Seki, M. 2017 Equilibrium radial positions of neutrally buoyant spherical particles over the circular cross-section in Poiseuille flow. J. Fluid Mech. 813, 750–767.CrossRefGoogle Scholar
Nakayama, S., Yamashita, H., Yabu, T., Itano, T. & Sugihara-Seki, M. 2019 Three regimes of inertial focusing for spherical particles suspended in circular tube flows. J. Fluid Mech. 871, 952–969.CrossRefGoogle Scholar
Nizkaya, T.V., Gekova, A.S., Harting, J., Asmolov, E.S. & Vinogradova, O.I. 2020 Inertial migration of oblate spheroids in a plane channel. Phys. Fluids 32 (11), 112017.CrossRefGoogle Scholar
Pan, T.-W., Li, A. & Glowinski, R. 2021 Numerical study of equilibrium radial positions of neutrally buoyant balls in circular Poiseuille flows. Phys. Fluids 33 (3), 033301.CrossRefGoogle Scholar
Pozrikidis, C. 2005 Orbiting motion of a freely suspended spheroid near a plane wall. J. Fluid Mech. 541, 105–114.CrossRefGoogle Scholar
Proudman, I. & Pearson, J.R.A. 1957 Expansions at small Reynolds numbers for the flow past a sphere and a circular cylinder. J. Fluid Mech. 2 (3), 237–262.CrossRefGoogle Scholar
Repetti, R.V. & Leonard, E.F. 1964 Segré–Silberberg annulus formation: a possible explanation. Nature 203 (4952), 1346–1348.CrossRefGoogle Scholar
Saffman, P.G. 1965 The lift on a small sphere in a slow shear flow. J. Fluid Mech. 22 (2), 385–400.CrossRefGoogle Scholar
Schmid, P.J., Henningson, D.S. & Jankowski, D.F. 2002 Stability and transition in shear flows applied mathematical sciences, vol. 142. Appl. Mech. Rev. 55 (3), B57–B59.CrossRefGoogle Scholar
Schonberg, J.A. & Hinch, E.J. 1989 Inertial migration of a sphere in Poiseuille flow. J. Fluid Mech. 203, 517–524.CrossRefGoogle Scholar
Segre, G. & Silberberg, A. 1962 a Behaviour of macroscopic rigid spheres in Poiseuille flow. Part 1. Determination of local concentration by statistical analysis of particle passages through crossed light beams. J. Fluid Mech. 14 (1), 115–135.CrossRefGoogle Scholar
Segre, G. & Silberberg, A. 1962 b Behaviour of macroscopic rigid spheres in Poiseuille flow. Part 2. Experimental results and interpretation. J. Fluid Mech. 14 (1), 136–157.CrossRefGoogle Scholar
Segre, G. & Silberberg, A. 1963 Non-Newtonian behavior of dilute suspensions of macroscopic spheres in a capillary viscometer. J. Colloid Sci. 18 (4), 312–317.CrossRefGoogle Scholar
Shao, X., Yu, Z. & Sun, B. 2008 Inertial migration of spherical particles in circular Poiseuille flow at moderately high Reynolds numbers. Phys. Fluids 20 (10), 103307.CrossRefGoogle Scholar
Staben, M.E., Zinchenko, A.Z. & Davis, R.H. 2003 Motion of a particle between two parallel plane walls in low-Reynolds-number Poiseuille flow. Phys. Fluids 15 (6), 1711–1733.CrossRefGoogle Scholar
Staben, M.E., Zinchenko, A.Z. & Davis, R.H. 2006 Dynamic simulation of spheroid motion between two parallel plane walls in low-Reynolds-number Poiseuille flow. J. Fluid Mech. 553, 187–226.CrossRefGoogle Scholar
Stover, C.A. & Cohen, C. 1990 The motion of rodlike particles in the pressure-driven flow between two flat plates. Rheol Acta 29, 192–203.CrossRefGoogle Scholar
Subramanian, G. & Koch, D.L. 2006 Inertial effects on the orientation of nearly spherical particles in simple shear flow. J. Fluid Mech. 557, 257–296.CrossRefGoogle Scholar
Subramanian, G. & Koch, D.L. 2005 Inertial effects on fibre motion in simple shear flow. J. Fluid Mech. 535, 383.CrossRefGoogle Scholar
Subramanian, G. & Marath, N.K. 2022 Rheology of dilute inertial suspensions. In Recent Advances in Rheology: Theory, Biorheology, Suspension and Interfacial Rheology (ed. D. De Kee & A. Ramachandran), pp. 9–1. AIP Publishing LLC.CrossRefGoogle Scholar
Sugihara-Seki, M. 1993 The motion of an elliptical cylinder in channel flow at low Reynolds numbers. J. Fluid Mech. 257, 575–596.CrossRefGoogle Scholar
Sugihara-Seki, M. 1996 The motion of an ellipsoid in tube flow at low Reynolds numbers. J. Fluid Mech. 324, 287–308.CrossRefGoogle Scholar
Suresh, S. 2007 Biomechanics and biophysics of cancer cells. Acta Biomater. 3 (4), 413–438.CrossRefGoogle ScholarPubMed
Swan, J.W. & Brady, J.F. 2010 Particle motion between parallel walls: hydrodynamics and simulation. Phys. Fluids 22 (10), 103301.CrossRefGoogle Scholar
Tachibana, M. 1973 On the behaviour of a sphere in the laminar tube flows. Rheol Acta 12 (1), 58–69.CrossRefGoogle Scholar
Toner, M. & Irimia, D. 2005 Blood-on-a-chip. Annu. Rev. Biomed. Engng 7, 77–103.CrossRefGoogle ScholarPubMed
Vasseur, P. & Cox, R.G. 1976 The lateral migration of a spherical particle in two-dimensional shear flows. J. Fluid Mech. 78 (2), 385–413.CrossRefGoogle Scholar