Published online by Cambridge University Press: 24 August 2017
Dynamics and mixing of a variable-density turbulent flow subject to an externally imposed acceleration field in the zero-Mach-number limit are studied in a series of direct numerical simulations. The flow configuration studied consists of alternating slabs of high- and low-density fluid in a triply periodic domain. Density ratios in the range of 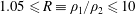 $1.05\leqslant R\equiv \unicode[STIX]{x1D70C}_{1}/\unicode[STIX]{x1D70C}_{2}\leqslant 10$ are investigated. The flow produces temporally evolving shear layers. A perpendicular density–pressure gradient is maintained in the mean as the flow evolves, with multi-scale baroclinic torques generated in the turbulent flow that ensues. For all density ratios studied, the simulations attain Reynolds numbers at the beginning of the fully developed turbulence regime. An empirical relation for the convection velocity predicts the observed entrainment-ratio and dominant mixed-fluid composition statistics. Two mixing-layer temporal evolution regimes are identified: an initial diffusion-dominated regime with a growth rate
$1.05\leqslant R\equiv \unicode[STIX]{x1D70C}_{1}/\unicode[STIX]{x1D70C}_{2}\leqslant 10$ are investigated. The flow produces temporally evolving shear layers. A perpendicular density–pressure gradient is maintained in the mean as the flow evolves, with multi-scale baroclinic torques generated in the turbulent flow that ensues. For all density ratios studied, the simulations attain Reynolds numbers at the beginning of the fully developed turbulence regime. An empirical relation for the convection velocity predicts the observed entrainment-ratio and dominant mixed-fluid composition statistics. Two mixing-layer temporal evolution regimes are identified: an initial diffusion-dominated regime with a growth rate 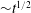 ${\sim}t^{1/2}$ followed by a turbulence-dominated regime with a growth rate
${\sim}t^{1/2}$ followed by a turbulence-dominated regime with a growth rate 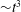 ${\sim}t^{3}$. In the turbulent regime, composition probability density functions within the shear layers exhibit a slightly tilted (‘non-marching’) hump, corresponding to the most probable mole fraction. The shear layers preferentially entrain low-density fluid by volume at all density ratios, which is reflected in the mixed-fluid composition.
${\sim}t^{3}$. In the turbulent regime, composition probability density functions within the shear layers exhibit a slightly tilted (‘non-marching’) hump, corresponding to the most probable mole fraction. The shear layers preferentially entrain low-density fluid by volume at all density ratios, which is reflected in the mixed-fluid composition.