Article contents
Group resonant interactions between surface and internal gravity waves in a two-layer system
Published online by Cambridge University Press: 02 April 2020
Abstract
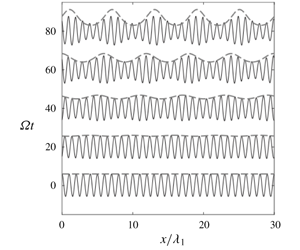
Nonlinear interactions between surface and internal gravity waves in a two-layer system are studied using explicit second-order nonlinear evolution equations in Hamiltonian form. Motivated by the detailed experiment of Lewis, Lake & Ko (J. Fluid Mech., vol. 63, 1974, pp. 773–800), our focus is on surface wave modulation by the group resonance mechanism that corresponds to near-resonant triad interactions between a long internal wave and short surface waves. Our numerical solutions show good agreement with laboratory measurements of the local wave amplitude and slope, and confirm that the surface modulation becomes significant when the group velocity of the surface waves matches the phase speed of the internal wave, as the linear modulation theory predicts. It is shown, however, that, after the envelope amplitude is increased sufficiently, the surface and internal waves start to exchange energy through near-resonant triad interactions, which is found to be crucial to accurately describe the long-term surface wave modulation by an internal wave. The reduced amplitude equations are also adopted to validate this observation. For oceanic applications, numerical solutions are presented for a density ratio close to one and it is found that significant energy exchanges occur through primary and successive resonant triad interactions.
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2020. Published by Cambridge University Press
References
- 11
- Cited by


