Hostname: page-component-586b7cd67f-2brh9 Total loading time: 0 Render date: 2024-11-27T23:39:49.896Z Has data issue: false hasContentIssue false
  • English
  • Français

Granular particle-shape heterogeneous mixtures discharging through a silo

Published online by Cambridge University Press:  11 February 2021

A. Vamsi Krishna Reddy Open the ORCID record for A. Vamsi Krishna Reddy [Opens in a new window] ,
Sonu Kumar Open the ORCID record for Sonu Kumar [Opens in a new window]  and
K. Anki Reddy Open the ORCID record for K. Anki Reddy [Opens in a new window]
A. Vamsi Krishna Reddy
Affiliation:
Department of Chemical Engineering, Indian Institute of Technology Guwahati, Guwahati781039, India
Sonu Kumar
Affiliation:
Department of Chemical Engineering, Indian Institute of Technology Guwahati, Guwahati781039, India
K. Anki Reddy*
Affiliation:
Department of Chemical Engineering, Indian Institute of Technology Guwahati, Guwahati781039, India
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

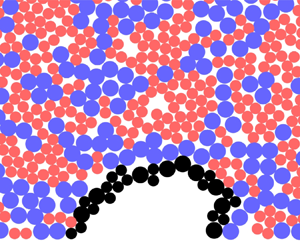
Process industries often involve handling non-cohesive solid materials which vary in size and shape. A comprehensive understanding of such systems helps in effective handling of industrial operations. Here, we studied heterogeneous mixtures of dumbbells and discs flowing out of a two-dimensional silo using discrete element method simulations. We analysed discharge dynamics of the mixtures in two regimes, namely the free-flow regime ($W/d>=15$) and the interrupted flow regime ($W/d<=10$), where $W$ and $d$ are the orifice width and diameter of each of the circles of a dumbbell. One of the intriguing results is a decrease in the flow rate $Q$ of mixtures with an increase in the fraction of dumbbells $X_{db}$ in both of the regimes analysed. This can be attributed to the geometrical interlocking among the particles and a hindrance to the rotation of dumbbells. The time-averaged (coarse-grained) flow fields reveal an increase in the size of the stagnant zone beside the orifice with an increase in $X_{db}$. The stagnant zone hinders the particles flowing next to it, which is another reason for a decrease in $Q$ with an increase in $X_{db}$. In the interrupted flow regime, we investigated clogged states of the mixtures using arch morphology, the fraction of dumbbells and number of particles in an arch, and avalanche sizes.

JFM classification

Granular media: Granular media
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 912 , 10 April 2021 , A22
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

‡

Present address: University of Notre Dame, Notre Dame, IN 46556, USA.

References

REFERENCES

Arévalo, R., Maza, D. & Pugnaloni, L.A. 2006 Identification of arches in two-dimensional granular packings. Phys. Rev. E 74, 021303.CrossRefGoogle ScholarPubMed
Artega, P. & Tüzün, U. 1990 Flow of binary mixtures of equal-density granules in hoppers—size segregation, flowing density and discharge rates. Chem. Engng Sci. 45 (1), 205–223.CrossRefGoogle Scholar
Ashour, A., Wegner, S., Trittel, T., Börzsönyi, T. & Stannarius, R. 2017 Outflow and clogging of shape-anisotropic grains in hoppers with small apertures. Soft Matt. 13, 402–414.CrossRefGoogle ScholarPubMed
Benyamine, M., Djermane, M., Dalloz-Dubrujeaud, B. & Aussillous, P. 2014 Discharge flow of a bidisperse granular media from a silo. Phys. Rev. E 90, 032201.CrossRefGoogle ScholarPubMed
Beverloo, W.A., Leniger, H.A. & van de Velde, J. 1961 The flow of granular solids through orifices. Chem. Engng Sci. 15 (3–4), 260–269.CrossRefGoogle Scholar
Börzsönyi, T., Somfai, E., Szabó, B., Wegner, S., Ashour, A. & Stannarius, R. 2017 Elongated grains in a hopper. EPJ Web Conf. 140, 06017.CrossRefGoogle Scholar
Börzsönyi, T., Somfai, E., Szabó, B., Wegner, S., Mier, P., Rose, G. & Stannarius, R. 2016 Packing, alignment and flow of shape-anisotropic grains in a 3D silo experiment. New J. Phys. 18 (9), 093017.CrossRefGoogle Scholar
Brilliantov, N.V., Spahn, F., Hertzsch, J.-M. & Pöschel, T. 1996 Model for collisions in granular gases. Phys. Rev. E 53, 5382–5392.CrossRefGoogle ScholarPubMed
Cao, Y.X., Chakrabortty, B., Barker, G.C., Mehta, A. & Wang, Y.J. 2013 Bridges in three-dimensional granular packings: experiments and simulations. Europhys. Lett. 102 (2), 24004.CrossRefGoogle Scholar
Chehata, D., Zenit, R. & Wassgren, C.R. 2003 Dense granular flow around an immersed cylinder. Phys. Fluids 15 (6), 1622–1631.CrossRefGoogle Scholar
Chevoir, F., Gaulard, F. & Roussel, N. 2007 Flow and jamming of granular mixtures through obstacles. Europhys. Lett. 79 (1), 14001.CrossRefGoogle Scholar
Cundall, P.A. & Strack, O.D.L. 1979 A discrete numerical model for granular assemblies. Géotechnique 29 (1), 47–65.CrossRefGoogle Scholar
Endo, K., Reddy, K.A. & Katsuragi, H. 2017 Obstacle-shape effect in a two-dimensional granular silo flow field. Phys. Rev. Fluids 2, 094302.CrossRefGoogle Scholar
Ertaş, D., Halsey, T.C., Levine, A.J. & Mason, T.G. 2002 Stability of monomer-dimer piles. Phys. Rev. E 66, 051307.CrossRefGoogle ScholarPubMed
Garcimartín, A., Zuriguel, I., Pugnaloni, L.A. & Janda, A. 2010 Shape of jamming arches in two-dimensional deposits of granular materials. Phys. Rev. E 82, 031306.CrossRefGoogle ScholarPubMed
Glasser, B.J. & Goldhirsch, I. 2001 Scale dependence, correlations, and fluctuations of stresses in rapid granular flows. Phys. Fluids 13 (2), 407–420.CrossRefGoogle Scholar
Guo, Z., Chen, X., Xu, Y. & Liu, H. 2015 Enhancing the linear flow of fine granules through the addition of elongated particles. Sci. Rep. 5, 16071.CrossRefGoogle ScholarPubMed
Harada, S., Mitsui, T. & Sato, K. 2012 Particle-like and fluid-like settling of a stratified suspension. Eur. Phys. J. E 35 (1), 1.CrossRefGoogle ScholarPubMed
Hidalgo, R.C., Lozano, C., Zuriguel, I. & Garcimartín, A. 2013 Force analysis of clogging arches in a silo. Granul. Matt. 15 (6), 841–848.CrossRefGoogle Scholar
Kozicki, J. & Tejchman, J. 2005 Application of a cellular automaton to simulations of granular flow in silos. Granul. Matt. 7 (1), 45–54.CrossRefGoogle Scholar
Kumar, S., Dhiman, M. & Reddy, K.A. 2019 Magnus effect in granular media. Phys. Rev. E 99, 012902.CrossRefGoogle ScholarPubMed
Liu, S.D., Zhou, Z.Y., Zou, R.P., Pinson, D. & Yu, A.B. 2014 Flow characteristics and discharge rate of ellipsoidal particles in a flat bottom hopper. Powder Technol. 253, 70–79.CrossRefGoogle Scholar
López-Rodríguez, D., Gella, D., Kiwing, T., Maza, D., Garcimartín, A. & Zuriguel, I. 2019 Effect of hopper angle on granular clogging. Phys. Rev. E 99, 032901.CrossRefGoogle ScholarPubMed
Lozano, C., Janda, A., Garcimartín, A., Maza, D. & Zuriguel, I. 2012 a Flow and clogging in a silo with an obstacle above the orifice. Phys. Rev. E 86, 031306.CrossRefGoogle Scholar
Lozano, C., Lumay, G., Zuriguel, I., Hidalgo, R.C. & Garcimartín, A. 2012 b Breaking arches with vibrations: the role of defects. Phys. Rev. Lett. 109, 068001.CrossRefGoogle ScholarPubMed
Lozano, C., Zuriguel, I. & Garcimartín, A. 2015 Stability of clogging arches in a silo submitted to vertical vibrations. Phys. Rev. E 91, 062203.CrossRefGoogle Scholar
Mankoc, C., Janda, A., Arévalo, R., Pastor, J.M., Zuriguel, I., Garcimartín, A. & Maza, D. 2007 The flow rate of granular materials through an orifice. Granul. Matt. 9 (6), 407–414.CrossRefGoogle Scholar
Medina, A., Cabrera, D., López-Villa, A. & Pliego, M. 2014 Discharge rates of dry granular material from bins with lateral exit holes. Powder Technol. 253, 270–275.CrossRefGoogle Scholar
Mehta, A. 2010 Spatial, dynamical and spatiotemporal heterogeneities in granular media. Soft Matt. 6, 2875–2883.CrossRefGoogle Scholar
Plimpton, S. 1995 Fast parallel algorithms for short-range molecular dynamics. J.Comput. Phys. 117 (1), 1–19.CrossRefGoogle Scholar
Pournin, L., Ramaioli, M., Folly, P. & Liebling, T.M. 2007 About the influence of friction and polydispersityon the jamming behavior of bead assemblies. Eur. Phys. J. E 23 (2), 229.CrossRefGoogle ScholarPubMed
Reddy, K.A., Talbot, J. & Kumaran, V. 2010 Dynamics of sheared inelastic dumbbells. J.Fluid Mech. 660, 475–498.CrossRefGoogle Scholar
Rubio-Largo, S.M., Janda, A., Maza, D., Zuriguel, I. & Hidalgo, R.C. 2015 Disentangling the free-fall arch paradox in silo discharge. Phys. Rev. Lett. 114, 238002.CrossRefGoogle ScholarPubMed
Serrano, D.A., Medina, A., Chavarria, G.R., Pliego, M. & Klapp, J. 2015 Mass flow rate of granular material flowing from tilted bins. Powder Technol. 286, 438–443.CrossRefGoogle Scholar
Stukowski, A. 2009 Visualization and analysis of atomistic simulation data with OVITO—the open visualization tool. Model. Simul. Mater. Sci. Engng 18 (1), 015012.CrossRefGoogle Scholar
To, K. & Lai, P.-Y. 2002 Jamming pattern in a two-dimensional hopper. Phys. Rev. E 66, 011308.CrossRefGoogle Scholar
Wambaugh, J.F., Reichhardt, C. & Olson, C.J. 2002 Ratchet-induced segregation and transport of nonspherical grains. Phys. Rev. E 65, 031308.CrossRefGoogle ScholarPubMed
Zhou, Y., Ruyer, P. & Aussillous, P. 2015 Discharge flow of a bidisperse granular media from a silo: discrete particle simulations. Phys. Rev. E 92, 062204.CrossRefGoogle ScholarPubMed
Zuriguel, I., Garcimartín, A., Maza, D., Pugnaloni, L.A. & Pastor, J.M. 2005 Jamming during the discharge of granular matter from a silo. Phys. Rev. E 71, 051303.CrossRefGoogle ScholarPubMed
Zuriguel, I., Janda, A., Garcimartín, A., Lozano, C., Arévalo, R. & Maza, D. 2011 Silo clogging reduction by the presence of an obstacle. Phys. Rev. Lett. 107, 278001.CrossRefGoogle ScholarPubMed