Hostname: page-component-586b7cd67f-dsjbd Total loading time: 0 Render date: 2024-12-02T21:52:33.272Z Has data issue: false hasContentIssue false
  • English
  • Français

Global and local statistics in turbulent convection at low Prandtl numbers

Published online by Cambridge University Press:  01 August 2016

Janet D. Scheel  and
Jörg Schumacher
Janet D. Scheel*
Affiliation:
Department of Physics, Occidental College, 1600 Campus Road, M21, Los Angeles, CA 90041, USA
Jörg Schumacher
Affiliation:
Institut für Thermo- und Fluiddynamik, Postfach 100565, Technische Universität Ilmenau, D-98684 Ilmenau, Germany
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Statistical properties of turbulent Rayleigh–Bénard convection at low Prandtl numbers 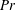$Pr$, which are typical for liquid metals such as mercury or gallium (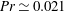$Pr\simeq 0.021$) or liquid sodium (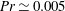$Pr\simeq 0.005$), are investigated in high-resolution three-dimensional spectral element simulations in a closed cylindrical cell with an aspect ratio of one and are compared to previous turbulent convection simulations in air for 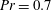$Pr=0.7$. We compare the scaling of global momentum and heat transfer. The scaling exponent $\unicode[STIX]{x1D6FD}$ of the power law 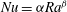$Nu=\unicode[STIX]{x1D6FC}Ra^{\unicode[STIX]{x1D6FD}}$ is 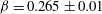$\unicode[STIX]{x1D6FD}=0.265\pm 0.01$ for 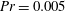$Pr=0.005$ and 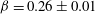$\unicode[STIX]{x1D6FD}=0.26\pm 0.01$ for 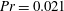$Pr=0.021$, which are smaller than that for convection in air (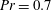$Pr=0.7$, 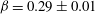$\unicode[STIX]{x1D6FD}=0.29\pm 0.01$). These exponents are in agreement with experiments. Mean profiles of the root-mean-square velocity as well as the thermal and kinetic energy dissipation rates have growing amplitudes with decreasing Prandtl number, which underlies a more vigorous bulk turbulence in the low-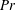$Pr$ regime. The skin-friction coefficient displays a Reynolds number dependence that is close to that of an isothermal, intermittently turbulent velocity boundary layer. The thermal boundary layer thicknesses are larger as 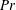$Pr$ decreases and conversely the velocity boundary layer thicknesses become smaller. We investigate the scaling exponents and find a slight decrease in exponent magnitude for the thermal boundary layer thickness as 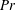$Pr$ decreases, but find the opposite case for the velocity boundary layer thickness scaling. A growing area fraction of turbulent patches close to the heating and cooling plates can be detected by exceeding a locally defined shear Reynolds number threshold. This area fraction is larger for lower 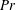$Pr$ at the same 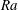$Ra$, but the scaling exponent of its growth with Rayleigh number is reduced. Our analysis of the kurtosis of the locally defined shear Reynolds number demonstrates that the intermittency in the boundary layer is significantly increased for the lower Prandtl number and for sufficiently high Rayleigh number compared to convection in air. This complements our previous findings of enhanced bulk intermittency in low-Prandtl-number convection.

JFM classification

Boundary Layers: Boundary layer structure Turbulent Flows: Turbulent convection
Type
Papers
Information
Journal of Fluid Mechanics , Volume 802 , 10 September 2016 , pp. 147 - 173
Check for updates
Copyright
© 2016 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ahlers, G., He, X., Funfschilling, D. & Bodenschatz, E. 2012 Heat transport by turbulent Rayleigh–Bénard convection for Pr ≃ 0. 8 and 4 × 1012 < Ra < 2 × 1015 : aspect ratio 𝛤 = 0. 50. New J. Phys. 14, 103012,1–39.Google Scholar
Aurnou, J. M. & Olson, P. L. 2001 Experiments on Rayleigh–Bénard convection, magnetoconvection and rotating magnetoconvection in liquid gallium. J. Fluid Mech. 430, 283–307.CrossRefGoogle Scholar
Blasius, H. 1908 Grenzschichten in Flüssigkeiten mit kleiner Reibung. Z. Math. Physik 56, 1–37.Google Scholar
Breuer, M., Wessling, S., Schmalzl, J. & Hansen, U. 2004 Effect of inertia in Rayleigh–Bénard convection. Phys. Rev. E 69, 026302,1–10.Google Scholar
Camussi, R. & Verzicco, R. 1998 Convective turbulence in mercury: scaling laws and spectra. Phys. Fluids 10, 516–527.Google Scholar
Chillà, F. & Schumacher, J. 2012 New perspectives in turbulent Rayleigh–Bénard convection. Eur. Phys. J. E 35, 58,1–25.Google ScholarPubMed
Cioni, S., Ciliberto, S. & Sommeria, J. 1996 Experimental study of high-Rayleigh-number convection in mercury and water. Dyn. Atmos. Oceans 24, 117–127.Google Scholar
Cioni, S., Ciliberto, S. & Sommeria, J. 1997a Strongly turbulent Rayleigh–Bénard convection in mercury: comparison with results at moderate Prandtl number. J. Fluid Mech. 335, 111–140.CrossRefGoogle Scholar
Cioni, S., Horanyi, S., Krebs, L. & Müller, U. 1997b Temperature fluctuation properties in sodium convection. Phys. Rev. E 56, R3753–R3756.Google Scholar
Coles, D. E. & Hirst, E. A. 1969 Computation of turbulent boundary layers. In Proceedings of AFOSR-IFP-Stanford Conference, Stanford University, CA, vol. II, 1968. Thermosciences Division, Stanford University.Google Scholar
Deville, M. O., Fischer, P. F. & Mund, E. H. 2002 High-Order Methods for Incompressible Fluid Flow. Cambridge University Press.CrossRefGoogle Scholar
Glazier, J. A., Segawa, T., Naert, A. & Sano, M. 1999 Evidence against ‘ultrahard’ thermal turbulence at very high Rayleigh numbers. Nature 398, 307–310.CrossRefGoogle Scholar
Fischer, P. F. 1997 An overlapping Schwarz Method for spectral element solution of the incompressible Navier–Stokes equations. J. Comput. Phys. 133, 84–101.CrossRefGoogle Scholar
Frick, P., Khalilov, R., Kolesnichenko, I., Mamykin, A., Pakholkov, V., Pavlinov, A. & Rogozhkin, S. 2015 Turbulent convective heat transfer in a long cylinder with liquid sodium. Europhys. Lett. 109, 14002,1–6.Google Scholar
Grossmann, S. & Lohse, D. 2000 Scaling in thermal convection: a unifying theory. J. Fluid Mech. 407, 27–56.CrossRefGoogle Scholar
Grossmann, S. & Lohse, D. 2001 Thermal convection for large Prandtl numbers. Phys. Rev. Lett. 86, 3316–3319.Google Scholar
Grossmann, S. & Lohse, D. 2011 Multiple scaling in the ultimate regime of thermal convection. Phys. Fluids 23, 045108,1–6.Google Scholar
Grötzbach, G. 2013 Challenges in low-Prandtl number heat transfer simulation and modelling. Nucl. Engng Des. 264, 41–55.Google Scholar
Hanasoge, S., Gizon, L. & Sreenivasan, K. R. 2015 Seismic sounding of convection in the Sun. Annu. Rev. Fluid Mech. 48, 191–217.Google Scholar
He, X., Funfschilling, D., Bodenschatz, E. & Ahlers, G. 2012 Heat transport by turbulent Rayleigh–Bénard convection for Pr ≃ 0. 8 and 4 × 1011 < Ra < 2 × 1014 : ultimate-state transition for aspect ratio 𝛤 = 1. 00. New J. Phys. 14, 063030,1–15.Google Scholar
Horanyi, S., Krebs, L. & Müller, U. 1999 Turbulent Rayleigh–Bénard convection in low-Prandtl number fluids. Intl J. Heat Mass Transfer 42, 3983–4003.Google Scholar
Ishihara, T., Gotoh, T. & Kaneda, Y. 2009 Study of high-Reynolds number isotropic turbulence by direct numerical simulation. Annu. Rev. Fluid Mech. 41, 165–180.Google Scholar
Kelley, D. H. & Sadoway, D. R. 2014 Mixing in a liquid metal electrode. Phys. Fluids 26, 057102,1–12.Google Scholar
Kerr, R. M. 1985 Higher-order derivative correlations and the alignment of small-scale structures in isotropic numerical turbulence. J. Fluid Mech. 153, 31–58.Google Scholar
Kerr, R. M. & Herring, J. R. 2000 Prandtl number dependence of Nusselt number in direct numerical simulations. J. Fluid Mech. 419, 325–344.Google Scholar
King, E. M. & Aurnou, J. M. 2013 Turbulent convection in liquid metal with and without rotation. Proc. Natl Acad. Sci. USA 110, 6688–6693.CrossRefGoogle ScholarPubMed
Landau, L. D. & Lifshitz, E. M. 1987 Fluid Mechanics. Pergamon.Google Scholar
Mishra, P. K. & Verma, M. 2010 Energy spectra and fluxes for Rayleigh–Bénard convection. Phys. Rev. E 81, 056316,1–12.Google ScholarPubMed
Petschel, K., Stellmach, S., Wilczek, M., Lülff, J. & Hansen, U. 2013 Dissipation layers in Rayleigh–Bénard convection: a unifying view. Phys. Rev. Lett. 110, 114502,1–5.Google Scholar
van der Poel, E. P., Stevens, R. J. A. M. & Lohse, D. 2013 Comparison between two- and three-dimensional Rayleigh–Bénard convection. J. Fluid Mech. 736, 177–194.Google Scholar
Prandtl, L. 1905 Über Flüssigkeitsbewegung bei sehr kleiner Reibung. In Verhandlungen des III. Internationalen Mathematiker-Kongresses, Heidelberg, 1904, pp. 484–491. B. G. Teubner.Google Scholar
Rossby, H. T. 1969 A study of Bénard convection with and without rotation. J. Fluid Mech. 36, 309–335.Google Scholar
Scheel, J. D., Emran, M. S. & Schumacher, J. 2013 Resolving the fine-scale structure in turbulent Rayleigh–Bénard convection. New J. Phys. 15, 113063,1–32.CrossRefGoogle Scholar
Scheel, J. D. & Schumacher, J. 2014 Local boundary layer scales in turbulent Rayleigh–Bénard convection. J. Fluid Mech. 758, 344–373.Google Scholar
Schlichting, H. & Gersten, K. 2000 Boundary-Layer Theory, 8th edn. Springer.Google Scholar
Schumacher, J., Götzfried, P. & Scheel, J. D. 2015 Enhanced enstrophy generation for turbulent convection in low-Prandtl number fluids. Proc. Natl Acad. Sci. USA 112, 9530–9535.Google Scholar
Shishkina, O. & Thess, A. 2009 Mean temperature profiles in turbulent Rayleigh–Bénard convection of water. J. Fluid Mech. 633, 449–460.Google Scholar
Stevens, R. J. A. M., van der Poel, E. P., Grossmann, S. & Lohse, D. 2013 The unifying theory of scaling in thermal convection: the updated prefactors. J. Fluid Mech. 730, 295–308.CrossRefGoogle Scholar
Takeshita, T, Segawa, T., Glazier, J. A. & Sano, M. 1996 Thermal turbulence in mercury. Phys. Rev. Lett. 76, 1465–1468.Google Scholar
Tolmien, W. 1929 The production of turbulence. NACA Tech. Mem. 609, 1–35.Google Scholar
Verma, M., Mishra, P. K., Pandey, A. & Paul, S. 2012 Scalings of field correlations and heat transport in turbulent convection. Phys. Rev. E 85, 016310,1–4.Google ScholarPubMed
Verzicco, R. & Camussi, R. 1999 Prandtl number effects in convective turbulence. J. Fluid Mech. 383, 55–73.Google Scholar
Verzicco, R. & Sreenivasan, K. R. 2008 A comparison of turbulent thermal convection between conditions of constant temperature and constant heat flux. J. Fluid Mech. 595, 203–219.Google Scholar
Wagner, S., Shishkina, O. & Wagner, C. 2012 Boundary layers and wind in cylindrical Rayleigh–Bénard cells. J. Fluid Mech. 697, 336–366.Google Scholar

Scheel supplementary movie

Isosurfaces of constant enstrophy for Pr=0.021, Ra=107, and 1×104 < Ω < 1×105. Also shown are the temperature field at cuts through the bottom and top boundary layers.

Download Scheel supplementary movie(Video)
Video 22.5 MB

Scheel supplementary movie

Isosurfaces of constant enstrophy for Pr=0.021, Ra=108, and 3.5×104 Ω < 5×104. Also shown are the temperature field at cuts through the bottom and top boundary layers

Download Scheel supplementary movie(Video)
Video 8.1 MB