Hostname: page-component-586b7cd67f-2plfb Total loading time: 0 Render date: 2024-12-01T00:46:54.690Z Has data issue: false hasContentIssue false
  • English
  • Français

Generation of attached Langmuir circulations by a suspended macroalgal farm

Published online by Cambridge University Press:  19 March 2021

Chao Yan Open the ORCID record for Chao Yan [Opens in a new window] ,
James C. McWilliams Open the ORCID record for James C. McWilliams [Opens in a new window]  and
Marcelo Chamecki Open the ORCID record for Marcelo Chamecki [Opens in a new window]
Chao Yan
Affiliation:
Department of Atmospheric and Oceanic Sciences, University of California, Los Angeles, CA90025, USA
James C. McWilliams
Affiliation:
Department of Atmospheric and Oceanic Sciences, University of California, Los Angeles, CA90025, USA
Marcelo Chamecki*
Affiliation:
Department of Atmospheric and Oceanic Sciences, University of California, Los Angeles, CA90025, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

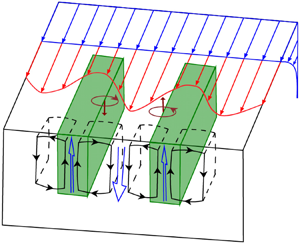
In this study, we focus on Langmuir turbulence in the deep ocean with the presence of a large macroalgal farm using a large eddy simulation method. The wave–current interactions are modelled by solving the wave-averaged equations. The hydrodynamic process over the farm is found to drive a persistent flow pattern similar to Langmuir circulations but is locked in space across the farm. These secondary circulations are generated because the cross-stream shear produced by the rows of canopy elements leads to a steady vertical vorticity field, which is then rotated to the downstream direction under the effect of vortex force. Since the driving mechanism is similar to the Craik–Leibovich type 2 instability theory, these secondary circulations are also termed as attached Langmuir circulations. We then apply a triple decomposition on the flow field to unveil the underlying kinematics and energy transfer between the mean flow, the secondary flow resulting from the farm drag and the transient eddies. Flow visualizations and statistics suggest that the attached Langmuir circulations result from the adjustment of the upper ocean mixed layer to the macroalgal farm, and they will weaken (if not disappear) when the flow reaches an equilibrium state within the farm. The triple-decomposed energy budgets reveal that the energy of the secondary flow is transferred from the mean flow under the action of canopy drag, while the transient eddies feed on wave energy transferred by the Stokes drift and energy conversion from the secondary flow.

JFM classification

Geophysical and Geological Flows: Ocean processes Turbulent Flows: Turbulence simulation Turbulent Flows: Turbulent boundary layers
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 915 , 25 May 2021 , A76
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Abdolahpour, M., Hambleton, M. & Ghisalberti, M. 2017 The wave-driven current in coastal canopies. J. Geophys. Res. 122, 3660–3674.CrossRefGoogle Scholar
Akselsen, A.H. & Ellingsen, S.Å. 2019 Weakly nonlinear transient waves on a shear current: ring waves and skewed Langmuir rolls. J. Fluid Mech. 863, 114–149.CrossRefGoogle Scholar
Akselsen, A.H. & Ellingsen, S.Å. 2020 Langmuir-type vortices in wall-bounded flows driven by a criss-cross wavy wall topography. J. Fluid Mech. 900, A19.CrossRefGoogle Scholar
Aylor, D.E. & Flesch, T.K. 2001 Estimating spore release rates using a Lagrangian stochastic simulation model. J. Appl. Meteorol. 40, 1196–1208.2.0.CO;2>CrossRefGoogle Scholar
Barton, A.D., Ward, B.A., Williams, R.G. & Follows, M.J. 2014 The impact of fine-scale turbulence on phytoplankton community structure. Limnol. Oceanogr. 4 (1), 34–49.CrossRefGoogle Scholar
Belcher, S.E., Jerram, N. & Hunt, J.C.R. 2003 Adjustment of a turbulent boundary layer to a canopy of roughness elements. J. Fluid Mech. 488, 369–398.CrossRefGoogle Scholar
Belcher, S.E., et al. 2012 A global perspective on Langmuir turbulence in the ocean surface boundary layer. Geophys. Res. Lett. 39 (18), L18605.CrossRefGoogle Scholar
Boller, M.L. & Carrington, E. 2006 The hydrodynamic effects of shape and size change during reconfiguration of a flexible macroalga. J. Expl Biol. 209 (10), 1894–1903.CrossRefGoogle ScholarPubMed
Bou-Zeid, E., Meneveau, C. & Parlange, M.B. 2005 A scale-dependent lagrangian dynamic model for large eddy simulation of complex turbulent flows. Phys. Fluids 17, 025105.CrossRefGoogle Scholar
Bradshaw, P. 1987 Turbulent secondary flows. Annu. Rev. Fluid Mech. 19 (1), 53–74.CrossRefGoogle Scholar
Cescatti, A. & Marcolla, B. 2004 Drag coefficient and turbulence intensity in conifer canopies. Agric. Forest Meteorol. 121 (3), 197–206.CrossRefGoogle Scholar
Chamecki, M., Chor, T., Yang, D. & Meneveau, C. 2019 Material transport in the ocean mixed layer: recent developments enabled by large eddy simulations. Rev. Geophys. 57 (4), 1338–1371.CrossRefGoogle Scholar
Charrier, B., Wichard, T. & Reddy, C.R.K. 2018 Protocols for Macroalgae Research. CRC.CrossRefGoogle Scholar
Chen, H., Liu, X. & Zou, Q. 2019 Wave-driven flow induced by suspended and submerged canopies. Adv. Water Resour. 123, 160–172.CrossRefGoogle Scholar
Craik, A.D.D. 1977 The generation of Langmuir circulations by an instability mechanism. J. Fluid Mech. 81 (2), 209–223.CrossRefGoogle Scholar
Craik, A.D.D. & Leibovich, S. 1976 A rational model for Langmuir circulations. J. Fluid Mech. 73 (3), 401–426.CrossRefGoogle Scholar
Dalrymple, R.A., Kirby, J.T. & Hwang, P.A. 1984 Wave diffraction due to areas of energy dissipation. ASCE J. Waterway Port Coastal Ocean Engng 110 (1), 67–79.CrossRefGoogle Scholar
D'Asaro, E.A. 2001 Turbulent vertical kinetic energy in the ocean mixed layer. J. Phys. Oceanogr. 31 (12), 3530–3537.2.0.CO;2>CrossRefGoogle Scholar
Dayton, P.K. 1985 Ecology of kelp communities. Annu. Rev. Ecol. Syst. 16, 215–245.CrossRefGoogle Scholar
Duggins, D.O., Eckman, J.E. & Sewell, A.T. 1990 Ecology of understory kelp environments. II. Effects of kelps on recruitment of benthic invertebrates. J. Expl Mar. Biol. Ecol. 143, 27–45.CrossRefGoogle Scholar
Dupont, S. & Brunet, Y. 2008 Influence of foliar density profile on canopy flow: a large-eddy simulation study. Agric. Forest Meteorol. 148, 976–990.CrossRefGoogle Scholar
Finnigan, J.J., Shaw, R.H. & Patton, E. 2009 Turbulence structure above a vegetation canopy. J. Fluid Mech. 637, 387–424.CrossRefGoogle Scholar
Fram, J.P., Stewart, H.L., Brzezinski, M.A., Gaylord, B., Reed, D.C., Williams, S.L. & MacIntyre, S. 2008 Physical pathways and utilization of nitrate supply to the giant kelp, macrocystis pyrifera. Limnol. Oceanogr. 53 (4), 1589–1603.CrossRefGoogle Scholar
Gaylord, B., et al. 2007 Spatial patterns of flow and their modification within and around a giant kelp forest. Limnol. Oceanogr. 52, 1838–1852.CrossRefGoogle Scholar
Grant, A.L.M. & Belcher, S.E. 2009 Characteristics of Langmuir turbulence in the ocean mixed layer. J. Phys. Oceanogr. 39 (8), 1871–1887.CrossRefGoogle Scholar
Harcourt, R.R. & D'Asaro, E.A. 2008 Large-eddy simulation of Langmuir turbulence in pure wind seas. J. Phys. Oceanogr. 38 (7), 1542–1562.CrossRefGoogle Scholar
Henderson, S.M. 2019 Motion of buoyant, flexible aquatic vegetation under waves: simple theoretical models and parameterization of wave dissipation. Coast. Engng 152, 103497.CrossRefGoogle Scholar
Huai, W.X., Hu, Y., Zeng, Y.H. & Han, J. 2012 Velocity distribution for open channel flows with suspended vegetation. Adv. Water Resour. 49, 56–61.CrossRefGoogle Scholar
Koehl, M.A.R. & Wainwright, S.A. 1977 Mechanical adaptations of a giant kelp. Limnol. Oceanogr. 22, 1067–1071.CrossRefGoogle Scholar
Kukulka, T., Plueddemann, A.J., Trowbridge, J.H. & Sullivan, P.P. 2010 Rapid mixed layer deepening by the combination of Langmuir and shear instabilities: a case study. J. Phys. Oceanogr. 40 (11), 2381–2400.CrossRefGoogle Scholar
Legg, B.J. & Powell, F.A. 1979 Spore dispersal in a barley crop: a mathematical model. Agric. Meteorol. 20 (1), 47–67.CrossRefGoogle Scholar
Leibovich, S. 1977 Convective instability of stably stratified water in the ocean. J. Fluid Mech. 82 (3), 561–581.CrossRefGoogle Scholar
Leibovich, S. 1983 The form and dynamics of Langmuir circulations. Annu. Rev. Fluid Mech. 15 (1), 391–427.CrossRefGoogle Scholar
Luhar, M., Coutu, S., Infantes, E., Fox, S. & Nepf, H. 2010 Wave-induced velocities inside a model seagrass bed. J. Geophys. Res. 115 (C12), C12005.CrossRefGoogle Scholar
Luhar, M., Infantes, E., Orfila, A., Terrados, J. & Nepf, H. 2013 Field observations of wave-induced streaming through a submerged seagrass (Posidonia oceanica) meadow. J. Geophys. Res. 118, 1955–1968.CrossRefGoogle Scholar
Luhar, M. & Nepf, H.M. 2011 Flow-induced reconfiguration of buoyant and flexible aquatic vegetation. Limnol. Oceanogr. 56 (6), 2003–2017.CrossRefGoogle Scholar
Marcolla, B., Pitacco, A. & Cescatti, A. 2003 Canopy architecture and turbulence structure in a coniferous forest. Boundary-Layer Meteorol. 108, 39–59.CrossRefGoogle Scholar
McWilliams, J. 2006 Fundamentals of Geophysical Fluid Dynamics. Cambridge University Press.Google Scholar
McWiliams, J., Sullivan, P. & Moeng, C. 1997 Langmuir turbulence in the ocean. J. Fluid Mech. 334, 1–30.CrossRefGoogle Scholar
Monismith, S.G. & Fong, D.A. 2004 A note on the potential transport of scalars and organisms by surface waves. Limnol. Oceanogr. 49, 1214–1217.CrossRefGoogle Scholar
Nepf, H.M. 2012 a Flow and transport in regions with aquatic vegetation. Annu. Rev. Fluid Mech. 44, 123–142.CrossRefGoogle Scholar
Nepf, H.M. 2012 b Hydrodynamics of vegetated channels. J. Hydraul Res. 50, 262–279.CrossRefGoogle Scholar
Pan, Y., Chamecki, M. & Isard, S.A. 2014 Large-eddy simulation of turbulence and particle dispersion inside the canopy roughness sublayer. J. Fluid Mech. 753, 499–534.CrossRefGoogle Scholar
Pan, Y., Chamecki, M. & Nepf, H.M. 2016 Estimating the instantaneous drag-wind relationship for a horizontally homogeneous canopy. Boundary-Layer Meteorol. 160, 63–82.CrossRefGoogle Scholar
Pinard, J.D. & Wilson, J.D. 2001 First- and second-order closure models for wind in a plant canopy. J. Appl. Meteorol. 40 (10), 1762–1768.2.0.CO;2>CrossRefGoogle Scholar
Plew, D.R. 2011 a Depth-averaged drag coefficient for modeling flow through suspended canopies. J. Hydraul. Engng ASCE 137, 234–247.CrossRefGoogle Scholar
Plew, D.R. 2011 b Shellfish farm-induced changes to tidal circulation in an embayment, and implications for seston depletion. Aquacult. Env. Interac. 1, 201–214.CrossRefGoogle Scholar
Plew, D.R., Spigel, R.H., Stevens, C.L., Nokes, R.I. & Davidson, M.J. 2006 Stratified flow interactions with a suspended canopy. Environ. Fluid Mech. 6, 519–539.CrossRefGoogle Scholar
Plew, D.R., Stevens, C.L., Spigel, R.H. & Hartstein, N.D. 2005 Hydrodynamic implications of large offshore mussel farms. IEEE J. Ocean Engng 30, 95–108.CrossRefGoogle Scholar
Polton, J.A., Smith, J.A., MacKinnon, J.A. & Tejada-Martínez, A.E. 2008 Rapid generation of high-frequency internal waves beneath a wind and wave forced oceanic surface mixed layer. Geophys. Res. Lett. 35 (13), L13602.CrossRefGoogle Scholar
Pope, S.B. 2000 Turbulent Flows. Cambridge University Press.CrossRefGoogle Scholar
Rominger, J.T. & Nepf, H.M. 2011 Flow adjustment and interior flow associated with a rectangular porous obstruction. J. Fluid Mech. 680, 636–659.CrossRefGoogle Scholar
van Rooijen, A., Lowe, R., Rijnsdorp, D., Ghisalberti, M., Jacobsen, N.G. & McCall, R. 2020 Wave-driven mean flow dynamics in submerged canopies. J. Geophys. Res. 125, e2019JC015935.Google Scholar
Rosman, J.H., Denny, M.W., Zeller, R.B., Monismith, S.G. & Koseff, J.R. 2013 Interaction of waves and currents with kelp forests (Macrocystis pyrifera): insights from a dynamically scaled laboratory model. Limnol. Oceanogr. 58, 790–802.CrossRefGoogle Scholar
Rosman, J.H., Koseff, J.R., Monismith, S.G. & Grover, J. 2007 A field investigation into the effects of a kelp forest (Macrocystis pyrifera) on coastal hydrodynamics and transport. J. Geophys. Res. 112, C02016.CrossRefGoogle Scholar
Rosman, J.H., Monismith, S.G., Denny, M.W. & Koseff, J.R. 2010 Currents and turbulence within a kelp forest (Macrocystis pyrifera): insights from a dynamically scaled laboratory model. Limnol. Oceanogr. 55 (3), 1145–1158.CrossRefGoogle Scholar
Schiel, D.R. & Forster, M.S. 2015 The Biology and Ecology of Giant Kelp Forests. University of California Press.CrossRefGoogle Scholar
Shaw, R.H. & Schumann, U. 1992 Large-eddy simulation of turbulent flow above and within a forest. Boundary-Layer Meteorol. 61, 47–64.CrossRefGoogle Scholar
Shrestha, K. & Anderson, W. 2019 Coastal Langmuir circulations induce phase-locked modulation of bathymetric stress. Environ. Fluid Mech. 20, 873–884.CrossRefGoogle Scholar
Skitka, J., Marston, J.B. & Fox-Kemper, B. 2020 Reduced-order quasilinear model of ocean boundary-layer turbulence. J. Phys. Oceanogr. 50 (3), 537–558.CrossRefGoogle Scholar
Skyllingstad, E.D. & Denbo, D.W. 1995 An ocean large-eddy simulation of Langmuir circulations and convection in the surface mixed layer. J. Geophys. Res. 100, 8501–8522.CrossRefGoogle Scholar
Stevens, C. & Plew, D. 2019 Bridging the separation between studies of the biophysics of natural and built marine canopies. Front. Mar. Sci. 6, 217.CrossRefGoogle Scholar
Stevens, C.L. & Petersen, J.K. 2011 Turbulent, stratified flow through a suspended shellfish canopy: implications for mussel farm design. Aquacult. Env. Interac. 36, 87–104.CrossRefGoogle Scholar
Stevens, R.J.A.M., Graham, J. & Meneveau, C. 2014 A concurrent precursor inflow method for large eddy simulations and applications to finite length wind farms. Renew. Energy 68, 46–50.CrossRefGoogle Scholar
Sullivan, P.P., Moeng, C.H., Stevens, B., Lenschow, D.H. & Mayor, S.D. 1998 Structure of the entrainment zone capping the convective atmospheric boundary layer. J. Atmos. Sci. 55, 3042–3064.2.0.CO;2>CrossRefGoogle Scholar
Suzuki, N. & Fox-Kemper, B. 2016 Understanding stokes forces in the wave-averaged equations. J. Geophys. Res. 121 (5), 3579–3596.CrossRefGoogle Scholar
Taylor, J.R. & Sarkar, S. 2008 Stratification effects in a bottom Ekman layer. J. Phys. Oceanogr. 38 (11), 2535–2555.CrossRefGoogle Scholar
Thorpe, S.A. 2004 Langmuir circulation. Annu. Rev. Fluid Mech. 36 (1), 55–79.CrossRefGoogle Scholar
Troell, M., Joyce, A., Chopin, T., Neori, A., Buschmann, A.H. & Fang, J.G. 2009 Ecological engineering in aquaculture - potential for integrated multi-trophic aquaculture (IMTA) in marine offshore systems. Aquaculture 297, 1–9.CrossRefGoogle Scholar
Tseung, H.L., Kikkert, G.A. & Plew, D. 2016 Hydrodynamics of suspended canopies with limited length and width. Environ. Fluid Mech. 16, 145–166.CrossRefGoogle Scholar
Utter, B. & Denny, M. 1996 Wave-induced forces on the giant kelp Macrocystis pyrifera (Agardh): field test of a computational model. J. Expl Biol. 199, 2645–2654.Google ScholarPubMed
Van Roekel, L.P., Fox-Kemper, B., Sullivan, P.P., Hamlington, P.E. & Haney, S.R. 2012 The form and orientation of Langmuir cells for misaligned winds and waves. J. Geophys. Res. 117, C05001.CrossRefGoogle Scholar
Vogel, S. 1989 Drag and reconfiguration of broad leaves in high winds. J. Expl Bot. 40, 941–948.CrossRefGoogle Scholar
Yan, C., Nepf, H.M., Huang, W. & Cui, G. 2017 Large eddy simulation of flow and scalar transport in a vegetated channel. Environ. Fluid Mech. 17, 497–519.CrossRefGoogle Scholar
Yang, D., Chen, B., Chamecki, M. & Meneveau, C. 2015 Oil plumes and dispersion in Langmuir, upper-ocean turbulence: large-eddy simulations and k-profile parameterization. J. Geophys. Res. 120, 4729–4759.CrossRefGoogle Scholar
Zhao, F.X., Huai, W. & Li, D. 2017 Numerical modeling of open channel flow with suspended canopy. Adv. Water Resour. 105, 132–143.CrossRefGoogle Scholar
Zhou, J. & Venayagamoorthy, S. 2019 Near-field mean flow dynamics of a cylindrical canopy patch suspended in deep water. J. Fluid Mech. 858, 634–655.CrossRefGoogle Scholar