Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Kozlov, V.
Kuznetsov, N.
and
Lokharu, E.
2015.
On bounds and non-existence in the problem of steady waves with vorticity.
Journal of Fluid Mechanics,
Vol. 765,
Issue. ,
Strauss, Walter A.
and
Wheeler, Miles H.
2016.
Bound on the Slope of Steady Water Waves with Favorable Vorticity.
Archive for Rational Mechanics and Analysis,
Vol. 222,
Issue. 3,
p.
1555.
Chen, Robin Ming
Walsh, Samuel
and
Wheeler, Miles H.
2016.
On the existence and qualitative theory of stratified solitary water waves.
Comptes Rendus. Mathématique,
Vol. 354,
Issue. 6,
p.
601.
Chen, Robin Ming
and
Walsh, Samuel
2016.
Continuous Dependence on the Density for Stratified Steady Water Waves.
Archive for Rational Mechanics and Analysis,
Vol. 219,
Issue. 2,
p.
741.
Kozlov, V.
Kuznetsov, N.
and
Lokharu, E.
2017.
On the Benjamin–Lighthill conjecture for water waves with vorticity.
Journal of Fluid Mechanics,
Vol. 825,
Issue. ,
p.
961.
Walsh, Samuel
Wheeler, Miles H.
and
Chen, Robin Ming
2018.
Existence and qualitative theory for stratified solitary water waves.
Annales de l'Institut Henri Poincaré C, Analyse non linéaire,
Vol. 35,
Issue. 2,
p.
517.
Basu, Biswajit
2018.
Solitary water waves propagating on an underlying uniform current over a finite depth.
Nonlinear Analysis,
Vol. 172,
Issue. ,
p.
25.
So, Seung Wook
and
Strauss, Walter A.
2018.
Upper bound on the slope of steady water waves with small adverse vorticity.
Journal of Differential Equations,
Vol. 264,
Issue. 6,
p.
4136.
Flamarion, Marcelo V.
Milewski, Paul A.
and
Nachbin, André
2019.
Rotational waves generated by current‐topography interaction.
Studies in Applied Mathematics,
Vol. 142,
Issue. 4,
p.
433.
Akers, Adelaide
and
Walsh, Samuel
2019.
Solitary water waves with discontinuous vorticity.
Journal de Mathématiques Pures et Appliquées,
Vol. 124,
Issue. ,
p.
220.
Kozlov, V.
Kuznetsov, N.
and
Lokharu, E.
2020.
Solitary waves on constant vorticity flows with an interior stagnation point.
Journal of Fluid Mechanics,
Vol. 904,
Issue. ,
Kozlov, V. A.
and
Lokharu, E. E.
2021.
On Rotational Waves of Limit Amplitude.
Functional Analysis and Its Applications,
Vol. 55,
Issue. 2,
p.
165.
Sinambela, Daniel
2021.
Large-Amplitude Solitary Waves in Two-Layer Density Stratified Water.
SIAM Journal on Mathematical Analysis,
Vol. 53,
Issue. 4,
p.
4812.
Kozlov, Vladimir
Lokharu, Evgeniy
and
Wheeler, Miles H.
2021.
Nonexistence of Subcritical Solitary Waves.
Archive for Rational Mechanics and Analysis,
Vol. 241,
Issue. 1,
p.
535.
Kozlov, Vladimir Arkad'evich
and
Lokharu, E E
2021.
О завихренных волнах предельной амплитуды.
Функциональный анализ и его приложения,
Vol. 55,
Issue. 2,
p.
107.
Lokharu, Evgeniy
2021.
On bounds for steady waves with negative vorticity.
Journal of Mathematical Fluid Mechanics,
Vol. 23,
Issue. 2,
Kozlov, Vladimir
and
Lokharu, Evgeniy
2023.
Global Bifurcation and Highest Waves on Water of Finite Depth.
Archive for Rational Mechanics and Analysis,
Vol. 247,
Issue. 5,
Kozlov, Vladimir
2023.
The subharmonic bifurcation of Stokes waves on vorticity flow.
Journal of Differential Equations,
Vol. 361,
Issue. ,
p.
183.
Haziot, Susanna V.
and
Wheeler, Miles H.
2023.
Large-Amplitude Steady Solitary Water Waves with Constant Vorticity.
Archive for Rational Mechanics and Analysis,
Vol. 247,
Issue. 2,
Kozlov, Vladimir
2024.
On the first subharmonic bifurcations in a branch of Stokes water waves.
Journal of Differential Equations,
Vol. 379,
Issue. ,
p.
676.
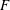 $F$ must be strictly greater than the critical value
$F$ must be strictly greater than the critical value  $F=1$. We also prove a related upper bound on
$F=1$. We also prove a related upper bound on  $F$, and hence on the amplitude, under more restrictive assumptions on the vorticity.
$F$, and hence on the amplitude, under more restrictive assumptions on the vorticity.