Hostname: page-component-586b7cd67f-dlnhk Total loading time: 0 Render date: 2024-11-24T15:56:22.772Z Has data issue: false hasContentIssue false
  • English
  • Français

Free-stream turbulence and the development of cross-flow disturbances

Published online by Cambridge University Press:  24 October 2013

Robert S. Downs III  and
Edward B. White
Robert S. Downs III
Affiliation:
Department of Aerospace Engineering, Texas A&M University, College Station, TX 77843, USA
Edward B. White*
Affiliation:
Department of Aerospace Engineering, Texas A&M University, College Station, TX 77843, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The cross-flow instability that arises in swept-wing boundary layers has resisted attempts to describe the path from disturbance initiation to transition. Following concerted research efforts, surface roughness and free-stream turbulence have been identified as the leading providers of initial disturbances for cross-flow instability growth. Although a significant body of work examines the role of free-stream turbulence in the cross-flow problem, the data more relevant to the flight environment (turbulence intensities less than 0.07 %) are sparse. A series of recent experiments indicates that variations within this range may affect the initiation or growth of cross-flow instability amplitudes, hindering comparison among results obtained in different disturbance environments. To address this problem, a series of wind tunnel experiments is performed in which the free-stream turbulence intensity is varied between 0.02 % and 0.2 % of free-stream velocity, 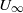${U}_{\infty } $. Measurements of the stationary and travelling mode amplitudes are made in the boundary layer of a 1.83 m chord, 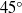$45{{}^\circ} $ swept-wing model. These results are compared to those of similar experiments at higher turbulence levels to broaden the current knowledge of this portion of the cross-flow problem. It is observed that both free-stream turbulence and surface roughness contribute to the initiation of unsteady disturbances, and that free-stream turbulence affects the development of both stationary and unsteady cross-flow disturbances. For the range tested, enhanced free-stream turbulence advances the transition location except when a subcritically spaced roughness array is employed.

JFM classification

Boundary Layers: Boundary Layers Boundary Layers: Boundary layer receptivity Boundary Layers: Boundary layer stability
Type
Papers
Information
Journal of Fluid Mechanics , Volume 735 , 25 November 2013 , pp. 347 - 380
Copyright
©2013 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bippes, H. 1999 Basic experiments on transition in three-dimensional boundary layers dominated by crossflow instability. Prog. Aerosp. Sci. 35 (4), 363–412.CrossRefGoogle Scholar
Bippes, H. & Lerche, T. 1997 Transition prediction in three-dimensional boundary-layer flows unstable to crossflow instability. AIAA Paper 97-1906.Google Scholar
Borodulin, V. I., Ivanov, A. V., Kachanov, Y. S. & Roschektaev, A. P. 2013 Receptivity coefficients at excitation of cross-flow waves by free stream vortices in the presence of surface roughness. J. Fluid Mech. 716, 487–527.Google Scholar
Carpenter, A. L., Saric, W. S. & Reed, H. L. 2010 Roughness receptivity in swept-wing boundary layers–experiments. Intl J. Engng Syst. Model. Simul. 2 (1/2), 128–138.Google Scholar
Carrillo, R. B. 1996 Distributed-roughness effects on stability and transition in swept-wing boundary layers. Master’s thesis, Arizona State University.Google Scholar
Chernyshev, S. L., Ivanov, A. I., Kiselev, A. P., Kuzminsky, V. A., Sboev, D. S. & Zhigulev, S. V. 2011 Investigations of influence of free stream turbulence and acoustic disturbances on the laminar–turbulent transition on LV6 aerofoil and swept wing models. AIAA Paper 2011-210.Google Scholar
Dagenhart, J. R. & Saric, W. S. 1999 Crossflow stability and transition experiments in swept-wing flow. NASA Tech. Pub. 1999-209344.Google Scholar
Deyhle, H. & Bippes, H. 1996 Disturbance growth in an unstable three-dimensional boundary layer and its dependence on environmental conditions. J. Fluid Mech. 316, 73–113.CrossRefGoogle Scholar
Deyhle, H., Höhler, G. & Bippes, H. 1993 Experimental investigation of instability wave propagation in a three-dimensional boundary-layer flow. AIAA J. 31 (4), 637–645.CrossRefGoogle Scholar
Downs, R. S. 2012 Environmental influences on crossflow instability. PhD thesis, Texas A&M University.Google Scholar
Downs, R. S., Lovig, E. N. & White, E. B. 2012 Experimental investigation of the crossflow instability in moderate free-stream turbulence. AIAA Paper 2012-2824.CrossRefGoogle Scholar
Eppink, J. & Wlezien, R. 2011 Data analysis for the NASA/Boeing hybrid laminar flow control crossflow experiment. AIAA Paper 2011-3879.Google Scholar
Ergin, F. G. & White, E. B. 2005 Multicomponent and unsteady velocity measurements of transient disturbances. AIAA Paper 2005-527.Google Scholar
Fischer, T. M. & Dallmann, U. 1991 Primary and secondary stability analysis of a three-dimensional boundary-layer flow. Phys. Fluids A 3 (10), 2378–2391.Google Scholar
Fransson, J. H. M., Matsubara, M. & Alfredsson, P. H. 2005 Transition induced by free stream turbulence. J. Fluid Mech. 527, 1–25.CrossRefGoogle Scholar
Gaponenko, V. R., Ivanov, A. V., Kachanov, Y. S. & Crouch, J. D. 2002 Swept-wing boundary-layer receptivity to surface non-uniformities. J. Fluid Mech. 461, 93–126.CrossRefGoogle Scholar
Gladden, R. D. 2001 Crossflow transition in elevated free stream turbulence. Master’s thesis, Arizona State University.Google Scholar
Green, J. E. 2008 Laminar flow control – back to the future? AIAA Paper 2008-3738.Google Scholar
Haynes, T. S. & Reed, H. L. 2000 Simulation of swept-wing vortices using nonlinear parabolized stability equations. J. Fluid Mech. 405, 325–349.Google Scholar
Högberg, M. & Henningson, D. 1998 Secondary instability of cross-flow vortices in Falkner–Skan–Cooke boundary layers. J. Fluid Mech. 368, 339–357.CrossRefGoogle Scholar
Hosseini, S. M., Tempelmann, D., Hanifi, A. & Henningson, D. S. 2013 Stabilization of a swept-wing boundary layer by distributed roughness elements. J. Fluid Mech. 718, R1-1–R1-11.CrossRefGoogle Scholar
Hunt, L. E. 2011 Boundary-layer receptivity to three-dimensional roughness arrays on a swept-wing. PhD thesis, Texas A&M University.Google Scholar
Hunt, L. E., Downs, R. S., Kuester, M. S., White, E. B. & Saric, W. S. 2010 Flow quality measurements in the Klebanoff–Saric wind tunnel. AIAA Paper 2010-4538.CrossRefGoogle Scholar
Hunt, L. E. & Saric, W. S. 2011 Boundary-layer receptivity of three-dimensional roughness arrays on a swept-wing. AIAA Paper 2011-3881.Google Scholar
Kawakami, M., Kohama, Y. & Okutsu, M. 1999 Stability characteristics of stationary crossflow vortices in three-dimensional boundary layer. AIAA Paper 99-0811.Google Scholar
Kohama, Y., Saric, W. S. & Hoos, J. A. 1991 A high-frequency, secondary instability of crossflow vortices that leads to transition. In Proc. of the Royal Aero. Soc. Conf. on Boundary-Layer Trans. and Control, pp. 1–12.Google Scholar
Kurian, T. & Fransson, J. H. M. 2009 Grid-generated turbulence revisited. Fluid Dyn. Res. 41 (2), 021403.Google Scholar
Kurian, T., Fransson, J. H. M. & Alfredsson, P. H. 2011 Boundary layer receptivity to free stream turbulence and surface roughness over a swept flat plate. Phys. Fluids 23 (3), 034107.CrossRefGoogle Scholar
Laws, E. M. & Livesey, J. L. 1978 Flow through screens. Annu. Rev. Fluid Mech. 10, 247–266.Google Scholar
Li, F., Choudhari, M., Chang, C.-L., Streett, C. & Carpenter, M. 2011 Computational modelling of roughness-based laminar flow control on a subsonic swept wing. AIAA J. 49 (3), 520–529.Google Scholar
Malik, M. R., Li, F., Choudhari, M. M. & Chang, C.-L. 1999 Secondary instability of crossflow vortices and swept-wing boundary-layer transition. J. Fluid Mech. 399, 85–115.Google Scholar
Morkovin, M. V. 1969 On the many faces of transition. In Viscous Drag Reduction (ed. Wells, C. S.), pp. 1–31. Plenum.Google Scholar
Müller, B. & Bippes, H. 1989 Experimental study of instability modes in a three-dimensional boundary layer. In Fluid Dynamics of Three-Dimensional Turbulent Shear Flows and Transition, AGARD CP 438, pp. 1–15. NATO AGARD.Google Scholar
Naguib, A. M., Gravante, S. P. & Wark, C. E. 1996 Extraction of turbulent wall-pressure time-series using an optimal filtering scheme. Exp. Fluids 22 (1), 14–22.Google Scholar
Nishino, T. & Shariff, K. 2010 Direct numerical simulation of a swept-wing boundary layer with an array of discrete roughness elements. In Seventh IUTAM Symposium on Laminar-Turbulent Transition (ed. Schlatter, P. & Henningson, D. S.), pp. 289–294. Springer.Google Scholar
Press, W. H., Teukolsky, S. A., Vetterling, W. T. & Flannery, B. P. 2007 Numerical Recipes: The Art of Scientific Computing, 3rd edn. Cambridge University Press.Google Scholar
Radeztsky, R. H., Reibert, M. S. & Saric, W. S. 1994 Development of stationary crossflow vortices on a swept wing. AIAA Paper 94-2373.Google Scholar
Radeztsky, R. H., Reibert, M. S. & Saric, W. S. 1999 Effect of isolated micron-sized roughness on transition in swept-wing flows. AIAA J. 37 (11), 1370–1377.Google Scholar
Radeztsky, R. H., Reibert, M. S. & Takagi, S. 1993 A software solution to temperature-induced hot-wire voltage drift. In Proceedings of the Third International Symposium on Thermal Anemometry (ed. Stock, D. E.), pp. 49–55.Google Scholar
Reed, H. L. & Saric, W. S. 1989 Stability of three-dimensional boundary layers. Annu. Rev. Fluid Mech. 21, 235–284.Google Scholar
Reibert, M. S. 1996 Nonlinear stability, saturation, and transition in crossflow-dominated boundary layers. PhD thesis, Arizona State University.Google Scholar
Reibert, M. S. & Saric, W. S. 1997 Review of swept-wing transition. AIAA Paper 97-1816.Google Scholar
Reibert, M. S., Saric, W. S., Carrillo, R. B. & Chapman, K. L. 1996 Experiments in nonlinear saturation of stationary crossflow vortices in a swept-wing boundary layer. AIAA Paper 96-0184.Google Scholar
Reshotko, E. 1975 A program for transition research. AIAA J. 13 (3), 261–265.Google Scholar
Reshotko, E., Saric, W. S. & Nagib, H. M. 1997 Flow quality issues for large wind tunnels. AIAA Paper 97-0225.Google Scholar
Riedel, H. & Sitzmann, M. 1998 In-flight investigations of atmospheric turbulence. Aerosp. Sci. Technol. 2 (5), 301–319.CrossRefGoogle Scholar
Saric, W. S. 1992 The ASU transition research facility. AIAA Paper 92-3910.Google Scholar
Saric, W. S. 2007 Boundary-layer stability and transition. In Springer Handbook of Experimental Fluid Mechanics (ed. Tropea, C., Yarin, A. L. & Foss, J. F.), pp. 886–896. Springer.Google Scholar
Saric, W. S., Carpenter, A. L. & Reed, H. L. 2011 Passive control of transition in three-dimensional boundary layers, with emphasis on discrete roughness elements. Phil. Trans. R. Soc. Lond. A 369 (1940), 1352–1364.Google ScholarPubMed
Saric, W. S., Carrillo, R. B. & Reibert, M. S. 1998 Nonlinear stability and transition in 3-D boundary layers. Meccanica 33 (5), 469–487.Google Scholar
Saric, W. S., Reed, H. L. & Kerschen, E. J. 2002 Boundary-layer receptivity to free stream disturbances. Annu. Rev. Fluid Mech. 34, 291–319.CrossRefGoogle Scholar
Saric, W. S., Reed, H. L. & White, E. B. 2003 Stability and transition of three-dimensional boundary layers. Annu. Rev. Fluid Mech. 35, 413–440.Google Scholar
Schrader, L.-U., Amin, S. & Brandt, L. 2010 Transition to turbulence in the boundary layer over a smooth and rough swept plate exposed to free stream turbulence. J. Fluid Mech. 646, 297–325.Google Scholar
Schrader, L.-U., Brandt, L. & Henningson, D. S. 2009 Receptivity mechanisms in three-dimensional boundary-layer flows. J. Fluid Mech. 618, 209–241.CrossRefGoogle Scholar
Suder, K. L., O’Brien, J. E. & Reshotko, E. 1988 Experimental study of bypass transition in a boundary layer. NASA Tech. Mem. 100913.Google Scholar
Takagi, S. & Itoh, N. 1994 Observation of travelling waves in the three-dimensional boundary layer along a yawed cylinder. Fluid Dyn. Res. 14 (4), 167–189.Google Scholar
Tan-atichat, J., Nagib, H. M. & Drubka, R. E. 1980 Effects of axisymmetric contractions on turbulence of various scales. NASA Contr. Rep. 165136.Google Scholar
Tempelmann, D., Hanifi, A. & Henningson, D. S. 2012a Swept-wing boundary-layer receptivity. J. Fluid Mech. 700, 490–501.Google Scholar
Tempelmann, D., Schrader, L.-U., Hanifi, A., Brandt, L. & Henningson, D. S. 2012b Swept wing boundary-layer receptivity to localized surface roughness. J. Fluid Mech. 711, 516–544.CrossRefGoogle Scholar
Tennekes, H. & Lumley, J. L. 1972 A First Course in Turbulence. MIT Press.Google Scholar
Wassermann, P. & Kloker, M. 2002 Mechanisms and passive control of crossflow-vortex-induced transition in a three-dimensional boundary layer. J. Fluid Mech. 456, 49–84.CrossRefGoogle Scholar
Westin, K. J. A., Boiko, A. V., Klingmann, B. G. B., Kozlov, V. V. & Alfredsson, P. H. 1994 Experiments in a boundary layer subjected to free stream turbulence. Part 1. Boundary layer structure and receptivity. J. Fluid Mech. 281, 193–218.Google Scholar
White, E. B. 2000 Breakdown of crossflow vortices. PhD thesis, Arizona State University.Google Scholar
White, E. B. & Ergin, F. G. 2004 Using laminar-flow velocity profiles to locate the wall behind roughness elements. Exp. Fluids 36 (5), 805–812.Google Scholar
White, E. B. & Saric, W. S. 2005 Secondary instability of crossflow vortices. J. Fluid Mech. 525, 275–308.Google Scholar
White, E. B., Saric, W. S., Gladden, R. D. & Gabet, P. M. 2001 Stages of swept-wing transition. AIAA Paper 2001-0271.Google Scholar
Wlezien, R. W., Spencer, S. A. & Grubb, J. P. 1994 Comparison of flow quality in subsonic pressure tunnels. AIAA Paper 94-2503.Google Scholar