Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Nagata, R.
Watanabe, T.
and
Nagata, K.
2018.
Turbulent/non-turbulent interfaces in temporally evolving compressible planar jets.
Physics of Fluids,
Vol. 30,
Issue. 10,
Zhang, Xinxian
Watanabe, Tomoaki
and
Nagata, Koji
2019.
Passive scalar mixing near turbulent/non-turbulent interface in compressible turbulent boundary layers.
Physica Scripta,
Vol. 94,
Issue. 4,
p.
044002.
Zhuang, Yi
Tan, Huijun
Wang, Weixing
Li, Xin
and
Guo, Yunjie
2019.
Fractal features of turbulent/non-turbulent interface in a shock wave/turbulent boundary-layer interaction flow.
Journal of Fluid Mechanics,
Vol. 869,
Issue. ,
Kohan, Khashayar F.
and
Gaskin, Susan
2020.
The effect of the geometric features of the turbulent/non-turbulent interface on the entrainment of a passive scalar into a jet.
Physics of Fluids,
Vol. 32,
Issue. 9,
Ma, Kai
Li, Jiang
Li, Qiang
and
Liu, Yang
2020.
Experimental study on evolution characteristics of plane subsonic-supersonic shear layer.
Aerospace Science and Technology,
Vol. 100,
Issue. ,
p.
105791.
Ghasemi, Abbas
Yun, Sangsig
and
Li, Xianguo
2021.
Fractal structures arising from interfacial instabilities in bio-oil atomization.
Scientific Reports,
Vol. 11,
Issue. 1,
Zhang, Xinxian
2021.
Frontiers of Digital Transformation.
p.
155.
Gang, Dundian
Yi, Shi-he
and
Niu, Haibo
2021.
Visualization of supersonic flow over a rotating blunt cylinder at a high angle of attack.
Journal of Visualization,
Vol. 24,
Issue. 6,
p.
1123.
Tang, M.X.
Wu, Y.
Zong, H.H.
Luo, Y.H.
Yang, H.S.
and
Guo, S.G.
2022.
Experimental investigation of supersonic boundary-layer tripping with a spanwise pulsed spark discharge array.
Journal of Fluid Mechanics,
Vol. 931,
Issue. ,
GANG, Dundian
YI, Shihe
ZHANG, Feng
and
NIU, Haibo
2022.
Effects of sweep angles on turbulent separation behaviors induced by blunt fin.
Chinese Journal of Aeronautics,
Vol. 35,
Issue. 3,
p.
90.
Zhang, Xinxian
Watanabe, Tomoaki
and
Nagata, Koji
2023.
Reynolds number dependence of the turbulent/non-turbulent interface in temporally developing turbulent boundary layers.
Journal of Fluid Mechanics,
Vol. 964,
Issue. ,
Hu, Yufa
Yi, Shihe
Liu, Xiaolin
and
Tian, Xudong
2024.
Effect of wall-seeping gas film on hypersonic laminar and transitional boundary layers.
Physics of Fluids,
Vol. 36,
Issue. 12,
Huang, Jianda
and
Bai, Honglei
2024.
Turbulent/non-turbulent interface of the turbulent boundary-layer over a spanwise-heterogeneous converging/diverging riblets rough wall.
Fluid Dynamics Research,
Vol. 56,
Issue. 2,
p.
025503.
Su, Shuhuai
Long, Yanguang
Wang, Jinjun
and
Li, Xinliang
2024.
Investigations on the turbulentnon-turbulent interface in supersonic compressible plate turbulent boundary layer.
Journal of Fluid Mechanics,
Vol. 988,
Issue. ,
Liu, Xiaolin
and
Yi, Shihe
2024.
Experimental investigation about the aerodynamic noise caused by hypersonic turbulence.
Physics of Fluids,
Vol. 36,
Issue. 1,
Ding, Hao
Tian, Lifeng
Wang, Ao
Huang, Kaiyou
and
Fu, Shuangxu
2025.
Structural characteristics of a supersonic turbulent boundary layer subject to convex–concave curvature coupling.
Physics of Fluids,
Vol. 37,
Issue. 2,
Sood, Pranav
Bhatt, Shibani
Choudhary, Harish
Gupta, Abhishek
Raghuvanshi, Neetesh Singh
Sapkal, Prajyot
Prabhakaran, Thara
and
Dixit, Shivsai Ajit
2025.
Experiments on scalar interface in plane turbulent wall jet: Statistics and fractality.
Physics of Fluids,
Vol. 37,
Issue. 1,
Watanabe, Tomoaki
and
Nagata, Koji
2025.
Influences of small-scale shear instability on passive-scalar mixing in a shear-free turbulent front.
Journal of Fluid Mechanics,
Vol. 1008,
Issue. ,
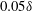 $0.05\unicode[STIX]{x1D6FF}$.
$0.05\unicode[STIX]{x1D6FF}$.

