Hostname: page-component-78c5997874-v9fdk Total loading time: 0 Render date: 2024-11-07T21:21:59.444Z Has data issue: false hasContentIssue false
  • English
  • Français

Fokker-Planck-Poisson kinetics: multi-phase flow beyond equilibrium

Published online by Cambridge University Press:  16 June 2021

Mohsen Sadr Open the ORCID record for Mohsen Sadr [Opens in a new window] ,
Marcel Pfeiffer Open the ORCID record for Marcel Pfeiffer [Opens in a new window]  and
M. Hossein Gorji Open the ORCID record for M. Hossein Gorji [Opens in a new window]
Mohsen Sadr*
Affiliation:
Applied and Computational Mathematics, RWTH Aachen University, Schinkestrasse 2, D-52062Aachen, Germany
Marcel Pfeiffer
Affiliation:
Institute of Space Systems, University of Stuttgart, Pfaffenwaldring 29, D-70569Stuttgart, Germany
M. Hossein Gorji
Affiliation:
Laboratory of Multiscale Studies in Building Physics, Empa, Swiss Federal Laboratories for Materials Science and Technology, Dübendorf, Switzerland
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

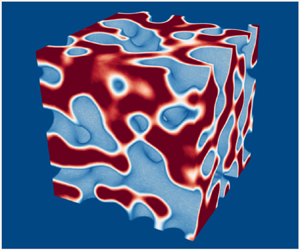
Multi-phase phenomena remain at the heart of many challenging fluid dynamics problems. Molecular fluxes at the interface determine the fate of neighbouring phases, yet their closure far from the continuum needs to be modelled. Along the hierarchy of kinetic approaches, a multi-phase particle method is devised in this study. This approach is built closely upon the previous studies on the kinetic method development for dense gases [Phys. Fluids, vol. 29 (12), 2017] and long-range interactions [J. Comput. Phys., vol. 378, 2019]. It is on this background that the current work on Fokker-Planck-Poisson modelling of multi-phase phenomena is initiated. Molecular interactions are expressed via stochastic forces driven by the white noise, coupled to the long-range attractions. The former is local and pursues diffusive approximation of molecular collisions, whereas the latter takes a global feature owing to mean-field forces. The obtained Fokker-Planck-Poisson combination provides an efficient work flow for physics-driven simulations suitable for multi-phase phenomena far from the equilibrium. Besides highlighting the computational efficiency of the method, various archetypical and complex problems ranging from inverted temperature gradients between droplets to spinodal decomposition are explored. Detailed discussions are provided on different characteristics of the droplets dispersed in low/high density background gases; including the departure of heat fluxes from Fourier's law as well as droplets growth in spinodal phases.

JFM classification

Rarefied Gas Flow: Kinetic theory Multiphase and Particle-laden Flows: Multiphase flow Micro-/Nano-fluid dynamics: Non-continuum effects
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 920 , 10 August 2021 , A46
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Alexander, F.J., Garcia, A.L. & Alder, B.J. 1995 A consistent Boltzmann algorithm. Phys. Rev. Lett. 74 (26), 5212–5215.CrossRefGoogle ScholarPubMed
Alharthy, N.S., Nguyen, T., Teklu, T., Kazemi, H. & Graves, R. 2013 Multiphase compositional modeling in small-scale pores of unconventional shale reservoirs. In SPE Annual Technical Conference and Exhibition. Society of Petroleum Engineers.CrossRefGoogle Scholar
Arnold, D.N., Brezzi, F., Cockburn, B. & Marini, L.D. 2002 Unified analysis of discontinuous galerkin methods for elliptic problems. SIAM J. Numer. Anal. 39 (5), 1749–1779.CrossRefGoogle Scholar
Bastea, S. & Lebowitz, J.L. 1997 Spinodal decomposition in binary gases. Phys. Rev. Lett. 78 (18), 3499–3502.CrossRefGoogle Scholar
Benson, C.M., Levin, D.A., Zhong, J., Gimelshein, S.F. & Montaser, A. 2004 Kinetic model for simulation of aerosol droplets in high-temperature environments. J. Thermophys. Heat Transfer 18 (1), 122–134.CrossRefGoogle Scholar
Bird, G.A. 1963 Approach to translational equilibrium in a rigid sphere gas. Phys. Fluids 6 (10), 1518–1519.CrossRefGoogle Scholar
Bird, G.A. 1994 Molecular Gas Dynamics and the Direct Simulation of Gas Flows. Clarendon Press.Google Scholar
Broadwell, J.E. 1964 Study of rarefied shear flow by the discrete velocity method. J. Fluid Mech. 19 (3), 401–414.CrossRefGoogle Scholar
Carnahan, N.F. & Starling, K.E. 1969 Equation of state for nonattracting rigid spheres. J. Chem. Phys. 51 (2), 635–636.CrossRefGoogle Scholar
Cercignani, C. 1988 a The Boltzmann equation. In The Boltzmann Equation and its Applications, pp. 40–103. Springer.CrossRefGoogle Scholar
Cercignani, C. 1988 b The Boltzmann Equation and Its Applications. Springer.CrossRefGoogle Scholar
Chapman, S. & Cowling, T.G. 1970 The Mathematical Theory of Non-Uniform Gases: An Account of the Kinetic Theory of Viscosity, Thermal Conduction and Diffusion in Gases. Cambridge University Press.Google Scholar
Cockburn, B., Dong, B., Guzmán, J., Restelli, M. & Sacco, R. 2009 A hybridizable discontinuous galerkin method for steady-state convection-diffusion-reaction problems. SIAM J. Sci. Comput. 31 (5), 3827–3846.CrossRefGoogle Scholar
Elsner, A. 1989 Interaction potential of a saturated fluid. Phys. Lett. A 138 (4–5), 168–172.CrossRefGoogle Scholar
Elsner, A. 1991 Calculation of the surface tension according to van der Waals. Phys. Lett. A 156 (3–4), 147–154.CrossRefGoogle Scholar
Enskog, D. 1922 Kinetische Theorie der Wärmeleitung: Reibung und Selbst-diffusion in Gewissen verdichteten gasen und flüssigkeiten. Almqvist & Wiksells boktryckeri-a.-b.Google Scholar
Espanol, P. & Revenga, M. 2003 Smoothed dissipative particle dynamics. Phys. Rev. E 67 (2), 026705.CrossRefGoogle ScholarPubMed
Fasoulas, S., Munz, C.-D., Pfeiffer, M., Beyer, J., Binder, T., Copplestone, S., Mirza, A., Nizenkov, P., Ortwein, P. & Reschke, W. 2019 Combining particle-in-cell and direct simulation monte carlo for the simulation of reactive plasma flows. Phys. Fluids 31 (7), 072006.CrossRefGoogle Scholar
Frezzotti, A. 1997 a Molecular dynamics and Enskog theory calculation of one dimensional problems in the dynamics of dense gases. Phys. A Stat. Mech. Appl. 240 (1–2), 202–211.CrossRefGoogle Scholar
Frezzotti, A. 1997 b A particle scheme for the numerical solution of the Enskog equation. Phys. Fluids 9 (5), 1329–1335.CrossRefGoogle Scholar
Frezzotti, A., Barbante, P. & Gibelli, L. 2019 Direct simulation Monte Carlo applications to liquid-vapor flows. Phys. Fluids 31 (6), 062103.CrossRefGoogle Scholar
Frezzotti, A., Gibelli, L., Lockerby, D.A. & Sprittles, J.E. 2018 Mean-field kinetic theory approach to evaporation of a binary liquid into vacuum. Phys. Rev. Fluids 3 (5), 054001.CrossRefGoogle Scholar
Frezzotti, A., Gibelli, L. & Lorenzani, S. 2005 Mean field kinetic theory description of evaporation of a fluid into vacuum. Phys. Fluids 17 (1), 012102.CrossRefGoogle Scholar
Frezzotti, A., Grosfils, P. & Toxvaerd, S. 2003 Evidence of an inverted temperature gradient during evaporation/condensation of a Lennard-Jones fluid. Phys. Fluids 15 (10), 2837–2842.CrossRefGoogle Scholar
Frezzotti, A.L.D.O., Nedea, S.V., Markvoort, A.J., Spijker, P. & Gibelli, L.I.V.I.O 2008 Comparison of molecular dynamics and kinetic modeling of gas-surface interaction. In AIP Conference Proceedings, vol. 1084, pp. 635–640. American Institute of Physics.CrossRefGoogle Scholar
Gamba, I.M. & Tharkabhushanam, S.H. 2009 Spectral-lagrangian methods for collisional models of non-equilibrium statistical states. J. Comput. Phys. 228 (6), 2012–2036.CrossRefGoogle Scholar
Ghoufi, A. & Malfreyt, P. 2010 Calculation of the surface tension from multibody dissipative particle dynamics and Monte Carlo methods. Phys. Rev. E 82 (1), 016706.CrossRefGoogle ScholarPubMed
Gorji, M.H. 2014 Fokker-Planck solution algorithm for rarefied gas flows and applications of complex gas-surface interactions. PhD thesis, Swiss Federal Institute of Technology in Zurich (ETHZ).CrossRefGoogle Scholar
Gorji, M.H. & Jenny, P. 2013 A Fokker–Planck based kinetic model for diatomic rarefied gas flows. Phys. Fluids 25 (6), 062002.CrossRefGoogle Scholar
Gorji, M.H. & Jenny, P. 2014 An efficient particle Fokker–Planck algorithm for rarefied gas flows. J. Comput. Phys. 262, 325–343.CrossRefGoogle Scholar
Gorji, M.H., Torrilhon, M. & Jenny, P. 2011 Fokker–Planck model for computational studies of monatomic rarefied gas flows. J. Fluid Mech. 680, 574–601.CrossRefGoogle Scholar
Grmela, M. 1971 Kinetic equation approach to phase transitions. J. Stat. Phys. 3 (3), 347–364.CrossRefGoogle Scholar
Hadjiconstantinou, N.G., Garcia, A.L. & Alder, B.J. 2000 The surface properties of a Van der Waals fluid. Phys. A Stat. Mech. Appl. 281 (1–4), 337–347.CrossRefGoogle Scholar
He, X. & Doolen, G.D. 2002 Thermodynamic foundations of kinetic theory and lattice Boltzmann models for multiphase flows. J. Stat. Phys. 107 (1–2), 309–328.CrossRefGoogle Scholar
Heylmun, J.C., Kong, B., Passalacqua, A. & Fox, R.O. 2019 A quadrature-based moment method for polydisperse bubbly flows. Comput. Phys. Commun. 244, 187–204.CrossRefGoogle Scholar
Hirschfelder, J., Bird, R.B. & Curtiss, C.F. 1964 Molecular Theory of Gases and Liquids. Wiley.Google Scholar
Hudgins, D., Gambino, N., Rollinger, B. & Abhari, R. 2016 Neutral cluster debris dynamics in droplet-based laser-produced plasma sources. J. Phys. D Appl. Phys. 49 (18), 185205.CrossRefGoogle Scholar
Jenny, P. & Gorji, M.H. 2019 Accurate particle time integration for solving Vlasov-Fokker-Planck equations with specified electromagnetic fields. J. Comput. Phys. 387, 430–445.CrossRefGoogle Scholar
Jenny, P., Torrilhon, M. & Heinz, S. 2010 A solution algorithm for the fluid dynamic equations based on a stochastic model for molecular motion. J. Comput. Phys. 229 (4), 1077–1098.CrossRefGoogle Scholar
Jun, E., Pfeiffer, M., Mieussens, L. & Gorji, M.H. 2019 Comparative study between cubic and ellipsoidal Fokker–Planck kinetic models. AIAA J. 57 (6), 2524–2533.CrossRefGoogle Scholar
Karkheck, J. & Stell, G. 1981 Kinetic mean-field theories. J. Chem. Phys. 75 (3), 1475–1487.CrossRefGoogle Scholar
Kobayashi, K., Hori, K., Kon, M., Sasaki, K. & Watanabe, M. 2016 Molecular dynamics study on evaporation and reflection of monatomic molecules to construct kinetic boundary condition in vapor–liquid equilibria. Heat Mass Transfer 52 (9), 1851–1859.CrossRefGoogle Scholar
Kon, M., Kobayashi, K. & Watanabe, M. 2014 Method of determining kinetic boundary conditions in net evaporation/condensation. Phys. Fluids 26 (7), 072003.CrossRefGoogle Scholar
Korteweg, D.J. 1901 Sur la forme que prennent les équations du mouvements des fluides si l'on tient compte des forces capillaires causées par des variations de densité considérables mais connues et sur la théorie de la capillarité dans l'hypothèse d'une variation continue de la densité. Arch. Néerland. Sci. Exact. Naturel. 6, 1–24.Google Scholar
Kremer, G.M. & Rosa, E. Jr. 1988 On Enskog's dense gas theory. I. The method of moments for monatomic gases. J. Chem. Phys. 89 (5), 3240–3247.CrossRefGoogle Scholar
Landau, L.D. & Lifshitz, E.M. 1959 Fluid Mechanics. Pergamon Press.Google Scholar
Li, Q., Luo, K.H. & Li, X.J. 2013 Lattice Boltzmann modeling of multiphase flows at large density ratio with an improved pseudopotential model. Phys. Rev. E 87 (5), 053301.CrossRefGoogle ScholarPubMed
Liu, M., Meakin, P. & Huang, H. 2007 Dissipative particle dynamics simulation of multiphase fluid flow in microchannels and microchannel networks. Phys. Fluids 19 (3), 033302.CrossRefGoogle Scholar
Meland, R. 2003 Molecular dynamics simulation of the inverted temperature gradient phenomenon. Phys. Fluids 15 (10), 3244–3247.CrossRefGoogle Scholar
Meland, R. & Ytrehus, T. 2004 Dependence of the inverted temperature gradient phenomenon on the condensation coefficient. Phys. Fluids 16 (3), 836–838.CrossRefGoogle Scholar
Montanero, J.M. & Santos, A. 1997 a Simulation of the Enskog equation a la bird. Phys. Fluids 9 (7), 2057–2060.CrossRefGoogle Scholar
Montanero, J.M. & Santos, A. 1997 b Viscometric effects in a dense hard-sphere fluid. Phys. A Stat. Mech. Appl. 240 (1–2), 229–238.CrossRefGoogle Scholar
Oksendal, B. 2013 Stochastic Differential Equations: An Introduction with Applications. Springer Science & Business Media.Google Scholar
Pao, Y.-P. 1971 Application of kinetic theory to the problem of evaporation and condensation. Phys. Fluids 14 (2), 306–312.CrossRefGoogle Scholar
Pfeiffer, M. & Gorji, M.H. 2017 Adaptive particle–cell algorithm for Fokker–Planck based rarefied gas flow simulations. Comput. Phys. Commun. 213, 1–8.CrossRefGoogle Scholar
Pfeiffer, M., Hindenlang, F., Binder, T., Copplestone, S.M., Munz, C.-D. & Fasoulas, S. 2019 A particle-in-cell solver based on a high-order hybridizable discontinuous galerkin spectral element method on unstructured curved meshes. Comput. Meth. Appl. Mech. Engng 349, 149–166.CrossRefGoogle Scholar
Piechór, K. 1994 Discrete velocity models of the Enskog-Vlasov equation. Transp. Theory Stat. Phys. 23 (1–3), 39–74.CrossRefGoogle Scholar
Resibois, P. 1978 H-theorem for the (modified) nonlinear Enskog equation. J. Stat. Phys. 19 (6), 593–609.CrossRefGoogle Scholar
Risken, H. 1989 The Fokker-Planck Equation: Methods of Solution and Applications. Springer.CrossRefGoogle Scholar
Rowley, L.A., Nicholson, D. & Parsonage, N.G. 1975 Monte Carlo grand canonical ensemble calculation in a gas-liquid transition region for 12-6 Argon. J. Comput. Phys. 17 (4), 401–414.CrossRefGoogle Scholar
Sadr, M. & Gorji, M.H. 2017 A continuous stochastic model for non-equilibrium dense gases. Phys. Fluids 29 (12), 122007.CrossRefGoogle Scholar
Sadr, M. & Gorji, M.H. 2019 Treatment of long-range interactions arising in the Enskog–Vlasov description of dense fluids. J. Comput. Phys. 378, 129–142.CrossRefGoogle Scholar
Schwarzkopf, J.D., Sommerfeld, M., Crowe, C.T. & Tsuji, Y. 2011 Multiphase Flows with Droplets and Particles. CRC Press.Google Scholar
Shan, X. & Chen, H. 1993 Lattice Boltzmann model for simulating flows with multiple phases and components. Phys. Rev. E 47 (3), 1815.CrossRefGoogle ScholarPubMed
Siggia, E.D. 1979 Late stages of spinodal decomposition in binary mixtures. Phys. Rev. A 20 (2), 595–605.CrossRefGoogle Scholar
Struchtrup, H. & Frezzotti, A. 2019 Grad's 13 moments approximation for Enskog-Vlasov equation. In AIP Conference Proceedings, vol. 2132, p. 120007. AIP Publishing LLC.CrossRefGoogle Scholar
Torrilhon, M. 2016 Modeling nonequilibrium gas flow based on moment equations. Annu. Rev. Fluid Mech. 48, 429–458.CrossRefGoogle Scholar
Van Beijeren, H. & Ernst, M.H. 1973 The modified Enskog equation. Physica 68 (3), 437–456.CrossRefGoogle Scholar
Vlasov, A.A. 1978 Many-Particle Theory and its Application to Plasma. Gordon and Breach.Google Scholar
Wang, P., Wu, L., Ho, M.T., Li, J., Li, Z.-H. & Zhang, Y. 2020 The kinetic Shakhov–Enskog model for non-equilibrium flow of dense gases. J. Fluid Mech. 883, A48.CrossRefGoogle Scholar
Watanabe, H., Ito, N. & Hu, C.-K. 2012 Phase diagram and universality of the Lennard-Jones gas-liquid system. J. Chem. Phys. 136 (20), 204102.CrossRefGoogle ScholarPubMed
Wörner, M. 2012 Numerical modeling of multiphase flows in microfluidics and micro process engineering: a review of methods and applications. Microfluid Nanofluid 12 (6), 841–886.CrossRefGoogle Scholar
Wu, L., Liu, H., Reese, J.M. & Zhang, Y. 2016 Non-equilibrium dynamics of dense gas under tight confinement. J. Fluid Mech. 794, 252–266.CrossRefGoogle Scholar
Wu, L., White, C., Scanlon, T.J., Reese, J.M. & Zhang, Y. 2013 Deterministic numerical solutions of the Boltzmann equation using the fast spectral method. J. Comput. Phys. 250, 27–52.CrossRefGoogle Scholar
Wu, L., Zhang, Y. & Reese, J.M. 2015 Fast spectral solution of the generalized Enskog equation for dense gases. J. Comput. Phys. 303, 66–79.CrossRefGoogle Scholar
Yasuoka, K., Matsumoto, M. & Kataoka, Y. 1994 Evaporation and condensation at a liquid surface. I. Argon. J. Chem. Phys. 101 (9), 7904–7911.CrossRefGoogle Scholar

Sadr et al. supplementary movie 1

See pdf file for movie caption

Download Sadr et al. supplementary movie 1(Video)
Video 880.7 KB

Sadr et al. supplementary movie 2

See pdf file for movie caption

Download Sadr et al. supplementary movie 2(Video)
Video 1.6 MB

Sadr et al. supplementary movie 3

See pdf file for movie caption

Download Sadr et al. supplementary movie 3(Video)
Video 1 MB

Sadr et al. supplementary movie 4

See pdf file for movie caption

Download Sadr et al. supplementary movie 4(Video)
Video 942.6 KB
Supplementary material: PDF

Sadr et al. supplementary material

Captions for movies 1-4

Download Sadr et al. supplementary material(PDF)
PDF 72.3 KB