Hostname: page-component-6587cd75c8-rlsgg Total loading time: 0 Render date: 2025-04-23T19:55:54.690Z Has data issue: false hasContentIssue false
  • English
  • Français

Flow patterns and energy spectra in forced quasi-two-dimensional turbulence: effect of system size and damping rate

Published online by Cambridge University Press:  02 October 2024

Hang-Yu Zhu Open the ORCID record for Hang-Yu Zhu [Opens in a new window] ,
Jin-Han Xie Open the ORCID record for Jin-Han Xie [Opens in a new window]  and
Ke-Qing Xia Open the ORCID record for Ke-Qing Xia [Opens in a new window]
Hang-Yu Zhu
Affiliation:
Centre for Complex Flows and Soft Matter Research, Southern University of Science and Technology, Shenzhen 518055, PR China Department of Mechanics and Aerospace Engineering, Southern University of Science and Technology, Shenzhen 518055 PR China Department of Mechanics, School of Aerospace Engineering, Huazhong University of Science and Technology, Wuhan 430074, PR China
Jin-Han Xie
Affiliation:
Department of Mechanics and Engineering Science at College of Engineering, and State Key Laboratory for Turbulence and Complex Systems, Peking University, Beijing 100871, PR China
Ke-Qing Xia*
Affiliation:
Centre for Complex Flows and Soft Matter Research, Southern University of Science and Technology, Shenzhen 518055, PR China Department of Mechanics and Aerospace Engineering, Southern University of Science and Technology, Shenzhen 518055 PR China Department of Physics, Southern University of Science and Technology, Shenzhen 518055, PR China
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

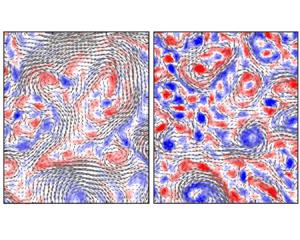
An experimental investigation is conducted to study the flow patterns, spectral properties and energy fluxes in thin-layer turbulence with varying system sizes and damping rates. It is found that although a system-size vortex (an indicator of spectral condensation) occurs for small system sizes and does not for large ones, the spectra for different system sizes consistently exhibit a scaling close to $k^{-3}$ in inverse cascade (another indicator of spectral condensation). On the other hand, under a fixed system size larger than the friction-dominated length scale, the energy spectrum in the inverse cascade range changes from $k^{-3}$ to $k^{-5/3}$ as the damping rate increases, suggesting that the friction-dominated length scale may not be a suitable parameter for predicting spectral transition. At lower damping rates and large system sizes, turbulent structures grow larger via inverse cascade, manifesting as long streamers, and the small-scale vortices are suppressed. This suppression leads to a reduction of energy flux at intermediate scales and a change in the spectral shape. The dimensionless Taylor microscale is found to exhibit a monotonic dependence on the damping rate. With the reduction in the damping rate, the Taylor microscale increases to become comparable with the forcing scale, and the spectrum in inverse cascade transits to a steeper scaling, $k^{-3}$, indicating that the dimensionless Taylor microscale may be used as a diagnostic parameter for spectral transition.

JFM classification

Turbulent Flows: Turbulent Flows
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 996 , 10 October 2024 , A39
Check for updates
Copyright
© The Author(s), 2024. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Alexakis, A. & Biferale, L. 2018 Cascades and transitions in turbulent flows. Phys. Rep. 767, 1–101.10.1016/j.physrep.2018.08.001CrossRefGoogle Scholar
Alexakis, A., Mininni, P.D. & Pouquet, A. 2005 Imprint of large-scale flows on turbulence. Phys. Rev. Lett. 95 (26), 264503.10.1103/PhysRevLett.95.264503CrossRefGoogle ScholarPubMed
Balk, A.M., Zakharov, V.E. & Nazarenko, S.V. 1990 Nonlocal turbulence of drift waves. Sov. Phys. JETP 71 (2), 249–260.Google Scholar
Biglari, H., Diamond, P.H. & Terry, P.W. 1990 Influence of sheared poloidal rotation on edge turbulence. Phys. Fluids 2 (1), 1–4.10.1063/1.859529CrossRefGoogle Scholar
Boffetta, G., Cenedese, A., Espa, S. & Musacchio, S. 2005 Effects of friction on 2D turbulence: an experimental study of the direct cascade. Europhys. Lett. 71 (4), 590.10.1209/epl/i2005-10111-6CrossRefGoogle Scholar
Boffetta, G. & Ecke, R.E. 2012 Two-dimensional turbulence. Annu. Rev. Fluid Mech. 44, 427–451.10.1146/annurev-fluid-120710-101240CrossRefGoogle Scholar
Boffetta, G. & Musacchio, S. 2010 Evidence for the double cascade scenario in two-dimensional turbulence. Phys. Rev. E 82 (1), 016307.CrossRefGoogle ScholarPubMed
Burgess, B.H., Dritschel, D.G. & Scott, R.K. 2017 Vortex scaling ranges in two-dimensional turbulence. Phys. Fluids 29 (11), 111104.10.1063/1.4993144CrossRefGoogle Scholar
Burgess, B.H. & Scott, R.K. 2017 Scaling theory for vortices in the two-dimensional inverse energy cascade. J. Fluid Mech. 811, 742–756.10.1017/jfm.2016.756CrossRefGoogle Scholar
Cerbus, R.T. & Goldburg, W.I. 2013 Intermittency in 2D soap film turbulence. Phys. Fluids 25 (10), 105111.10.1063/1.4824658CrossRefGoogle Scholar
Chen, S.-Y., Ecke, R.E., Eyink, G.L., Rivera, M., Wan, M.-P. & Xiao, Z.-L. 2006 Physical mechanism of the two-dimensional inverse energy cascade. Phys. Rev. Lett. 96 (8), 084502.10.1103/PhysRevLett.96.084502CrossRefGoogle ScholarPubMed
Chertkov, M., Connaughton, C., Kolokolov, I. & Lebedev, V. 2007 Dynamics of energy condensation in two-dimensional turbulence. Phys. Rev. Lett. 99 (8), 084501.10.1103/PhysRevLett.99.084501CrossRefGoogle ScholarPubMed
Clercx, H.J.H., Van Heijst, G.J.F. & Zoeteweij, M.L. 2003 Quasi-two-dimensional turbulence in shallow fluid layers: the role of bottom friction and fluid layer depth. Phys. Rev. E 67 (6), 066303.10.1103/PhysRevE.67.066303CrossRefGoogle ScholarPubMed
Danilov, S.D. & Gurarie, D. 2000 Quasi-two-dimensional turbulence. Phys.-Uspekhi 43 (9), 863–900.CrossRefGoogle Scholar
De Wit, X.M., van Kan, A. & Alexakis, A. 2022 Bistability of the large-scale dynamics in quasi-two-dimensional turbulence. J. Fluid Mech. 939, R2.10.1017/jfm.2022.209CrossRefGoogle Scholar
Doludenko, A.N., Fortova, S.V., Kolokolov, I.V. & Lebedev, V.V. 2022 Coherent vortex versus chaotic state in two-dimensional turbulence. Ann. Phys. 447, 169072.10.1016/j.aop.2022.169072CrossRefGoogle Scholar
Falkovich, G. 2016 Interaction between mean flow and turbulence in two dimensions. Proc. Math. Phys. Engng 472 (2191), 20160287.Google ScholarPubMed
Fang, L. & Ouellette, N.T. 2017 Multiple stages of decay in two-dimensional turbulence. Phys. Fluids 29 (11), 111105.10.1063/1.4996776CrossRefGoogle Scholar
Fang, L. & Ouellette, N.T. 2021 Spectral condensation in laboratory two-dimensional turbulence. Phys. Rev. Fluids 6 (10), 104605.CrossRefGoogle Scholar
Fontane, J., Dritschel, D.G. & Scott, R.K. 2013 Vortical control of forced two-dimensional turbulence. Phys. Fluids 25 (1), 015101.CrossRefGoogle Scholar
Francois, N., Xia, H., Punzmann, H. & Shats, M. 2018 Rectification of chaotic fluid motion in two-dimensional turbulence. Phys. Rev. Fluids 3 (12), 124602.10.1103/PhysRevFluids.3.124602CrossRefGoogle Scholar
Frisch, U. 1995 Turbulence: The Legacy of A.N. Kolmogorov. Cambridge University Press.CrossRefGoogle Scholar
Frishman, A. 2017 The culmination of an inverse cascade: mean flow and fluctuations. Phys. Fluids 29 (12), 125102.CrossRefGoogle Scholar
Frishman, A., Laurie, J. & Falkovich, G. 2017 Jets or vortices – what flows are generated by an inverse turbulent cascade? Phys. Rev. Fluids 2 (3), 032602.CrossRefGoogle Scholar
Goldburg, W.I., Cressman, J.R., Vörös, Z., Eckhardt, B. & Schumacher, J. 2001 Turbulence in a free surface. Phys. Rev. E 63 (6), 065303.10.1103/PhysRevE.63.065303CrossRefGoogle Scholar
Jiménez, J. 2020 Dipoles and streams in two-dimensional turbulence. J. Fluid Mech. 904, A39.10.1017/jfm.2020.769CrossRefGoogle Scholar
van Kan, A. & Alexakis, A. 2019 Condensates in thin-layer turbulence. J. Fluid Mech. 864, 490–518.CrossRefGoogle Scholar
Kolokolov, I.V. & Lebedev, V.V. 2020 Coherent vortex in two-dimensional turbulence: interplay of viscosity and bottom friction. Phys. Rev. E 102 (2), 023108.10.1103/PhysRevE.102.023108CrossRefGoogle ScholarPubMed
Kraichnan, R.H. 1967 Inertial ranges in two-dimensional turbulence. Phys. Fluids 10 (7), 1417–1423.CrossRefGoogle Scholar
Kramer, W., Keetels, G.H., Clercx, H.J.H. & van Heijst, G.J.F. 2011 Structure-function scaling of bounded two-dimensional turbulence. Phys. Rev. E 84 (2), 026310.10.1103/PhysRevE.84.026310CrossRefGoogle ScholarPubMed
Lee, T.D. 1951 Difference between turbulence in a two-dimensional fluid and in a three-dimensional fluid. J. Appl. Phys. 22 (4), 524–524.10.1063/1.1699997CrossRefGoogle Scholar
Liao, Y. & Ouellette, N.T. 2013 Spatial structure of spectral transport in two-dimensional flow. J. Fluid Mech. 725, 281–298.10.1017/jfm.2013.187CrossRefGoogle Scholar
Lilly, D.K. 1972 Numerical simulation studies of two-dimensional turbulence: I. Models of statistically steady turbulence. Geophys. Fluid Dyn. 3 (4), 289–319.CrossRefGoogle Scholar
Lovecchio, S., Zonta, F. & Soldati, A. 2015 Upscale energy transfer and flow topology in free-surface turbulence. Phys. Rev. E 91 (3), 033010.CrossRefGoogle ScholarPubMed
McWilliams, J.C. 1984 The emergence of isolated coherent vortices in turbulent flow. J. Fluid Mech. 146, 21–43.CrossRefGoogle Scholar
McWilliams, J.C. 1990 A demonstration of the suppression of turbulent cascades by coherent vortices in two-dimensional turbulence. Phys. Fluids 2 (4), 547–552.10.1063/1.857755CrossRefGoogle Scholar
Nazarenko, S. & Laval, J.-P. 2000 Non-local two-dimensional turbulence and Batchelor's regime for passive scalars. J. Fluid Mech. 408, 301–321.10.1017/S0022112099007922CrossRefGoogle Scholar
Paret, J. & Tabeling, P. 1997 Experimental observation of the two-dimensional inverse energy cascade. Phys. Rev. Lett. 79 (21), 4162–4165.CrossRefGoogle Scholar
Paret, J. & Tabeling, P. 1998 Intermittency in the two-dimensional inverse cascade of energy: experimental observations. Phys. Fluids 10 (12), 3126–3136.10.1063/1.869840CrossRefGoogle Scholar
Rivera, M., Vorobieff, P. & Ecke, R.E. 1998 Turbulence in flowing soap films: velocity, vorticity, and thickness fields. Phys. Rev. Lett. 81 (7), 1417–1420.CrossRefGoogle Scholar
Rivera, M. & Wu, X.-L. 2002 Homogeneity and the inertial range in driven two-dimensional turbulence. Phys. Fluids 14 (9), 3098–3108.CrossRefGoogle Scholar
Rivera, M., Wu, X.-L. & Yeung, C. 2001 Universal distribution of centers and saddles in two-dimensional turbulence. Phys. Rev. Lett. 87 (4), 044501.CrossRefGoogle ScholarPubMed
Rivera, M.K., Daniel, W.B., Chen, S.-Y. & Ecke, R.E. 2003 Energy and enstrophy transfer in decaying two-dimensional turbulence. Phys. Rev. Lett. 90 (10), 104502.10.1103/PhysRevLett.90.104502CrossRefGoogle ScholarPubMed
Rivera, M.K. & Ecke, R.E. 2016 Lagrangian statistics in weakly forced two-dimensional turbulence. Chaos 26 (1), 013103.CrossRefGoogle ScholarPubMed
Scarano, F. & Riethmuller, M.L. 2000 Advances in iterative multigrid PIV image processing. Exp. Fluids 29 (Suppl 1), S051–S060.CrossRefGoogle Scholar
Schumacher, J. 2003 Probing surface flows with Lagrangian tracers. Prog. Theor. Phys. Suppl. 150, 255–266.CrossRefGoogle Scholar
Scott, R.K. 2007 Nonrobustness of the two-dimensional turbulent inverse cascade. Phys. Rev. E – Stat. Nonlinear Soft Matt. Phys. 75 (4), 046301.10.1103/PhysRevE.75.046301CrossRefGoogle ScholarPubMed
Shaing, K.-C., Crume, E.C. Jr. & Houlberg, W.A. 1990 Bifurcation of poloidal rotation and suppression of turbulent fluctuations: a model for the L–H transition in tokamaks. Phys. Fluids 2 (6), 1492–1498.10.1063/1.859473CrossRefGoogle Scholar
Shats, M.G., Xia, H. & Punzmann, H. 2005 Spectral condensation of turbulence in plasmas and fluids and its role in low-to-high phase transitions in toroidal plasma. Phys. Rev. E 71 (4), 046409.10.1103/PhysRevE.71.046409CrossRefGoogle ScholarPubMed
Shats, M.G., Xia, H., Punzmann, H. & Falkovich, G. 2007 Suppression of turbulence by self-generated and imposed mean flows. Phys. Rev. Lett. 99 (16), 164502.CrossRefGoogle ScholarPubMed
Sommeria, J. 1986 Experimental study of the two-dimensional inverse energy cascade in a square box. J. Fluid Mech. 170, 139–168.CrossRefGoogle Scholar
Suri, B., Tithof, J., Mitchell, R., Grigoriev, R.O. & Schatz, M.F. 2014 Velocity profile in a two-layer Kolmogorov-like flow. Phys. Fluids 26 (5), 053601.10.1063/1.4873417CrossRefGoogle Scholar
Tithof, J., Martell, B.C. & Kelley, D.H. 2018 Three-dimensionality of one-and two-layer electromagnetically driven thin-layer flows. Phys. Rev. Fluids 3 (6), 064602.10.1103/PhysRevFluids.3.064602CrossRefGoogle Scholar
Tran, C.V. & Bowman, J.C. 2004 Robustness of the inverse cascade in two-dimensional turbulence. Phys. Rev. E 69 (3), 036303.10.1103/PhysRevE.69.036303CrossRefGoogle ScholarPubMed
Valente, P.C. & Vassilicos, J.C. 2015 The energy cascade in grid-generated non-equilibrium decaying turbulence. Phys. Fluids 27 (4), 045103.10.1063/1.4916628CrossRefGoogle Scholar
Xia, H., Byrne, D., Falkovich, G. & Shats, M.G. 2011 Upscale energy transfer in thick turbulent fluid layers. Nat. Phys. 7 (4), 321–324.CrossRefGoogle Scholar
Xia, H., Francois, N., Punzmann, H., Byrne, D. & Shats, M.G. 2016 Simultaneous observation of energy and enstrophy cascades in thin-layer turbulence. In International Journal of Modern Physics: Conference Series, vol. 42, 1660185. World Scientific.10.1142/S201019451660185XCrossRefGoogle Scholar
Xia, H., Punzmann, H., Falkovich, G. & Shats, M.G. 2008 Turbulence–condensate interaction in two dimensions. Phys. Rev. Lett. 101 (19), 194504.CrossRefGoogle ScholarPubMed
Xia, H. & Shats, M. 2012 Structure formation in spectrally condensed turbulence. In International Journal of Modern Physics: Conference Series, vol. 19, pp. 257–261. World Scientific.CrossRefGoogle Scholar
Xia, H., Shats, M.G. & Falkovich, G. 2009 Spectrally condensed turbulence in thin layers. Phys. Fluids 21 (12), 125101.CrossRefGoogle Scholar
Xiao, Z.-L., Wan, M.-P., Chen, S.-Y. & Eyink, G.L. 2009 Physical mechanism of the inverse energy cascade of two-dimensional turbulence: a numerical investigation. J. Fluid Mech. 619, 1–44.CrossRefGoogle Scholar
Yang, J. 2021 Finite-size anisotropic particles in two-dimensional turbulent flows. PhD thesis, The Australian National University (Australia).Google Scholar
Yang, J., Davoodianidalik, M., Xia, H., Punzmann, H., Shats, M. & Francois, N. 2019 Passive propulsion in turbulent flows. Phys. Rev. Fluids 4 (10), 104608.10.1103/PhysRevFluids.4.104608CrossRefGoogle Scholar
Zhou, Z.-Y., Fang, L., Ouellette, N.T. & Xu, H.-T. 2020 Vorticity gradient stretching in the direct enstrophy transfer process of two-dimensional turbulence. Phys. Rev. Fluids 5 (5), 054602.CrossRefGoogle Scholar
Zhu, H.-Y., Xie, J.-H. & Xia, K.-Q. 2023 Circulation in quasi-2D turbulence: experimental observation of the area rule and bifractality. Phys. Rev. Lett. 130 (21), 214001.10.1103/PhysRevLett.130.214001CrossRefGoogle ScholarPubMed