Hostname: page-component-586b7cd67f-2brh9 Total loading time: 0 Render date: 2024-11-24T15:55:08.676Z Has data issue: false hasContentIssue false
  • English
  • Français

Flow around an inclined circular disk

Published online by Cambridge University Press:  31 July 2018

Song Gao ,
Longbin Tao ,
Xinliang Tian Open the ORCID record for Xinliang Tian [Opens in a new window]  and
Jianmin Yang
Song Gao
Affiliation:
State Key Laboratory of Ocean Engineering, Shanghai Jiao Tong University, Shanghai 200240, PR China Collaborative Innovation Center for Advanced Ship and Deep-Sea Exploration, Shanghai 200240, PR China
Longbin Tao
Affiliation:
Department of Naval Architecture, Ocean and Marine Engineering, University of Strathclyde, Glasgow G4 0LZ, UK
Xinliang Tian*
Affiliation:
State Key Laboratory of Ocean Engineering, Shanghai Jiao Tong University, Shanghai 200240, PR China Collaborative Innovation Center for Advanced Ship and Deep-Sea Exploration, Shanghai 200240, PR China
Jianmin Yang
Affiliation:
State Key Laboratory of Ocean Engineering, Shanghai Jiao Tong University, Shanghai 200240, PR China Collaborative Innovation Center for Advanced Ship and Deep-Sea Exploration, Shanghai 200240, PR China
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Direct numerical simulations are performed for the uniform flow around an inclined circular disk. The diameter–thickness aspect ratio (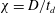$\unicode[STIX]{x1D712}=D/t_{d}$) of the disk is 50 and the inclination angle ($\unicode[STIX]{x1D6FC}$) is considered over the range of $0^{\circ }\leqslant \unicode[STIX]{x1D6FC}\leqslant 80^{\circ }$, where 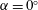$\unicode[STIX]{x1D6FC}=0^{\circ }$ refers to the condition where the flow is normal to the disk. The Reynolds number (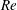$\mathit{Re}$), based on the short axis of projection in the streamwise direction, is defined as 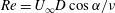$\mathit{Re}=U_{\infty }D\cos \unicode[STIX]{x1D6FC}/\unicode[STIX]{x1D708}$, where $U_{\infty }$ is the velocity of the flow and $\unicode[STIX]{x1D708}$ is the kinematic viscosity. 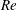$\mathit{Re}$ is investigated over the range of 50 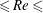${\leqslant}\mathit{Re}\leqslant$ 300. In the considered 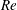$\mathit{Re}$–$\unicode[STIX]{x1D6FC}$ parametric space, five states are observed and denoted as: (I) steady state (SS); (II) periodic state (PS); (III) periodic state with a low frequency modulation (PSL); (IV) quasi-periodic state (QP) and (V) chaotic state (CS). Both 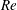$\mathit{Re}$ and $\unicode[STIX]{x1D6FC}$ affect the bifurcation mechanism. The bifurcating sequence occurring at $\unicode[STIX]{x1D6FC}=0^{\circ }$ is generally observed over the whole 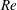$\mathit{Re}$–$\unicode[STIX]{x1D6FC}$ space, although it is advanced at small $\unicode[STIX]{x1D6FC}$ and delayed at large $\unicode[STIX]{x1D6FC}$. The advancement of thresholds for different states is due to the effects introduced by inclination, which tend to select the plane of symmetry for the wake in order to regulate the wake and intensify some flow features. Nevertheless, the bifurcations are still in the dominant position when leading a state without stable symmetry, i.e. the planar symmetry could not be recovered by small $\unicode[STIX]{x1D6FC}$. These phenomena are further discussed with respect to the vortex shedding patterns behind the disk. Furthermore, for any fixed disk, the wake behaviour is only associated with that found in the steady vertical state of a freely falling disk. The fully coupled fluid–body system is fundamentally different from the fixed cases.

JFM classification

Nonlinear Dynamical Systems: Bifurcation Vortex Flows: Vortex instability Wakes/Jets: Wakes
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 851 , 25 September 2018 , pp. 687 - 714
Check for updates
Copyright
© 2018 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

‡

These authors contributed equally to this work.

References

Auguste, F., Fabre, D. & Magnaudet, J. 2010 Bifurcations in the wake of a thick circular disk. Theor. Comput. Fluid Dyn. 24 (1), 305–313.Google Scholar
Auguste, F., Magnaudet, J. & Fabre, D. 2013 Falling styles of disks. J. Fluid Mech. 719, 388–405.Google Scholar
Berger, E., Scholz, D. & Schumm, M. 1990 Coherent vortex structures in the wake of a sphere and a circular disk at rest and under forced vibrations. J. Fluids Struct. 4 (3), 231–257.Google Scholar
Calvert, J. R. 1967 Experiments on the flow past an inclined disk. J. Fluid Mech. 29 (04), 691–703.Google Scholar
Chrust, M., Bouchet, G. & Dušek, J. 2010 Parametric study of the transition in the wake of oblate spheroids and flat cylinders. J. Fluid Mech. 665, 199–208.Google Scholar
Chrust, M., Bouchet, G. & Dušek, J. 2013 Numerical simulation of the dynamics of freely falling discs. Phys. Fluids 25 (4), 044102.Google Scholar
Chrust, M., Dauteuille, C., Bobinski, T., Rokicki, J., Goujon-Durand, S., Wesfreid, J. E., Bouchet, G. & Dušek, J. 2015 Effect of inclination on the transition scenario in the wake of fixed disks and flat cylinders. J. Fluid Mech. 770, 189–209.Google Scholar
Dickinson, M. H. & Gotz, K. G. 1993 Unsteady aerodynamic performance of model wings at low reynolds numbers. J. Expl Biol. 174 (1), 45–64.Google Scholar
Dong, H., Mittal, R. & Najjar, F. M. 2006 Wake topology and hydrodynamic performance of low-aspect-ratio flapping foils. J. Fluid Mech. 566, 309–343.Google Scholar
Ern, P., Risso, F., Fabre, D. & Magnaudet, J. 2012 Wake-induced oscillatory paths of bodies freely rising or falling in fluids. Annu. Rev. Fluid Mech. 44, 97–121.Google Scholar
Fabre, D., Auguste, F. & Magnaudet, J. 2008 Bifurcations and symmetry breaking in the wake of axisymmetric bodies. Phys. Fluids 20, 051702.Google Scholar
Fernandes, P. C., Risso, F., Ern, P. & Magnaudet, J. 2007 Oscillatory motion and wake instability of freely rising axisymmetric bodies. J. Fluid Mech. 573, 479–502.Google Scholar
Field, S. B., Klaus, M., Moore, M. G. & Nori, F. 1997 Chaotic dynamics of falling disks. Nature 388 (6639), 252–254.Google Scholar
Horowitz, M. & Williamson, C. H. K. 2010 The effect of Reynolds number on the dynamics and wakes of freely rising and falling spheres. J. Fluid Mech. 651, 251–294.Google Scholar
Jenny, M., Dušek, J. & Bouchet, G. 2004 Instabilities and transition of a sphere falling or ascending freely in a Newtonian fluid. J. Fluid Mech. 508, 201–239.Google Scholar
Johnson, T. A. & Patel, V. C. 1999 Flow past a sphere up to a Reynolds number of 300. J. Fluid Mech. 378, 19–70.Google Scholar
Kuo, Y. H. & Baldwin, L. V. 1967 The formation of elliptical wakes. J. Fluid Mech. 27 (02), 353–360.Google Scholar
Marshall, D. & Stanton, T. E. 1931 On the eddy system in the wake of flat circular plates in three dimensional flow. Proc. R. Soc. Lond. A 130 (813), 295–301.Google Scholar
Meliga, P., Chomaz, J. M. & Sipp, D. 2009 Global mode interaction and pattern selection in the wake of a disk: a weakly nonlinear expansion. J. Fluid Mech. 633, 159–189.Google Scholar
Michael, P. 1966 Steady motion of a disk in a viscous fluid. Phys. Fluids 9, 466–471.Google Scholar
Natarajan, R. & Acrivos, A. 1993 The instability of the steady flow past spheres and disks. J. Fluid Mech. 254, 323–344.Google Scholar
OpenFOAM 2009 The Open Source CFD Toolbox, Programmer’s Guide, Version 1.6. OpenCFD Limited.Google Scholar
Rimon, Y. 1969 Numerical solution of the incompressible time-dependent viscous flow past a thin oblate spheroid. Phys. Fluids 12, II–65–75.Google Scholar
Rivet, J. P., Henon, M., Frisch, U. & D’Humieres, D. 1988 Simulating fully three-dimensional external flow by lattice gas methods. Europhys. Lett. 7, 231.Google Scholar
Roberts, J. B. 1973 Coherence measurements in an axisymmetric wake. AIAA J. 11, 1569–1571.Google Scholar
Roos, F. W. & Willmarth, W. W. 1971 Some experimental results on sphere and disk drag. AIAA J. 9, 285–291.Google Scholar
Shenoy, A. R. & Kleinstreuer, C. 2008 Flow over a thin circular disk at low to moderate Reynolds numbers. J. Fluid Mech. 605, 253–262.Google Scholar
Shenoy, A. R. & Kleinstreuer, C. 2010 Influence of aspect ratio on the dynamics of a freely moving circular disk. J. Fluid Mech. 653, 463–487.Google Scholar
Taira, K. & Colonius, T. 2009 Three-dimensional flows around low-aspect-ratio flat-plate wings at low Reynolds numbers. J. Fluid Mech. 623, 187–207.Google Scholar
Tian, X., Hu, Z., Lu, H. & Yang, J. 2017a Direct numerical simulations on the flow past an inclined circular disk. J. Fluids Struct. 72, 152–168.Google Scholar
Tian, X., Xiao, L., Zhang, X., Yang, J., Tao, L. & Yang, D. 2017b Flow around an oscillating circular disk at low to moderate Reynolds numbers. J. Fluid Mech. 812, 1119–1145.Google Scholar
Tomboulides, A. G. & Orszag, S. A. 2000 Numerical investigation of transitional and weak turbulent flow past a sphere. J. Fluid Mech. 416, 45–73.Google Scholar
Weller, H. G., Tabor, G., Jasak, H. & Fureby, C. 1998 A tensorial approach to computational continuum mechanics using object-oriented techniques. Comput. Phys. 12, 620–631.Google Scholar
Willmarth, W. W., Hawk, N. E. & Harvey, R. L. 1964 Steady and unsteady motions and wakes of freely falling disks. Phys. Fluids 7 (2), 197–208.Google Scholar
Yang, J., Liu, M., Wu, G., Liu, Q. & Zhang, X. 2015 Low-frequency characteristics in the wake of a circular disk. Phys. Fluids 27 (6), 064101.Google Scholar
Yang, J., Liu, M., Wu, G., Zhong, W. & Zhang, X. 2014a Numerical study on coherent structure behind a circular disk. J. Fluids Struct. 51, 172–188.Google Scholar
Yang, J., Tian, X. & Li, X. 2014b Hydrodynamic characteristics of an oscillating circular disk under steady in-plane current conditions. Ocean Engng 75, 53–63.Google Scholar
Zhong, H. J., Chen, S. Y. & Lee, C. B. 2011 Experimental study of freely falling thin disks: transition from planar zigzag to spiral. Phys. Fluids 23 (1), 011702.Google Scholar
Zhong, H. J. & Lee, C. B. 2012 The wake of falling disks at low Reynolds numbers. Acta Mechanica Sin. 28, 1–5.Google Scholar
Zhou, W., Chrust, M. & Dušek, J. 2017 Path instabilities of oblate spheroids. J. Fluid Mech. 833, 445–468.Google Scholar

Gao et al. supplementary movie 1

Periodic state: Re = 150, α = 20°

Download Gao et al. supplementary movie 1(Video)
Video 6 MB

Gao et al. supplementary movie 2

Periodic state: Re = 250, α = 60°

Download Gao et al. supplementary movie 2(Video)
Video 3.9 MB

Gao et al. supplementary movie 3

Periodic state with a low-frequency modulation: Re = 250, α = 50°

Download Gao et al. supplementary movie 3(Video)
Video 8.9 MB

Gao et al. supplementary movie 4

Quasi-periodic state: Re = 200, α = 15°

Download Gao et al. supplementary movie 4(Video)
Video 7.3 MB