Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Samanta, Arghya
2013.
Shear wave instability for electrified falling films.
Physical Review E,
Vol. 88,
Issue. 5,
Praveen Kumar, A. Ananth
Usha, R.
Banerjee, Tamal
and
Bandyopadhyay, Dipankar
2013.
Instabilities of a free bilayer flowing on an inclined porous medium.
Physical Review E,
Vol. 88,
Issue. 6,
Goyal, Himanshu
Ananth Praveen Kumar, A.
Bandyopadhyay, Dipankar
Usha, R.
and
Banerjee, Tamal
2013.
Instabilities of a confined two-layer flow on a porous medium: An Orr–Sommerfeld analysis.
Chemical Engineering Science,
Vol. 97,
Issue. ,
p.
109.
Anjalaiah
Usha, R.
and
Millet, S.
2013.
Thin film flow down a porous substrate in the presence of an insoluble surfactant: Stability analysis.
Physics of Fluids,
Vol. 25,
Issue. 2,
Dietze, Georg F.
and
Ruyer-Quil, Christian
2013.
Wavy liquid films in interaction with a confined laminar gas flow.
Journal of Fluid Mechanics,
Vol. 722,
Issue. ,
p.
348.
Samanta, Arghya
2013.
Effect of surfactant on two-layer channel flow.
Journal of Fluid Mechanics,
Vol. 735,
Issue. ,
p.
519.
Samanta, A.
Goyeau, B.
and
Ruyer-Quil, C.
2013.
A falling film on a porous medium.
Journal of Fluid Mechanics,
Vol. 716,
Issue. ,
p.
414.
Ding, Zijing
Wong, Teck Neng
Liu, Rong
and
Liu, Qiusheng
2013.
Viscous liquid films on a porous vertical cylinder: Dynamics and stability.
Physics of Fluids,
Vol. 25,
Issue. 6,
Kandel, H. N.
and
Pascal, J. P.
2013.
Inclined fluid-film flow with bottom filtration.
Physical Review E,
Vol. 88,
Issue. 5,
Chesnokov, A. A.
and
Kovtunenko, P. V.
2014.
Weak Discontinuities in Solutions of Long‐Wave Equations for Viscous Flow.
Studies in Applied Mathematics,
Vol. 132,
Issue. 1,
p.
50.
Wu, Zheng Ren
Fu, Guan
and
Song, Zhao Xia
2014.
Surface Wave on a Viscous Fluid Film down an Inclined Uneven Wall.
Applied Mechanics and Materials,
Vol. 684,
Issue. ,
p.
154.
Samanta, Arghya
2014.
Shear-imposed falling film.
Journal of Fluid Mechanics,
Vol. 753,
Issue. ,
p.
131.
Chakraborty, S.
Nguyen, P.-K.
Ruyer-Quil, C.
and
Bontozoglou, V.
2014.
Extreme solitary waves on falling liquid films.
Journal of Fluid Mechanics,
Vol. 745,
Issue. ,
p.
564.
Ding, Zijing
and
Wong, Teck Neng
2015.
Falling liquid films on a slippery substrate with Marangoni effects.
International Journal of Heat and Mass Transfer,
Vol. 90,
Issue. ,
p.
689.
Yadav, Anjalaiah
Chakraborty, S.
and
Usha, R.
2015.
Steady solution of an inverse problem in gravity-driven shear-thinning film flow: Reconstruction of an uneven bottom substrate.
Journal of Non-Newtonian Fluid Mechanics,
Vol. 219,
Issue. ,
p.
65.
Ghosh, Sukhendu
and
Usha, R.
2015.
Stability Analysis of a Gravity Driven Miscible Two-fluid Flow: Role of Wall Slip.
Procedia IUTAM,
Vol. 15,
Issue. ,
p.
264.
Thual, O.
Lacaze, L.
Mouzouri, M.
and
Boutkhamouine, B.
2015.
Critical slope for laminar transcritical shallow-water flows.
Journal of Fluid Mechanics,
Vol. 783,
Issue. ,
Anjalaiah
and
Usha, R.
2015.
Effects of velocity slip on the inertialess instability of a contaminated two-layer film flow.
Acta Mechanica,
Vol. 226,
Issue. 9,
p.
3111.
Usha, R.
and
Anjalaiah
2016.
Steady solution and spatial stability of gravity-driven thin-film flow: reconstruction of an uneven slippery bottom substrate.
Acta Mechanica,
Vol. 227,
Issue. 6,
p.
1685.
Ghosh, Sukhendu
and
Usha, R.
2016.
Stability of viscosity stratified flows down an incline: Role of miscibility and wall slip.
Physics of Fluids,
Vol. 28,
Issue. 10,
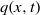 and the film thickness
and the film thickness  . Linear stability analysis of the averaged equations shows good agreement with the Orr–Sommerfeld analysis. The effect of a slip at the wall on the primary instability has been found to be non-trivial. Close to the instability onset, the effect is destabilising whereas it becomes stabilising at larger values of the Reynolds number. Nonlinear travelling waves are amplified by the presence of the slip. Comparisons to direct numerical simulations show a remarkable agreement for all tested values of parameters. The averaged equations capture satisfactorily the speed, shape and velocity distribution in the waves. The Navier slip condition is observed to significantly enhance the backflow phenomenon in the capillary region of the solitary waves with a possible effect on heat and mass transfer.
. Linear stability analysis of the averaged equations shows good agreement with the Orr–Sommerfeld analysis. The effect of a slip at the wall on the primary instability has been found to be non-trivial. Close to the instability onset, the effect is destabilising whereas it becomes stabilising at larger values of the Reynolds number. Nonlinear travelling waves are amplified by the presence of the slip. Comparisons to direct numerical simulations show a remarkable agreement for all tested values of parameters. The averaged equations capture satisfactorily the speed, shape and velocity distribution in the waves. The Navier slip condition is observed to significantly enhance the backflow phenomenon in the capillary region of the solitary waves with a possible effect on heat and mass transfer.