Published online by Cambridge University Press: 26 October 2018
We report experimental observations of turbulent flow with spherical particles in a square duct. Three particle sizes, namely 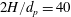 $2H/d_{p}=40$, 16 and 9 (
$2H/d_{p}=40$, 16 and 9 (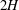 $2H$ being the duct full height and
$2H$ being the duct full height and  $d_{p}$ being the particle diameter), are investigated. The particles are nearly neutrally buoyant with a density ratio of 1.0035 and 1.01 with respect to the suspending fluid. Refractive index matched–particle image velocimetry (RIM–PIV) is used for fluid velocity measurement even at the highest particle volume fraction (20 %) and particle tracking velocimetry (PTV) for the particle velocity statistics for the flows seeded with particles of the two largest sizes, whereas only pressure measurements are reported for the smallest particles. Settling effects are seen at the lowest bulk Reynolds number
$d_{p}$ being the particle diameter), are investigated. The particles are nearly neutrally buoyant with a density ratio of 1.0035 and 1.01 with respect to the suspending fluid. Refractive index matched–particle image velocimetry (RIM–PIV) is used for fluid velocity measurement even at the highest particle volume fraction (20 %) and particle tracking velocimetry (PTV) for the particle velocity statistics for the flows seeded with particles of the two largest sizes, whereas only pressure measurements are reported for the smallest particles. Settling effects are seen at the lowest bulk Reynolds number  $Re_{2H}\approx$ 10 000, whereas, at the highest
$Re_{2H}\approx$ 10 000, whereas, at the highest 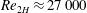 $Re_{2H}\approx 27\,000$, particles are in almost full suspension. The friction factor of the suspensions is found to be significantly larger than that of single-phase duct flow at the lower
$Re_{2H}\approx 27\,000$, particles are in almost full suspension. The friction factor of the suspensions is found to be significantly larger than that of single-phase duct flow at the lower 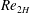 $Re_{2H}$ investigated; however, the difference decreases when increasing the flow rate and the total drag approaches the values of the single-phase flow at the higher Reynolds number considered,
$Re_{2H}$ investigated; however, the difference decreases when increasing the flow rate and the total drag approaches the values of the single-phase flow at the higher Reynolds number considered, 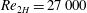 $Re_{2H}=27\,000$. The pressure drop is found to decrease with the particle diameter for volume fractions lower than
$Re_{2H}=27\,000$. The pressure drop is found to decrease with the particle diameter for volume fractions lower than  $\unicode[STIX]{x1D719}=10\,\%$ for nearly all
$\unicode[STIX]{x1D719}=10\,\%$ for nearly all 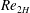 $Re_{2H}$ investigated. However, at the highest volume fraction
$Re_{2H}$ investigated. However, at the highest volume fraction 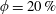 $\unicode[STIX]{x1D719}=20\,\%$, we report a peculiar non-monotonic behaviour: the pressure drop first decreases and then increases with increasing particle size. The decrease of the turbulent drag with particle size at the lowest volume fractions is related to an attenuation of the turbulence. The drag increase for the two largest particle sizes at
$\unicode[STIX]{x1D719}=20\,\%$, we report a peculiar non-monotonic behaviour: the pressure drop first decreases and then increases with increasing particle size. The decrease of the turbulent drag with particle size at the lowest volume fractions is related to an attenuation of the turbulence. The drag increase for the two largest particle sizes at 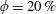 $\unicode[STIX]{x1D719}=20\,\%$, however, occurs despite this large reduction of the turbulent stresses, and it is therefore due to significant particle-induced stresses. At the lowest Reynolds number, the particles reside mostly in the bottom half of the duct, where the mean velocity significantly decreases; the flow is similar to that in a moving porous bed near the bottom wall and to turbulent duct flow with low particle concentration near the top wall.
$\unicode[STIX]{x1D719}=20\,\%$, however, occurs despite this large reduction of the turbulent stresses, and it is therefore due to significant particle-induced stresses. At the lowest Reynolds number, the particles reside mostly in the bottom half of the duct, where the mean velocity significantly decreases; the flow is similar to that in a moving porous bed near the bottom wall and to turbulent duct flow with low particle concentration near the top wall.