Hostname: page-component-cd9895bd7-q99xh Total loading time: 0 Render date: 2024-12-26T17:14:33.107Z Has data issue: false hasContentIssue false
  • English
  • Français

Evolution of the density self-correlation in developing Richtmyer–Meshkov turbulence

Published online by Cambridge University Press:  24 October 2013

C. D. Tomkins ,
B. J. Balakumar ,
G. Orlicz ,
K. P. Prestridge  and
J. R. Ristorcelli
C. D. Tomkins*
Affiliation:
Physics Division, Los Alamos National Laboratory, Los Alamos, NM 87545, USA
B. J. Balakumar
Affiliation:
Physics Division, Los Alamos National Laboratory, Los Alamos, NM 87545, USA
G. Orlicz
Affiliation:
Physics Division, Los Alamos National Laboratory, Los Alamos, NM 87545, USA
K. P. Prestridge
Affiliation:
Physics Division, Los Alamos National Laboratory, Los Alamos, NM 87545, USA
J. R. Ristorcelli
Affiliation:
Physics Division, Los Alamos National Laboratory, Los Alamos, NM 87545, USA
*
†Email address for correspondence: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Turbulent mixing in a Richtmyer–Meshkov unstable light–heavy–light (air–${\mathrm{SF} }_{6} $–air) fluid layer subjected to a shock (Mach 1.20) and a reshock (Mach 1.14) is investigated using ensemble statistics obtained from simultaneous velocity–density measurements. The mixing is driven by an unstable array of initially symmetric vortices that induce rapid material mixing and create smaller-scale vortices. After reshock the flow appears to transition to a turbulent (likely three-dimensional) state, at which time our planar measurements are used to probe the developing flow field. The density self-correlation $b= - \langle \rho v\rangle $ (where 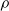$\rho $ and $v$ are the fluctuating density and specific volume, respectively) and terms in its evolution equation are directly measured experimentally for the first time. Amongst other things, it is found that production terms in the $b$ equation are balanced by the dissipation terms, suggesting a form of equilibrium in $b$. Simultaneous velocity measurements are used to probe the state of the incipient turbulence. A length-scale analysis suggests that an inertial range is beginning to form, consistent with the onset of a mixing transition. The developing turbulence is observed to reduce non-Boussinesq effects in the flow, which are found to be small over much of the layer after reshock. Second-order two-point structure functions of the density field exhibit a power-law behaviour with a steeper exponent than the standard $2/ 3$ power found in canonical turbulence. The absence of a significant 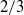$2/ 3$ region is observed to be consistent with the state of the flow, and the emergence of the steeper power-law region is discussed.

JFM classification

Instability: Transition to turbulence Turbulent Flows: Turbulence modelling Mixing: Turbulent mixing
Type
Papers
Information
Journal of Fluid Mechanics , Volume 735 , 25 November 2013 , pp. 288 - 306
Copyright
©2013 Cambridge University Press.

References

Antonia, R., Zhu, Y. & Kim, J. 1993 On the measurement of lateral velocity derivatives in turbulent flows. Exp. Fluids 15, 65–69.Google Scholar
Arnett, W. D., Bahcall, J. N., Kirshner, R. P. & Woosley, S. E. 1987 Supernova 1987A. Annu. Rev. Astron. Astrophys. 27, 629–700.Google Scholar
Bakosi, J. & Ristorcelli, J. R. 2011 Stochastic diffusion process for density-dependent multi-material turbulent mixing. Tech. Rep. LA-UR-11-01618. Los Alamos National Laboratory.Google Scholar
Balakumar, B. J., Orlicz, G. C., Ristorcelli, J. R., Balasubramanian, S., Prestridge, K. P. & Tomkins, C. D. 2012 Turbulent mixing in a Richtmyer–Meshkov fluid layer after reshock: velocity and density statistics. J. Fluid Mech. 696, 67–93.Google Scholar
Balakumar, B. J., Orlicz, G. C., Tomkins, C. D. & Prestridge, K. P. 2008 Simultaneous particle-image velocimetry–planar laser-induced fluorescence measurements of Richtmyer–Meshkov instability growth in a gas curtain with and without reshock. Phys. Fluids 20 (12), 124103.CrossRefGoogle Scholar
Banerjee, A., Gore, R. & Andrews, M. 2010a Development and validation of a turbulent mix model for variable-density and compressible flows. Phys. Rev. E 82, 046309.CrossRefGoogle ScholarPubMed
Banerjee, A., Kraft, W. & Andrews, M. 2010b Detailed measurements of a statistically steady Rayleigh–Taylor mixing layer from small to high Atwood numbers. J. Fluid Mech. 659, 127–190.Google Scholar
Besnard, D., Harlow, F. H., Rauenzahn, R. M. & Zemach, C. 1992 Turblence transport equations for variable-density turbulence and their relationship to two-field models. Tech. Rep. LA-12303-MS. Los Alamos National Laboratory.Google Scholar
Chassaing, P., Antonia, R. A., Anselmet, F., Joly, L. & Sarkar, S. 2002 Variable Density Fluid Turbulence, Fluid Mechanics and its Applications, vol. 69, Kluwer.Google Scholar
Dimotakis, P. 2000 The mixing transition in turbulent flows. J. Fluid Mech. 409, 69–98.Google Scholar
Dimotakis, P. E. 2005 Turbulent mixing. Annu. Rev. Fluid Mech. 37, 329–356.Google Scholar
Gregoire, O., Souffland, D. & Gauthier, S. 2005 A second-order turbulence model for gaseous mixtures induced by Richtmyer–Meshkov instability. J. Turbul. 6, N29.Google Scholar
Hill, D. J., Pantano, C. & Pullin, D. I. 2006 Large-eddy simulation and multiscale modelling of a Richtmyer–Meshkov instability with reshock. J. Fluid Mech. 557, 29–61.Google Scholar
Launder, B. E. 1989 Second-moment closure: Present ... and future? Intl J. Heat Fluid Flow 10 (4), 282–300.Google Scholar
Lindl, J. D., McCrory, R. L. & Campbell, E. M. 1992 Progress toward ignition and burn propagation in inertial confinement fusion. Phys. Today 377 (9), 32–40.Google Scholar
Livescu, D. & Ristorcelli, J. R. 2008 Variable-density mixing in buoyancy-driven turbulence. J. Fluid Mech. 605, 145–180.CrossRefGoogle Scholar
Livescu, D. & Ristorcelli, J. R. 2009 Mixing asymmetry in variable density turbulence. Adv. Turbul. XII 132 (1), 545–548.Google Scholar
Livescu, D., Ristorcelli, J. R., Gore, R. A., Dean, S. H., Cabot, W. H. & Cook, A. W. 2009 High-Reynolds number Rayleigh–Taylor turbulence. J. Turbul. 10 (13), 1–32.Google Scholar
Lombardini, M., Pullin, D. I. & Meiron, D. I. 2012 Transition to turbulence in shock-driven mixing: a Mach number study. J. Fluid Mech. 690, 203–226.Google Scholar
Moran-Lopez, J. T. & Schilling, O. 2013 Multicomponent Reynolds-averaged Navier–Stokes simulations of reshocked Richtmyer–Meshkov instability-induced mixing. High Energy Density Phys. 9, 112–121.CrossRefGoogle Scholar
Orlicz, G. 2012 Incident shock Mach number effects on Richtmyer–Meshkov mixing with simultaneous density and velocity measurements. PhD thesis, University of New Mexico.Google Scholar
Reid, R. C., Prausnitz, J. M. & Sherwood, T. K. 1977 The properties of gases and liquids. McGraw-Hill.Google Scholar
Rightley, P. M., Vorobieff, P. & Benjamin, R. F. 1997 Evolution of a shock-accelerated thin fluid layer. Phys. Fluids 9 (6), 1770–1782.Google Scholar
Robey, H. F., Zhou, Y., Buckingham, A. C., Keiter, P., Remington, B. A. & Drake, R. P. 2003 The time scale for the transition to turbulence in a high Reynolds number, accelerated flow. Phys. Plasmas 10 (3), 614–622.Google Scholar
Schilling, O. & Latini, M. 2010 High-order WENO simulations of three-dimensional reshocked Richtmyer–Meshkov instability to late times: dynamics, dependence on initial conditions, and comparisons to experimental data. Math. Acta Sci. 30B (2), 595–620.Google Scholar
Schwarzkopf, J., Livescu, D., Gore, R., Rauenzahn, R. & Ristorcelli, J. R. 2011 Application of a second-moment closure model to mixing processes involving multicomponent miscible fluids. J. Turbul. 12 (49), 1–35.CrossRefGoogle Scholar
Tanaka, T. & Eaton, J. 2007 A correction method for measuring turbulence kinetic energy dissipation rate by PIV. Exp. Fluids 42, 893–902.Google Scholar
Tomkins, C., Kumar, S., Orlicz, G. & Prestridge, K. 2008 An experimental investigation of mixing mechanisms in shock-accelerated flow. J. Fluid Mech. 611, 131–150.Google Scholar
Tomkins, C., Prestridge, K., Rightley, P., Marr-Lyon, M., Vorobieff, P. & Benjamin, R. 2003 A quantitative study of the interaction of two Richtmyer–Meshkov-unstable gas cylinders. Phys. Fluids 15 (4), 986–1004.Google Scholar
Vorobieff, P., Mohamed, N. G., Tomkins, C., Goodenough, C., Marr-Lyon, M. & Benjamin, R. F. 2003 Scaling evolution in shock-induced transition to turbulence. Phys. Rev. E 68 (6), 065301.Google Scholar
Vorobieff, P., Rightley, P. M. & Benjamin, R. F. 1998 Power-law spectra of incipient gas-curtain turbulence. Phys. Rev. Lett. 81 (11), 2240–2243.Google Scholar
Vorobieff, P., Rightley, P. M. & Benjamin, R. F. 1999 Shock-driven gas curtain: fractal dimension evolution in transition to turbulence. Physica D 133, 469–476.Google Scholar
Warhaft, Z. 2000 Passive scalars in turbulent flows. Annu. Rev. Fluid Mech. 32, 203–240.Google Scholar
Weber, C., Haehn, N., Oakley, J., Rothamer, D. & Bonazza, R. 2012 Turbulent mixing measurements in the Richtmyer–Meshkov instability. Phys. Fluids 24, 074105.CrossRefGoogle Scholar
Williams, F. 1994 Combustion Theory. Perseus Books Publishing.Google Scholar
Yang, J., Kubota, T. & Zukoski, E. E. 1993 Applications of shock-induced mixing to supersonic combustion. AIAA J. 31 (5), 854–862.Google Scholar
Youngs, D. L. 1984 Numerical simulation of turbulent mixing by Rayleigh–Taylor instability. Physica D 12, 32–44.Google Scholar
Zabusky, N. J. 1999 Vortex paradigm for accelerated inhomogeneous flows: visiometrics for the Rayleigh–Taylor and Richtmyer–Meshkov environments. Annu. Rev. Fluid Mech. 31, 495–536.Google Scholar
Zhou, Y., Robey, H. F. & Buckingham, A. C. 2003a Onset of turbulence in accelerated high-Reynolds-number flow. Phys. Rev. E 67, 056305.Google Scholar
Zhou, Y., Robey, H. F., Buckingham, A. C., Keiter, P., Remington, B. A. & Drake, R. P. 2003b The time scale for the transition to turbulence in a high Reynolds number, accelerated flow. Phys. Plasmas 10 (3), 614–622.Google Scholar