No CrossRef data available.
Article contents
Equatorial modons in thermal rotating shallow water model
Published online by Cambridge University Press: 08 April 2024
Abstract
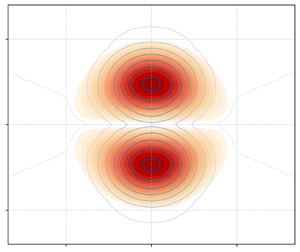
Exact steady eastward-moving vortex-dipole solutions, the equatorial modons, are constructed in the asymptotic limit of low divergence and small temperature variations in the thermal rotating shallow water (TRSW) model on the equatorial beta-plane. This regime is known to be relevant for the tropical atmosphere. The model itself is a generalization, allowing for horizontal temperature gradients, of the classical rotating shallow water model. The asymptotic modons can carry a temperature anomaly and exist also on the inhomogeneous temperature background. The modon configurations are then used to initialize numerical simulations, in order to check whether such coherent structures can exist in the full TRSW model. The results show that this is, indeed, the case. The parameter regimes and limitations on the structure of the temperature anomaly inside, in order for the modons to persist, are established. It is also shown that the modons keep their coherence even while evolving on the background of meridionally inhomogeneous temperature fields, or while interacting with sharp temperature fronts. A general scenario of disaggregation of the modons, if they are out of the stability domain, is exhibited and analysed.
JFM classification
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2024. Published by Cambridge University Press



