Hostname: page-component-586b7cd67f-2brh9 Total loading time: 0 Render date: 2024-11-28T14:49:08.494Z Has data issue: false hasContentIssue false
  • English
  • Français

Entrainment and mixing in a laboratory model of oceanic overflow

Published online by Cambridge University Press:  04 April 2014

Philippe Odier ,
Jun Chen  and
Robert E. Ecke
Philippe Odier*
Affiliation:
Condensed Matter and Thermal Physics Group and Center for Nonlinear Studies, Los Alamos National Laboratory, Los Alamos, NM 87545, USA Laboratoire de Physique, ENS Lyon, 46 allée d’Italie, 69364 Lyon CEDEX 07, France
Jun Chen
Affiliation:
Condensed Matter and Thermal Physics Group and Center for Nonlinear Studies, Los Alamos National Laboratory, Los Alamos, NM 87545, USA School of Mechanical Engineering, Purdue University, West Lafayette, IN 47907, USA
Robert E. Ecke
Affiliation:
Condensed Matter and Thermal Physics Group and Center for Nonlinear Studies, Los Alamos National Laboratory, Los Alamos, NM 87545, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

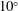We present experimental measurements of a wall-bounded gravity current, motivated by characterizing natural gravity currents such as oceanic overflows. We use particle image velocimetry and planar laser-induced fluorescence to simultaneously measure the velocity and density fields as they evolve downstream of the initial injection from a turbulent channel flow onto a plane inclined at $10^\circ $ with respect to horizontal. The turbulence level of the input flow is controlled by injecting velocity fluctuations upstream of the output nozzle. The initial Reynolds number based on the Taylor microscale of the flow, 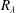$R_{\lambda }$, is varied between 40 and 120, and the effects of the initial turbulence level are assessed. The bulk Richardson number 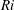$\mathit{Ri}$ for the flow is ${\sim }$0.3 whereas the gradient Richardson number 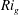$\mathit{Ri}_g$ varies between 0.04 and 0.25, indicating that shear dominates the stabilizing effect of stratification. Kelvin–Helmholtz instability results in vigorous vertical transport of mass and momentum. We present baseline characterization of standard turbulence quantities and calculate, in several different ways, the fluid entrainment coefficient $E$, a quantity of considerable interest in mixing parameterization for ocean circulation models. We also determine the properties of mixing as represented by the flux Richardson number 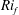$\mathit{Ri}_f$ as a function of $\mathit{Ri}_g$ and diapycnal mixing parameter $K_{\rho }$ versus the buoyancy Reynolds number $\mathit{Re}_b$. We find reasonable agreement with results from natural flows.

JFM classification

Geophysical and Geological Flows: Gravity currents Geophysical and Geological Flows: Stratified flows Mixing: Turbulent mixing
Type
Papers
Information
Journal of Fluid Mechanics , Volume 746 , 10 May 2014 , pp. 498 - 535
Copyright
© 2014 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alahyari, A. & Longmire, E. K. 1994 Particle image velocimetry in a variable density flow: application to a dynamically evolving microburst. Exp. Fluids 17, 434–440.Google Scholar
Alavian, V. 1986 Behavior of density currents on an incline. ASCE J. Hydraul. Engng 112, 27–42.CrossRefGoogle Scholar
Atsavapranee, P. & Gharib, M. 1997 Structures in stratified plane mixing layers and the effects of cross-shear. J. Fluid Mech. 342, 53–86.Google Scholar
Bacon, S. 1998 Decadal variablity in the outflow from the Nordic seas to the deep Atlantic Ocean. Nature 394, 871–874.Google Scholar
Baines, P. G. 2001 Mixing in flows down gentle slopes into stratified environment. J. Fluid Mech. 443, 237–270.Google Scholar
Baines, P. G. 2002 Two-dimensional plumes in stratified environments. J. Fluid Mech. 471, 315–337.Google Scholar
Baines, P. G. 2005 Mixing regimes for the flow of dense fluid down slopes into stratified environment. J. Fluid Mech. 538, 245–267.CrossRefGoogle Scholar
Barry, M. E., Ivey, G. N., Winters, K. B. & Imberger, J. 2001 Measurements of diapycnal diffusivities in stratified fluids. J. Fluid Mech. 442, 267–291.Google Scholar
Bluteau, C. E., Jones, N. L. & Ivey, G. N. 2013 Turbulent mixing efficiency at an energetic ocean site. J. Geophys. Res.-Oceans 118, 4662–4672.Google Scholar
Borg, A., Bolinder, J. & Fuchs, L. 2001 Simultaneous velocity and concentration measurements in the near field of a turbulent low-pressure jet by digital particle image velocimetry – planar laser-induced fluorescence. Exp. Fluids 31, 140–152.CrossRefGoogle Scholar
Bouffard, D. & Boegman, L. 2013 A diapycnal diffusivity model for stratified environmental flows. Dyn. Atmos. Oceans 61–62, 14–34.CrossRefGoogle Scholar
Broecker, W. S. 1997 Thermohaline circulation, the Achilles heel of our climate system: will man-made $\mathrm{CO}_{2}$ upset the current balance?. Science 278 (5343), 1582–1588.Google Scholar
Cenedese, C. & Adduce, C. 2008 Mixing in a density-driven current flowing down a slope in a rotating fluid. J. Fluid Mech. 604, 369–388.CrossRefGoogle Scholar
Cenedese, C. & Adduce, C. 2010 A new parameterization for entrainment in overflows. J. Phys. Oceanogr. 40 (8), 1835–1850.Google Scholar
Cenedese, C., Whitehead, J. A., Ascarelli, T. A. & Ohiwa, M. 2004 A dense current flowing down a sloping bottom in a rotating fluid. J. Phys. Oceanogr. 34, 188–203.Google Scholar
Chen, J., Meneveau, C. & Katz, J. 2006 Scale interactions of turbulence subjected to a straining–relaxation–destraining cycle. J. Fluid Mech. 562, 123–150.Google Scholar
Crimaldi, J. P. 1997 The effect of photobleaching and velocity fluctuations on single-point LIF measurements. Exp. Fluids 23 (4), 325–330.Google Scholar
Crimaldi, J. P. 2008 Planar laser induced fluorescence in aqueous flows. Exp. Fluids 44 (6), 851–863.Google Scholar
Daviero, G. J., Roberts, P. J. W. & Maile, K. 2001 Refractive index matching in large-scale stratified experiments. Exp. Fluids 31, 119–126.CrossRefGoogle Scholar
Doron, P., Bertuccioli, L., Katz, J. & Osborn, T. 2001 Turbulence characteristics and dissipation estimates in the costal ocean bottom boundary layer from PIV data. J. Phys. Oceanogr. 31, 2108–2134.Google Scholar
Du, H., Fuh, R. A., Li, J., Corkan, A. & Lindsey, J. S. 1998 PhotochemCAD: a computer-aided design and research tool in photochemistry. Photochem. Photobiol. 68, 141–142.Google Scholar
Ellison, T. H. 1957 Turbulent transport of heat and momentum from an infinite rough plane. J. Fluid Mech. 2 (5), 456–466.Google Scholar
Ellison, T. H. & Turner, J. S. 1959 Turbulent entrainment in stratified flows. J. Fluid Mech. 6, 423–448.Google Scholar
Feng, H., Olsen, M. G., Hill, J. C. & Fox, R. O. 2007 Simultaneous velocity and concentration filed measurements of passive-scalar mixing in a confined rectangular jet. Exp. Fluids 42, 847–862.CrossRefGoogle Scholar
Ferrier, A. J., Funk, D. R. & Roberts, P. J. W 1993 Application of optical techniques to the study of plumes in stratified fluids. Dyn. Atmos. Oceans 20, 155–183.Google Scholar
Girton, J. & Sanford, T. 2003 Desent and modification of the overflow plume in the Denmark Strait. J. Phys. Oceanogr. 33, 1351–1364.Google Scholar
Girton, J., Sanford, T. & Kase, R. 2001 Synoptic sections of the Denmark Strait overflow. Geophys. Res. Lett. 28, 1619–1622.Google Scholar
Hallworth, M. A., Phillips, J. C., Huppert, H. E., Stephen, R. & Sparks, J. 1993 Entrainment in turbulent gravity currents. Nature 362, 829–831.Google Scholar
Hannoun, I. A., Fernando, H. J. S. & List, E. J. 1988 Turbulent structure near a sharp density interface. J. Fluid Mech. 189, 189–209.Google Scholar
Hansen, B. & Østerhus, S. 2000 North Atlantic–Nordic seas exchange. Prog. Oceanogr. 45, 109–208.Google Scholar
Hjertager, L. K., Hjertager, B. H., Deen, N. G. & Solberg, T. 2003 Measurement of turbulent mixing in a confined wake flow using combined PIV and PLIF. Can. J. Chem. Engng 81, 1149–1158.Google Scholar
Hu, H., Kobayashi, T., Segawa, S. & Taniguchi, N. 2000 Particle image velocimetry and planar laser-induced fluorescence measurements on lobed jet mixing flows. Exp. Fluids (Suppl.), S141–S157.Google Scholar
Huang, H., Diabiri, D. & Gharib, M. 1997 On the error of digital particle image velocimetry. Meas. Sci. Technol. 8, 1427–1440.Google Scholar
Hult, E. L., Troy, C. D. & Koseff, J. R. 2011 The mixing efficiency of interfacial waves breaking at a ridge: 2. Local mixing processes. J. Geophys. Res.-Oceans 116, C02004.Google Scholar
Huppert, H. E. 2006 Gravity currents: a personal perspective. J. Fluid Mech. 554, 299–322.Google Scholar
Ilicak, M., Özgökmen, T. M., Baumert, H. Z. & Iskandarani, M. 2008 Performance of two-equation turbulence closures in three-dimensional simulations of the red sea overflow. Ocean Model. 24, 122–139.Google Scholar
Istweire, E. C., Koseff, J. R., Briggs, D. A. & Ferziger, J. H. 1993 Turbulence in stratified shear flows – implications for interpreting shear-induced mixing in the ocean. J. Fluid Mech. 23 (7), 1508–1522.Google Scholar
Ivey, G. N., Winters, K. B. & Koseff, J. R. 2008 Density stratification, turbulence, but how much mixing?. Annu. Rev. Fluid Mech. 40, 169–184.CrossRefGoogle Scholar
Jackson, L., Hullberg, R. & Legg, S. 2008 A parameterization of shear-driven turbulence for ocean climate models. J. Phys. Oceanogr. 38, 1033–1053.Google Scholar
Kang, H., Stuart, C. & Meneveau, C. 2003 Decaying turbulence in an active-grid-generated flow and comparisions with large-eddy-simulation. J. Fluid Mech. 480, 129–160.CrossRefGoogle Scholar
Karasso, P. S. & Mungal, M. G. 1997 Plif measurements in aqueous flows using the Nd:YAG laser. Exp. Fluids 27, 82–87.Google Scholar
Keane, R. & Adrian, R. 1990 Optimization of particle image velocimeters, part I, double pulsed systems. Meas. Sci. Technol. 1, 1202–1215.Google Scholar
Knauss, J. A. 1978 Introduction to Physical Oceanography. Prentice-Hall Inc.Google Scholar
Kneller, B. C., Bennett, S. J. & McCaffrey, W. D. 1999 Velocity structure, turbulence and fluid stresses in experimental gravity current. J. Geophys. Res. 104, 5381–5391.Google Scholar
Koop, C. G. & Browand, F. K. 1979 Instability and turbulence in a stratified fluid with shear. J. Fluid Mech. 93 (01), 135–159.Google Scholar
Krug, D., Holzner, M., Luethi, B., Wolf, M., Kinzelbach, W. & Tsinober, A. 2013 Experimental study of entrainment and interface dynamics in a gravity current. Exp. Fluids 54 (5), 1530.Google Scholar
Lane-Serff, G. F. & Baines, P. G. 1998 Eddy formation by dense flows on slopes in a rotating fluid. J. Fluid Mech. 363, 229–252.Google Scholar
Launder, B. E. & Rodi, W. 1983 The turbulent wall jet – measurements and modeling. Annu. Rev. Fluid Mech. 15, 429–459.Google Scholar
Legg, S., Hallberg, R. W. & Girton, J. B. 2006 Comparison of entrainment in overflows simulated by $z$ -coordinate, isopycnal and non-hydrostatic models. Ocean Model. 11, 69–97.CrossRefGoogle Scholar
Linden, P. F. 1979 Mixing in stratified fluids. Geophys. Astrophys. Fluid Dyn. 13, 2–23.Google Scholar
Lowe, R. J., Linden, P. F. & Rottman, J. W. 2002 A laboratory study of the velocity structure in an intrusive gravity current. J. Fluid Mech. 456, 33–48.Google Scholar
Makita, H. 1991 Realization of a large-scale turbulence field in a small wind tunnel. Fluid Dyn. Res. 8, 53–64.Google Scholar
McDougall, T. J. 1979 On the elimination of refractive-index variations in turbulent density-stratified liquid flows. J. Fluid Mech. 93, 83–96.Google Scholar
Mellor, G. L. & Yamada, T. 1974 A hierarchy of turbulence closure models for planetary boundary layers. J. Atmos. Sci. 31, 1791–1806.Google Scholar
Mellor, G. L. & Yamada, T. 1982 Development of a turbulence closure model for geophysical fluid problems. Rev. Geophys. Space Phys. 20, 851–875.Google Scholar
Monin, A. & Yaglom, A. 1971 Statistical Fluid Mechanics. MIT Press.Google Scholar
Morton, B. R., Taylor, G. I. & Turner, J. S. 1956 Turbulent gravitational convection from maintained and instantaneous sources. Proc. R. Soc. Lond. A234, 1–23.Google Scholar
Mydlarski, L. & Warhaft, Z. 1996 On the onset of high-Reynolds-number grid-generated wind tunnel turbulence. J. Fluid Mech. 320, 331–368.Google Scholar
Odier, P., Chen, J. & Ecke, R. E. 2012 Understanding and modeling turbulent fluxes and entrainment in a gravity current. Physica D 241 (3, SI), 260–268.Google Scholar
Odier, P., Chen, J., Rivera, M. & Ecke, R. 2009 Fluid mixing in stratified gravity currents: the Prandtl mixing length. Phys. Rev. Lett. 102, 134504.CrossRefGoogle ScholarPubMed
Osborn, T. R. 1980 Estimates of the local-rate of vertical diffusion from dissipation measurements. J. Phys. Oceanogr. 10 (1), 83–89.Google Scholar
Özgökmen, T. M., Fischer, P. F., Duan, J. & Iliescu, T. 2004 Three-dimensional turbulent bottom density currents from a high-order nonhydrostatic spectral element model. J. Phys. Oceanogr. 34, 2006–2026.Google Scholar
Pardyjak, E. R., Monti, P. & Fernando, H. J. S. 2002 Flux richardson number measurements in stable atmosphere shear flows. J. Fluid Mech. 459, 307–316.Google Scholar
Peltier, W. & Caulfield, C. 2003 Mixing efficiency in stratified shear flows. Annu. Rev. Fluid Mech. 35, 135–167.Google Scholar
Pope, S. 2000 Turbulent Flows. Cambridge University Press.Google Scholar
Price, J., Baringer, M., Lueck, R., Johnson, G., Ambar, I., Parrilla, G., Cantos, A., Kennelly, M. A. & Sanford, T. B. 1993 Mediterranean outflow mixing and dynamics. Science 259, 1278–1282.Google Scholar
Princevac, M., Fernando, H. J. S. & Whiteman, C. D. 2005 Turbulent entrainment into natural gravity-driven flows. J. Fluid Mech. 533, 259–268.Google Scholar
Raffel, M., Willert, C. & Kompenhans, J. 1998 Particle Image Velocimetry – A Practical Guide. Springer.Google Scholar
Raffel, M., Willert, C. E., Wereley, S. T. & Kompenhans, J. 2007 Particle Image Velocimetry – A Practical Guide. 2nd edn. Springer.Google Scholar
Rohr, J. J., Itsweire, E. C., Helland, K. N. & Van Atta, C. W. 1988 Growth and decay of turbulence in a stably stratified shear-flow. J. Fluid Mech. 195, 77–111.Google Scholar
Roth, G. & Katz, J. 2001 Five techniques for increasing the speed and accuracy of PIV interrogaton. Meas. Sci. Technol. 12, 238–245.Google Scholar
Schwarz, W. H. & Cosart, W. P. 1961 The two-dimensional turbulent wall-jet. J. Fluid Mech. 10, 481–495.Google Scholar
Shapiro, G. I. & Zatsepin, A. G. 1997 Gravity current down a steeply inclined slope in a rotating fluid. Ann. Geophys. 15, 366–374.Google Scholar
Shih, L. H., Koseff, J. R., Ivey, G. N. & Ferziger, J. H. 2005 Parameterization of turbulent fluxes and scales using homogeneous sheared stably stratified turbulence simulations. J. Fluid Mech. 525, 193–214.Google Scholar
Simpson, J. E. 1982 Gravity currents in the laboratory, atmosphere, and ocean. Annu. Rev. Fluid Mech. 14, 213–234.CrossRefGoogle Scholar
Simpson, J. 1987 Gravity Currents: In the Environment and the Laboratory. Ellis Horwood Ltd.Google Scholar
Stillinger, D. C., Helland, K. N. & Atta, C. W. Van 1983 Experiments on the transition of homogeneous turbulence to internal waves in a stratified fluid. J. Fluid Mech. 131, 91.Google Scholar
Strang, E. J. & Fernando, H. J. S. 2001a Entrainment and mixing in stratified shear flows. J. Fluid Mech. 428, 349–386.Google Scholar
Strang, E. J. & Fernando, H. J. S. 2001b Vertical mixing and transports through a stratified shear layer. J. Phys. Oceanogr. 31, 2026–2048.Google Scholar
Strang, E. J. & Fernando, H. J. S. 2004 Shear-induced mixing and transport from a rectangular cavity. J. Fluid Mech. 520, 23–49.Google Scholar
Sutherland, B. R. 2002 Interfacial gravity currents. I. Mixing and entrainment. Exp. Fluids 14, 2244–2254.CrossRefGoogle Scholar
Tanaka, T. & Eaton, J. K. 2007 A correction method for measuring turbulence kinetic energy dissipation rate by PIV. Exp. Fluids.Google Scholar
Tennekes, H. & Lumley, J. 1972 A First Course in Turbulence. MIT Press.Google Scholar
Thomas, P. J. & Linden, P. F. 2007 Rotating gravity currents: small-scale and large-scale laboratory experiments and geostrophic model. J. Fluid Mech. 578, 35–65.CrossRefGoogle Scholar
Townsend, A. A. 1958 The effects of radiative transfer on turbulent flow of a stratified fluid. J. Fluid Mech. 361–372.Google Scholar
Troy, C. & Koseff, J. 2005 The generation and quantitative visualization of breaking internal waves. Exp. Fluids 38, 549–562.CrossRefGoogle Scholar
Turner, J. S. 1973 Buoyancy Effects in Fluids. Cambridge University Press.Google Scholar
Turner, J. S. 1986 Turbulent entrainment: the development of the entrainment assumption, and its application to geophysical flows. J. Fluid Mech. 173, 431–471.Google Scholar
Wells, M., Cenedese, C. & Caulfield, C. P. 2010 The relationship between flux coefficient and entrainment ratio in density currents. J. Phys. Oceanogr. 40 (12), 2713–2727.Google Scholar
Willebrand, J., Barnier, B., Böning, C., Dieterich, C., Killworth, P. D., Le Provost, C., Jia, Y., Molines, J. -M. & New, A. L. 2001 Circulation characteristics in three eddy-permitting models of the North Atlantic. Prog. Oceanogr. 48, 123–161.Google Scholar
Wunsch, C. & Ferrari, R. 2004 Vertical mixing, energy and thegeneral circulation of the oceans. Annu. Rev. Fluid Mech. 36, 281–314.Google Scholar
Wygnanski, I., Katz, Y. & Horev, E. 1992 On the applicability of various scaling laws to the turbulent wall jet. J. Fluid Mech. 234, 669–690.Google Scholar