Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Miller Bertolami, M. M.
Battich, T.
Córsico, A. H.
Christensen-Dalsgaard, J.
and
Althaus, L. G.
2019.
Asteroseismic signatures of the helium core flash.
Nature Astronomy,
Vol. 4,
Issue. 1,
p.
67.
Bowman, Dominic M.
Burssens, Siemen
Pedersen, May G.
Johnston, Cole
Aerts, Conny
Buysschaert, Bram
Michielsen, Mathias
Tkachenko, Andrew
Rogers, Tamara M.
Edelmann, Philipp V. F.
Ratnasingam, Rathish P.
Simón-Díaz, Sergio
Castro, Norberto
Moravveji, Ehsan
Pope, Benjamin J. S.
White, Timothy R.
and
De Cat, Peter
2019.
Low-frequency gravity waves in blue supergiants revealed by high-precision space photometry.
Nature Astronomy,
Vol. 3,
Issue. 8,
p.
760.
Bowman, D. M.
Aerts, C.
Johnston, C.
Pedersen, M. G.
Rogers, T. M.
Edelmann, P. V. F.
Simón-Díaz, S.
Van Reeth, T.
Buysschaert, B.
Tkachenko, A.
and
Triana, S. A.
2019.
Photometric detection of internal gravity waves in upper main-sequence stars.
Astronomy & Astrophysics,
Vol. 621,
Issue. ,
p.
A135.
Augustson, K. C.
and
Mathis, S.
2019.
A Model of Rotating Convection in Stellar and Planetary Interiors. I. Convective Penetration.
The Astrophysical Journal,
Vol. 874,
Issue. 1,
p.
83.
Aerts, Conny
Mathis, Stéphane
and
Rogers, Tamara M.
2019.
Angular Momentum Transport in Stellar Interiors.
Annual Review of Astronomy and Astrophysics,
Vol. 57,
Issue. 1,
p.
35.
Lecoanet, Daniel
Cantiello, Matteo
Quataert, Eliot
Couston, Louis-Alexandre
Burns, Keaton J.
Pope, Benjamin J. S.
Jermyn, Adam S.
Favier, Benjamin
and
Bars, Michael Le
2019.
Low-frequency Variability in Massive Stars: Core Generation or Surface Phenomenon?.
The Astrophysical Journal Letters,
Vol. 886,
Issue. 1,
p.
L15.
Augustson, K. C.
Mathis, S.
and
Astoul, A.
2020.
A Model of Rotating Convection in Stellar and Planetary Interiors. II. Gravito-inertial Wave Generation.
The Astrophysical Journal,
Vol. 903,
Issue. 2,
p.
90.
Khater, Mostafa M. A.
Zheng, Qiang
Qin, Haiyong
Attia, Raghda A. M.
and
Chen, Chuanjun
2020.
On Highly Dimensional Elastic and Nonelastic Interaction between Internal Waves in Straight and Varying Cross-Section Channels.
Mathematical Problems in Engineering,
Vol. 2020,
Issue. ,
p.
1.
Léard, P.
Favier, B.
Le Gal, P.
and
Le Bars, M.
2020.
Coupled convection and internal gravity waves excited in water around its density maximum at
4°C.
Physical Review Fluids,
Vol. 5,
Issue. 2,
Bowman, D. M.
Burssens, S.
Simón-Díaz, S.
Edelmann, P. V. F.
Rogers, T. M.
Horst, L.
Röpke, F. K.
and
Aerts, C.
2020.
Photometric detection of internal gravity waves in upper main-sequence stars.
Astronomy & Astrophysics,
Vol. 640,
Issue. ,
p.
A36.
Gossan, Sarah E
Fuller, Jim
and
Roberts, Luke F
2020.
Wave heating from proto-neutron star convection and the core-collapse supernova explosion mechanism.
Monthly Notices of the Royal Astronomical Society,
Vol. 491,
Issue. 4,
p.
5376.
Burns, Keaton J.
Vasil, Geoffrey M.
Oishi, Jeffrey S.
Lecoanet, Daniel
and
Brown, Benjamin P.
2020.
Dedalus: A flexible framework for numerical simulations with spectral methods.
Physical Review Research,
Vol. 2,
Issue. 2,
Lecoanet, Daniel
2020.
Fluid Mechanics of Planets and Stars.
Vol. 595,
Issue. ,
p.
31.
Couston, Louis-Alexandre
Lecoanet, Daniel
Favier, Benjamin
and
Le Bars, Michael
2020.
Shape and size of large-scale vortices: A generic fluid pattern in geophysical fluid dynamics.
Physical Review Research,
Vol. 2,
Issue. 2,
Jermyn, Adam S
Chitre, Shashikumar M
Lesaffre, Pierre
and
Tout, Christopher A
2020.
Convective differential rotation in stars and planets – II. Observational and numerical tests.
Monthly Notices of the Royal Astronomical Society,
Vol. 498,
Issue. 3,
p.
3782.
Barker, A J
Jones, C A
and
Tobias, S M
2020.
Angular momentum transport, layering, and zonal jet formation by the GSF instability: non-linear simulations at a general latitude.
Monthly Notices of the Royal Astronomical Society,
Vol. 495,
Issue. 1,
p.
1468.
Wu, Samantha
and
Fuller, Jim
2021.
A Diversity of Wave-driven Presupernova Outbursts.
The Astrophysical Journal,
Vol. 906,
Issue. 1,
p.
3.
Couston, Louis-Alexandre
and
Siegert, Martin
2021.
Dynamic flows create potentially habitable conditions in Antarctic subglacial lakes.
Science Advances,
Vol. 7,
Issue. 8,
Bouffard, M
Favier, B
Lecoanet, D
and
Le Bars, M
2021.
Internal gravity waves in a stratified layer atop a convecting liquid core in a non-rotating spherical shell.
Geophysical Journal International,
Vol. 228,
Issue. 1,
p.
337.
Couston, Louis-Alexandre
2021.
Turbulent convection in subglacial lakes.
Journal of Fluid Mechanics,
Vol. 915,
Issue. ,
 $Ra\in [4\times 10^{7},10^{9}]$) and large stratification (Brunt–Väisälä frequencies
$Ra\in [4\times 10^{7},10^{9}]$) and large stratification (Brunt–Väisälä frequencies 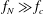 $f_{N}\gg f_{c}$, where
$f_{N}\gg f_{c}$, where 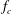 $f_{c}$ is the convective frequency), simulations are in good agreement with a theory that assumes waves are generated by Reynolds stresses due to eddies in the turbulent region as described in Lecoanet & Quataert (Mon. Not. R. Astron. Soc., vol. 430 (3), 2013, pp. 2363–2376). Specifically, we demonstrate that the wave energy flux spectrum scales like
$f_{c}$ is the convective frequency), simulations are in good agreement with a theory that assumes waves are generated by Reynolds stresses due to eddies in the turbulent region as described in Lecoanet & Quataert (Mon. Not. R. Astron. Soc., vol. 430 (3), 2013, pp. 2363–2376). Specifically, we demonstrate that the wave energy flux spectrum scales like 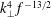 $k_{\bot }^{4}\,f^{-13/2}$ for weakly damped waves (with
$k_{\bot }^{4}\,f^{-13/2}$ for weakly damped waves (with 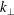 $k_{\bot }$ and
$k_{\bot }$ and 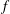 $f$ the waves’ horizontal wavenumbers and frequencies, respectively), and that the total wave energy flux decays with
$f$ the waves’ horizontal wavenumbers and frequencies, respectively), and that the total wave energy flux decays with  $z$, the distance from the convective region, like
$z$, the distance from the convective region, like  $z^{-13/8}$.
$z^{-13/8}$.