Article contents
The elemental shear dynamo
Published online by Cambridge University Press: 17 April 2012
Abstract
A quasi-linear theory is presented for how randomly forced, barotropic velocity fluctuations cause an exponentially growing, large-scale (mean) magnetic dynamo in the presence of a uniform parallel shear flow. It is a ‘kinematic’ theory for the growth of the mean magnetic energy from a small initial seed, neglecting the saturation effects of the Lorentz force. The quasi-linear approximation is most broadly justifiable by its correspondence with computational solutions of nonlinear magnetohydrodynamics, and it is rigorously derived in the limit of small magnetic Reynolds number, 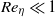 . Dynamo action occurs even without mean helicity in the forcing or flow, but random helicity variance is then essential. In a sufficiently large domain and with a small seed wavenumber in the direction perpendicular to the mean shearing plane, a positive exponential growth rate
. Dynamo action occurs even without mean helicity in the forcing or flow, but random helicity variance is then essential. In a sufficiently large domain and with a small seed wavenumber in the direction perpendicular to the mean shearing plane, a positive exponential growth rate  can occur for arbitrary values of
can occur for arbitrary values of 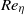 , viscous Reynolds number
, viscous Reynolds number 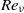 , and random-force correlation time
, and random-force correlation time 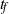 and orientation angle
and orientation angle 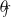 in the shearing plane. The value of
in the shearing plane. The value of 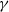 is independent of the domain size. The shear dynamo is ‘fast’, with finite
is independent of the domain size. The shear dynamo is ‘fast’, with finite 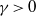 in the limit of
in the limit of 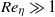 . Averaged over random realizations of the forcing history, the ensemble-mean magnetic field grows more slowly, if at all, compared to the r.m.s. field (magnetic energy). In the limit of small
. Averaged over random realizations of the forcing history, the ensemble-mean magnetic field grows more slowly, if at all, compared to the r.m.s. field (magnetic energy). In the limit of small 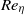 and
and 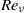 , the dynamo behaviour is related to the well-known alpha–omega ansatz when the force is slowly varying (
, the dynamo behaviour is related to the well-known alpha–omega ansatz when the force is slowly varying (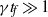 ) and to the ‘incoherent’ alpha–omega ansatz when the force is more rapidly fluctuating.
) and to the ‘incoherent’ alpha–omega ansatz when the force is more rapidly fluctuating.
- Type
- Papers
- Information
- Copyright
- Copyright © Cambridge University Press 2012 The online version of this article is published within an Open Access environment subject to the conditions of the Creative Commons Attribution-NonCommercial-ShareAlike licence <http://creativecommons.org/licenses/by-nc-sa/2.5/>. The written permission of Cambridge University Press must be obtained for commercial re-use.
References
- 16
- Cited by


