Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Das, Sayan
Mandal, Shubhadeep
Som, S. K.
and
Chakraborty, Suman
2017.
Effect of interfacial slip on the deformation of a viscoelastic drop in uniaxial extensional flow field.
Physics of Fluids,
Vol. 29,
Issue. 3,
Mukherjee, Siddhartha
Goswami, Prakash
Dhar, Jayabrata
Dasgupta, Sunando
and
Chakraborty, Suman
2017.
Ion-size dependent electroosmosis of viscoelastic fluids in microfluidic channels with interfacial slip.
Physics of Fluids,
Vol. 29,
Issue. 7,
Bandopadhyay, Aditya
and
Ghosh, Uddipta
2018.
Electrohydrodynamic Phenomena.
Journal of the Indian Institute of Science,
Vol. 98,
Issue. 2,
p.
201.
Lei, Jie-Chao
Chang, Chien C.
and
Wang, Chang-Yi
2019.
Electro-osmotic pumping through a bumpy microtube: Boundary perturbation and detection of roughness.
Physics of Fluids,
Vol. 31,
Issue. 1,
Mahapatra, Bimalendu
and
Bandopadhyay, Aditya
2020.
Electroosmosis of a viscoelastic fluid over non-uniformly charged surfaces: Effect of fluid relaxation and retardation time.
Physics of Fluids,
Vol. 32,
Issue. 3,
Zhou, S.
2020.
Effective electrostatic forces between two neutral surfaces with atomic scale strip shape surface charge separation.
Journal of Molecular Liquids,
Vol. 312,
Issue. ,
p.
113272.
Mukherjee, Siddhartha
DasGupta, Sunando
and
Chakraborty, Suman
2020.
Temperature-gradient-induced massive augmentation of solute dispersion in viscoelastic micro-flows.
Journal of Fluid Mechanics,
Vol. 897,
Issue. ,
Choudhary, Akash
Li, Di
Renganathan, T.
Xuan, Xiangchun
and
Pushpavanam, S.
2020.
Electrokinetically enhanced cross-stream particle migration in viscoelastic flows.
Journal of Fluid Mechanics,
Vol. 898,
Issue. ,
Das, Pradipta Kr.
Snider, Arthur David
and
Bhethanabotla, Venkat R.
2020.
Acoustic streaming in second-order fluids.
Physics of Fluids,
Vol. 32,
Issue. 12,
Mahapatra, Bimalendu
and
Bandopadhyay, Aditya
2021.
Numerical analysis of combined electroosmotic-pressure driven flow of a viscoelastic fluid over high zeta potential modulated surfaces.
Physics of Fluids,
Vol. 33,
Issue. 1,
Ghosh, Uddipta
Mukherjee, Siddhartha
and
Chakraborty, Suman
2021.
Electrophoretic motion of a non-uniformly charged particle in a viscoelastic medium in thin electrical double layer limit.
Journal of Fluid Mechanics,
Vol. 924,
Issue. ,
Natu, Aditya
and
Ghosh, Uddipta
2021.
Electrokinetics of polymeric fluids in narrow rectangular confinements.
Soft Matter,
Vol. 17,
Issue. 38,
p.
8712.
Hegde, Amit Sanjay
and
Harikrishnan, A.R.
2021.
Slip hydrodynamics of combined electroosmotic and pressure driven flows of power law fluids through narrow confinements.
European Journal of Mechanics - B/Fluids,
Vol. 89,
Issue. ,
p.
525.
Mahapatra, Bimalendu
and
Bandopadhyay, Aditya
2021.
Microconfined electroosmotic flow of a complex fluid with asymmetric charges: Interplay of fluid rheology and physicochemical heterogeneity.
Journal of Non-Newtonian Fluid Mechanics,
Vol. 289,
Issue. ,
p.
104479.
Dewangan, Mainendra Kumar
Ghosh, Uddipta
Le Borgne, Tanguy
and
Méheust, Yves
2022.
Coupled electrohydrodynamic transport in rough fractures: a generalized lubrication theory.
Journal of Fluid Mechanics,
Vol. 942,
Issue. ,
Kumar, Vishal
Mukherjee, Joydeb
Sinha, Sudipta Kumar
and
Ghosh, Uddipta
2022.
Combined electromechanically driven pulsating flow of nonlinear viscoelastic fluids in narrow confinements.
Journal of The Royal Society Interface,
Vol. 19,
Issue. 189,
Xiao, Yang
Cao, Baofeng
Shi, Jinquan
Wu, Huanran
He, Xiang
Zhao, Chang
Chu, Jian
and
Liu, Hanlong
2023.
State-of-the-art review on the application of microfluidics in biogeotechnology.
Transportation Geotechnics,
Vol. 41,
Issue. ,
p.
101030.
Lobasov, Alexander S.
Rudyak, Valery Ya.
and
Minakov, Andrey V.
2024.
Investigation of the slip boundary conditions effect on the flow regimes, mixing efficiency and heat transfer in a T-type micromixer.
Chemical Engineering and Processing - Process Intensification,
Vol. 198,
Issue. ,
p.
109726.
Borthakur, Rajnandan
and
Ghosh, Uddipta
2024.
Electrophoretic maneuvering of nonuniformly charged particles suspended in linear flows: Impact of the medium viscoelasticity.
Physical Review Fluids,
Vol. 9,
Issue. 2,
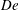 $De$) as the gauge function. Modified slip velocities correct up to
$De$) as the gauge function. Modified slip velocities correct up to 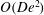 $O(De^{2})$ are presented. Two sample cases are considered to demonstrate the effects of viscoelasticity on slip velocity: (i) an axially periodic patterned potential and (ii) a step-change-like variation in the surface potential. The central result of our analysis is that, unlike Newtonian fluids, the electroosmotic slip velocity for second-order fluids does not, in general, align with the direction of the applied external electric field. Proceeding further forward, we show that the slip velocity in a given direction may, in fact, depend on the applied electric field strength in a mutually orthogonal direction, considering three dimensionality of the flow structure. In addition, we demonstrate that the modified slip velocity is not proportional to the zeta potential, as in the cases of Newtonian fluids; rather it depends strongly on the gradients of the interfacial potential as well. Our results are likely to have potential implications so far as the design of charge modulated microfluidic devices transporting rheologically complex fluids is concerned, such as for mixing and bio-reactive system analysis in lab-on-a-chip-based micro-total-analysis systems handling bio-fluids.
$O(De^{2})$ are presented. Two sample cases are considered to demonstrate the effects of viscoelasticity on slip velocity: (i) an axially periodic patterned potential and (ii) a step-change-like variation in the surface potential. The central result of our analysis is that, unlike Newtonian fluids, the electroosmotic slip velocity for second-order fluids does not, in general, align with the direction of the applied external electric field. Proceeding further forward, we show that the slip velocity in a given direction may, in fact, depend on the applied electric field strength in a mutually orthogonal direction, considering three dimensionality of the flow structure. In addition, we demonstrate that the modified slip velocity is not proportional to the zeta potential, as in the cases of Newtonian fluids; rather it depends strongly on the gradients of the interfacial potential as well. Our results are likely to have potential implications so far as the design of charge modulated microfluidic devices transporting rheologically complex fluids is concerned, such as for mixing and bio-reactive system analysis in lab-on-a-chip-based micro-total-analysis systems handling bio-fluids.