Hostname: page-component-586b7cd67f-tf8b9 Total loading time: 0 Render date: 2024-11-24T09:44:01.489Z Has data issue: false hasContentIssue false
  • English
  • Français

Effects of kinematic and magnetic boundary conditions on the dynamics of convection-driven plane layer dynamos

Published online by Cambridge University Press:  02 November 2022

Souvik Naskar Open the ORCID record for Souvik Naskar [Opens in a new window]  and
Anikesh Pal Open the ORCID record for Anikesh Pal [Opens in a new window]
Souvik Naskar
Affiliation:
Department of Mechanical Engineering, Indian Institute of Technology, Kanpur 208016, India
Anikesh Pal*
Affiliation:
Department of Mechanical Engineering, Indian Institute of Technology, Kanpur 208016, India
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

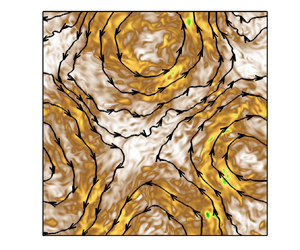
Rapidly rotating convection-driven dynamos are investigated under different kinematic and magnetic boundary conditions using direct numerical simulations. At a fixed rotation rate, represented by the Ekman number $Ek=5\times 10^{-7}$, the thermal forcing is varied from $2$ to $20$ times its value at the onset of convection ($\mathcal {R}=Ra/Ra_c=2\unicode{x2013}20$, where $Ra$ is the Rayleigh number), keeping the fluid properties constant ($Pr=Pr_m=1$, where $Pr$ and $Pr_m$ are the thermal and magnetic Prandtl numbers). The statistical behaviour of the dynamos, including the force balance, energetics, and heat transport, depends on the boundary conditions that dictate both the boundary layer and the interior dynamics. At a fixed thermal forcing ($\mathcal {R}=3$), the structure and strength of the magnetic field produced by the dynamos, especially near the walls, depend on both velocity and magnetic boundary conditions. Though the leading-order force balance in the bulk remains geostrophic, the Lorentz force becomes comparable to the Coriolis force inside the thermal boundary layer with no-slip, perfectly conducting conditions. In this case, a term signifying the work done by the Lorentz force in the turbulent kinetic energy (TKE) equation is found to have some components that extract energy from the velocity field to produce the magnetic field, while some other components extract energy from the magnetic field to produce TKE. However, with no-slip, pseudo-vacuum conditions, all the components of the work done by the Lorentz force perform unidirectional energy transfer to produce magnetic energy from the kinetic energy of the fluid to sustain dynamo action. We find heat transfer enhancement in the rotating dynamo convection, as compared with non-magnetic rotating convection, with the peak enhancement lying in the range $\mathcal {R}=3\unicode{x2013}4$. For free-slip conditions, in the absence of an Ekman layer, the dynamo action may alter the heat transport significantly by suppressing the formation of large-scale vortices. However, the highest heat transfer enhancement is found at $\mathcal {R}=3$ with no-slip, perfectly conducting walls, which can be attributed to a local magnetorelaxation of the rotational constraint due to enhanced Lorentz force inside the thermal boundary layer.

JFM classification

MHD and Electrohydrodynamics: MHD turbulence MHD and Electrohydrodynamics: Magneto convection Turbulent Flows: Rotating turbulence
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 951 , 25 November 2022 , A7
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Aurnou, J.M., Bertin, V., Grannan, A.M., Horn, S. & Vogt, T. 2018 Rotating thermal convection in liquid gallium: multi-modal flow, absent steady columns. J. Fluid Mech. 846, 846–876.CrossRefGoogle Scholar
Aurnou, J.M., Horn, S. & Julien, K. 2020 Connections between nonrotating, slowly rotating, and rapidly rotating turbulent convection transport scalings. Phys. Rev. Res. 2 (4), 043115.CrossRefGoogle Scholar
Aurnou, J.M. & King, E.M. 2017 The cross-over to magnetostrophic convection in planetary dynamo systems. Proc. R. Soc. Lond. A 473 (2199), 20160731.Google ScholarPubMed
Brucker, K.A. & Sarkar, S. 2010 A comparative study of self-propelled and towed wakes in a stratified fluid. J. Fluid Mech. 652, 373–404.CrossRefGoogle Scholar
Calkins, M.A. 2018 Quasi-geostrophic dynamo theory. Phys. Earth Planet. Inter. 276, 182–189.CrossRefGoogle Scholar
Calkins, M.A., Julien, K., Tobias, S.M. & Aurnou, J.M. 2015 A multiscale dynamo model driven by quasi-geostrophic convection. J. Fluid Mech. 780, 143–166.CrossRefGoogle Scholar
Cattaneo, F. & Hughes, D.W. 2006 Dynamo action in a rotating convective layer. J. Fluid Mech. 553, 401–418.CrossRefGoogle Scholar
Chandrasekhar, S. 1961 Hydrodynamic and Hydromagnetic Stability. Courier Corporation.Google Scholar
Cheng, J.S., Madonia, M., Guzmán, A.J.A. & Kunnen, R.P.J. 2020 Laboratory exploration of heat transfer regimes in rapidly rotating turbulent convection. Phys. Rev. Fluids 5 (11), 113501.CrossRefGoogle Scholar
Cheng, J.S., Stellmach, S., Ribeiro, A., Grannan, A., King, E.M. & Aurnou, J.M. 2015 Laboratory-numerical models of rapidly rotating convection in planetary cores. Geophys. J. Intl 201 (1), 1–17.CrossRefGoogle Scholar
Childress, S. & Soward, A.M. 1972 Convection-driven hydromagnetic dynamo. Phys. Rev. Lett. 29 (13), 837.CrossRefGoogle Scholar
Cnossen, I. 2014 The importance of geomagnetic field changes versus rising $\textrm {CO}_{2}$ levels for long-term change in the upper atmosphere. J. Space Weather Space Clim. 4, A18.CrossRefGoogle Scholar
Ecke, R.E. & Niemela, J.J. 2014 Heat transport in the geostrophic regime of rotating Rayleigh–Bénard convection. Phys. Rev. Lett. 113 (11), 114301.CrossRefGoogle ScholarPubMed
Erdmann, W., Kmita, H., Kosicki, J.Z. & Kaczmarek, Ł. 2021 How the geomagnetic field influences life on Earth – an integrated approach to geomagnetobiology. Orig. Life Evol. Biosph. 51, 231–257.CrossRefGoogle ScholarPubMed
Fautrelle, Y. & Childress, S. 1982 Convective dynamos with intermediate and strong fields. Geophys. Astrophys. Fluid Dyn. 22 (3–4), 235–279.CrossRefGoogle Scholar
Favier, B., Silvers, L.J. & Proctor, M.R.E. 2014 Inverse cascade and symmetry breaking in rapidly rotating Boussinesq convection. Phys. Fluids 26 (9), 096605.CrossRefGoogle Scholar
Guervilly, C., Hughes, D.W. & Jones, C.A. 2014 Large-scale vortices in rapidly rotating Rayleigh–Bénard convection. J. Fluid Mech. 758, 407–435.CrossRefGoogle Scholar
Guervilly, C., Hughes, D.W. & Jones, C.A. 2015 Generation of magnetic fields by large-scale vortices in rotating convection. Phys. Rev. E 91 (4), 041001.CrossRefGoogle ScholarPubMed
Guervilly, C., Hughes, D.W. & Jones, C.A. 2017 Large-scale-vortex dynamos in planar rotating convection. J. Fluid Mech. 815, 333–360.CrossRefGoogle Scholar
Guzmán, A.J.A., Madonia, M., Cheng, J.S., Ostilla-Mónico, R., Clercx, H.J.H. & Kunnen, R.P.J. 2020 Competition between Ekman plumes and vortex condensates in rapidly rotating thermal convection. Phys. Rev. Lett. 125 (21), 214501.CrossRefGoogle Scholar
Guzmán, A.J.A., Madonia, M., Cheng, J.S., Ostilla-Mónico, R., Clercx, H.J.H. & Kunnen, R.P.J. 2021 Force balance in rapidly rotating Rayleigh–Bénard convection. J. Fluid Mech. 928, A16.CrossRefGoogle ScholarPubMed
Iyer, K.P., Scheel, J.D., Schumacher, J. & Sreenivasan, K.R. 2020 Classical $1/3$ scaling of convection holds up to ${Ra}= 10^{15}$. Proc. Natl Acad. Sci. USA 117 (14), 7594–7598.CrossRefGoogle Scholar
Jones, C.A. & Roberts, P.H. 2000 Convection-driven dynamos in a rotating plane layer. J. Fluid Mech. 404, 311–343.CrossRefGoogle Scholar
Julien, K., Aurnou, J.M., Calkins, M.A., Knobloch, E., Marti, P., Stellmach, S. & Vasil, G.M. 2016 A nonlinear model for rotationally constrained convection with Ekman pumping. J. Fluid Mech. 798, 50–87.CrossRefGoogle Scholar
Julien, K., Knobloch, E., Rubio, A.M. & Vasil, G.M. 2012 a Heat transport in low-Rossby-number Rayleigh–Bénard convection. Phys. Rev. Lett. 109 (25), 254503.CrossRefGoogle ScholarPubMed
Julien, K., Legg, S., McWilliams, J. & Werne, J. 1996 Rapidly rotating turbulent Rayleigh–Bénard convection. J. Fluid Mech. 322, 243–273.CrossRefGoogle Scholar
Julien, K., Rubio, A.M., Grooms, I. & Knobloch, E. 2012 b Statistical and physical balances in low Rossby number Rayleigh–Bénard convection. Geophys. Astrophys. Fluid Dyn. 106 (4–5), 392–428.CrossRefGoogle Scholar
Kerr, R.M. 2001 Energy budget in Rayleigh–Bénard convection. Phys. Rev. Lett. 87, 244502.CrossRefGoogle ScholarPubMed
King, E.M. & Aurnou, J.M. 2013 Turbulent convection in liquid metal with and without rotation. Proc. Natl Acad. Sci. USA 110 (17), 6688–6693.CrossRefGoogle ScholarPubMed
King, E.M., Stellmach, S. & Aurnou, J.M. 2012 Heat transfer by rapidly rotating Rayleigh–Bénard convection. J. Fluid Mech. 691, 568–582.CrossRefGoogle Scholar
King, E.M., Stellmach, S., Noir, J., Hansen, U. & Aurnou, J.M. 2009 Boundary layer control of rotating convection systems. Nature 457 (7227), 301–304.CrossRefGoogle ScholarPubMed
Kolhey, P., Stellmach, S. & Heyner, D. 2022 Influence of boundary conditions on rapidly rotating convection and its dynamo action in a plane fluid layer. Phys. Rev. Fluids 7 (4), 043502.CrossRefGoogle Scholar
Kunnen, R.P.J. 2021 The geostrophic regime of rapidly rotating turbulent convection. J. Turbul. 22 (4–5), 267–296.CrossRefGoogle Scholar
Kunnen, R.P.J., Geurts, B.J. & Clercx, H.J.H. 2009 Turbulence statistics and energy budget in rotating Rayleigh–Bénard convection. Eur. J. Mech. (B/Fluids) 28 (4), 578–589.CrossRefGoogle Scholar
Kunnen, R.P.J., Geurts, B.J. & Clercx, H.J.H. 2010 Experimental and numerical investigation of turbulent convection in a rotating cylinder. J. Fluid Mech. 642, 445–476.CrossRefGoogle Scholar
Kunnen, R.P.J., Ostilla-Mónico, R., Van Der Poel, E.P., Verzicco, R. & Lohse, D. 2016 Transition to geostrophic convection: the role of the boundary conditions. J. Fluid Mech. 799, 413–432.CrossRefGoogle Scholar
Maffei, S., Calkins, M.A., Julien, K. & Marti, P. 2019 Magnetic quenching of the inverse cascade in rapidly rotating convective turbulence. Phys. Rev. Fluids 4 (4), 041801.CrossRefGoogle Scholar
Maffei, S., Krouss, M.J., Julien, K. & Calkins, M.A. 2021 On the inverse cascade and flow speed scaling behaviour in rapidly rotating Rayleigh–Bénard convection. J. Fluid Mech. 913, A18.CrossRefGoogle Scholar
Meneguzzi, M. & Pouquet, A. 1989 Turbulent dynamos driven by convection. J. Fluid Mech. 205, 297–318.CrossRefGoogle Scholar
Moffatt, K. & Dormy, E. 2019 Self-Exciting Fluid Dynamos. Cambridge University Press.CrossRefGoogle Scholar
Naskar, S. & Pal, A. 2022 Direct numerical simulations of optimal thermal convection in rotating plane layer dynamos. J. Fluid Mech. 942, A37.CrossRefGoogle Scholar
Nieves, D., Rubio, A.M. & Julien, K. 2014 Statistical classification of flow morphology in rapidly rotating Rayleigh–Bénard convection. Phys. Fluids 26 (8), 086602.CrossRefGoogle Scholar
Pal, A. 2020 Deep learning emulation of subgrid-scale processes in turbulent shear flows. Geophys. Res. Lett. 47 (12), e2020GL087005.CrossRefGoogle Scholar
Pal, A. & Chalamalla, V.K. 2020 Evolution of plumes and turbulent dynamics in deep-ocean convection. J. Fluid Mech. 889, A35.CrossRefGoogle Scholar
Pal, A., de Stadler, M.B. & Sarkar, S. 2013 The spatial evolution of fluctuations in a self-propelled wake compared to a patch of turbulence. Phys. Fluids 25, 095106.CrossRefGoogle Scholar
Pal, A. & Sarkar, S. 2015 Effect of external turbulence on the evolution of a wake in stratified and unstratified environments. J. Fluid Mech. 772, 361–385.CrossRefGoogle Scholar
Plumley, M. & Julien, K. 2019 Scaling laws in Rayleigh–Bénard convection. Earth Space Sci. 6 (9), 1580–1592.CrossRefGoogle Scholar
Plumley, M., Julien, K., Marti, P. & Stellmach, S. 2016 The effects of Ekman pumping on quasi-geostrophic Rayleigh–Bénard convection. J. Fluid Mech. 803, 51–71.CrossRefGoogle Scholar
Plumley, M., Julien, K., Marti, P. & Stellmach, S. 2017 Sensitivity of rapidly rotating Rayleigh–Bénard convection to Ekman pumping. Phys. Rev. Fluids 2 (9), 094801.CrossRefGoogle Scholar
Rüdiger, G. & Hollerbach, R. 2006 The Magnetic Universe: Geophysical and Astrophysical Dynamo Theory. John Wiley & Sons.Google Scholar
Rubio, A.M., Julien, K., Knobloch, E. & Weiss, J.B. 2014 Upscale energy transfer in three-dimensional rapidly rotating turbulent convection. Phys. Rev. Lett. 112 (14), 144501.CrossRefGoogle ScholarPubMed
Schmitz, S. & Tilgner, A. 2010 Transitions in turbulent rotating Rayleigh–Bénard convection. Geophys. Astrophys. Fluid Dyn. 104 (5–6), 481–489.CrossRefGoogle Scholar
Schwaiger, T., Gastine, T. & Aubert, J. 2019 Force balance in numerical geodynamo simulations: a systematic study. Geophys. J. Int. 219 (Supplement_1), S101–S114.CrossRefGoogle Scholar
Soward, A.M. 1974 A convection-driven dynamo. I. The weak field case. Phil. Trans. R. Soc. Lond. A 275 (1256), 611–646.Google Scholar
St Pierre, M.G. 1993 The strong field branch of the Childress–Soward dynamo. In Solar and Planetary Dynamos (ed. M.R.E. Proctor & A.D. Gilbert), pp. 245–277. Cambridge University Press.CrossRefGoogle Scholar
Stellmach, S. & Hansen, U. 2004 Cartesian convection driven dynamos at low Ekman number. Phys. Rev. E 70 (5), 056312.CrossRefGoogle ScholarPubMed
Stellmach, S., Lischper, M., Julien, K., Vasil, G., Cheng, J.S., Ribeiro, A., King, E.M. & Aurnou, J.M. 2014 Approaching the asymptotic regime of rapidly rotating convection: boundary layers versus interior dynamics. Phys. Rev. Lett. 113 (25), 254501.CrossRefGoogle ScholarPubMed
Tarduno, J.A. 2018 Subterranean clues to the future of our planetary magnetic shield. Proc. Natl Acad. Sci. USA 115 (52), 13154–13156.CrossRefGoogle Scholar
Thelen, J.-C. & Cattaneo, F. 2000 Dynamo action driven by convection: the influence of magnetic boundary conditions. Mon. Not. R. Astron. Soc. 315 (2), L13–L17.CrossRefGoogle Scholar
Tilgner, A. 2012 Transitions in rapidly rotating convection driven dynamos. Phys. Rev. Lett. 109 (24), 248501.CrossRefGoogle ScholarPubMed
Tilgner, A. 2014 Magnetic energy dissipation and mean magnetic field generation in planar convection-driven dynamos. Phys. Rev. E 90 (1), 013004.CrossRefGoogle ScholarPubMed
Tobias, S.M. 2021 The turbulent dynamo. J. Fluid Mech. 912, P1.CrossRefGoogle ScholarPubMed
Tobias, S.M., Cattaneo, F. & Boldyrev, S. 2012 MHD dynamos and turbulence. In Ten Chapters in Turbulence (ed. P.A. Davidson, Y. Kaneda & K.R. Sreenivasan), pp. 351–404. Cambridge University Press.CrossRefGoogle Scholar
Weiss, S., Stevens, R.J.A.M., Zhong, J.-Q., Clercx, H.J.H., Lohse, D. & Ahlers, G. 2010 Finite-size effects lead to supercritical bifurcations in turbulent rotating Rayleigh–Bénard convection. Phys. Rev. Lett. 105 (22), 224501.CrossRefGoogle ScholarPubMed
Yan, M. & Calkins, M.A. 2022 a Asymptotic behaviour of rotating convection-driven dynamos in the plane layer geometry. J. Fluid Mech. arXiv:2202.01382.Google Scholar
Yan, M. & Calkins, M.A. 2022 b Strong large scale magnetic fields in rotating convection-driven dynamos: the important role of magnetic diffusion. Phys. Rev. Res. 4 (1), L012026.CrossRefGoogle Scholar
Yan, M., Tobias, S.M. & Calkins, M.A. 2021 Scaling behaviour of small-scale dynamos driven by Rayleigh–Bénard convection. J. Fluid Mech. 915, A15.CrossRefGoogle Scholar

Naskar and Pal Supplementary Movie

See "Naskar and Pal Supplementary Movie Caption"

Download Naskar and Pal Supplementary Movie(Video)
Video 14.8 MB
Supplementary material: PDF

Naskar and Pal Supplementary Movie Caption

Naskar and Pal Supplementary Movie Caption

Download Naskar and Pal Supplementary Movie Caption(PDF)
PDF 46.3 KB