Hostname: page-component-cd9895bd7-gxg78 Total loading time: 0 Render date: 2024-12-22T23:54:08.907Z Has data issue: false hasContentIssue false
  • English
  • Français

Effects of inertia and turbulence on rheological measurements of neutrally buoyant suspensions

Published online by Cambridge University Press:  13 December 2016

Esperanza Linares-Guerrero ,
Melany L. Hunt  and
Roberto Zenit
Esperanza Linares-Guerrero
Affiliation:
Division of Engineering and Applied Science, California Institute of Technology, Pasadena, CA 91125, USA
Melany L. Hunt*
Affiliation:
Division of Engineering and Applied Science, California Institute of Technology, Pasadena, CA 91125, USA
Roberto Zenit
Affiliation:
Instituto de Investigaciones en Materiales, Universidad Nacional Autónoma de México, Apdo. Postal 70-360, México D.F. 04510, México
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

For low-Reynolds-number shear flows of neutrally buoyant suspensions, the shear stress is often modelled using an effective viscosity that depends only on the solid fraction. As the Reynolds number ($Re$) is increased and inertia becomes important, the effective viscosity also depends on the Reynolds number itself. The current experiments measure the torque for flows of neutrally buoyant particles in a coaxial-cylinder rheometer for solid fractions, 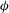$\unicode[STIX]{x1D719}$, from 10 % to 50 % and Reynolds numbers based on particle diameter from 2 to 1000. For experiments for Reynolds of 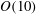$O(10)$ and solid fractions less than 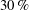$30\,\%$, the effective viscosity increases with Reynolds number, in good agreement with recent numerical simulations found in the literature. At higher solid fractions over the same range of $Re$, the results show a decrease in torque with shear rate. For Reynolds numbers greater than 100 and lower solids concentrations, the effective viscosity continues to increase with Reynolds number. However, based on comparisons with pure fluid measurements the increase in the measured effective viscosity results from the transition to turbulence. The particles augment the turbulence by increasing the magnitude of the measured torques and causing the flow to transition at lower Reynolds numbers. For the highest solid fractions, the measurements show a significant increase in the magnitude of the torques, but the effective viscosity is independent of Reynolds number.

JFM classification

Complex Fluids: Suspensions Instability: Transition to turbulence
Type
Papers
Information
Journal of Fluid Mechanics , Volume 811 , 25 January 2017 , pp. 525 - 543
Check for updates
Copyright
© 2016 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Acrivos, A., Fan, X. & Mauri, R. 1994 On the measurements of the relative viscosity of suspensions. J. Rheol. 38 (5), 1285–1296.Google Scholar
Andereck, D., Liu, S. S. & Swinney, H. L. 1986 Flow regimes in a circular couette system with independently rotating cylinders. J. Fluid Mech. 164, 155–183.Google Scholar
Audus, D. J., Hassan, A. M., Garboczi, E. J. & Douglas, J. F. 2015 Interplay of particle shape and suspension properties: a study of cube like particles. Soft Matt. 11, 3360–3366.Google Scholar
Bagnold, R. A. 1954 Experiments on a gravity-free dispersion of large solid spheres in a Newtonian fluid under shear. Proc. R. Soc. Lond. A 225 (1160), 49–53.Google Scholar
Batchelor, G. K. 1970 The stress system in a suspension of force-free particles. J. Fluid Mech. 41, 545–570.CrossRefGoogle Scholar
van den Berg, T. H., Doering, C. R., Lohse, D. & Lathrop, D. 2003 Smooth and rough boundaries in turbulent Taylor–Couette flow. Phys. Rev. E 68, 036307.Google Scholar
Breedveld, V., Ende, D., Van Den Tripathi, A. & Acrivos, A. 1998 The measurement of the shear-induced particle and fluid tracer diffusivities in concentrated suspensions by a novel method. J. Fluid Mech. 375, 297–318.CrossRefGoogle Scholar
Breugem, W. P. 2012 A second-order accurate immersed boundary method for fully resolved simulations of particle-laden flows. J. Comput. Phys. 231 (13), 4469–4498.Google Scholar
Brown, E., Forman, N. A., Orellana, C. S., Zhang, H., Maynor, B. W., Betts, D. E., Desimone, J. M. & Jaeger, H. M. 2010 Generality of shear thickening in dense suspensions. Nat. Mater. 9, 220–224.Google Scholar
Cadot, O., Couder, Y., Daerr, A., Douady, S. & Tsinober, A. 1997 Energy injection in closed turbulent flows: stirring through boundary layers versus inerial stirring. Phys. Rev. E 56, 427–433.Google Scholar
Cartellier, A. & Riviere, N. 2001 Bubble-induced agitation and microstructure in uniform bubbly flows at small to moderate particle Reynolds numbers. Phys. Fluids 13, 2165–2181.Google Scholar
Coles, D. 1965 Transition in circular Couette flow. J. Fluid Mech. 21, 385–424.Google Scholar
Dijksmann, J. A., Wandersmann, E., Slotterback, S., Berardi, C. R., Updegraff, W. D., van Hecke, M. & Losert, W. 2010 From frictional to viscous behavior: three-dimensional imaging and rheology of gravitational suspensions. Phys. Rev. E 82, 60301.Google Scholar
Fall, A., Bertrand, F., Ovarlez, G. & Bonn, D. 2009 Yield stress and shear banding in granular suspensions. Phys. Rev. Lett. 103, 178301.CrossRefGoogle ScholarPubMed
Foss, D. R. & Brady, J. F. 2000 Structure, diffusion and rheology of Brownian suspensions by stokesian dynamics simulation. J. Fluid Mech. 407, 167–200.Google Scholar
Gore, R. A. & Crowe, C. T. 1989 Effect of particle size on modulating turbulent intensity. Intl J. Multiphase Flow 15 (2), 279–285.Google Scholar
Hanes, D. M. & Inman, D. L. 1985 Observations of rapidly flowing granular-fluid materials. J. Fluid Mech. 150, 357–380.CrossRefGoogle Scholar
Hunt, M. L., Zenit, R., Campbell, C. S. & Brennen, C. E. 2002 Revisiting the 1954 suspension experiments of R. A. Bagnold. J. Fluid Mech. 452, 1–24.CrossRefGoogle Scholar
Joseph, G. G., Zenit, R., Hunt, M. L. & Rosenwinkel, A. M. 2001 Particle-wall collisions in a viscous fluid. J. Fluid Mech. 433, 329–346.Google Scholar
Koos, E., Linares-Guerrero, E., Hunt, M. L. & Brennen, C. E. 2012 Rheological measurements of large particles in high shear rate flows. Phys. Fluids 24, 013302.Google Scholar
Kulkarni, P. M. & Morris, J. F. 2008 Suspension properties at finite Reynolds number from simulated shear flow. Phys. Fluids 20, 40602.Google Scholar
Larson, R. G. 1998 Structure and Rheology of Complex Fluids. Oxford University Press.Google Scholar
Lee, S. H., Chung, H. T., Park, C. W. & Kim, H. B. 2009 Experimental investigation of the effect of axial wall slits on Taylor–Couette flow. Fluid Dyn. Res. 41, 045502.Google Scholar
Mallavajula, R. K., Kock, D. L. & Archer, L. A. 2013 Intrinsic viscosity of a suspension of cubes. Phys. Rev. E 88, 052302.Google Scholar
Martinez-Mercado, J., Palacios-Morales, C. A. & Zenit, R. 2007 Measurement of pseudoturbulence intensity in monodispersed bubbly liquids for 10 < Re < 500. Phys. Fluids 19, 103302.Google Scholar
Matas, J. P., Morris, J. F. & Guazzelli, É. 2003 Transition to turbulence in particulate pipe flow. Phys. Rev. Lett. 90 (1), 4501–4504.CrossRefGoogle ScholarPubMed
Mendez-Diaz, S., Serrano-Garcia, J. C., Zenit, R. & Hernandez-Cordero, J. A. 2013 Power spectral distributions of pseudo-turbulent bubbly flows. Phys. Fluids 25, 043303.Google Scholar
Picano, F., Breugem, W.-P. & Brandt, L. 2015 Turbulent channel flow of dense suspensions of neutrally buoyant spheres. J. Fluid Mech. 764, 463–487.Google Scholar
Picano, F., Breugem, W. P., Mitra, D. & Brandt, L. 2013 Shear thickening in non-Newtonian suspensions: an excluded volume effect. Phys. Rev. Lett. 111, 098302.CrossRefGoogle Scholar
Prasad, D. & Kytömaa, H. K. 1995 Particle stress and viscous compaction during shear of dense suspensions. Intl J. Multiphase Flow 21 (5), 775–785.Google Scholar
Ravelet, F., Delfos, R. & Westerweel, J. 2010 Influence of global rotation and reynolds number on the large-scale features of a turbulent Taylor–Couette flow. Phys. Fluids 22, 055103.CrossRefGoogle Scholar
Risso, F. 2011 Theoretical model for k3 spectra in dispersed multiphase flows. Phys. Fluids 23, 011701.Google Scholar
Savage, S. B. & Mckeown, S. 1983 Shear stresses developed during rapid shear of concentrated suspensions of large spherical particles between concentric cylinders. J. Fluid Mech. 127, 453–472.Google Scholar
Stickel, J. J. & Powell, R. L. 2005 Fluid mechanics and rheology of dense suspensions. Annu. Rev. Fluid Mech. 37 (1), 129–149.Google Scholar
Swinney, H. L. & Gollub, J. P. 1978 The transition to turbulence. Phys. Today 31 (8), 41–49.Google Scholar
Tanaka, T. & Eaton, J. K. 2008 Classification of turbulence modification by dispersed spheres using a novel dimensionless number. Phys. Rev. Lett. 101, 114502.Google Scholar
Taylor, G. I. 1936a Fluid friction between rotating cylinders, I. Torque measurements. Proc. R. Soc. Lond. A 157 (892), 546–564.Google Scholar
Taylor, G. I. 1936b Fluid friction between rotating cylinders, II. Distribution of velocity between concentric cylinders when outer one is rotating and inner one is at rest. Proc. R. Soc. Lond. A 157 (892), 565–578.Google Scholar
Trulsson, M., Andreotti, B. & Claudin, P. 2012 Transition from the viscous to inertial regime in dense suspensions. Phys. Rev. Lett. 109, 118305.CrossRefGoogle ScholarPubMed
VanAtta, C. 1966 Exploratory measurements in spiral turbulence. J. Fluid Mech. 25, 495–512.Google Scholar
Verberg, R. & Koch, D. 2006 Rheology of particle suspensions with low to moderate fluid inertia at finite particle inertia. Phys. Fluids 18, 083303.Google Scholar
Wendt, F. 1933 Turbulente strömungen zwischen zwei rotierenden konaxialen zylindern. Ing.-Arch. 4, 577–595.Google Scholar
Yang, F. L. & Hunt, M. L. 2006 Dynamics of particle-particle collisions in a viscous liquid. Phys. Fluids 18, 121506.Google Scholar
Yeo, K. & Maxey, M. R. 2013 Dynamics and rheology of concentrated, finite-Reynolds-number suspensions in a homogeneous shear flow. Phys. Fluids 25, 533303.Google Scholar