Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Wang, Guiquan
Park, Hyungwon John
and
Richter, David H.
2020.
Effect of computational domain size on inertial particle one-point statistics in open channel flow.
International Journal of Multiphase Flow,
Vol. 125,
Issue. ,
p.
103195.
Zhou, Tian
Zhao, Lihao
Huang, Weixi
and
Xu, Chunxiao
2020.
Non-monotonic effect of mass loading on turbulence modulations in particle-laden channel flow.
Physics of Fluids,
Vol. 32,
Issue. 4,
Kim, Junhyuk
and
Lee, Changhoon
2020.
Deep unsupervised learning of turbulence for inflow generation at various Reynolds numbers.
Journal of Computational Physics,
Vol. 406,
Issue. ,
p.
109216.
Lee, Seulgi
and
Lee, Changhoon
2020.
Behavior of settling particles in homogeneous shear turbulence.
Physical Review Fluids,
Vol. 5,
Issue. 10,
Baker, Lucia J.
and
Coletti, Filippo
2021.
Particle–fluid–wall interaction of inertial spherical particles in a turbulent boundary layer.
Journal of Fluid Mechanics,
Vol. 908,
Issue. ,
Zhu, Hangyu
Pan, Chong
Wang, Guohua
Liang, Yirui
Ji, Xiaocang
and
Wang, Jinjun
2021.
Attached eddy-like particle clustering in a turbulent boundary layer under net sedimentation conditions.
Journal of Fluid Mechanics,
Vol. 920,
Issue. ,
Wang, Ping
Li, Jinchi
and
Zheng, Xiaojing
2021.
The effect of gravity on turbulence modulation in particle-laden horizontal open channel flow.
Physics of Fluids,
Vol. 33,
Issue. 8,
Shim, Hyoeun
and
Lee, Changhoon
2021.
Two-way interaction between isotropic turbulence and dispersed bubbles.
Journal of Mechanical Science and Technology,
Vol. 35,
Issue. 4,
p.
1527.
Wang, Ping
Wei, Qingqing
and
Zheng, Xiaojing
2021.
Differences of turbulence modulation by heavy particles on solid wall and erodible bed surface.
Physics of Fluids,
Vol. 33,
Issue. 11,
Bragg, A. D.
Richter, D. H.
and
Wang, G.
2021.
Mechanisms governing the settling velocities and spatial distributions of inertial particles in wall-bounded turbulence.
Physical Review Fluids,
Vol. 6,
Issue. 6,
Zheng, Xiaojing
Feng, Shengjun
and
Wang, Ping
2021.
Modulation of turbulence by saltating particles on erodible bed surface.
Journal of Fluid Mechanics,
Vol. 918,
Issue. ,
Wang, Yixiang
Lam, Kit Ming
and
Tse, Kam Tim
2021.
Direct numerical simulation of bidisperse inertial particles settling in turbulent channel flow.
Physics of Fluids,
Vol. 33,
Issue. 2,
Bragg, A. D.
Richter, D. H.
and
Wang, G.
2021.
Settling strongly modifies particle concentrations in wall-bounded turbulent flows even when the settling parameter is asymptotically small.
Physical Review Fluids,
Vol. 6,
Issue. 12,
Liu, Hongyou
He, Xibo
and
Zheng, Xiaojing
2021.
An investigation of particles effects on wall-normal velocity fluctuations in sand-laden atmospheric surface layer flows.
Physics of Fluids,
Vol. 33,
Issue. 10,
Chen, Pinzhuo
Chen, Sheng
Wu, Tianyi
Ruan, Xuan
and
Li, Shuiqing
2022.
Deposition velocity of inertial particles driven by wall-normal external force in turbulent channel flow.
Physical Review Fluids,
Vol. 7,
Issue. 10,
ur Rehman, Sajjad
Lee, Junghoon
and
Lee, Changhoon
2022.
Effect of Weissenberg number on polymer-laden turbulence.
Physical Review Fluids,
Vol. 7,
Issue. 6,
Brandt, Luca
and
Coletti, Filippo
2022.
Particle-Laden Turbulence: Progress and Perspectives.
Annual Review of Fluid Mechanics,
Vol. 54,
Issue. 1,
p.
159.
Jaiswal, Atul
Bui, Minh Duc
and
Rutschmann, Peter
2022.
Evaluation of RANS-DEM and LES-DEM Methods in OpenFOAM for Simulation of Particle-Laden Turbulent Flows.
Fluids,
Vol. 7,
Issue. 10,
p.
337.
Peng, Cheng
and
Wang, Lian-Ping
2023.
Mechanisms and models of particle drag enhancements in turbulent environments.
Journal of Fluid Mechanics,
Vol. 959,
Issue. ,
Liu, Hongyou
He, Xibo
and
Zheng, Xiaojing
2023.
Amplitude modulation in particle-laden atmospheric surface layers.
Journal of Fluid Mechanics,
Vol. 957,
Issue. ,
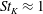 $St_{K}\approx 1$), the so-called preferential sweeping occurs in association with coherent streamwise vortices, while the effect of crossing trajectories becomes significant for
$St_{K}\approx 1$), the so-called preferential sweeping occurs in association with coherent streamwise vortices, while the effect of crossing trajectories becomes significant for 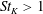 $St_{K}>1$. Consequently, in either case, the settling particles deposit on the wall without strong accumulation in low-speed streaks in the viscous sublayer. When particles settle through near-wall turbulence from the upper wall, more small-scale vortical structures are generated in the outer layer as low-speed fluid is pulled farther in the direction of gravity, while the opposite is true near the lower wall.
$St_{K}>1$. Consequently, in either case, the settling particles deposit on the wall without strong accumulation in low-speed streaks in the viscous sublayer. When particles settle through near-wall turbulence from the upper wall, more small-scale vortical structures are generated in the outer layer as low-speed fluid is pulled farther in the direction of gravity, while the opposite is true near the lower wall.


