Hostname: page-component-586b7cd67f-t7fkt Total loading time: 0 Render date: 2024-11-28T06:28:19.287Z Has data issue: false hasContentIssue false
  • English
  • Français

Effect of inclination angle on heat transport properties in two-dimensional Rayleigh–Bénard convection with smooth and rough boundaries

Published online by Cambridge University Press:  18 October 2022

Krishan Chand ,
Mukesh Sharma  and
Arnab Kr. De Open the ORCID record for Arnab Kr. De [Opens in a new window]
Krishan Chand
Affiliation:
Department of Mechanical Engineering, Indian Institute of Technology Guwahati, Guwahati, Assam 781039, India
Mukesh Sharma
Affiliation:
Department of Mechanical Engineering, Indian Institute of Technology Guwahati, Guwahati, Assam 781039, India
Arnab Kr. De*
Affiliation:
Department of Mechanical Engineering, Indian Institute of Technology Guwahati, Guwahati, Assam 781039, India
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

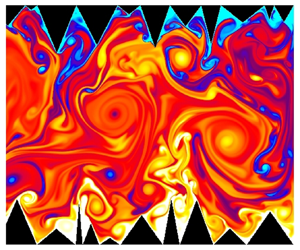
Using direct numerical simulations, two-dimensional tilted Rayleigh–Bénard convection (RBC) is studied in both smooth and roughness-facilitated convection cells of double aspect ratio ($\varGamma =2$) for air as a working fluid. We investigate the effect of inclination angle ($0^{\circ } \leq \phi \leq 90^{\circ }$) on heat flux ($Nu$), Reynolds number ($Re$) and flow structures. In a Rayleigh number range $10^{6}\leq Ra\leq 10^{9}$, we address the $Ra$ dependence of $Nu(\phi )$ trend. In the smooth case, while greater tilt results in highest heat flux below $Ra=10^{8}$, $Nu$ drops with $\phi$ monotonically above it (RBC transports heat most efficiently), which explains the different $Nu(\phi )$ trend observed in the previous studies due to $Ra$ dependence (Guo et al., J. Fluid Mech., vol. 762, 2015, pp. 273–287; Shishkina & Horn, J. Fluid Mech., vol. 790, 2016, R3; Khalilov et al., Phys. Rev. Fluids, vol. 3, 2018, 043503). For the smooth case, we identify the control parameters ($\phi =75^{\circ }$ and $Ra=10^{7}$) that yield maximum heat flux (an increment of $18\,\%$ with respect to the level case). On the other hand, among the three roughness set-ups used in the present study, the tallest roughness configuration yields the maximum increment in heat flux ($25\,\%$) in vertical convection ($\phi =90^{\circ }$) at $Ra=10^{6}$. With increase in $Ra$, $Re$ changes with $\phi$ marginally in the smooth case, whereas it shows notable changes in its roughness counterpart. We find that the weakening of thermal stratification is related directly to the height of roughness peaks. While $Ra$ delays the onset of thermal stratification (in terms of inclination angle) in the smooth case, an increase in roughness height plays the same role in roughness-facilitated convection cells.

JFM classification

Convection: Plumes/thermals Turbulent Flows: Turbulent convection
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 950 , 10 November 2022 , A16
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ahlers, G., Brown, E. & Nikolaenko, A. 2006 The search for slow transients, and the effect of imperfect vertical alignment, in turbulent Rayleigh–Bénard convection. J. Fluid Mech. 557, 347–367.CrossRefGoogle Scholar
Ahlers, G., Grossmann, S. & Lohse, D. 2009 Heat transfer and large scale dynamics in turbulent Rayleigh–Bénard convection. Rev. Mod. Phys. 81, 503–537.CrossRefGoogle Scholar
Castaing, B., Gunaratne, G., Heslot, F., Kadanoff, L., Libchaber, A., Thomae, S., Wu, X. & Zanetti, S. 1989 Scaling of hard thermal turbulence in Rayleigh–Bénard convection. J. Fluid Mech. 204, 1–30.CrossRefGoogle Scholar
Chand, K., De, A.K. & Mishra, P.K. 2021 b Enhanced heat flux and flow structures in turbulent Rayleigh–Bénard convection with rough boundaries. Phys. Rev. Fluids 6, 124605.CrossRefGoogle Scholar
Chand, K., Sharma, M. & De, A.K. 2021 a Significance of near-wall dynamics in enhancement of heat flux for roughness aided turbulent Rayleigh–Bénard convection. Phys. Fluids 33 (6), 065114.CrossRefGoogle Scholar
Chand, K., Sharma, M., Venugopal, V.T. & De, A.K. 2019 Statistics of coherent structures in two-dimensional turbulent Rayleigh–Bénard convection. Phys. Fluids 31 (11), 115112.CrossRefGoogle Scholar
Chilla, F., Rastello, M., Chaumat, S. & Castaing, B. 2004 Long relaxation times and tilt sensitivity in Rayleigh–Bénard turbulence. Eur. Phys. J. B 40, 223–227.CrossRefGoogle Scholar
Chilla, F. & Schumacher, J. 2012 New perspectives in turbulent Rayleigh–Bénard convection. Eur. Phys. J. E 35, 58.CrossRefGoogle ScholarPubMed
Churchill, S.W. & Chu, H.H.S. 1975 Correlating equations for laminar and turbulent free convection from a vertical plate. Intl J. Heat Mass Transfer 18 (11), 1323–1329.CrossRefGoogle Scholar
De, A.K. 2016 A diffuse interface immersed boundary method for convective heat and fluid flow. Intl J. Heat Mass Transfer 92, 957–969.CrossRefGoogle Scholar
De, A.K. 2018 A diffuse interface immersed boundary method for complex moving boundary problems. J. Comput. Phys. 366, 226–251.CrossRefGoogle Scholar
De, A.K. & Sarkar, S. 2020 Three-dimensional wake dynamics behind a tapered cylinder with large taper ratio. Phys. Fluids 32, 063604.CrossRefGoogle Scholar
De, A.K. & Sarkar, S. 2021 a Dependence of wake structure on pitching frequency behind a thin panel at $Re=1000$. J. Fluid Mech. 924, A33.CrossRefGoogle Scholar
De, A.K. & Sarkar, S. 2021 b Spatial wake transition past a thin pitching plate. Phys. Rev. E 104, 025106.CrossRefGoogle Scholar
Doering, C.R. 2019 Thermal forcing and ‘classical’ and ‘ultimate’ regimes of Rayleigh–Bénard convection. J. Fluid Mech. 868, 1–4.CrossRefGoogle Scholar
Doering, C.R. 2020 Turning up the heat in turbulent thermal convection. Proc. Natl Acad. Sci. 117 (18), 9671–9673.CrossRefGoogle ScholarPubMed
Dropkin, D. & Somerscales, E. 1965 Heat transfer by natural convection in liquids confined by two parallel plates which are inclined at various angles with respect to the horizontal. Trans. ASME J. Heat Transfer 87 (1), 77–82.CrossRefGoogle Scholar
Frick, P., Khalilov, R., Kolesnichenko, I., Mamykin, A., Pakholkov, V., Pavlinov, A. & Rogozhkin, S. 2015 Turbulent convective heat transfer in a long cylinder with liquid sodium. Europhys. Lett. 109 (1), 14002.CrossRefGoogle Scholar
Fujii, T., Takeuchi, M., Fujii, M., Suzaki, K. & Uehara, H. 1970 Experiments on natural-convection heat transfer from the outer surface of a vertical cylinder to liquids. Intl J. Heat Mass Transfer 13 (5), 753–787.CrossRefGoogle Scholar
George, W.K. & Capp, S.P. 1979 A theory for natural convection turbulent boundary layers next to heated vertical surfaces. Intl J. Heat Mass Transfer 22 (6), 813–826.CrossRefGoogle Scholar
Goluskin, D. & Doering, C.R. 2016 Bounds for convection between rough boundaries. J. Fluid Mech. 804, 370–386.CrossRefGoogle Scholar
Grossmann, S. & Lohse, D. 2000 Scaling in thermal convection: a unifying theory. J. Fluid Mech. 407, 27–56.CrossRefGoogle Scholar
Guo, S., Zhou, S., Cen, X., Qu, L., Lu, Y., Sun, L. & Shang, X. 2015 The effect of cell tilting on turbulent thermal convection in a rectangular cell. J. Fluid Mech. 762, 273–287.CrossRefGoogle Scholar
Guo, S., Zhou, S., Qu, L., Cen, X. & Lu, Y. 2017 Evolution and statistics of thermal plumes in tilted turbulent convection. Eur. J. Mech. B/Fluids 111, 933–942.Google Scholar
Howard, L.N. 1963 Heat transport by turbulent convection. J. Fluid Mech. 17 (3), 405–432.CrossRefGoogle Scholar
Huang, Y.-X. & Zhou, Q. 2013 Counter-gradient heat transport in two-dimensional turbulent Rayleigh–Bénard convection. J. Fluid Mech. 737, R3.CrossRefGoogle Scholar
Jiang, L., Sun, C. & Calzavarini, E. 2019 Robustness of heat transfer in confined inclined convection at high Prandtl number. Phys. Rev. E 99, 013108.CrossRefGoogle ScholarPubMed
Johnston, H. & Doering, C.R. 2009 Comparison of turbulent thermal convection between conditions of constant temperature and constant flux. Phys. Rev. Lett. 102, 064501.CrossRefGoogle ScholarPubMed
Khalilov, R., Kolesnichenko, I., Pavlinov, A., Mamykin, A., Shestakov, A. & Frick, P. 2018 Thermal convection of liquid sodium in inclined cylinders. Phys. Rev. Fluids 3, 043503.CrossRefGoogle Scholar
Kolesnichenko, I., Mamykin, A., Pavlinov, A., Pakholkov, V., Rogozhkin, S., Frick, P., Khalilov, R. & Shepelev, S. 2015 Experimental study on free convection of sodium in a long cylinder. Therm. Engng 62 (1), 414–422.CrossRefGoogle Scholar
Kraichnan, R.H. 1962 Turbulent thermal convection at arbitrary Prandtl number. Phys. Fluids 5, 1374.CrossRefGoogle Scholar
Kushwaha, V.K. & De, A.K. 2020 Aerodynamics of multiple freely falling plates. Phys. Fluids 32 (10), 103603.CrossRefGoogle Scholar
Langebach, R. & Haberstroh, C. 2014 Natural convection in inclined pipes – a new correlation for heat transfer estimations. AIP Conf. Proc. 1573 (1), 1504–1511.CrossRefGoogle Scholar
Malkus, W.V.R. & Chandrasekhar, S. 1954 The heat transport and spectrum of thermal turbulence. Proc. R. Soc. Lond. A 225 (1161), 196–212.Google Scholar
Ng, C.S., Ooi, A., Lohse, D. & Chung, D. 2015 Vertical natural convection: application of the unifying theory of thermal convection. J. Fluid Mech. 764, 349–361.CrossRefGoogle Scholar
van der Poel, E.P., Ostilla-Mónico, R., Verzicco, R., Grossmann, S. & Lohse, D. 2015 a Logarithmic mean temperature profiles and their connection to plume emissions in turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 115, 154501.CrossRefGoogle ScholarPubMed
van der Poel, E.P., Stevens, R.J.A.M. & Lohse, D. 2011 Connecting flow structures and heat flux in turbulent Rayleigh–Bénard convection. Phys. Rev. E 84, 045303.CrossRefGoogle ScholarPubMed
van der Poel, E.P., Stevens, R.J.A.M. & Lohse, D. 2013 Comparison between two- and three-dimensional Rayleigh–Bénard convection. J. Fluid Mech. 736, 177–194.CrossRefGoogle Scholar
van der Poel, E.P., Verzicco, R., Grossmann, S. & Lohse, D. 2015 b Plume emission statistics in turbulent Rayleigh–Bénard convection. J. Fluid Mech. 772, 5–15.CrossRefGoogle Scholar
Priestley, C.H.B. 1954 Convection from a large horizontal surface. Austral. J. Phys. 7 (1), 176–201.CrossRefGoogle Scholar
Riedinger, X., Tisserand, J.-C., Seychelles, F., Castaing, B. & Chilla, F. 2013 Heat transport regimes in an inclined channel. Phys. Fluids 25 (1), 015117.CrossRefGoogle Scholar
Roche, P.-E., Castaing, B., Chabaud, B. & Hébral, B. 2001 Observation of the $\frac {1}{2}$ power law in Rayleigh–Bénard convection. Phys. Rev. E 63, 045303.CrossRefGoogle Scholar
Saunders, O.A. & Tizard, H.T. 1939 Natural convection in liquids. Proc. R. Soc. Lond. A 172 (948), 55–71.Google Scholar
Schmidt, E. & Beckmann, W. 1930 Das temperatur- und geschwindigkeitsfeld vor einer wärme abgebenden senkrechten platte bei natürlicher konvektion. Forsch. Ingenieurwes 1, 391–406.CrossRefGoogle Scholar
Sharma, M., Chand, K. & De, A.K. 2022 Investigation of flow dynamics and heat transfer mechanism in turbulent Rayleigh–Bénard convection over multi-scale rough surfaces. J. Fluid Mech. 941, A20.CrossRefGoogle Scholar
Shishkina, O. 2016 Momentum and heat transport scalings in laminar vertical convection. Phys. Rev. E 93, 051102.CrossRefGoogle ScholarPubMed
Shishkina, O. & Horn, S. 2016 Thermal convection in inclined cylindrical containers. J. Fluid Mech. 790, R3.CrossRefGoogle Scholar
Shishkina, O., Stevens, R.J.A.M., Grossmann, S. & Lohse, D. 2010 Boundary layer structure in turbulent thermal convection and its consequences for the required numerical resolution. New J. Phys. 12 (7), 075022.CrossRefGoogle Scholar
Shishkina, O. & Wagner, C. 2011 Modelling the influence of wall roughness on heat transfer in thermal convection. J. Fluid Mech. 686, 568–582.CrossRefGoogle Scholar
Shraiman, B.I. & Siggia, E.D. 1990 Heat transport in high-Rayleigh-number convection. Phys. Rev. A 42, 3650.CrossRefGoogle ScholarPubMed
Spiegel, E.A. 1963 A generalization of the mixing-length theory of turbulent convection. Astrophys. J. 138, 216.CrossRefGoogle Scholar
Stringano, G., Pascazio, G. & Verzicco, R. 2006 Turbulent thermal convection over grooved plates. J. Fluid Mech. 557, 307–336.CrossRefGoogle Scholar
Sugiyama, K., Calzavarini, E., Grossmann, S. & Lohse, D. 2009 Flow organization in two-dimensional non-Oberbeck–Boussinesq Rayleigh–Bénard convection in water. J. Fluid Mech. 637, 105–135.CrossRefGoogle Scholar
Sun, C., Xi, H.-D. & Xia, K.-Q. 2005 Azimuthal symmetry, flow dynamics, and heat transport in turbulent thermal convection in a cylinder with an aspect ratio of 0.5. Phys. Rev. Lett. 95, 074502.CrossRefGoogle Scholar
Toppaladoddi, S., Succi, S. & Wettlaufer, J.S. 2015 Turbulent transport processes at rough surfaces with geophysical applications. Procedia IUTAM 15, 34–40, iUTAM Symposium on Multiphase Flows with Phase Change: Challenges and Opportunities.CrossRefGoogle Scholar
Toppaladoddi, S., Succi, S. & Wettlaufer, J.S. 2017 Roughness as a route to the ultimate regime of thermal convection. Phys. Rev. Lett. 118, 074503.CrossRefGoogle Scholar
Toppaladoddi, S., Wells, A.J., Doering, C.R. & Wettlaufer, J.S. 2021 Thermal convection over fractal surfaces. J. Fluid Mech. 907, A12.CrossRefGoogle Scholar
Vasilev, A.Y., Kolesnichenko, I.V., Mamykin, A.D., Frick, P.G., Khalilov, R.I., Rogozhkin, S.A. & Pakholkov, V.V. 2015 Turbulent convective heat transfer in an inclined tube filled with sodium. Tech. Phys. 60, 1305–1309.CrossRefGoogle Scholar
Verzicco, R. & Camussi, R. 1999 Prandtl number effects in convective turbulence. J. Fluid Mech. 383, 55–73.CrossRefGoogle Scholar
Vishnu, V.T., De, A.K. & Mishra, P.K. 2019 Dynamics and statistics of reorientations of large-scale circulation in turbulent rotating Rayleigh–Bénard convection. Phys. Fluids 31 (5), 055112.CrossRefGoogle Scholar
Vishnu, V.T., De, A.K. & Mishra, P.K. 2020 Dynamics of large-scale circulation and energy transfer mechanism in turbulent Rayleigh–Bénard convection in a cubic cell. Phys. Fluids 32 (9), 095115.CrossRefGoogle Scholar
Wang, Q., Liu, H.-R., Verzicco, R., Shishkina, O. & Lohse, D. 2021 Regime transitions in thermally driven high-Rayleigh number vertical convection. J. Fluid Mech. 917, A6.CrossRefGoogle Scholar
Wang, Q., Verzicco, R., Lohse, D. & Shishkina, O. 2020 Multiple states in turbulent large-aspect-ratio thermal convection: what determines the number of convection rolls? Phys. Rev. Lett. 125, 074501.CrossRefGoogle ScholarPubMed
Wang, Q., Wan, Z.-H., Yan, R. & Sun, D.-J. 2018 b Multiple states and heat transfer in two-dimensional tilted convection with large aspect ratios. Phys. Rev. Fluids 3, 113503.CrossRefGoogle Scholar
Wang, Q., Wan, Z.-H., Yan, R. & Sun, D.-J. 2019 Flow organization and heat transfer in two-dimensional tilted convection with aspect ratio 0.5. Phys. Fluids 31 (2), 025102.CrossRefGoogle Scholar
Wang, Q., Xia, S., Wang, B., Sun, D., Zhou, Q. & Wan, Z. 2018 a Flow reversals in two-dimensional thermal convection in tilted cells. J. Fluid Mech. 849, 355–372.CrossRefGoogle Scholar
Weiss, S. & Ahlers, G. 2013 Effect of tilting on turbulent convection: cylindrical samples with aspect ratio $\gamma = 0.50$. J. Fluid Mech. 715, 314–334.CrossRefGoogle Scholar
Xin, S. & Le Quere, P. 1995 Direct numerical simulations of two-dimensional chaotic natural convection in a differentially heated cavity of aspect ratio 4. J. Fluid Mech. 304, 87–118.CrossRefGoogle Scholar
Yang, H., Wei, Y., Zhu, Z., Dou, H. & Qian, Y. 2018 Statistics of heat transfer in two-dimensional turbulent Rayleigh–Bénard convection at various Prandtl number. Entropy 20 (8), 582.CrossRefGoogle ScholarPubMed
Zhang, S., Chen, X., Xia, Z., Xi, H.-D., Zhou, Q. & Chen, S. 2021 Stabilizing/destabilizing the large-scale circulation in turbulent Rayleigh–Bénard convection with sidewall temperature control. J. Fluid Mech. 915, A14.CrossRefGoogle Scholar
Zhang, Y., Sun, C., Bao, Y. & Zhou, Q. 2018 How surface roughness reduces heat transport for small roughness heights in turbulent Rayleigh–Bénard convection. J. Fluid Mech. 836, R2.CrossRefGoogle Scholar
Zhang, Y., Zhou, Q. & Sun, C. 2017 Statistics of kinetic and thermal energy dissipation rates in two-dimensional turbulent Rayleigh–Bénard convection. J. Fluid Mech. 814, 165–184.CrossRefGoogle Scholar
Zhou, Q., Sugiyama, K., Stevens, R.J.A.M., Grossmann, S., Lohse, D. & Xia, K. 2011 Horizontal structures of velocity and temperature boundary layers in two-dimensional numerical turbulent Rayleigh–Bénard convection. Phys. Fluids 23, 125104.Google Scholar
Zhou, Q. & Xia, K. 2010 Physical and geometrical properties of thermal plumes in turbulent Rayleigh–Bénard convection. New J. Phys. 12, 075006.CrossRefGoogle Scholar
Zhu, X., Mathai, V., Stevens, R.J.A.M., Verzicco, R. & Lohse, D. 2018 Transition to the ultimate regime in two-dimensional Rayleigh–Bénard convection. Phys. Rev. Lett. 120, 144502.CrossRefGoogle Scholar
Zhu, X., Stevens, R.J.A.M., Shishkina, O., Verzicco, R. & Lohse, D. 2019 ${N}u \sim {R}a^{1/2}$ scaling enabled by multiscale wall roughness in Rayleigh–Bénard turbulence. J. Fluid Mech. 869, R4.CrossRefGoogle Scholar
Zhu, X., Stevens, R.J.A.M., Verzicco, R. & Lohse, D. 2017 Roughness facilitated local $1/2$ scaling does not imply the onset of the ultimate regime of thermal convection. Phys. Rev. Lett. 119, 154501.CrossRefGoogle Scholar
Zwirner, L. & Shishkina, O. 2018 Confined inclined thermal convection in low-Prandtl-number fluids. J. Fluid Mech. 850, 984–1008.CrossRefGoogle Scholar