Hostname: page-component-586b7cd67f-t8hqh Total loading time: 0 Render date: 2024-11-28T07:17:54.308Z Has data issue: false hasContentIssue false
  • English
  • Français

Effect of confinement in wall-bounded non-colloidal suspensions

Published online by Cambridge University Press:  21 June 2016

Stany Gallier ,
Elisabeth Lemaire ,
Laurent Lobry  and
Francois Peters
Stany Gallier*
Affiliation:
SAFRAN-Herakles, Le Bouchet Research Center, 91710 Vert le Petit, France
Elisabeth Lemaire
Affiliation:
University of Nice, CNRS, LPMC-UMR 7336, Parc Valrose, 06100 Nice, France
Laurent Lobry
Affiliation:
University of Nice, CNRS, LPMC-UMR 7336, Parc Valrose, 06100 Nice, France
Francois Peters
Affiliation:
University of Nice, CNRS, LPMC-UMR 7336, Parc Valrose, 06100 Nice, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

This paper presents three-dimensional numerical simulations of non-colloidal dense suspensions in a wall-bounded shear flow at zero Reynolds number. Simulations rely on a fictitious domain method with a detailed modelling of particle–particle and wall–particle lubrication forces, as well as contact forces including particle roughness and friction. 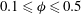This study emphasizes the effect of walls on the structure, velocity and rheology of a moderately confined suspension (channel gap to particle radius ratio of 20) for a volume fraction range $0.1\leqslant {\it\phi}\leqslant 0.5$. The wall region shows particle layers with a hexagonal structure. The size of this layered zone depends on volume fraction and is only weakly affected by friction. This structure implies a wall slip which is in good accordance with empirical models. Simulations show that this wall slip can be mitigated by reducing particle roughness. For 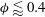${\it\phi}\lessapprox 0.4$, wall-induced layering has a moderate impact on the viscosity and second normal stress difference 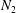$N_{2}$. Conversely, it significantly alters the first normal stress difference $N_{1}$ and can result in positive 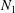$N_{1}$, in better agreement with some experiments. Friction enhances this effect, which is shown to be due to a substantial decrease in the contact normal stress 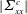$|{\it\Sigma}_{xx}^{c}|$ (where $x$ is the velocity direction) because of particle layering in the wall region.

JFM classification

Complex Fluids: Complex Fluids Non-Newtonian Flows: Rheology Complex Fluids: Suspensions
Type
Papers
Information
Journal of Fluid Mechanics , Volume 799 , 25 July 2016 , pp. 100 - 127
Check for updates
Copyright
© 2016 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Batchelor, G. K. & Green, J. T. 1972 The hydrodynamic interaction of two small freely-moving spheres in a linear flow field. J. Fluid Mech. 56 (02), 375–400.Google Scholar
Bian, X., Litvinov, S., Ellero, M. & Wagner, N. J. 2014 Hydrodynamic shear thickening of particulate suspension under confinement. J. Non-Newtonian Fluid Mech. 213, 39–49.Google Scholar
Blanc, F., Lemaire, E., Meunier, A. & Peters, F. 2013 Microstructure in sheared non-Brownian concentrated suspensions. J. Rheol. 57 (1), 273–292.Google Scholar
Blanc, F., Peters, F. & Lemaire, E. 2011 Experimental signature of the pair trajectories of rough spheres in the shear-induced microstructure in noncolloidal suspensions. Phys. Rev. Lett. 107 (20), 208302.Google Scholar
Bossis, G., Meunier, A. & Sherwood, J. D. 1991 Stokesian dynamics simulations of particle trajectories near a plane. Phys. Fluids A 3 (8), 1853–1858.Google Scholar
Brady, J. F. & Bossis, G. 1988 Stokesian dynamics. Annu. Rev. Fluid Mech. 20 (1), 111–157.Google Scholar
Chaoui, M. & Feuillebois, F. 2003 Creeping flow around a sphere in a shear flow close to a wall. Q. J. Mech. Appl. Maths 56 (3), 381–410.Google Scholar
Cheng, X., McCoy, J. H., Israelachvili, J. N. & Cohen, I. 2011 Imaging the microscopic structure of shear thinning and thickening colloidal suspensions. Science 333 (6047), 1276–1279.CrossRefGoogle ScholarPubMed
Coussot, P. 2005 Rheometry of Pastes, Suspensions, and Granular Materials: Applications in Industry and Environment. Wiley.Google Scholar
Couturier, É., Boyer, F., Pouliquen, O. & Guazzelli, E. 2011 Suspensions in a tilted trough: second normal stress difference. J. Fluid Mech. 10, 26–39.Google Scholar
Dai, S., Bertevas, E., Qi, F. & Tanner, R. 2013 Viscometric functions for noncolloidal sphere suspensions with Newtonian matrices. J. Rheol. 57 (2), 493–510.Google Scholar
Davit, Y. & Peyla, P. 2008 Intriguing viscosity effects in confined suspensions: a numerical study. Europhys. Lett. 83 (6), 64001.Google Scholar
Dbouk, T., Lobry, L. & Lemaire, E. 2013 Normal stresses in concentrated non-Brownian suspensions. J. Fluid Mech. 715, 239–272.Google Scholar
Eral, H. B., van den Ende, D., Mugele, F. & Duits, M. H. 2009 Influence of confinement by smooth and rough walls on particle dynamics in dense hard-sphere suspensions. Phys. Rev. E 80 (6), 061403.Google Scholar
Fernandez, N., Mani, R., Rinaldi, D., Kadau, D., Mosquet, M., Lombois-Burger, H., Cayer-Barrioz, J., Herrmann, H., Spencer, N. & Isa, L. 2013 Microscopic mechanism for shear thickening of non-Brownian suspensions. Phys. Rev. Lett. 111 (10), 108301.CrossRefGoogle ScholarPubMed
Gallier, S.2014 Simulation numérique de suspensions frictionnelles. Application aux propergols solides. PhD thesis, Université de Nice-Sophia Antipolis.Google Scholar
Gallier, S., Lemaire, E., Lobry, L. & Peters, F. 2014a A fictitious domain approach for the simulation of dense suspensions. J. Comput. Phys. 256, 367–387.Google Scholar
Gallier, S., Lemaire, E., Peters, F. & Lobry, L. 2014b Rheology of sheared suspensions of rough frictional particles. J. Fluid Mech. 757, 514–549.Google Scholar
Gamonpilas, C., Morris, J. F. & Denn, M. M. 2016 Shear and normal stress measurements in non-Brownian monodisperse and bidisperse suspensions. J. Rheol. 60 (2), 289–296.Google Scholar
Ganatos, P., Weinbaum, S. & Pfeffer, R. 1982 Gravitational and zero-drag motion of a sphere of arbitrary size in an inclined channel at low Reynolds number. J. Fluid Mech. 124, 27–43.Google Scholar
Garland, S., Gauthier, G., Martin, J. & Morris, J. F. 2013 Normal stress measurements in sheared non-Brownian suspensions. J. Rheol. 57 (1), 71–88.Google Scholar
Jana, S. C., Kapoor, B. & Acrivos, A. 1995 Apparent wall slip velocity coefficients in concentrated suspensions of noncolloidal particles. J. Rheol. 39 (6), 1123–1132.Google Scholar
Jeffrey, D. J. 1992 The calculation of the low Reynolds number resistance functions for two unequal spheres. Phys. Fluids A 4, 16.Google Scholar
Jeffrey, D. J., Morris, J. F. & Brady, J. F. 1993 The pressure moments for two rigid spheres in low-Reynolds-number flow. Phys. Fluids A 5 (10), 2317–2325.Google Scholar
Kim, S. & Karrila, S. J. 1991 Microhydrodynamics: Principles and Selected Applications, vol. 507. Butterworth-Heinemann Boston.Google Scholar
Kromkamp, J., van den Ende, D., Kandhai, D., van der Sman, R. & Boom, R. 2006 Lattice Boltzmann simulation of 2d and 3d non-Brownian suspensions in Couette flow. Chem. Engng Sci. 61 (2), 858–873.Google Scholar
Kulkarni, S. D. & Morris, J. F. 2009 Ordering transition and structural evolution under shear in Brownian suspensions. J. Rheol. 53 (2), 417–439.Google Scholar
Lootens, D., Van Damme, H., Hémar, Y. & Hébraud, P. 2005 Dilatant flow of concentrated suspensions of rough particles. Phys. Rev. Lett. 95 (26), 268302.Google Scholar
Mari, R., Seto, R., Morris, J. F. & Denn, M. M. 2014 Shear thickening, frictionless and frictional rheologies in non-Brownian suspensions. J. Rheol. 58 (6), 1693–1724.Google Scholar
Metzger, B., Rahli, O. & Yin, X. 2013 Heat transfer across sheared suspensions: role of the shear-induced diffusion. J. Fluid Mech. 724, 527–552.CrossRefGoogle Scholar
Michailidou, V. N., Petekidis, G., Swan, J. W. & Brady, J. F. 2009 Dynamics of concentrated hard-sphere colloids near a wall. Phys. Rev. Lett. 102 (6), 068302.Google Scholar
Morris, J. F. 2009 A review of microstructure in concentrated suspensions and its implications for rheology and bulk flow. Rheol. Acta 48 (8), 909–923.Google Scholar
Nguyen, N. Q. & Ladd, A. J. C. 2002 Lubrication corrections for lattice-Boltzmann simulations of particle suspensions. Phys. Rev. E 66 (4), 046708.Google Scholar
Peyla, P. & Verdier, C. 2011 New confinement effects on the viscosity of suspensions. Europhys. Lett. 94 (4), 44001.Google Scholar
Pieper, S. & Schmid, H. 2016 Layer-formation of non-colloidal suspensions in a parallel plate rheometer under steady shear. J. Non-Newtonian Fluid Mech. 234, 1–7.Google Scholar
Rintoul, M. D. & Torquato, S. 1996 Computer simulations of dense hard-sphere systems. J. Chem. Phys. 105 (20), 9258–9265.Google Scholar
Royer, J. R., Blair, D. L. & Hudson, S. D. 2016 A rheological signature of frictional interactions in shear thickening suspensions. Phys. Rev. Lett. 116 (18), 188301.Google Scholar
Sangani, A., Acrivos, A. & Peyla, P. 2011 Roles of particle–wall and particle–particle interactions in highly confined suspensions of spherical particles being sheared at low Reynolds numbers. Phys. Fluids 23, 083302.CrossRefGoogle Scholar
Seto, R., Mari, R., Morris, J. F. & Denn, M. M. 2013 Discontinuous shear thickening of frictional hard-sphere suspensions. Phys. Rev. Lett. 111 (21), 218301.Google Scholar
Shäfer, J., Dippel, S. & Wolf, D. E. 1996 Force schemes in simulations of granular materials. J. Phys. I 6 (1), 5–20.Google Scholar
Sierou, A. & Brady, J. F. 2001 Accelerated Stokesian dynamics simulations. J. Fluid Mech. 448, 115–146.Google Scholar
Sierou, A. & Brady, J. F. 2002 Rheology and microstructure in concentrated noncolloidal suspensions. J. Rheol. 46, 1031.Google Scholar
Silbert, L. E., Ertaş, D., Grest, G. S., Halsey, T. C., Levine, D. & Plimpton, S. J. 2001 Granular flow down an inclined plane: Bagnold scaling and rheology. Phys. Rev. E 64 (5), 051302.Google Scholar
Singh, A. & Nott, P. R. 2000 Normal stresses and microstructure in bounded sheared suspensions via Stokesian dynamics simulations. J. Fluid Mech. 412, 279–301.CrossRefGoogle Scholar
Singh, A. & Nott, P. R. 2003 Experimental measurements of the normal stresses in sheared Stokesian suspensions. J. Fluid Mech. 490, 293–320.Google Scholar
Smart, J. R. & Leighton, D. T. 1989 Measurement of the hydrodynamic surface roughness of noncolloidal spheres. Phys. Fluids A 1 (1), 52–60.Google Scholar
Snook, B., Butler, J. E. & Guazzelli, É. 2015 Dynamics of shear-induced migration of spherical particles in oscillatory pipe flow. J. Fluid Mech. 786, 128–153.Google Scholar
Stickel, J. J. & Powell, R. L. 2005 Fluid mechanics and rheology of dense suspensions. Annu. Rev. Fluid Mech. 37, 129–149.Google Scholar
Volkov, I., Cieplak, M., Koplik, J. & Banavar, J. 2002 Molecular dynamics simulations of crystallization of hard spheres. Phys. Rev. E 66 (6), 061401.Google Scholar
Yeo, K. & Maxey, M. R. 2010a Anomalous diffusion of wall-bounded non-colloidal suspensions in a steady shear flow. Europhys. Lett. 92 (2), 24008.Google Scholar
Yeo, K. & Maxey, M. R. 2010b Dynamics of concentrated suspensions of non-colloidal particles in Couette flow. J. Fluid Mech. 649, 205–231.CrossRefGoogle Scholar
Yeo, K. & Maxey, M. R. 2010c Ordering transition of non-Brownian suspensions in confined steady shear flow. Phys. Rev. E 81 (5), 051502.Google Scholar
Zarraga, I. E., Hill, D. A. & Leighton, D. T. Jr 2000 The characterization of the total stress of concentrated suspensions of noncolloidal spheres in Newtonian fluids. J. Rheol. 44, 185.Google Scholar
Zurita-Gotor, M., Bławzdziewicz, J. & Wajnryb, E. 2007 Swapping trajectories: a new wall-induced cross-streamline particle migration mechanism in a dilute suspension of spheres. J. Fluid Mech. 592, 447–469.CrossRefGoogle Scholar