Hostname: page-component-586b7cd67f-t7fkt Total loading time: 0 Render date: 2024-11-25T01:49:30.281Z Has data issue: false hasContentIssue false
  • English
  • Français

Dynamics of the jet wiping process via integral models

Published online by Cambridge University Press:  01 February 2021

M.A. Mendez Open the ORCID record for M.A. Mendez [Opens in a new window] ,
A. Gosset Open the ORCID record for A. Gosset [Opens in a new window] ,
B. Scheid Open the ORCID record for B. Scheid [Opens in a new window] ,
M. Balabane  and
J.-M. Buchlin
M.A. Mendez*
Affiliation:
Environmental and Applied Fluid Dynamics Department, von Karman Institute for Fluid Dynamics, 1640 Rhode-St-Genése, Belgium
A. Gosset
Affiliation:
Naval and Industrial Engineering Department, Universidade da Coruña, Campus de Esteiro, errol 15403, Spain
B. Scheid
Affiliation:
TIPs, Université libre de Bruxelles C.P. 165/67, 1050 Brussels, Belgium, EU
M. Balabane
Affiliation:
Laboratoire Analyse, Géométrie et Applications, Université Paris 13, Villetaneuse 93430, France
J.-M. Buchlin
Affiliation:
Environmental and Applied Fluid Dynamics Department, von Karman Institute for Fluid Dynamics, 1640 Rhode-St-Genése, Belgium
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

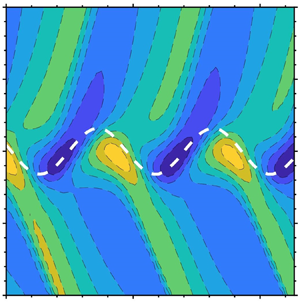
The jet wiping process is a cost-effective coating technique that uses impinging gas jets to control the thickness of a liquid layer dragged along a moving strip. This process is fundamental in various coating industries (mainly in hot-dip galvanizing) and is characterized by an unstable interaction between the gas jet and the liquid film that results in wavy final coating films. To understand the dynamics of the wave formation, we extend classic laminar boundary layer models for falling films to the jet wiping problem, including the self-similar integral boundary layer and the weighted integral boundary layer models. Moreover, we propose a transition and turbulence model to explore modelling extensions to larger Reynolds numbers and to analyse the impact of the modelling strategy on the liquid film dynamics. The validity of the long-wave formulation was first analysed on a simpler problem, consisting of a liquid film falling over an upward-moving wall, using volume of fluid simulations. This validation proved the robustness of the integral formulation in conditions that are well outside their theoretical limits of validity. Finally, the three models were used to study the response of the liquid coat to harmonic and non-harmonic oscillations and pulsations in the impinging jet. The impact of these disturbances on the average coating thickness and wave amplitude is analysed, and the range of dimensionless frequencies yielding maximum disturbance amplification is presented.

JFM classification

Multiphase and Particle-laden Flows: Gas/liquid flow Materials Processing Flows: Coating Interfacial Flows (free surface): Thin films
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 911 , 25 March 2021 , A47
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Alekseenko, S.V., Markovich, D.M. & Shtork, S.I. 1996 Wave flow of rivulets on the outer surface of an inclined cylinder. Phys. Fluids 8 (12), 3288–3299.CrossRefGoogle Scholar
Alekseenko, S.V., Nakoryakov, V.E. & Pokusaev, B.G. 1985 Wave formation on vertical falling liquid films. Intl J. Multiphase Flow 11 (5), 607–627.CrossRefGoogle Scholar
Alekseenko, S.V., Nakoryakov, V.E. & Pokusaev, B.G. 1994 Wave Flow of Liquid Films. Begell House.Google Scholar
Aniszewski, W., Zaleski, S., Popinet, S. & Saade, Y. 2019 Planar jet stripping of liquid coatings: numerical studies. Preprint.CrossRefGoogle Scholar
Beltaos, S. 1976 Oblique impingement of plane turbulent jets. J. Hydraul. Div. ASCE 102, 1177–1191.Google Scholar
Brackbill, J.U, Kothe, D.B. & Zemach, C. 1992 A continuum method for modeling surface tension. J. Comput. Phys. 100 (2), 335–354.CrossRefGoogle Scholar
Buchlin, J.M. 1997 Modelling of gas jet wiping. In Thin Liquid Films and Coating Processes, VKI Lecture Series. von Karman Institute for Fluid Dynamics.Google Scholar
Chang, H.-C. & Demekhin, E.A. 1996 Solitary wave formation and dynamics on falling films. In Advances in Applied Mechanics, pp. 1–58. Elsevier.CrossRefGoogle Scholar
Chang, H.-H. & Demekhin, E.A. 2002 Complex Wave Dynamics on Thin Films. Elsevier Science.Google Scholar
Craster, R.V. & Matar, O.K. 2009 Dynamics and stability of thin liquid films. Rev. Mod. Phys. 81 (3), 1131–1198.CrossRefGoogle Scholar
De Vita, F., Lagrée, P.-Y., Chibbaro, S. & Popinet, S. 2020 Beyond shallow water: appraisal of a numerical approach to hydraulic jumps based upon the boundary layer theory. Eur. J. Mech. (B/Fluids) 79, 233–246.CrossRefGoogle Scholar
Demekhin, E.A. & Shkadov, V.Y. 1985 Three-dimensional waves in a liquid flowing down a wall. Fluid Dyn. 19 (5), 689–695.CrossRefGoogle Scholar
Denner, F., Charogiannis, A., Pradas, M., Markides, C.N., van Wachem, B.G.M. & Kalliadasis, S. 2018 Solitary waves on falling liquid films in the inertia-dominated regime. J. Fluid Mech. 837, 491–519.CrossRefGoogle Scholar
Deryagin, S.M. & Levi, B.M. 1964 Film Coating Theory. The Focal Press.Google Scholar
Deshpande, S.S., Anumolu, L. & Trujillo, M.F. 2012 Evaluating the performance of the two-phase flow solver interFoam. Comput. Sci. Disc. 5 (1), 014016.CrossRefGoogle Scholar
Dietze, G.F. 2016 On the Kapitza instability and the generation of capillary waves. J. Fluid Mech. 789, 368–401.CrossRefGoogle Scholar
Dietze, G.F., Al-Sibai, F. & Kneer, R. 2009 Experimental study of flow separation in laminar falling liquid films. J. Fluid Mech. 637, 73–104.CrossRefGoogle Scholar
Dietze, G.F., Leefken, A. & Kneer, R. 2008 Investigation of the backflow phenomenon in falling liquid films. J. Fluid Mech. 595, 435–459.CrossRefGoogle Scholar
Dietze, G.F., Rohlfs, W., Nährich, K., Kneer, R. & Scheid, B. 2014 Three-dimensional flow structures in laminar falling liquid films. J. Fluid Mech. 743, 75–123.CrossRefGoogle Scholar
Dietze, G.F. & Ruyer-Quil, C. 2013 Wavy liquid films in interaction with a confined laminar gas flow. J. Fluid Mech. 722, 348–393.CrossRefGoogle Scholar
Doro, E.O. & Aidun, C.K. 2013 Interfacial waves and the dynamics of backflow in falling liquid films. J. Fluid Mech. 726, 261–284.CrossRefGoogle Scholar
van Driest, E.R. 1956 On turbulent flow near a wall. J. Aeronaut. Sci. 23 (11), 1007–1011.CrossRefGoogle Scholar
Ellen, C.H. & Tu, C.V. 1983 An analysis of jet stripping of molten metallic coatings. In Eighth Australasian Fluid Mechanics Conference.Google Scholar
Ellen, C.H. & Tu, C.V. 1984 An analysis of jet stripping of liquid coatings. J. Fluids Engng 106 (4), 399–404.CrossRefGoogle Scholar
Elrod, H.G. & Ng, C.W. 1967 A theory for turbulent fluid films and its application to bearings. Trans. ASME J. Lubr. Technol. 89 (3), 346–362.CrossRefGoogle Scholar
Elsaadawy, E.A., Hanumanth, G.S., Balthazaar, A.K.S., McDermid, J.R., Hrymak, A.N. & Forbes, J.F. 2007 a Coating weight model for the continuous hot-dip galvanizing process. Metall. Trans. B 38B, 413–424.CrossRefGoogle Scholar
Elsaadawy, E.A., Hanumanth, G.S., Balthazaar, A.K.S., McDermid, J.R., Hrymak, A.N. & Forbes, J.F. 2007 b Coating weight model for the continuous hot-dip galvanizing process. Metall. Trans. B 38 (3), 413–424.CrossRefGoogle Scholar
Eßl, W., Pfeiler, C., Reiss, G., Ecker, W., Riener, C.K. & Angeli, G. 2017 LES-VOF simulation and POD analysis of the gas-jet wiping process in continuous galvanizing lines. Steel Res. Intl 89 (2), 1700362.CrossRefGoogle Scholar
Frank, A.M. 2006 Shear driven solitary waves on a liquid film. Phys. Rev. E 74 (6), 065301.CrossRefGoogle ScholarPubMed
Frank, A.M. 2008 Numerical simulation of gas driven waves in a liquid film. Phys. Fluids 20 (12), 122102.CrossRefGoogle Scholar
Gao, D., Morley, N.B. & Dhir, V. 2003 Numerical simulation of wavy falling film flow using VOF method. J. Comput. Phys. 192 (2), 624–642.CrossRefGoogle Scholar
Gatapova, E.Y. & Kabov, O.A. 2008 Shear-driven flows of locally heated liquid films. Intl J. Heat Mass Transfer 51 (19–20), 4797–4810.CrossRefGoogle Scholar
Geshev, P.I. 2014 A simple model for calculating the thickness of a turbulent liquid film moved by gravity and gas flow. Thermophys. Aeromech. 21 (5), 553–560.CrossRefGoogle Scholar
Ginting, B.M. & Mundani, R.-P. 2018 Artificial viscosity technique: a Riemann-solver-free method for 2D urban flood modelling on complex topography. In Advances in Hydroinformatics, pp. 51–74. Springer.CrossRefGoogle Scholar
Gosset, A. 2007 Study of the interaction between a gas flow and a liquid film entrained by a moving surface. PhD thesis, Université Libre de Bruxelles- von Karman Institute for Fluid Dynamics.Google Scholar
Gosset, A. & Buchlin, J.-M. 2007 Jet wiping in hot-dip galvanization. J. Fluids Engng 129 (4), 466.CrossRefGoogle Scholar
Gosset, A., Mendez, M.A. & Buchlin, J.-M. 2019 An experimental analysis of the stability of the jet wiping process: part I – characterization of the coating uniformity. Exp. Therm. Fluid Sci. 103, 51–65.CrossRefGoogle Scholar
Haar, D.T. 1965 Wave flow of thin layers of a viscous fluid. In Collected Papers of P.L. Kapitza, pp. 662–709. Elsevier.CrossRefGoogle Scholar
Hernandez-Duenas, G. & Beljadid, A. 2016 A central-upwind scheme with artificial viscosity for shallow-water flows in channels. Adv. Water Resour. 96, 323–338.CrossRefGoogle Scholar
Hirs, G.G. 1973 A bulk-flow theory for turbulence in lubricant films. Trans. ASME J. Lubr. Technol. 95 (2), 137–145.CrossRefGoogle Scholar
Hocking, G.C., Sweatman, W.L., Fitt, A.D. & Breward, C. 2010 Deformations during jet-stripping in the galvanizing process. J. Engng Maths 70 (1–3), 297–306.Google Scholar
Howison, S. 2005 Practical Applied Mathematics. Cambridge University Press.CrossRefGoogle Scholar
Ishigai, S., Nakanisi, S., Koizumi, T. & Oyabu, Z. 1972 Hydrodynamics and heat transfer of vertical falling liquid films: part 1, classification of flow regimes. Bull. JSME 15 (83), 594–602.CrossRefGoogle Scholar
James, F., Lagrée, P.-Y., Le, M.H. & Legrand, M. 2019 Towards a new friction model for shallow water equations through an interactive viscous layer. ESAIM: Proc. 53 (1), 269–299.CrossRefGoogle Scholar
Johnstone, A.D., Kosasih, B., Phan, L.Q., Dixon, A. & Renshaw, W. 2019 Coating film profiles generated by fluctuating location of the wiping pressure and shear stress. ISIJ Intl 59 (2), 319–325.CrossRefGoogle Scholar
Kalliadasis, S., Ruyer-Quil, C., Scheid, B. & Velarde, M.G. 2012 Falling Liquid Films. Springer.CrossRefGoogle Scholar
Kapitza, P.L. 1948 a Wave flow of thin layers of a viscous fluid: II. Fluid flow in the presence of continuous gas flow and heat transfer. In Collected Papers of P.L. Kapitza (ed. D. Ter Haar), vol. II. Pergamon.Google Scholar
Kapitza, P.L. 1948 b Wave flow of thin layers of viscous fluid: I. Free flow. In Collected Papers of P.L. Kapitza (ed. D. Ter Haar), vol. II. Pergamon.Google Scholar
Karimi, G. & Kawaji, M. 1999 Flow characteristics and circulatory motion in wavy falling films with and without counter-current gas flow. Intl J. Multiphase Flow 25 (6), 1305–1319.Google Scholar
Katopodes, N.D. 2018 Free-Surface Flow: Shallow Water Dynamics. Butterworth-Heinemann.Google Scholar
King, C.J. 1966 Turbulent liquid phase mass transfer at free gas-liquid interface. Ind. Engng Chem. Res. 5 (1), 1–8.Google Scholar
Kurganov, A. & Liu, Y. 2012 New adaptive artificial viscosity method for hyperbolic systems of conservation laws. J. Comput. Phys. 231 (24), 8114–8132.CrossRefGoogle Scholar
Lacanette, D., Gosset, A., Vincent, S., Buchlin, J.-M. & Arquis, É. 2006 Macroscopic analysis of gas-jet wiping: numerical simulation and experimental approach. Phys. Fluids 18 (4), 042103.CrossRefGoogle Scholar
Lavalle, G., Li, Y., Mergui, S., Grenier, N. & Dietze, G.F. 2019 Suppression of the Kapitza instability in confined falling liquid films. J. Fluid Mech. 860, 608–639.CrossRefGoogle Scholar
Lavalle, G., Vila, J.-P., Lucquiaud, M. & Valluri, P. 2017 Ultraefficient reduced model for countercurrent two-layer flows. Phys. Rev. Fluids 2 (1), 014001.CrossRefGoogle Scholar
LeVeque, R.J. 2002 Finite Volume Methods for Hyperbolic Problems. Cambridge University Press.CrossRefGoogle Scholar
Liu, J. & Gollub, J.P. 1994 Solitary wave dynamics of film flows. Phys. Fluids 6 (5), 1702–1712.CrossRefGoogle Scholar
Liu, J., Schneider, J.B. & Gollub, J.P. 1995 Three-dimensional instabilities of film flows. Phys. Fluids 7 (1), 55–67.CrossRefGoogle Scholar
Lunz, D. & Howell, P.D. 2018 Dynamics of a thin film driven by a moving pressure source. Phys. Rev. Fluids 3 (11), 114801.CrossRefGoogle Scholar
Malamataris, N.A. & Balakotaiah, V. 2008 Flow structure underneath the large amplitude waves of a vertically falling film. AIChE J. 54 (7), 1725–1740.CrossRefGoogle Scholar
Mattsson, A.E. & Rider, W.J. 2014 Artificial viscosity: back to the basics. Intl J. Numer. Meth. Fluids 77 (7), 400–417.CrossRefGoogle Scholar
Mendez, M.A., Gosset, A. & Buchlin, J.-M. 2019 Experimental analysis of the stability of the jet wiping process, part II: multiscale modal analysis of the gas jet-liquid film interaction. Exp. Therm. Fluid Sci. 106, 48–67.CrossRefGoogle Scholar
Mendez, M.A., Gosset, A., Myrillas, K. & Buchlin, J. -M. 2017 a Numerical modal analysis of the jet wiping instability. In European Coating Symposium 2017.Google Scholar
Mendez, M.A., Scelzo, M.T. & Buchlin, J. -M. 2018 Multiscale modal analysis of an oscillating impinging gas jet. Exp. Therm. Fluid Sci. 91, 256–276.CrossRefGoogle Scholar
Mendez, M.A., Scheid, B. & Buchlin, J.-M. 2017 b Low Kapitza falling liquid films. Chem. Engng Sci. 170, 122–138.CrossRefGoogle Scholar
Meza, C.E. & Balakotaiah, V. 2008 Modeling and experimental studies of large amplitude waves on vertically falling films. Chem. Engng Sci. 63 (19), 4704–4734.CrossRefGoogle Scholar
Mudawar, I. & Houpt, R.A. 1993 Mass and momentum transport in smooth falling liquid films laminarized at relatively high Reynolds numbers. Intl J. Heat Mass Transfer 36 (14), 3437–3448.CrossRefGoogle Scholar
Mudawwar, I.A. & El-Masri, M.A. 1986 Momentum and heat transfer across freely-falling turbulent liquid films. Intl J. Multiphase Flow 12 (5), 771–790.CrossRefGoogle Scholar
Mukhopadhyay, S., Chhay, M. & Ruyer-Quil, C. 2017 Modelling transitional falling liquid films. In 23éme Congrés Français de Mécanique.Google Scholar
Myrillas, K., Gosset, A., Rambaud, P. & Buchlin, J.M. 2009 CFD simulation of gas-jet wiping process. Eur. Phys. J.-Spec. Top. 166 (1), 93–97.Google Scholar
Myrillas, K., Rambaud, P., Mataigne, J.-M., Gardin, P., Vincent, S. & Buchlin, J.-M. 2013 Numerical modeling of gas-jet wiping process. Chem. Engng Process. 68, 26–31.CrossRefGoogle Scholar
Nosoko, T. & Miyara, A. 2004 The evolution and subsequent dynamics of waves on a vertically falling liquid film. Phys. Fluids 16 (4), 1118–1126.CrossRefGoogle Scholar
Nosoko, T., Yoshimura, P.N., Nagata, T. & Oyakawa, K. 1996 Characteristics of two-dimensional waves on a falling liquid film. Chem. Engng Sci. 51 (5), 725–732.CrossRefGoogle Scholar
Pfeiler, C., Eßl, W., Reiss, G., Riener, C.K., Angeli, G. & Kharicha, A. 2017 Investigation of the gas-jet wiping process - two-phase large eddy simulations elucidate impingement dynamics and wave formation on zinc coatings. Steel Res. Intl 88 (9), 1600507.CrossRefGoogle Scholar
Riazi, M.R. 1996 Modeling of gas absorption into turbulent films with chemical reaction. Gas Sep. Purif. 10 (1), 41–46.CrossRefGoogle Scholar
Rio, E. & Boulogne, F. 2017 Withdrawing a solid from a bath: how much liquid is coated? Adv. Colloid Interface Sci. 247, 100–114.CrossRefGoogle ScholarPubMed
Ritcey, A., McDermid, J.R. & Ziada, S. 2017 The maximum skin friction and flow field of a planar impinging gas jet. J. Fluids Engng 139 (10), 101204.CrossRefGoogle Scholar
Rohlfs, W., Pischke, P. & Scheid, B. 2017 Hydrodynamic waves in films flowing under an inclined plane. Phys. Rev. Fluids 2 (4), 044003.CrossRefGoogle Scholar
Rohlfs, W. & Scheid, B. 2014 Phase diagram for the onset of circulating waves and flow reversal in inclined falling films. J. Fluid Mech. 763, 322–351.CrossRefGoogle Scholar
Ruyer-Quil, C., Kofman, N., Chasseur, D. & Mergui, S. 2014 Dynamics of falling liquid films. Eur. Phys. J. E 37 (4), 30.CrossRefGoogle ScholarPubMed
Ruyer-Quil, C. & Manneville, P. 2000 Improved modeling of flows down inclined planes. Eur. Phys. J. B 15 (2), 357–369.CrossRefGoogle Scholar
Ruyer-Quil, C. & Manneville, P. 2002 Further accuracy and convergence results on the modeling of flows down inclined planes by weighted-residual approximations. Phys. Fluids 14 (1), 170–183.CrossRefGoogle Scholar
Salamon, T.R., Armstrong, R.C. & Brown, R.A. 1994 Traveling waves on vertical films: numerical analysis using the finite element method. Phys. Fluids 6 (6), 2202–2220.CrossRefGoogle Scholar
Samanta, A. 2014 Shear-imposed falling film. J. Fluid Mech. 753, 131–149.CrossRefGoogle Scholar
Scheid, B., Ruyer-Quil, C. & Manneville, P. 2006 Wave patterns in film flows: modelling and three-dimensional waves. J. Fluid Mech. 562, 183.CrossRefGoogle Scholar
Schlichting, H. & Gersten, K. 2000 Boundary-Layer Theory. Springer.CrossRefGoogle Scholar
Shampine, L.F. 2005 a Solving hyperbolic PDEs in MATLAB. Appl. Numer. Anal. Comput. Maths 2 (3), 346–358.CrossRefGoogle Scholar
Shampine, L.F. 2005 b Two-step Lax–Friedrichs method. Appl. Maths Lett. 18 (10), 1134–1136.CrossRefGoogle Scholar
Shkadov, V.Y. 1971 Wave-flow theory for a thin viscous liquid layer. Fluid Dyn. 3 (2), 12–15.CrossRefGoogle Scholar
Shkadov, V.Y. & Beloglazkin, A.N. 2017 Integral boundary layer relations in the theory of wave flows for capillary liquid films. Moscow Univ. Mech. Bull. 72 (6), 133–144.CrossRefGoogle Scholar
Snoeijer, J.H., Ziegler, J., Andreotti, B., Fermigier, M. & Eggers, J. 2008 Thick films of viscous fluid coating a plate withdrawn from a liquid reservoir. Phys. Rev. Lett. 100 (24), 244502.CrossRefGoogle ScholarPubMed
Thompson, A.B., Gomes, S.N., Pavliotis, G.A. & Papageorgiou, D.T. 2016 Stabilising falling liquid film flows using feedback control. Phys. Fluids 28 (1), 012107.CrossRefGoogle Scholar
Thompson, A.B., Tseluiko, D. & Papageorgiou, D.T. 2015 Falling liquid films with blowing and suction. J. Fluid Mech. 787, 292–330.CrossRefGoogle Scholar
Thornton, J.A. & Graff, H.F. 1976 An analytical description of the jet finishing process for hot-dip metallic coatings on strip. Metall. Mater. Trans. B 7 (4), 607–618.CrossRefGoogle Scholar
Tihon, J., Serifi, K., Argyriadi, K. & Bontozoglou, V. 2006 Solitary waves on inclined films: their characteristics and the effects on wall shear stress. Exp. Fluids 41 (1), 79–89.CrossRefGoogle Scholar
Tomlin, R.J., Gomes, S.N., Pavliotis, G.A. & Papageorgiou, D.T. 2019 Optimal control of thin liquid films and transverse mode effects. SIAM J. Appl. Dyn. Syst. 18 (1), 117–149.CrossRefGoogle Scholar
Toro, E.F. 2001 Shock-Capturing Methods for Free-Surface. John Wiley & Sons.Google Scholar
Tu, C.V. & Ellen, C.H. 1986 Stability of liquid coating in the jet stripping process. In 9th Australasian Fluid Mechanics Conference.Google Scholar
Tu, C.V. & Wood, D.H. 1996 Wall pressure and shear stress measurements beneath an impinging jet. Exp. Therm. Fluid Sci. 13 (4), 364–373.CrossRefGoogle Scholar
Tuck, E.O. 1983 Continuous coating with gravity and jet stripping. Phys. Fluids 26 (9), 2352.CrossRefGoogle Scholar
Tuck, E.O. & Vanden-Broeck, J.-M. 1984 Influence of surface tension on jet-stripped continuous coating of sheet materials. AIChE J. 30 (5), 808–811.CrossRefGoogle Scholar
Vellingiri, R., Tseluiko, D., Savva, N. & Kalliadasis, S. 2013 Dynamics of a liquid film sheared by a co-flowing turbulent gas. Intl J. Multiphase Flow 56, 93–104.CrossRefGoogle Scholar
Whitham, G.B. 1999 Linear and Nonlinear Waves. John Wiley & Sons.CrossRefGoogle Scholar
Yoneda, H. 1993 Analysis of air-knife coating. PhD thesis, University of Minnesota, Minneapolis, MN.Google Scholar
Zhou, J.G., Causon, D.M., Mingham, C.G. & Ingram, D.M. 2001 The surface gradient method for the treatment of source terms in the shallow-water equations. J. Comput. Phys. 168 (1), 1–25.CrossRefGoogle Scholar

Mendez et al. supplementary movie 1

This figure shows the dimensionless film thickness of coating along a substrate moving upward during an unsteady jet wiping process. The impinging jet oscillates producing a time-dependent pressure gradient profile (blue line dotted, wrt bottom axis in Skhadov-like units). The film thickness evolution (top axis) is computed using three integral models presented in this work, namely the IBL (dashed black line), WIBL (continuous blue line), and TTBL (dotted red line).

Download Mendez et al. supplementary movie 1(Video)
Video 190.3 KB

Mendez et al. supplementary movie 2

Animation of Figure 15, Bottom. This figure shows the dimensionless film thickness of coating along a substrate moving upward during an unsteady jet wiping process. The impinging jet oscillates producing a time-dependent pressure gradient profile (blue line dotted, wrt bottom axis in Skhadov-like units). The film thickness evolution (top axis) is computed using three integral models presented in this work, namely the IBL (dashed black line), WIBL (continuous blue line), and TTBL (dotted red line).

Download Mendez et al. supplementary movie 2(Video)
Video 186.8 KB