Hostname: page-component-586b7cd67f-t7fkt Total loading time: 0 Render date: 2024-12-01T09:09:12.980Z Has data issue: false hasContentIssue false
  • English
  • Français

Dynamics of laser-induced cavitation bubbles near two perpendicular rigid walls

Published online by Cambridge University Press:  19 February 2018

Emil-Alexandru Brujan Open the ORCID record for Emil-Alexandru Brujan [Opens in a new window] ,
Tatsuya Noda ,
Atsushi Ishigami ,
Toshiyuki Ogasawara  and
Hiroyuki Takahira
Emil-Alexandru Brujan*
Affiliation:
Department of Hydraulics, University Politehnica Bucharest, 060042 Bucharest, Romania
Tatsuya Noda
Affiliation:
Department of Mechanical Engineering, Osaka Prefecture University, Sakai, Osaka 599-8531, Japan
Atsushi Ishigami
Affiliation:
Department of Mechanical Engineering, Osaka Prefecture University, Sakai, Osaka 599-8531, Japan
Toshiyuki Ogasawara
Affiliation:
Department of Mechanical Engineering, Osaka Prefecture University, Sakai, Osaka 599-8531, Japan
Hiroyuki Takahira
Affiliation:
Department of Mechanical Engineering, Osaka Prefecture University, Sakai, Osaka 599-8531, Japan
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The behaviour of a laser-induced cavitation bubble near two perpendicular rigid walls and its dependence on the distance between bubble and walls is investigated experimentally. It was shown by means of high-speed photography with 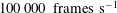$100\,000~\text{frames}~\text{s}^{-1}$ that an inclined jet is formed during bubble collapse and the bubble migrates in the direction of the jet. At a given position of the bubble with respect to the horizontal wall, the inclination of the jet increases with decreasing distance between the bubble and the second, vertical wall. A bubble generated at equal distances from the walls develops a jet that is directed in their bisection. The penetration of the jet into the opposite bubble surface leads to the formation of an asymmetric toroidal bubble that is perpendicular to the jet direction. At a large distance from the rigid walls, the toroidal bubble collapses in the radial direction, eventually disintegrating into tiny microbubbles. When the bubble is in contact with the horizontal wall at its maximum expansion, the toroidal ring collapses in both radial and toroidal directions, starting from the bubble part opposite to the vertical wall, and the bubble achieves a crescent shape at the moment of second collapse. The bubble oscillation is accompanied by a strong migration along the horizontal wall.

JFM classification

Drops and Bubbles: Bubble dynamics Drops and Bubbles: Cavitation Wakes/Jets: Jets
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 841 , 25 April 2018 , pp. 28 - 49
Check for updates
Copyright
© 2018 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Benjamin, T. B. & Ellis, A. T. 1966 The collapse of cavitation bubbles and the pressures thereby produced against solid boundaries. Phil. Trans. R. Soc. Lond. A 260, 221–240.Google Scholar
Best, J. P. & Blake, J. R. 1994 An estimate of the Kelvin impulse of a transient cavity. J. Fluid. Mech. 261, 75–93.Google Scholar
Blake, J. R. & Gibson, D. C. 1989 Cavitation bubbles near boundaries. Annu. Rev. Fluid Mech. 19, 99–123.Google Scholar
Blake, J. R., Hooton, M. C., Robinson, P. B. & Tong, R. P. 1997 Collapsing cavities, toroidal bubbles and jet impact. Phil. Trans. R. Soc. Lond. A 355, 537–550.Google Scholar
Blake, J. R., Robinson, P. B., Shima, A. & Tomita, Y. 1993 Interaction of two cavitation bubbles with a rigid boundary. J. Fluid Mech. 255, 707–721.CrossRefGoogle Scholar
Blake, J. R., Taib, B. B. & Doherty, G. 1986 Transient cavities near boundaries. Part 1. Rigid boundary. J. Fluid Mech. 170, 479–497.Google Scholar
Blake, J. R., Taib, B. B. & Doherty, G. 1987 Transient cavities near boundaries. Part 2. Free surface. J. Fluid Mech. 181, 197–212.CrossRefGoogle Scholar
Brujan, E. A., Ikeda, T. & Matsumoto, Y. 2012 Collapse of micrometer-sized cavitation bubbles near a rigid boundary. Microfluid. Nanofluid. 13, 957–966.Google Scholar
Brujan, E. A., Keen, G. S., Vogel, A. & Blake, J. R. 2002 The final stage of the collapse of a cavitation bubble close to a rigid boundary. Phys. Fluids 14, 85–92.CrossRefGoogle Scholar
Brujan, E. A., Nahen, K., Schmidt, P. & Vogel, A. 2001a Dynamics of laser-induced cavitation bubbles near an elastic boundary. J. Fluid Mech. 433, 251–281.Google Scholar
Brujan, E. A., Nahen, K., Schmidt, P. & Vogel, A. 2001b Dynamics of laser-induced cavitation bubbles near elastic boundaries: influence of the elastic modulus. J. Fluid Mech. 433, 283–314.Google Scholar
Brujan, E. A., Pearson, A. & Blake, J. R. 2005 Pulsating, buoyant bubbles close to a rigid boundary and near the null final Kelvin impluse state. Intl J. Multiphase Flow 31, 302–317.CrossRefGoogle Scholar
Chahine, G. L. & Genoux, P. F. 1983 Collapse of a cavitating vortex ring. J. Fluids Engng 105, 400–405.Google Scholar
Chahine, G. L., Kapahi, A., Choi, J. K. & Hsiao, C. T. 2016 Modeling of surface cleaning by cavitation bubble dynamics and collapse. Ultrason. Sonochem 29, 528–549.Google Scholar
Fong, S. W., Klaseboer, E., Turangan, C. K., Khoo, B. C. & Hung, K. C. 2006 Numerical analysis of a gas bubble near bio-materials in an ultrasound field. Ultrasound Med. Biol. 32, 925–942.Google Scholar
Gregorcic, P., Petkovsek, R. & Mozina, J. 2007 Investigation of a cavitation bubble between a rigid boundary and a free surface. J. Appl. Phys. 102, 094904.Google Scholar
Han, B., Kohler, K., Jungnickel, K., Mettin, R., Lauterborn, W. & Vogel, A. 2015 Dynamics of laser-induced bubble pairs. J. Fluid Mech. 771, 706–742.Google Scholar
Hsiao, C. T., Jayaprakash, A., Kapahi, A., Choi, J. K. & Chahine, G. L. 2014 Modeling of material pitting from cavitation bubble collapse. J. Fluid Mech. 755, 142–175.Google Scholar
Jayaprakash, A., Hsiao, C. T. & Chahine, G. 2012 Numerical and experimental study of the interaction of a spark-generated bubble and a vertical wall. J. Fluids Engng 134, 031301.CrossRefGoogle Scholar
Klaseboer, E. & Khoo, B. C. 2004 An oscillating bubble near an elastic material. J. Appl. Phys. 96, 5808–5818.Google Scholar
Klaseboer, E., Khoo, B. C. & Hung, K. C. 2005 Dynamics of an oscillating bubble near a floating structure. J. Fluids Struct. 21, 395–412.Google Scholar
Lauterborn, W. & Bolle, H. J. 1975 Experimental investigations of cavitation-bubble collapse in the neighbourhood of a solid boundary. J. Fluid Mech. 72, 391–400.CrossRefGoogle Scholar
Lee, M., Klaseboer, E. & Khoo, B. C. 2007 On the boundary integral method for the rebounding bubble. J. Fluid Mech. 570, 407–429.Google Scholar
Li, D. S., Kripfgans, O. D., Fabiili, M. L., Fowlkes, J. B. & Bull, J. S. 2014 Formation of toroidal bubbles from acoustic droplet vaporization. Appl. Phys. Lett. 104, 063706.Google Scholar
Li, S., Han, R., Zhang, A. M. & Wang, Q. X. 2016 Analysis of pressure field generated by a collapsing bubble. Ocean Engng 117, 22–38.Google Scholar
Lindau, O. & Lauterborn, W. 2003 Cinematographic observation of the collapse and rebound of a laser-produced cavitation bubble near a wall. J. Fluid Mech. 479, 327–348.Google Scholar
Liu, Y. L., Wang, Q. X., Wang, S. P. & Zhang, A. M. 2016 The motion of a 3D toroidal bubble and its interaction with a free surface near an inclined boundary. Phys. Fluids 28, 122101.Google Scholar
Philipp, A. & Lauterborn, W. 1998 Cavitation erosion by single laser-produced bubbles. J. Fluid Mech. 361, 75–116.CrossRefGoogle Scholar
Rayleigh, Lord 1917 On the pressure developed in a liquid during the collapse of a spherical cavity. Phil. Mag. 34, 94–98.CrossRefGoogle Scholar
Reuter, F., Gonzales, S. R., Mettin, R. & Ohl, C. D. 2017 Flow fields and vortex dynamics of bubbles collapsing near a solid boundary. Phys. Rev. Fluids 2, 064202.Google Scholar
Robinson, P. B. & Blake, J. R. 1994 Dynamics of cavitation bubble interactions. In Proc. IUTAM Symp. ‘Bubble Dynamics and Interface Phenomena’ (ed. Blake, J. R. et al. ), pp. 55–64. Kluwer.Google Scholar
Shima, A., Tomita, Y., Gibson, D. C. & Blake, J. R. 1989 The growth and collapse of cavitation bubbles near composite surfaces. J. Fluid Mech. 203, 199–214.Google Scholar
Tomita, Y., Kodama, T. & Shima, A. 1991 Secondary cavitation due to interaction of a collapsing bubble with a rising free surface. Appl. Phys. Lett. 59, 274–276.CrossRefGoogle Scholar
Tomita, Y., Robinson, P. B., Tong, R. P. & Blake, J. R. 2002 Growth and collapse of cavitation bubbles near a curved rigid boundary. J. Fluid Mech. 466, 259–283.Google Scholar
Tomita, Y. & Sato, K. 2017 Pulsed jets driven by two interacting cavitation bubbles produced at different times. J. Fluid Mech. 819, 465–493.CrossRefGoogle Scholar
Tomita, Y. & Shima, A. 1986 Mechanisms of impulsive pressure generation and damage pit formation by bubble collapse. J. Fluid Mech. 169, 535–564.CrossRefGoogle Scholar
Tomita, Y. & Shima, A. 1990 High-speed photographic observations of laser-induced cavitation bubbles in water. Acustica 71, 161–171.Google Scholar
Tong, R. P., Schiffers, W. P., Shaw, S. J., Blake, J. R. & Emmony, D. C. 1999 The role of ‘splashing’ in the collapse of a laser-generated cavity near a rigid boundary. J. Fluid Mech. 380, 339–361.Google Scholar
Vogel, A., Busch, S. & Parlitz, U. 1996 Shock wave emission and cavitation bubble generation by picosecond and nanosecond optical breakdown in water. J. Acoust. Soc. Am. 100, 148–165.CrossRefGoogle Scholar
Vogel, A., Lauterborn, W. & Timm, R. 1989 Optical and acoustic investigations of the dynamics of laser-produced cavitation bubbles near a solid boundary. J. Fluid Mech. 206, 299–338.Google Scholar
Wang, Q. 1998 The evolution of a gas bubble near an inclined wall. Theor. Comput. Fluid Dyn. 12, 29–51.Google Scholar
Wang, Q. X. 2004 Numerical simulation of violent bubble motion. Phys. Fluids 16, 1610–1619.Google Scholar
Wang, S., Duan, W. & Wang, Q. X. 2015 The bursting of a toroidal bubble at a free surface. Ocean Engng 109, 611–622.Google Scholar
Wang, Q. X. & Manmi, K. 2014 Three dimensional microbubble dynamics near a wall subject to high intensity ultrasound. Phys. Fluids 26, 032104.Google Scholar
Wang, Q. X., Manmi, K. & Calvisi, M. L. 2015 Numerical modelling of the 3D dynamics of ultrasound contrast agent microbubbles using the boundary integral method. Phys. Fluids 27, 022104.CrossRefGoogle Scholar
Zhang, S., Duncan, J. H. & Chahine, G. L. 1993 The final stage of the collapse of a cavitation bubble near a rigid wall. J. Fluid Mech. 257, 147–181.Google Scholar
Zhang, A. M. & Liu, Y. L. 2015 Improved three-dimensional bubble dynamics model based on boundary element method. J. Comput. Phys. 294, 208–223.Google Scholar
Zhang, A. M., Yang, Y., Tan, D. S. & Wang, Q. 2015 Experimental study on bubble dynamics subject to buoyancy. J. Fluid Mech. 776, 137–160.Google Scholar
Zhang, Y. L., Yeo, K. S., Khoo, B. C. & Wang, C. 2001 3D jet impact and toroidal bubbles. J. Comput. Phys. 166, 336–360.Google Scholar

Brujan et al. supplementary movie 1

Dynamics of a cavitation bubble situated near two perpendicular rigid walls for γh= 1.87, γv= 1.92, and Rmax= 0.83 mm. Side view

Download Brujan et al. supplementary movie 1(Video)
Video 181.5 KB

Brujan et al. supplementary movie 2

Dynamics of a cavitation bubble situated near two perpendicular rigid walls for γh= 1.11, γv= 15.25, and Rmax= 0.83 mm. Side view 2

Download Brujan et al. supplementary movie 2(Video)
Video 120.2 KB

Brujan et al. supplementary movie 3

Dynamics of a cavitation bubble situated near two perpendicular rigid walls for γh = 1.08, γv = 2.17, and Rmax = 0.85 mm. Side view

Download Brujan et al. supplementary movie 3(Video)
Video 130.7 KB

Brujan et al. supplementary movie 4

Dynamics of a cavitation bubble situated near two perpendicular rigid walls for γh= 1, γv = 16.8, and Rmax = 0.82 mm. Top view

Download Brujan et al. supplementary movie 4(Video)
Video 208 KB

Brujan et al. supplementary movie 5

Dynamics of a cavitation bubble situated near two perpendicular rigid walls for γh= 0.97, γv= 4.11, and Rmax = 0.73 mm. Top view

Download Brujan et al. supplementary movie 5(Video)
Video 200.4 KB

Brujan et al. supplementary movie 6

Dynamics of a cavitation bubble situated near two perpendicular rigid walls for γh = 1, γv = 2.59, and Rmax = 0.71 mm. Top view

Download Brujan et al. supplementary movie 6(Video)
Video 175.4 KB

Brujan et al. supplementary movie 7

Dynamics of a cavitation bubble situated near two perpendicular rigid walls for γh = 0.97, γv = 1.87, and Rmax= 0.73 mm. Top view

Download Brujan et al. supplementary movie 7(Video)
Video 176.9 KB

Brujan et al. supplementary movie 8

Dynamics of a cavitation bubble situated near two perpendicular rigid walls for γh = 1.87, γv = 2.81, and Rmax = 0.83 mm. Side view

Download Brujan et al. supplementary movie 8(Video)
Video 200.4 KB