Published online by Cambridge University Press: 06 February 2014
A systematic study of the effects of cell geometry on the dynamics of large-scale flows in turbulent thermal convection is carried out in horizontal cylindrical cells of different lengths filled with water. Four different flow modes are identified with increasing aspect ratio 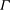 $\Gamma $. For small aspect ratios (
$\Gamma $. For small aspect ratios (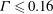 $\Gamma \leq 0.16$), the flow is highly confined in a thin disc-like cell with a quasi-two-dimensional (quasi-2D) large-scale circulation (LSC) in the circular plane of the cell. For larger aspect ratios (
$\Gamma \leq 0.16$), the flow is highly confined in a thin disc-like cell with a quasi-two-dimensional (quasi-2D) large-scale circulation (LSC) in the circular plane of the cell. For larger aspect ratios (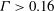 $\Gamma >0.16$), we observe periodic switching of the angular orientation
$\Gamma >0.16$), we observe periodic switching of the angular orientation  $\theta $ of the rotation plane of LSC between the two longest diagonals of the cell. The sides of the container along which the LSC oscillates changes at a critical aspect ratio
$\theta $ of the rotation plane of LSC between the two longest diagonals of the cell. The sides of the container along which the LSC oscillates changes at a critical aspect ratio 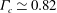 $\Gamma _{c}\simeq 0.82$. The measured switching period is equal to the LSC turnover time for
$\Gamma _{c}\simeq 0.82$. The measured switching period is equal to the LSC turnover time for 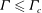 $\Gamma \leq \Gamma _c$, shows a sharp increase at
$\Gamma \leq \Gamma _c$, shows a sharp increase at  $\Gamma _{c}$ and decays exponentially to the LSC turnover time with increasing
$\Gamma _{c}$ and decays exponentially to the LSC turnover time with increasing 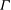 $\Gamma $. For
$\Gamma $. For 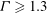 $\Gamma \geq 1.3$, a periodic rocking of LSC along the long axis of the cylinder is also observed. The measured probability density function
$\Gamma \geq 1.3$, a periodic rocking of LSC along the long axis of the cylinder is also observed. The measured probability density function 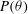 $P(\theta )$ of the LSC orientation
$P(\theta )$ of the LSC orientation 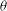 $\theta $ peaks at the two diagonal positions, and its shape is described by a phenomenological model proposed by Brown & Ahlers (Phys. Fluids, vol. 20, 2008b, 075101; J. Fluid Mech., vol. 638, 2009, pp. 383–400). Using this model, we describe the dynamics of the LSC orientation
$\theta $ peaks at the two diagonal positions, and its shape is described by a phenomenological model proposed by Brown & Ahlers (Phys. Fluids, vol. 20, 2008b, 075101; J. Fluid Mech., vol. 638, 2009, pp. 383–400). Using this model, we describe the dynamics of the LSC orientation 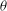 $\theta $ by stochastic motion in a double-well potential. The potential is predicted from a model in which the sidewall shape produces an orientation-dependent pressure on the LSC. This model also captures key features of the four flow modes. The experiment reveals an interesting array of rich dynamics of LSC in the horizontal cylinders, which are very different from those observed in the upright cylindrical convection cells. The success of the model for both upright and horizontal cylinders suggests that it can be applied to different geometries.
$\theta $ by stochastic motion in a double-well potential. The potential is predicted from a model in which the sidewall shape produces an orientation-dependent pressure on the LSC. This model also captures key features of the four flow modes. The experiment reveals an interesting array of rich dynamics of LSC in the horizontal cylinders, which are very different from those observed in the upright cylindrical convection cells. The success of the model for both upright and horizontal cylinders suggests that it can be applied to different geometries.