Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Son, Taeho
Yang, Eunjin
Yu, Eusun
Oh, Kyu Hwan
Moon, Myoung-Woon
and
Kim, Ho-Young
2017.
Effects of surface nanostructures on self-cleaning and anti-fogging characteristics of transparent glass.
Journal of Mechanical Science and Technology,
Vol. 31,
Issue. 11,
p.
5407.
Mekhitarian, Loucine
Sobac, Benjamin
Dehaeck, Sam
Haut, Benoît
and
Colinet, Pierre
2017.
Evaporation dynamics of completely wetting drops on geometrically textured surfaces.
EPL (Europhysics Letters),
Vol. 120,
Issue. 1,
p.
16001.
Kim, Jungchul
Ha, Jonghyun
and
Kim, Ho-Young
2017.
Capillary rise of non-aqueous liquids in cellulose sponges.
Journal of Fluid Mechanics,
Vol. 818,
Issue. ,
Ha, Jonghyun
Kim, Jungchul
Jung, Yeonsu
Yun, Giseok
Kim, Do-Nyun
and
Kim, Ho-Young
2018.
Poro-elasto-capillary wicking of cellulose sponges.
Science Advances,
Vol. 4,
Issue. 3,
Hu, Ran
Wu, Dong‐Sheng
Yang, Zhibing
and
Chen, Yi‐Feng
2018.
Energy Conversion Reveals Regime Transition of Imbibition in a Rough Fracture.
Geophysical Research Letters,
Vol. 45,
Issue. 17,
p.
8993.
Telecka, Agnieszka
Mandsberg, Nikolaj Kofoed
Li, Tao
Ludvigsen, Emil
Ndoni, Sokol
Di Mundo, Rosa
Palumbo, Fabio
Fiutowski, Jacek
Chiriaev, Serguei
and
Taboryski, Rafael
2018.
Mapping the transition to superwetting state for nanotextured surfaces templated from block-copolymer self-assembly.
Nanoscale,
Vol. 10,
Issue. 44,
p.
20652.
Chen, Enhui
Yuan, Quanzi
and
Zhao, Ya-Pu
2018.
Topography-induced symmetry transition of droplets on quasi-periodically patterned surfaces.
Soft Matter,
Vol. 14,
Issue. 30,
p.
6198.
Kim, Taehong
and
Kim, Wonjung
2018.
Viscous dewetting of metastable liquid films on substrates with microgrooves.
Journal of Colloid and Interface Science,
Vol. 520,
Issue. ,
p.
11.
Parihar, Vartika
Bandyopadhyay, Saumyadwip
Das, Soumen
and
Dasgupta, Sunando
2018.
Anisotropic Electrowetting on Wrinkled Surfaces: Enhanced Wetting and Dependency on Initial Wetting State.
Langmuir,
Vol. 34,
Issue. 5,
p.
1844.
Pi, Yueyang
Liu, Xuejuan
Zhang, Bo
and
Zhang, Chi
2019.
Study of hemiwicking with lattice Boltzmann simulations: A wetting state is dynamically trapped by pinning of imbibition front.
AIP Advances,
Vol. 9,
Issue. 12,
Mu, Lizhong
Yoshikawa, Harunori N.
Zoueshtiagh, Farzam
Ogawa, Tetsuya
Motosuke, Masahiro
and
Ueno, Ichiro
2019.
Quick Liquid Propagation on a Linear Array of Micropillars.
Langmuir,
Vol. 35,
Issue. 28,
p.
9139.
Rokoni, Arif
Kim, Dong-Ook
and
Sun, Ying
2019.
Micropattern-controlled wicking enhancement in hierarchical micro/nanostructures.
Soft Matter,
Vol. 15,
Issue. 32,
p.
6518.
Krishnan, Siva Rama
Bal, John
and
Putnam, Shawn A.
2019.
A simple analytic model for predicting the wicking velocity in micropillar arrays.
Scientific Reports,
Vol. 9,
Issue. 1,
Morrissette, Jared M.
Ghosh, Aritra
Campos, Raymond
Mates, Joseph E.
Mabry, Joseph M.
and
Megaridis, Constantine M.
2019.
Fluorinated Nanocomposite Coatings for Confinement and Pumpless Transport of Low‐Surface‐Tension Liquids.
Advanced Materials Interfaces,
Vol. 6,
Issue. 19,
Germain, Thomas
and
Putnam, Shawn A.
2020.
The Effect of the Stiffness of Soft Materials on Hemiwicking Performance.
p.
1239.
Lininger, Andrew
Zhu, Alexander Y.
Park, Joon-Suh
Palermo, Giovanna
Chatterjee, Sharmistha
Boyd, Jonathan
Capasso, Federico
and
Strangi, Giuseppe
2020.
Optical properties of metasurfaces infiltrated with liquid crystals.
Proceedings of the National Academy of Sciences,
Vol. 117,
Issue. 34,
p.
20390.
Nakamura, Hayate
Delafosse, Victor
Dietze, Georg F.
Yoshikawa, Harunori N.
Zoueshtiagh, Farzam
Mu, Lizhong
Tsukahara, Takahiro
and
Ueno, Ichiro
2020.
Enhancement of Meniscus Pump by Multiple Particles.
Langmuir,
Vol. 36,
Issue. 16,
p.
4447.
Nakamura, Hayate
Ogawa, Tetsuya
Inoue, Motochika
Hori, Takuma
Mu, Lizhong
Yoshikawa, Harunori N.
Zoueshtiagh, Farzam
Dietze, Georg
Tsukahara, Takahiro
and
Ueno, Ichiro
2020.
Pumping effect of heterogeneous meniscus formed around spherical particle.
Journal of Colloid and Interface Science,
Vol. 562,
Issue. ,
p.
133.
Kim, Jungchul
Moon, Myoung-Woon
and
Kim, Ho-Young
2020.
Capillary rise in superhydrophilic rough channels.
Physics of Fluids,
Vol. 32,
Issue. 3,
Kashyap, Varun
and
Ghasemi, Hadi
2020.
Solar heat localization: concept and emerging applications.
Journal of Materials Chemistry A,
Vol. 8,
Issue. 15,
p.
7035.
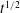 $t^{1/2}$ with
$t^{1/2}$ with  $t$ being time. We show that our model is consistent with pillar arrays of a wide range of pitch-to-height ratios, either square or skewed. At the microscopic scale, where the meniscus extension from individual pillars at the wetting front is considered, the extension distance begins to grow like
$t$ being time. We show that our model is consistent with pillar arrays of a wide range of pitch-to-height ratios, either square or skewed. At the microscopic scale, where the meniscus extension from individual pillars at the wetting front is considered, the extension distance begins to grow like  $t$ but the spreading slows down to behave like
$t$ but the spreading slows down to behave like  $t^{1/3}$ when the meniscus is far from the pillar. Our microscopic flow modelling allows us to find pillar spacing conditions under which the assumption of densely spaced pillars is valid.
$t^{1/3}$ when the meniscus is far from the pillar. Our microscopic flow modelling allows us to find pillar spacing conditions under which the assumption of densely spaced pillars is valid.


