Hostname: page-component-586b7cd67f-2brh9 Total loading time: 0 Render date: 2024-11-28T07:45:03.075Z Has data issue: false hasContentIssue false
  • English
  • Français

The dynamics of a non-dilute vesicle suspension in a simple shear flow

Published online by Cambridge University Press:  23 May 2013

Hong Zhao  and
Eric S. G. Shaqfeh
Hong Zhao*
Affiliation:
Department of Mechanical Engineering, Stanford University, Stanford, CA 94305, USA
Eric S. G. Shaqfeh
Affiliation:
Department of Mechanical Engineering, Stanford University, Stanford, CA 94305, USA Department of Chemical Engineering, Stanford University, Stanford, CA 94305, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We simulate multivesicle suspensions undergoing simple shear deformation by using the Stokes-flow boundary-integral equation method to quantify the effect of vesicle–vesicle interactions on the microstructure and rheology of the suspension. The binary collisions between vesicles are found to cause a vesicle’s instantaneous inclination angle to deviate considerably from its stationary tank-treading angle. The strength of the binary interaction becomes significant when the distance between centroids is less than three vesicle radii, which is consistent with observations from experiments. Vesicle interactions in the suspension delay the transition from tank-treading (TT) to trembling (TR)/tumbling (TU), which can be explained by the augmentation of the inclination angles in a binary collision when the viscosity ratio $\lambda $ is close to its critical value. Accordingly, in a non-dilute suspension the global minimum of the particle shear viscosity occurs at higher values of 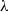$\lambda $ due to the close correlation between the vesicle’s orientation and particle shear stress. In the high-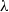$\lambda $ regime, collisions in a suspension disrupt the TU cycles of individual vesicles, resulting in intermittent TU/TR motions. In addition, an entangled state is observed to occur for an interacting vesicle pair in the TR/TU regime, where the centroids are aligned approximately parallel to the vorticity direction and rotates about it. The persistence of this close-range interaction results in two distinct peaks in the pair distribution of vesicles. However, their existence is limited to suspensions at low volume fraction, as they are otherwise disrupted by the three-body interactions that occur more frequently in a dense suspension.

JFM classification

Biological Fluid Dynamics: Capsule/cell dynamics Low-Reynolds-number flows: Low-Reynolds-number flows Complex Fluids: Suspensions
Type
Papers
Information
Journal of Fluid Mechanics , Volume 725 , 25 June 2013 , pp. 709 - 731
Copyright
©2013 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Batchelor, G. K. & Green, J. T. 1972 The determination of the bulk stress in a suspension of spherical particles to order ${C}^{2} $ . J. Fluid Mech. 56, 401–427.CrossRefGoogle Scholar
Cunha, F. R. D. A. & Hinch, E. J. 1996 Shear-induced dispersion in a dilute suspension of rough spheres. J. Fluid Mech. 309, 211–223.Google Scholar
Danker, G. & Misbah, C. 2007 Rheology of a dilute suspension of vesicles. Phys. Rev. Lett. 98, 088104.Google Scholar
Evans, E. & Rawicz, W. 1990 Entropy-driven tension and bending elasticity in condensed-fluid membranes. Phys. Rev. Lett. 64 (17), 2094–2097.CrossRefGoogle ScholarPubMed
Farutin, A., Biben, T. & Misbah, C. 2010 Analytical progress in the theory of vesicles under linear flow. Phys. Rev. E 81, 061904.Google Scholar
Hashimoto, H. 1959 On the periodic fundamental solutions of the Stokes equations and their application to viscous flow past a cubic array of spheres. J. Fluid Mech. 5, 317–328.Google Scholar
Helfrich, W. 1973 Elastic properties of lipid bilayers: theory and possible experiments. Z. Naturforsch 28 (11), 693–703.CrossRefGoogle ScholarPubMed
Kantsler, V., Segre, E. & Steinberg, V. 2008 Dynamics of interacting vesicles and rheology of vesicle suspension in shear flow. Eur. Phys. Lett. 82, 58005.CrossRefGoogle Scholar
Lac, E. & Barthés-Biesel, D. 2008 Pairwise interaction of capsules in simple shear flow: three-dimensional effects. Phys. Fluids 20, 040801.Google Scholar
Lac, E., Morel, A. & Barthés-Biesel, D. 2007 Hydrodynamic interaction between two identical capsules in simple shear flow. J. Fluid Mech. 573, 149–169.Google Scholar
Levant, M., Deschamps, J., Afik, E. & Steinberg, V. 2012 Characteristic spatial scale of vesicle pair interactions in a plane linear flow. Phys. Rev. E 85, 056306.Google Scholar
Loewenberg, M. & Hinch, E. J. 1996 Numerical simulation of a concentrated emulsion in shear flow. J. Fluid Mech. 321, 395–419.Google Scholar
Loewenberg, M. & Hinch, E. J. 1997 Collision of two deformable drops in shear flow. J. Fluid Mech. 338, 299–315.CrossRefGoogle Scholar
Loop, C. T. 1987 Smooth subdivision surfaces based on triangles. Master’s thesis, University of Utah.Google Scholar
Pozrikidis, C. 1992 Boundary Integral and Singularity Methods for Linearized Viscous Flow. Cambridge University Press.Google Scholar
Saintillan, D., Darve, E. & Shaqfeh, E. S. G. 2005 A smooth particle-mesh Ewald algorithm for Stokes suspension simulations: the sedimentation of fibres. Phys. Fluids 17, 033301.Google Scholar
Smart, J. R. & Leighton, D. T. 1991 Measurement of the drift of a droplet due to the presence of a plane. Phys. Fluids A 3 (1), 21–28.CrossRefGoogle Scholar
Sokolowski, J. & Zolesio, J. P. 1992 Introduction to Shape Optimization: Shape Sensitivity Analysis. Springer.Google Scholar
Stam, J. 1998 Evaluation of loop triangular subdivision surfaces at arbitrary parameter values. In Computer Graphics.CrossRefGoogle Scholar
Turitsyn, K. S. & Vergeles, S. S. 2008 Wrinkling of vesicles during transient dynamics in elongational flow. Phys. Rev. Lett. 100, 028103.Google Scholar
Vitkova, V., Mader, M.-A., Polack, B., Misbah, C. & Podgorski, T. 2008 Micro–macro link in rheology of erythrocyte and vesicle suspensions. Biophs. J. 95 (7), L33–L35.Google Scholar
Wilson, H. J. & Davis, R. H. 2000 The viscosity of a dilute suspension of rough spheres. J. Fluid Mech. 421, 339–367.CrossRefGoogle Scholar
Yazdani, A. & Bagchi, P. 2012 Three-dimensional numerical simulation of vesicle dynamics using a front-tracking method. Phys. Rev. E 85 (5), 056308.Google Scholar
Zabusky, N. J., Segre, E., Deschamps, J., Kantsler, V. & Steinberg, V. 2011 Dynamics of vesicles in shear and rotational flows: modal dynamics and phase diagram. Phys. Fluids 23 (4), 041905.Google Scholar
Zhao, H., Isfahani, A. H. G., Olson, L. & Freund, J. B. 2010 A spectral boundary integral method for micro-circulatory cellular flows. J. Comput. Phys. 229 (10), 3726–3744.CrossRefGoogle Scholar
Zhao, H. & Shaqfeh, E. S. G. 2011 The dynamics of a vesicle in simple shear flow. J. Fluid Mech. 674, 578–604.Google Scholar
Zhao, H., Shaqfeh, E. S. G. & Narsimhan, V. 2012 Shear-induced particle migration and margination in a cellular suspension. Phys. Fluids 24 (1), 01192.Google Scholar
Zhao, H., Spann, A. P. & Shaqfeh, E. S. G. 2011 The dynamics of a vesicle in a wall-bounded shear flow. Phys. Fluids 23 (12), 121901.Google Scholar
Zhong-can, O. Y. & Helfrich, W. 1989 Bending energy of vesicle membranes: general expressions for the first, second, and third variation of the shape energy and applications to spheres and cylinders. Phys. Rev. A 39 (10), 5280–5288.Google Scholar