Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Chen, Weilin
Ji, Chunning
and
Xu, Dong
2019.
Vortex-induced vibrations of two side-by-side circular cylinders with two degrees of freedom in laminar cross-flow.
Computers & Fluids,
Vol. 193,
Issue. ,
p.
104288.
Munir, Adnan
Zhao, Ming
Wu, Helen
and
Lu, Lin
2019.
Effects of gap ratio on flow-induced vibration of two rigidly coupled side-by-side cylinders.
Journal of Fluids and Structures,
Vol. 91,
Issue. ,
p.
102726.
Huera-Huarte, F. J.
and
Gharib, Morteza
2019.
Role of the near-tip region of a fin in fish propulsion.
Physical Review Fluids,
Vol. 4,
Issue. 6,
Huera-Huarte, F.J.
and
Jiménez-González, J.I.
2019.
Effect of diameter ratio on the flow-induced vibrations of two rigidly coupled circular cylinders in tandem.
Journal of Fluids and Structures,
Vol. 89,
Issue. ,
p.
96.
Chen, Weilin
Ji, Chunning
Xu, Dong
An, Hongwei
and
Zhang, Zhimeng
2020.
Flow-induced vibrations of two side-by-side circular cylinders at low Reynolds numbers.
Physics of Fluids,
Vol. 32,
Issue. 2,
Fan, Xiantao
Wang, Zhongchen
Chen, Xiaoyu
Wang, Yang
and
Tan, Wei
2020.
Experimental investigation on flow-induced vibration of flexible multi cylinders in atmospheric boundary layer.
International Journal of Mechanical Sciences,
Vol. 183,
Issue. ,
p.
105815.
Wu, Gefei
Lin, Weiqun
Du, Xiaoqing
Shi, Chunlin
and
Zhu, Jiongyi
2020.
On the flip-flopping phenomenon of two side-by-side circular cylinders at a high subcritical Reynolds number of 1.4 × 105.
Physics of Fluids,
Vol. 32,
Issue. 9,
Yuan, Fangyang
Cao, Yang
Tu, Chengxu
and
Lin, Jianzhong
2020.
Control of vortex shedding from two side-by-side cylinders using a pair of tangential jets.
AIP Advances,
Vol. 10,
Issue. 10,
Huera-Huarte, F. J.
2020.
Vortex-induced vibrations of a low mass-damping rigid circular cylinder with forced periodic rotations.
Physical Review Fluids,
Vol. 5,
Issue. 12,
Satheesh, S.
Díaz-Ojeda, H. R.
González, L. M.
and
Huera-Huarte, F. J.
2020.
Hydrodynamic Forces on a Cylinder With a Flexible Splitter Plate Near the Free Surface.
Journal of Offshore Mechanics and Arctic Engineering,
Vol. 142,
Issue. 6,
Fan, Xiantao
Wang, Zhongchen
Wang, Yang
and
Tan, Wei
2021.
The effect of vortices structures on the flow-induced vibration of three flexible tandem cylinders.
International Journal of Mechanical Sciences,
Vol. 192,
Issue. ,
p.
106132.
Fan, Xiantao
Guo, Kai
and
Wang, Yang
2022.
Toward a high performance and strong resilience wind energy harvester assembly utilizing flow-induced vibration: Role of hysteresis.
Energy,
Vol. 251,
Issue. ,
p.
123921.
Chen, Weilin
Ji, Chunning
Srinil, Narakorn
Yan, Yuhao
and
Zhang, Zhimeng
2022.
Effects of upstream wake on vortex-induced vibrations and wake patterns of side-by-side circular cylinders.
Marine Structures,
Vol. 84,
Issue. ,
p.
103223.
Alam, Md. Mahbub
2022.
Fluid force, moment, and torque measurements of oscillating prism and cylinder using loadcell.
Physics of Fluids,
Vol. 34,
Issue. 12,
Chen, Daomin
Wang, Ning
Chen, Zhenyu
and
Yu, Yue
2022.
Parametrically Excited Vibrations in a Nonlinear Damped Triple-Well Oscillator with Resonant Frequency.
Journal of Vibration Engineering & Technologies,
Vol. 10,
Issue. 2,
p.
781.
Chen, Weilin
Ji, Chunning
Xu, Dong
and
Alam, Md. Mahbub
2022.
Three-dimensional direct numerical simulations of two interfering side-by-side circular cylinders at intermediate spacing ratios.
Applied Ocean Research,
Vol. 123,
Issue. ,
p.
103162.
Zhang, Wei
Li, Xiuquan
and
He, Ziqiang
2023.
Two-degrees of freedom flow-induced vibration of circular cylinder with nonlinear stiffness.
Ocean Engineering,
Vol. 286,
Issue. ,
p.
115506.
Irawan, Yosua Heru
Raza, Syed Ahmad
and
Chern, Ming-Jyh
2023.
Passively enhanced VIV responses of side-by-side cylinders at moderate Reynolds number.
Applied Ocean Research,
Vol. 138,
Issue. ,
p.
103668.
Yu, Zhipeng
Wang, Enhao
Bao, Yan
Xiao, Qing
Li, Xiang
Incecik, Atilla
and
Lin, Bowen
2024.
VIV of two rigidly coupled side-by-side cylinders at subcritical Re.
International Journal of Mechanical Sciences,
Vol. 267,
Issue. ,
p.
108961.
Botella, Héctor
Fariña, Richard A.
and
Huera-Huarte, Francisco
2024.
Delta wing design in earliest nektonic vertebrates.
Communications Biology,
Vol. 7,
Issue. 1,
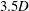 $3.5D$, where
$3.5D$, where  $D$ is the external diameter. The wake interference leads to responding amplitudes that are reduced if compared to those of isolated cylinders undergoing VIV, while responding frequencies are increased. The transverse force coefficients observed in the lock-in region increase and the upper branch shifts to smaller reduced velocities. The phase between motion and excitation is also shifted and values measured in the lower branch of the response tend to be smaller than those typical of isolated cylinders. At the smallest separation distances investigated, the wakes of the cylinders are synchronised in an out-of-phase mode of shedding, characterised by a biased flow towards the oscillating cylinder.
$D$ is the external diameter. The wake interference leads to responding amplitudes that are reduced if compared to those of isolated cylinders undergoing VIV, while responding frequencies are increased. The transverse force coefficients observed in the lock-in region increase and the upper branch shifts to smaller reduced velocities. The phase between motion and excitation is also shifted and values measured in the lower branch of the response tend to be smaller than those typical of isolated cylinders. At the smallest separation distances investigated, the wakes of the cylinders are synchronised in an out-of-phase mode of shedding, characterised by a biased flow towards the oscillating cylinder.


