Hostname: page-component-586b7cd67f-t7fkt Total loading time: 0 Render date: 2024-11-24T21:47:04.176Z Has data issue: false hasContentIssue false
  • English
  • Français

Dynamic-mode decomposition based analysis of shear coaxial jets with and without transverse acoustic driving

Published online by Cambridge University Press:  01 February 2016

Jia-Chen Hua Open the ORCID record for Jia-Chen Hua [Opens in a new window] ,
Gemunu H. Gunaratne ,
Douglas G. Talley ,
James R. Gord  and
Sukesh Roy
Jia-Chen Hua
Affiliation:
Department of Physics, University of Houston, Houston, TX 77204, USA
Gemunu H. Gunaratne
Affiliation:
Department of Physics, University of Houston, Houston, TX 77204, USA
Douglas G. Talley
Affiliation:
Aerospace Systems Directorate, Air Force Research Laboratory, Edwards Air Force Base, CA 93524, USA
James R. Gord
Affiliation:
Aerospace Systems Directorate, Air Force Research Laboratory, Wright-Patterson Air Force Base, OH 45433, USA
Sukesh Roy*
Affiliation:
Spectral Energies, LLC, Dayton, OH 45431, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Modal decompositions of unperturbed and acoustically driven injector flows from shear coaxial jets are implemented using dynamic-mode decomposition, which is a natural approach in the search for collective oscillatory behaviour in nonlinear systems. Previous studies using proper orthogonal decomposition had revealed the most energetic pairs of coherent structures in injector flows. One of the difficulties in extracting lower-energy coherent structures follows from the need to differentiate robust flow constituents from noise and other irregular facets of a flow. The identification of robust features is critical for applications such as flow control as well, since only they can be used for the tasks. A dynamic-mode decomposition based algorithm for this differentiation is introduced and used to identify different classes of robust dynamic modes. They include (1) background modes located outside the injector flow that decay rapidly, (2) injector modes – including those presented in earlier studies – located in the vicinity of the flow, (3) modes that persist under acoustic driving, (4) modes responding linearly to the driving and, most interestingly, (5) a mode whose density exhibits antiphase oscillatory behaviour in the observation plane and that appears only when $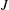J$, the outer-to-inner-jet momentum flux ratio, is sufficiently large; we infer that this is a projection of a mode rotating about the symmetry axis and born via a spontaneous symmetry breaking. Each of these classes of modes is analysed as 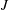$J$ is increased, and their consequences for the flow patterns are discussed.

JFM classification

Wakes/Jets: Jets Nonlinear Dynamical Systems: Low-dimensional models Nonlinear Dynamical Systems: Nonlinear Dynamical Systems
Type
Papers
Information
Journal of Fluid Mechanics , Volume 790 , 10 March 2016 , pp. 5 - 32
Check for updates
Copyright
© 2016 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bagheri, S., Schlatter, P., Schmid, P. J. & Henningson, D. S. 2009 Global stability of a jet in crossflow. J. Fluid Mech. 624, 33–44.Google Scholar
Berkooz, G., Holmes, P. & Lumley, J. L. 1993 The proper orthogonal decomposition in the analysis of turbulent flows. Annu. Rev. Fluid Mech. 25, 539–575.CrossRefGoogle Scholar
Budišić, M., Mohr, R. M. & Mezić, I. 2012 Applied Koopmanism. Chaos 22 (4), 047510.Google Scholar
Chen, K. K., Tu, J. H. & Rowley, C. W. 2012 Variants of dynamic mode decomposition: boundary condition, Koopman and Fourier analysis. J. Nonlinear Sci. 22, 887–915.Google Scholar
Cross, M. C. & Hohenberg, P. C. 1993 Pattern-formation outside of equilibrium. Rev. Mod. Phys. 65 (3), 851–1112.Google Scholar
Davis, D. W. & Chehroudi, B. 2006 Shear-coaxial jets from a Rocket-like injector in a transverse acoustic field at high pressures. In 44th AIAA Aerospace Sciences Meeting Reno, NV, Jan. 9–12, 2006.Google Scholar
Davis, D. W. & Chehroudi, B. 2007 Measurements in an acoustically driven coaxial jet under sub-, near-, and supercritical conditions. J. Propul. Power 23 (2), 364–374.Google Scholar
Hong, L., Fusetti, A., De Rosa, M. & Oschwald, M. 2006 Experimental investigation on the acoustic characteristics of LOZ/CH4 flame. In ICLASS-2006, 10th International Conference on Liquid Atomization and Spray Systems, Kyoto, Japan, Aug. 27–Sept. 1, 2006.Google Scholar
Hopfinger, E. J. 1998 Liquid jet instability and atomization in a coaxial gas stream. In Advances in Turbulence VII (ed. Frisch, U.), Fluid Mechanics and its Applications, vol. 46, pp. 69–78; 7th European Turbulence Conference (ETC7), St. Jean Cap Ferrat, France, Jun. 30–Jul. 03, 1998. Springer.CrossRefGoogle Scholar
Jovanovib́, M. R., Schmid, P. J. & Nichols, J. W. 2014 Sparsity-promoting dynamic mode decomposition. Phys. Fluids 26, 024103.Google Scholar
Leyva, I. A., Rodriguez, J., Chehroudi, B. & Talley, D. 2007 Preliminary results on coaxial jet spread angles and the effects of variable phase transverse acoustic fields. In 46th AIAA Aerospace Sciences Meeting and Exhibit Reno, NV, Jan. 7–10, 2008.Google Scholar
Lumley, J. L. 1967 The structure of inhomogeneous flow. In Atmospheric Turbulence and Radio Wave Propagation, pp. 166–176. Publishing House Nauka.Google Scholar
Mezic, I. 2013 Analysis of fluid flows via spectral properties of the Koopman operator. In Annual Review of Fluid Mechanics, Vol. 45 (ed. Davis, S. H. & Moin, P.), Annual Review of Fluid Mechanics, vol. 45, pp. 357–378.Google Scholar
Mezic, I. & Banaszuk, A. 2004 Comparison of systems with complex behavior. Physica D 197 (1), 101–133.Google Scholar
Miesse, C. C. 1955 The effect of ambient pressure oscillations on the disintegration and dispersion of a liquid jet. J. Jet Propulsion 25 (10), 525–530.Google Scholar
Motheau, E., Mery, Y., Nicoud, F. & Poinsot, T. 2013 Analysis and modeling of entropy modes in a realistic aeronautical gas turbine. Trans. ASME J. Engng Gas Turbines Power 135 (9), 092602.Google Scholar
Palacios, A., Gunaratne, G. H., Gorman, M. & Robbins, K. A. 1997 Cellular pattern formation in circular domains. Chaos 7 (3), 463–475.Google Scholar
Rowley, C. W., Mezic, I., Bagheri, S., Schlatter, P. & Henningson, D. S. 2009 Spectral analysis of nonlinear flows. J. Fluid Mech. 641, 115–127.Google Scholar
Roy, S., Hua, J.-C., Barnhill, W., Gunaratne, G. H. & Gord, J. R. 2015 Deconvolution of reacting-flow dynamics using proper orthogonal and dynamic mode decompositions. Phys. Rev. E 91 (1), 013001.Google Scholar
Schmid, P. J. 2009 Dynamic mode decomposition of experimental data. In Proceedings of 8th International Symposium on Particle Image Velocimetry, PIV09-0141, Melbourne, Victoria.Google Scholar
Schmid, P. J. 2010 Dynamic mode decomposition of numerical and experimental data. J. Fluid Mech. 656, 5–28.Google Scholar
Sirovich, L. 1987a Turbulence and the dynamics of coherent structures. 1. Coherent structures. Q. Appl. Maths 45 (3), 561–571.Google Scholar
Sirovich, L. 1987b Turbulence and the dynamics of coherent structures. 2. Symmetries and transformations. Q. Appl. Maths 45 (3), 573–582.Google Scholar
Sirovich, L. 1987c Turbulence and the dynamics of coherent structures. 3. Dynamics and scaling. Q. Appl. Maths 45 (3), 583–590.Google Scholar
Teshome, S.2012 Droplet combustion and non-reactive shear-coaxial jets with transverse acoustic excitation. PhD thesis. UCLA Electronic Theses and Dissertations.Google Scholar
Tirunagari, S., Hulkkonene, T., Vuorinen, V., Kaario, O. & Larmi, M. 2012a Proper orthogonal decomposition analysis of cross sectional fuel spray data. In ICLASS 2012, 12th Triennial International Conference on Liquid Atomization and Spray Systems, Heidelberg, Germany, September 2–6, 2012.Google Scholar
Tirunagari, S., Vuorinen, V., Kaario, O. & Larmi, M. 2012b Analysis of proper orthogonal decomposition and dynamic mode decomposition on LES of subsonic jets. CSI J. Comput. 1 (3), 20–26.Google Scholar
Villermaux, E., Rehab, H. & Hopfinger, E. J. 1998 Shear instabilities in the near field of coaxial jets. Phys. Fluids 10 (9), S2; 15th Annual Fluid Mechanics Photo Contest at the Meeting of the American Physical Society Division of Fluid Dynamics, San Francisco, CA, Nov. 1997.Google Scholar
Wicker, R. B. & Eaton, J. K. 1994 Near-field of a coaxial jet with and without axial excitation. AIAA J. 32 (3), 542–546; AIAA 31st Aerospace Sciences Meeting, Reno, NV, Jan. 11–14, 1993.CrossRefGoogle Scholar