Published online by Cambridge University Press: 07 February 2014
This paper discusses the interaction between droplets and entrained turbulent air flow in the far-downstream locations of a confined polydispersed isothermal spray. Simultaneous and planar measurements of droplet and gas velocities in the spray along with droplet size are obtained with the application of a novel experimental technique, developed by Hardalupas et al. (Exp. Fluids, vol. 49, 2010, pp. 417–434), which combines interferometric laser imaging for droplet sizing (ILIDS) with particle image velocimetry (PIV). These measurements quantified the spatial correlation coefficients of droplet–gas velocity fluctuations (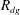 $R_{dg}$) and droplet–droplet velocity fluctuations (
$R_{dg}$) and droplet–droplet velocity fluctuations (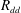 $R_{dd}$) conditional on droplet size classes, for various separation distances, and for axial and cross-stream velocity components. At the measurement location close to the spray edge, with increasing droplet size,
$R_{dd}$) conditional on droplet size classes, for various separation distances, and for axial and cross-stream velocity components. At the measurement location close to the spray edge, with increasing droplet size, 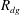 $R_{dg}$ was found to increase in axial direction and decrease in cross-stream direction. This suggests that as the gas-phase turbulence becomes more anisotropic away from the spray axis, the gravitational influence on droplet–gas correlated motion tends to increase. The effective length scales of the correlated droplet–gas motion were evaluated and compared with that for gas and droplet motion. The role of different turbulent eddies of the gas flow on the droplet–gas interaction was examined. The flow structures were extracted using proper orthogonal decomposition (POD) of the instantaneous gas velocity data, and their contribution on the spatial droplet–gas velocity correlation was evaluated, which quantified the momentum transfer between the two phases at different length scales of the gas flow. The droplets were observed to augment turbulence for the first three POD modes (larger scales) and attenuate it for the rest of the modes (smaller scales). It has been realized that apart from droplet Stokes number and mass loading, the dynamic range of length scales of the gas flow and the relative turbulent kinetic energy content of the flow structures (POD modes) must be considered in order to conclude if the droplets enhance or reduce the carrier-phase turbulence especially at the lower wavenumbers.
$R_{dg}$ was found to increase in axial direction and decrease in cross-stream direction. This suggests that as the gas-phase turbulence becomes more anisotropic away from the spray axis, the gravitational influence on droplet–gas correlated motion tends to increase. The effective length scales of the correlated droplet–gas motion were evaluated and compared with that for gas and droplet motion. The role of different turbulent eddies of the gas flow on the droplet–gas interaction was examined. The flow structures were extracted using proper orthogonal decomposition (POD) of the instantaneous gas velocity data, and their contribution on the spatial droplet–gas velocity correlation was evaluated, which quantified the momentum transfer between the two phases at different length scales of the gas flow. The droplets were observed to augment turbulence for the first three POD modes (larger scales) and attenuate it for the rest of the modes (smaller scales). It has been realized that apart from droplet Stokes number and mass loading, the dynamic range of length scales of the gas flow and the relative turbulent kinetic energy content of the flow structures (POD modes) must be considered in order to conclude if the droplets enhance or reduce the carrier-phase turbulence especially at the lower wavenumbers.