Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Philippi, Julien
Lagrée, Pierre-Yves
and
Antkowiak, Arnaud
2016.
Drop impact on a solid surface: short-time self-similarity.
Journal of Fluid Mechanics,
Vol. 795,
Issue. ,
p.
96.
Li, Yun
Zheng, Yi
Lan, Zhong
Xu, Wei
and
Ma, Xuehu
2017.
The evolution of droplet impacting on thin liquid film at superhydrophilic surface.
Applied Physics Letters,
Vol. 111,
Issue. 23,
Cimpeanu, R.
and
Moore, Madeleine Rose
2018.
Early-time jet formation in liquid–liquid impact problems: theory and simulations.
Journal of Fluid Mechanics,
Vol. 856,
Issue. ,
p.
764.
Che, Zhizhao
and
Matar, Omar K.
2018.
Impact of droplets on immiscible liquid films.
Soft Matter,
Vol. 14,
Issue. 9,
p.
1540.
Ionkin, Nikolay
and
Harris, Daniel M.
2018.
Note: A versatile 3D-printed droplet-on-demand generator.
Review of Scientific Instruments,
Vol. 89,
Issue. 11,
Jian, Zhen
Josserand, Christophe
Popinet, Stéphane
Ray, Pascal
and
Zaleski, Stéphane
2018.
Two mechanisms of droplet splashing on a solid substrate.
Journal of Fluid Mechanics,
Vol. 835,
Issue. ,
p.
1065.
Moore, Madeleine Rose
Whiteley, J. P.
and
Oliver, J. M.
2018.
On the deflection of a liquid jet by an air-cushioning layer.
Journal of Fluid Mechanics,
Vol. 846,
Issue. ,
p.
711.
Ersoy, Nuri Erdem
and
Eslamian, Morteza
2019.
Capillary surface wave formation and mixing of miscible liquids during droplet impact onto a liquid film.
Physics of Fluids,
Vol. 31,
Issue. 1,
Marcotte, Florence
Michon, Guy-Jean
Séon, Thomas
and
Josserand, Christophe
2019.
Ejecta, Corolla, and Splashes from Drop Impacts on Viscous Fluids.
Physical Review Letters,
Vol. 122,
Issue. 1,
Haldar, Krishnayan
and
Chakraborty, Sudipto
2019.
Investigation of chemical reaction during sodium alginate drop impact on calcium chloride film.
Physics of Fluids,
Vol. 31,
Issue. 7,
Wen, H.L.
Yu, C.H.
and
Sheu, Tony W.H.
2020.
On the development of LS-assisted VOF method for incompressible interfacial flows.
Journal of Computational Physics,
Vol. 406,
Issue. ,
p.
109188.
Jian, Zhen
Channa, Murad Ali
Kherbeche, Abderrahmane
Chizari, Hossain
Thoroddsen, Sigurdur T.
and
Thoraval, Marie-Jean
2020.
To Split or Not to Split: Dynamics of an Air Disk Formed under a Drop Impacting on a Pool.
Physical Review Letters,
Vol. 124,
Issue. 18,
Wang, Yuqian
Pei, Yiqiang
Qin, Jing
Lu, Lili
Li, Xiang
and
Peng, Zhenshan
2020.
Study on Hydrodynamic Characteristics of Fuel Droplet Impact on Oil Film.
Vol. 1,
Issue. ,
Chen, Z.
Shu, C.
Wang, Y.
and
Yang, L. M.
2020.
Oblique drop impact on thin film: Splashing dynamics at moderate impingement angles.
Physics of Fluids,
Vol. 32,
Issue. 3,
Jian, Zhen
Deng, Peng
and
Thoraval, Marie-Jean
2020.
Air sheet contraction.
Journal of Fluid Mechanics,
Vol. 899,
Issue. ,
Mirjalili, Shahab
and
Mani, Ali
2020.
Transitional stages of thin air film entrapment in drop-pool impact events.
Journal of Fluid Mechanics,
Vol. 901,
Issue. ,
Ni, Zhongyuan
Chu, Fuqiang
Feng, Yanhui
Yao, Shuhuai
and
Wen, Dongsheng
2021.
Large-Scale Dewetting via Surfactant-Laden Droplet Impact.
Langmuir,
Vol. 37,
Issue. 46,
p.
13729.
Galeano-Rios, Carlos A.
Cimpeanu, Radu
Bauman, Isabelle A.
MacEwen, Annika
Milewski, Paul A.
and
Harris, Daniel M.
2021.
Capillary-scale solid rebounds: experiments, modelling and simulations.
Journal of Fluid Mechanics,
Vol. 912,
Issue. ,
Li, G.Y.
Ma, X.J.
Zhang, B.W.
and
Xu, H.W.
2021.
An integrated smoothed particle hydrodynamics method for numerical simulation of the droplet impacting with heat transfer.
Engineering Analysis with Boundary Elements,
Vol. 124,
Issue. ,
p.
1.
Biroun, Mehdi H.
Haworth, Luke
Agrawal, Prashant
Orme, Bethany
McHale, Glen
Torun, Hamdi
Rahmati, Mohammad
and
Fu, YongQing
2021.
Surface Acoustic Waves to Control Droplet Impact onto Superhydrophobic and Slippery Liquid-Infused Porous Surfaces.
ACS Applied Materials & Interfaces,
Vol. 13,
Issue. 38,
p.
46076.
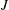 $J$: when the gas cushioning is weak, the jet is formed after a sequence of bubbles are entrapped and the jet speed is mostly selected by the Reynolds number of the impact. On the other hand, when the air cushioning is important, the lubrication of the gas beneath the drop and the liquid film controls the dynamics, leading to a single bubble entrapment and a weaker jet velocity.
$J$: when the gas cushioning is weak, the jet is formed after a sequence of bubbles are entrapped and the jet speed is mostly selected by the Reynolds number of the impact. On the other hand, when the air cushioning is important, the lubrication of the gas beneath the drop and the liquid film controls the dynamics, leading to a single bubble entrapment and a weaker jet velocity.


