Hostname: page-component-745bb68f8f-mzp66 Total loading time: 0 Render date: 2025-01-08T04:46:47.939Z Has data issue: false hasContentIssue false
  • English
  • Français

Drag reduction of circular cylinders by porous coating on the leeward side

Published online by Cambridge University Press:  19 January 2017

Katharina Klausmann  and
Bodo Ruck
Katharina Klausmann*
Affiliation:
Laboratory of Building- and Environmental Aerodynamics, Institute for Hydromechanics, Karlsruhe Institute of Technology, Kaiserstrasse 12, 76131 Karlsruhe, Germany
Bodo Ruck
Affiliation:
Laboratory of Building- and Environmental Aerodynamics, Institute for Hydromechanics, Karlsruhe Institute of Technology, Kaiserstrasse 12, 76131 Karlsruhe, Germany
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The present paper describes the effect of drag reduction of circular cylinders due to a porous coating on their leeward sides. To investigate the coating effect, experiments were conducted in a wind tunnel of Goettingen type. Systematic drag measurements were carried out for different cylinder configurations and flow velocities. The drag measurements were complemented by pressure and particle image velocimetry (PIV) flow field measurements around selected cylinders. The Reynolds numbers were varied in the subcritical range of $3\times 10^{4}<Re<1.4\times 10^{5}$. The results show that a thin porous layer on the leeward side, either incorporated in the cylinder shape or applied on the cylinder surface, leads to an increase of base pressure on the leeward side of the cylinder. It causes a reduction of drag and dampens oscillation amplitudes when compared to a cylinder without coating. Results obtained for different configurations with varying key parameters (coating angles, layer thicknesses and pore sizes of the porous material) clearly indicate the drag-reducing and amplitude-damping potential of leeward coating. The amount of drag reduction and amplitude damping depends on the combination of key parameters. It was demonstrated that the lowered drag coefficients 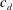$c_{d}$ were almost constant in the tested range of Reynolds numbers. A maximum reduction of drag of 13.2 % was measured. In addition, the results revealed a strong reduction of the pressure fluctuations around cylinders with a leeward coating due to the shift of the vortex region further downstream.

JFM classification

Flow Control: Drag reduction Low-Reynolds-number flows: Porous media
Type
Papers
Information
Journal of Fluid Mechanics , Volume 813 , 25 February 2017 , pp. 382 - 411
Check for updates
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Achenbach, E. 1971 Influence of surface roughness on the cross-flow around a circular cylinder. J. Fluid Mech. 46, 321–335.CrossRefGoogle Scholar
Ackeret, J. 1926 Grenzschichtabsaugung. Zeitschrift des VDI 70 (35), 1153–1158.Google Scholar
Allen, H. J. & Vincenti, W. G. 1944 Wall interference in a two-dimensional-flow wind tunnel, with consideration effect of compressibility. NACA 782, 155–183.Google Scholar
Bearman, P. W. & Harvey, J. K. 1993 Control of circular cylinder flow by the use of dimples. AIAA J. 31 (10), 1753–1756.CrossRefGoogle Scholar
Beavers, G. S. & Joseph, D. D. 1967 Boundary conditions at a naturally permeable wall. J. Fluid Mech. 30 (01), 197–207.CrossRefGoogle Scholar
Bechert, D. W. & Hoppe, G.1985 On the drag reduction of the shark skin. AIAA Shear Flow Control Conference, AIAA Paper 85-05.Google Scholar
Bhattacharyya, S. & Singh, A. K. 2011 Reduction in drag and vortex shedding frequency through porous sheath around a circular cylinder. Intl J. Numer. Methods Fluids 65, 683–698.CrossRefGoogle Scholar
Bloor, S. 1964 The transition to turbulence in the wake of a circular cylinder. J. Fluid Mech. 19 (2), 290.Google Scholar
Breugem, W. P., Boersma, B. J. & Uittenbogaard, R. E.2006 The influence of wall permeability on turbulent channel flow. 562, 35–72.Google Scholar
Bruneau, C.-H. & Mortazavi, I. 2006 Control of vortex shedding around a pipe section using a porous sheath. Intl J. Offshore Polar Engng 16 (2), 90–96.Google Scholar
Bruneau, C.-H. & Mortazavi, I. 2008 Numerical modelling and passive flow control using porous media. Comput. Fluids 37 (5), 488–498.CrossRefGoogle Scholar
Bruneau, C.-H., Mortazavi, I. & Gilliéron, P. 2006 Flow regularisation and drag reduction around blunt bodies using porous devices. European Drag Reduction and Flow Control Meeting, ERCOFTAC.Google Scholar
Carberry, J., Sheridan, J. & Rockwell, D. 2005 Controlled oscillations of a cylinder: forces and wake modes. J. Fluids Struct. 538 (1), 31.Google Scholar
Ceccio, S. L. 2010 Friction drag reduction of external flows with bubble and gas injection. Annu. Rev. Fluid Mech. 42 (1), 183–203.Google Scholar
Cetiner, O. & Rockwell, D. 2001 Streamwise oscillations of a cylinder in steady current. Part 2. Free-surface effects on vortex formation and loading. J. Fluid Mech. 427, 29–59.Google Scholar
Choi, H., Jeon, W.-P. & Kim, J. 2008 Control of flow over a bluff body. Annu. Rev. Fluid Mech. 40 (1), 113–139.CrossRefGoogle Scholar
Choi, J., Jeon, W. P. & Choi, H. 2006 Mechanism of drag reduction by dimples on a sphere. Phys. Fluids 18 (4), 1–5.CrossRefGoogle Scholar
Choi, K.-S., Jukes, T. & Whalley, R. 2011 Turbulent boundary-layer control with plasma actuators. Phil. Trans. R. Soc. Lond. A 369 (1940), 1443–1458.Google ScholarPubMed
Dalton, C. 1971 Allen and Vincenti Blockage corrections in a wind tunnel. AIAA J. 9 (9), 1864–1865.CrossRefGoogle Scholar
Fage, A. & Warsap, J. H. 1929 The effects of turbulence and surface roughness on the drag of a circular cylinder. Aero. Res. Counc. R&M 1283, HMSO.Google Scholar
Fransson, J. H. M., Konieczny, P. & Alfredsson, P. H. 2004 Flow around a porous cylinder subject to continuous suction or blowing. J. Fluids Struct. 19 (8), 1031–1048.CrossRefGoogle Scholar
Frohnapfel, B., Jovanović, J. & Delgado, A. 2007 Experimental investigations of turbulent drag reduction by surface-embedded grooves. J. Fluid Mech. 590, 107–116.Google Scholar
Galbraith, R. A. 1980 Flow pattern around a shrouded cylinder at Re = 5 × 103 . J. Wind Engng Ind. Aerodyn. 6, 227–242.Google Scholar
Galbraith, R. A. 1981 Aspects of the flow in the immediate vicinity of a porous shroud. J. Wind Engng Ind. Aerodyn. 8, 251–258.CrossRefGoogle Scholar
Hahn, S., Je, J. & Choi, H. 2002 Direct numerical simulation of turbulent channel flow with permeable walls. J. Fluid Mech. 450, 259–285.CrossRefGoogle Scholar
Hoerner, S. F. 1965 Fluid-Dynamic Drag. Selbstverlag.Google Scholar
Hoyt, J. W. 1972 The effect of additives on fluid friction. J. Basic Engng 94 (2), 258–285.CrossRefGoogle Scholar
James, D. F. & Truong, Q.-S. 1972 Wind load on cylinder with spanwise protrusion. J. Engng Mech. ASCE 98, 1573–1589.Google Scholar
von Kármán, T. 1911 Über den Mechanismus des Widerstandes den ein bewegter Körper in einer Flüssigkeit erfährt. Nachrichten von der Gesellschaft der Wissenschaften zu Göttingen, Mathematisch-Physikalische Klasse 1911, 509–517.Google Scholar
Kong, F. Y. & Schetz, J. A. 1982 Turbulent boundary layer over porous surfaces with different surface geometries. AIAA Paper 82-0030.Google Scholar
Kuznetsov, A. V. & Becker, S. M. 2004 Effect of the interface roughness on turbulent convectice heat transfer in a composite porous/fluid duct. Intl Commun. Heat Mass Transfer 31 (1), 11–20.CrossRefGoogle Scholar
de Lemos, M. J. S. 2005 Turbulent kinetic energy distribution across the interface between a porous medium and a clear region. Intl Commun. Heat Mass Transfer 32 (1–2), 107–115.Google Scholar
de Lemos, M. J. S. & Silva, R. A. 2006 Turbulent flow over a layer of a highly permeable medium simulated with a diffusion-jump model for the interface. Intl J. Heat Mass Transfer 49, 546–556.CrossRefGoogle Scholar
Lin, J., Towfighi, J. & Rockwell, D. 1995 Near-wake of a circular cylinder: control by steady and unsteady surface injection. J. Fluids Struct. 9, 659–669.CrossRefGoogle Scholar
Lumley, L. 1969 Drag reduction by additives. Annu. Rev. Fluid Mech. 1, 367–387.CrossRefGoogle Scholar
Maskell, E. C. 1963 A theory of blockage effects on bulff bodies and stalled wings in a closed wind tunnel. Aero. Res. Counc. R&M 3400, HMSO.Google Scholar
Merkle, C. L. & Deutsch, S. 1992 Microbubble drag reduction in liquid turbulent boundary layers. Appl. Mech. Rev. 45 (3), 103–127.CrossRefGoogle Scholar
Moessner, M. & Radespiel, R. 2015 Modelling of turbulent flow over porous media using a volume averaging approach and a Reynolds stress model. Comput. Fluids 108, 25–42.Google Scholar
Moreau, E. 2007 Airflow control by non-thermal plasma actuators. J. Phys. D 40 (3), 605–636.CrossRefGoogle Scholar
Perlin, M. & Ceccio, S. 2015 Mitigation of Hydrodynamics Resistance, 1st edn. World Scientific.CrossRefGoogle Scholar
Price, P. 1956 Suppression of the fluid-induced vibration of circular cylinders. J. Engng Mech. ASCE 82 (3), 1–22.Google Scholar
Roshko, A.1954 On the drag and shedding frequency of two-dimensional bluff bodies NACA TN 3169.Google Scholar
Roshko, R. 1955 On the wake and drag of bluff bodies. J. Aero. Sci. 22 (2), 124–132.Google Scholar
Roshko, R. 1961 Experiments on the flow past a circular cylinder at very high Reynolds number. J. Fluid Mech. 10, 345–356.CrossRefGoogle Scholar
Schlichting, H. 1948 Ein Näherungsverfahren zur Berechnung der laminaren Reibungsschicht mit Absaugung*. Ingenieur-Archiv 16 (3), 201–220.CrossRefGoogle Scholar
Shih, W. C. L., Wang, C., Coles, D. & Roshko, A. 1993 Experiments on flow past rough circular cylinders at large Reynolds numbers. J. Wind Engng Ind. Aerodyn. 49 (1–3), 351–368.CrossRefGoogle Scholar
Stansby, P. K. 1974 The effects of end plates on the base pressure coefficient of a circular cylinder. Aeronaut. J. 78, 36–37.CrossRefGoogle Scholar
Strouhal, V. 1878 Über eine besondere Art der Tonerregung. Ann. Phys. Chem. 5 (10), 216–251.CrossRefGoogle Scholar
Szepessy, S. 1993 On the control of circular cylinder flow by end plates. Eur. J. Mech. (B/Fluids) 12 (2), 217–243.Google Scholar
Vafai, K. & Thiyagaraja, R. 1987 Analysis of flow and heat transfer at the interface region of a porous medium. Intl J. Heat Mass Transfer 30 (7), 1391–1405.Google Scholar
West, G. S. & Apelt, C. J. 1993 Measurements of fluctuating pressures and forces on a circular cylinder in the Reynolds number range 104 to 2, 5 × 105 . J. Fluids Struct. 7, 227–244.Google Scholar
Westerweel, J. & Scarano, F. 2005 Universal outlier detection for PIV data. Exp. Fluids 39, 1096–1100.Google Scholar
Wieselsberger, C. 1913 Der Luftwiderstand von Kugeln. Z. Lufttechnik Motorluftschiffahrt 5, 140–144.Google Scholar
Wieselsberger, C. 1921 Neuere Feststellungen über die Gesetze des Flüssigkeits- und Luftwiderstandes. Physik. Z. 114, 321–328.Google Scholar
Wong, H. Y. 1979 A means of controling bluff body flow separation. J. Ind. Aerodyn. 4, 183–201.Google Scholar
Zagni, A. F. E. & Smith, K. V. H. 1976 Channel flow over permeable beds of graded spheres. J. Hydraul. Div. ASCE 102, 207–222.Google Scholar
Zdravkovich, M. M. 1997 Flow Around Circular Cylinders. Vol 1: Fundamentals. Oxford University Press.Google Scholar
Zdravkovich, M. M. 2003 Flow Around Circular Cylinders. Vol 2: Applications. Oxford University Press.CrossRefGoogle Scholar
Zippe, H. J. & Graf, W. H. 1983 Turbulent boundary-layer flow over permeable and non-permeable rough surfaces. J. Hydraul. Res. 21 (1), 51–65.CrossRefGoogle Scholar