Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
McConnochie, Craig D.
and
Kerr, Ross C.
2016.
The effect of a salinity gradient on the dissolution of a vertical ice face.
Journal of Fluid Mechanics,
Vol. 791,
Issue. ,
p.
589.
Ramudu, Eshwan
Hirsh, Benjamin Henry
Olson, Peter
and
Gnanadesikan, Anand
2016.
Turbulent heat exchange between water and ice at an evolving ice–water interface.
Journal of Fluid Mechanics,
Vol. 798,
Issue. ,
p.
572.
Gayen, Bishakhdatta
Griffiths, Ross W.
and
Kerr, Ross C.
2016.
Simulation of convection at a vertical ice face dissolving into saline water.
Journal of Fluid Mechanics,
Vol. 798,
Issue. ,
p.
284.
Magorrian, Samuel J.
and
Wells, Andrew J.
2016.
Turbulent plumes from a glacier terminus melting in a stratified ocean.
Journal of Geophysical Research: Oceans,
Vol. 121,
Issue. 7,
p.
4670.
McConnochie, Craig D.
and
Kerr, Ross C.
2016.
The turbulent wall plume from a vertically distributed source of buoyancy.
Journal of Fluid Mechanics,
Vol. 787,
Issue. ,
p.
237.
McConnochie, C. D.
and
Kerr, R. C.
2017.
Testing a common ice‐ocean parameterization with laboratory experiments.
Journal of Geophysical Research: Oceans,
Vol. 122,
Issue. 7,
p.
5905.
Hogg, Charlie A. R.
Dalziel, Stuart B.
Huppert, Herbert E.
and
Imberger, Jörg
2017.
Inclined gravity currents filling basins: the impact of peeling detrainment on transport and vertical structure.
Journal of Fluid Mechanics,
Vol. 820,
Issue. ,
p.
400.
McConnochie, Craig D.
and
Kerr, Ross C.
2017.
Enhanced ablation of a vertical ice wall due to an external freshwater plume.
Journal of Fluid Mechanics,
Vol. 810,
Issue. ,
p.
429.
McConnochie, Craig D.
and
Kerr, Ross C.
2018.
Dissolution of a sloping solid surface by turbulent compositional convection.
Journal of Fluid Mechanics,
Vol. 846,
Issue. ,
p.
563.
Kaye, N. B.
and
Cooper, P.
2018.
Source and boundary condition effects on unconfined and confined vertically distributed turbulent plumes.
Journal of Fluid Mechanics,
Vol. 850,
Issue. ,
p.
1032.
Loganathan, R. M.
and
Hunt, G. R.
2019.
Analytical solutions for flow induced by a vertically distributed turbulent plume.
Environmental Fluid Mechanics,
Vol. 19,
Issue. 4,
p.
801.
Meroni, Agostino N.
McConnochie, Craig D.
Cenedese, Claudia
Sutherland, Bruce
and
Snow, Kate
2019.
Nonlinear influence of the Earth’s rotation on iceberg melting.
Journal of Fluid Mechanics,
Vol. 858,
Issue. ,
p.
832.
Mondal, Mainak
Gayen, Bishakhdatta
Griffiths, Ross W.
and
Kerr, Ross C.
2019.
Ablation of sloping ice faces into polar seawater.
Journal of Fluid Mechanics,
Vol. 863,
Issue. ,
p.
545.
Li, Jinlong
Tang, Yao
Shi, Xilin
Xu, Wenjie
and
Yang, Chunhe
2019.
Modeling the construction of energy storage salt caverns in bedded salt.
Applied Energy,
Vol. 255,
Issue. ,
p.
113866.
Vreugdenhil, Catherine A.
and
Taylor, John R.
2019.
Stratification Effects in the Turbulent Boundary Layer beneath a Melting Ice Shelf: Insights from Resolved Large-Eddy Simulations.
Journal of Physical Oceanography,
Vol. 49,
Issue. 7,
p.
1905.
Pegler, Samuel S.
and
Davies Wykes, Megan S.
2020.
Shaping of melting and dissolving solids under natural convection.
Journal of Fluid Mechanics,
Vol. 900,
Issue. ,
Hewitt, Ian J.
2020.
Subglacial Plumes.
Annual Review of Fluid Mechanics,
Vol. 52,
Issue. 1,
p.
145.
Jinlong, Li
Wenjie, Xu
Jianjing, Zheng
Wei, Liu
Xilin, Shi
and
Chunhe, Yang
2020.
Modeling the mining of energy storage salt caverns using a structural dynamic mesh.
Energy,
Vol. 193,
Issue. ,
p.
116730.
Noble, T. L.
Rohling, E. J.
Aitken, A. R. A.
Bostock, H. C.
Chase, Z.
Gomez, N.
Jong, L. M.
King, M. A.
Mackintosh, A. N.
McCormack, F. S.
McKay, R. M.
Menviel, L.
Phipps, S. J.
Weber, M. E.
Fogwill, C. J.
Gayen, B.
Golledge, N. R.
Gwyther, D. E.
Hogg, A. McC.
Martos, Y. M.
Pena‐Molino, B.
Roberts, J.
van de Flierdt, T.
and
Williams, T.
2020.
The Sensitivity of the Antarctic Ice Sheet to a Changing Climate: Past, Present, and Future.
Reviews of Geophysics,
Vol. 58,
Issue. 4,
Jackson, R. H.
Nash, J. D.
Kienholz, C.
Sutherland, D. A.
Amundson, J. M.
Motyka, R. J.
Winters, D.
Skyllingstad, E.
and
Pettit, E. C.
2020.
Meltwater Intrusions Reveal Mechanisms for Rapid Submarine Melt at a Tidewater Glacier.
Geophysical Research Letters,
Vol. 47,
Issue. 2,
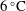 $6\,^{\circ }\text{C}$, where there is a transition from turbulent dissolution to turbulent melting. We quantify the turbulent convective dissolution of vertical ice bodies in the polar oceans, and compare our results with some field observations.
$6\,^{\circ }\text{C}$, where there is a transition from turbulent dissolution to turbulent melting. We quantify the turbulent convective dissolution of vertical ice bodies in the polar oceans, and compare our results with some field observations.


