Article contents
Disorganization of a hole tone feedback loop by an axisymmetric obstacle on a downstream end plate
Published online by Cambridge University Press: 29 September 2014
Abstract
This study investigates the suppression of the sound produced when a jet, issued from a circular nozzle or hole in a plate, goes through a similar hole in a second plate. The sound, known as a hole tone, is encountered in many practical engineering situations. The mean velocity of the air jet 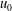 $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}u_0$ was
$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}u_0$ was 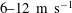 $6\text {--}12\ \mathrm{m}\ {\mathrm{s}}^{-1}$. The nozzle and the end plate hole both had a diameter of 51 mm, and the impingement length
$6\text {--}12\ \mathrm{m}\ {\mathrm{s}}^{-1}$. The nozzle and the end plate hole both had a diameter of 51 mm, and the impingement length  $L_{im}$ between the nozzle and the end plate was 50–90 mm. We propose a novel passive control method of suppressing the tone with an axisymmetric obstacle on the end plate. We find that the effect of the obstacle is well described by the combination (
$L_{im}$ between the nozzle and the end plate was 50–90 mm. We propose a novel passive control method of suppressing the tone with an axisymmetric obstacle on the end plate. We find that the effect of the obstacle is well described by the combination (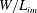 $W/L_{im}$,
$W/L_{im}$, 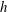 $h$) where
$h$) where 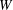 $W$ is the distance from the edge of the end plate hole to the inner wall of the obstacle, and
$W$ is the distance from the edge of the end plate hole to the inner wall of the obstacle, and 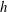 $h$ is the obstacle height. The tone is suppressed when backflows from the obstacle affect the jet shear layers near the nozzle exit. We do a direct sound computation for a typical case where the tone is successfully suppressed. Axisymmetric uniformity observed in the uncontrolled case is broken almost completely in the controlled case. The destruction is maintained by the process in which three-dimensional vortices in the jet shear layers convect downstream, interact with the obstacle and recursively disturb the jet flow from the nozzle exit. While regions near the edge of the end plate hole are responsible for producing the sound in the controlled case as well as in the uncontrolled case, acoustic power in the controlled case is much lower than in the uncontrolled case because of the disorganized state.
$h$ is the obstacle height. The tone is suppressed when backflows from the obstacle affect the jet shear layers near the nozzle exit. We do a direct sound computation for a typical case where the tone is successfully suppressed. Axisymmetric uniformity observed in the uncontrolled case is broken almost completely in the controlled case. The destruction is maintained by the process in which three-dimensional vortices in the jet shear layers convect downstream, interact with the obstacle and recursively disturb the jet flow from the nozzle exit. While regions near the edge of the end plate hole are responsible for producing the sound in the controlled case as well as in the uncontrolled case, acoustic power in the controlled case is much lower than in the uncontrolled case because of the disorganized state.
JFM classification
- Type
- Papers
- Information
- Copyright
- © 2014 Cambridge University Press
References
- 2
- Cited by


