Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Tufts, Matthew W.
Bisek, Nicholas J.
and
Kimmel, Roger L.
2019.
Implicit Large-Eddy Simulation of Discrete Roughness Boundary-Layer Transition with Added Perturbations.
Zhang, Chuanhong
2020.
Evolution of the second mode in a hypersonic boundary layer.
Physics of Fluids,
Vol. 32,
Issue. 12,
Xu, Jiakuan
Liu, Jianxin
Mughal, Shahid
Yu, Peixun
and
Bai, Junqiang
2020.
Secondary instability of Mack mode disturbances in hypersonic boundary layers over micro-porous surface.
Physics of Fluids,
Vol. 32,
Issue. 4,
Hader, Christoph
and
Fasel, Hermann F.
2020.
Wave packets on a flared cone at Mach 6.
Leinemann, Madlen
Hader, Christoph
and
Fasel, Hermann F.
2020.
Direct Numerical Simulations of the Nonlinear Transition Regime on a Flat Plate at Mach 6.
Mittal, Ankita
and
Girimaji, Sharath S.
2020.
Nonlinear evolution of perturbations in high Mach number wall-bounded flow: Pressure–dilatation effects.
Physics of Fluids,
Vol. 32,
Issue. 3,
Tufts, Matthew W.
Bisek, Nicholas J.
and
Kimmel, Roger L.
2020.
High-Fidelity Simulations of Discrete Roughness Boundary-Layer Transition with Added Perturbations.
Journal of Spacecraft and Rockets,
Vol. 57,
Issue. 5,
p.
1079.
Cui, Zhiliang
Zhao, Jin
He, Lichao
Jin, Haichuan
Zhang, Jun
and
Wen, Dongsheng
2020.
A reactive molecular dynamics study of hyperthermal atomic oxygen erosion mechanisms for graphene sheets.
Physics of Fluids,
Vol. 32,
Issue. 11,
Tufts, Matthew W.
Borg, Matthew P.
Bisek, Nicholas J.
and
Kimmel, Roger
2020.
High-Fidelity Simulation of HIFiRE-5 Boundary-Layer Transition.
Xiong, Youde
Yu, Tao
Lin, Liquan
Zhao, Jiaquan
and
Wu, Jie
2020.
Nonlinear Instability Characterization of Hypersonic Laminar Boundary Layer.
AIAA Journal,
Vol. 58,
Issue. 12,
p.
5254.
Dwivedi, Anubhav
Gs, Sidharth
Hollender, Charles
and
Candler, Graham V.
2020.
Input-output analysis for Görtler-type instability in axisymmetric hypersonic boundary-layers.
Zhu, Wenkai
Chen, Xi
Zhu, Yiding
and
Lee, Cunbiao
2020.
Nonlinear interactions in the hypersonic boundary layer on the permeable wall.
Physics of Fluids,
Vol. 32,
Issue. 10,
Unnikrishnan, S.
and
Gaitonde, Datta V.
2020.
Linear, nonlinear and transitional regimes of second-mode instability.
Journal of Fluid Mechanics,
Vol. 905,
Issue. ,
Hader, Christoph
and
Fasel, Hermann F.
2020.
Three-dimensional wave packet in a Mach 6 boundary layer on a flared cone.
Journal of Fluid Mechanics,
Vol. 885,
Issue. ,
Flood, John T.
Taubert, Lutz
and
Craig, Stuart A.
2020.
First and Mack-mode instabilities in a flat-plate boundary layer at Mach 4.
Hartman, Andrew
Hader, Christoph
and
Fasel, Hermann F.
2020.
Numerical Investigation of Nonlinear Entropy-Layer Instability Waves for Hypersonic Boundary-Layers.
Hader, Christoph
Leinemann, Madlen
and
Fasel, Hermann F.
2020.
Direct Numerical Simulations of Hypersonic Boundary-Layer Transition for a slender cone.
Park, Donghun
Park, Jaeyoung
Kim, Minwoo
Lim, Jiseop
Kim, Seungtae
and
Jee, Solkeun
2021.
Influence of initial phase on subharmonic resonance in an incompressible boundary layer.
Physics of Fluids,
Vol. 33,
Issue. 4,
Hartman, Andrew B.
Hader, Christoph
and
Fasel, Hermann F.
2021.
Nonlinear transition mechanism on a blunt cone at Mach 6: oblique breakdown.
Journal of Fluid Mechanics,
Vol. 915,
Issue. ,
Hartman, Andrew
Hader, Christoph
and
Fasel, Hermann F.
2021.
Direct Numerical Simulations of laminar-turbulent boundary-layer transition for blunt cones at Mach 6: Effect of Varying Nose Bluntness.
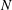 $N$-factors) for the primary instability were obtained for a frequency of approximately
$N$-factors) for the primary instability were obtained for a frequency of approximately 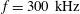 $f=300~\text{kHz}$. Low grid-resolution simulations were carried out in order to identify the azimuthal wavenumber that led to the strongest growth rates with respect to the secondary instability for a fundamental and subharmonic resonance scenario. It was found that for the BAM6QT conditions the fundamental resonance is much stronger compared to the subharmonic resonance. Subsequently, for the case which led to the strongest fundamental resonance onset, detailed investigations were carried out using high-resolution DNS. The simulation results exhibit streamwise streaks of very high skin friction and of high heat transfer at the cone surface. Streamwise ‘hot’ streaks on the flared cone surface were also observed in the experiments carried out at the BAM6QT facility using temperature sensitive paint. The presented findings provide strong evidence that the fundamental breakdown is a dominant and viable path to transition for the BAM6QT conditions.
$f=300~\text{kHz}$. Low grid-resolution simulations were carried out in order to identify the azimuthal wavenumber that led to the strongest growth rates with respect to the secondary instability for a fundamental and subharmonic resonance scenario. It was found that for the BAM6QT conditions the fundamental resonance is much stronger compared to the subharmonic resonance. Subsequently, for the case which led to the strongest fundamental resonance onset, detailed investigations were carried out using high-resolution DNS. The simulation results exhibit streamwise streaks of very high skin friction and of high heat transfer at the cone surface. Streamwise ‘hot’ streaks on the flared cone surface were also observed in the experiments carried out at the BAM6QT facility using temperature sensitive paint. The presented findings provide strong evidence that the fundamental breakdown is a dominant and viable path to transition for the BAM6QT conditions.

