Hostname: page-component-586b7cd67f-l7hp2 Total loading time: 0 Render date: 2024-11-28T18:44:13.790Z Has data issue: false hasContentIssue false
  • English
  • Français

Direct numerical simulation of turbulent heat transfer across a sheared wind-driven gas–liquid interface

Published online by Cambridge University Press:  13 September 2016

Ryoichi Kurose ,
Naohisa Takagaki ,
Atsushi Kimura  and
Satoru Komori
Ryoichi Kurose*
Affiliation:
Department of Mechanical Engineering and Science and Advanced Research Institute of Fluid Science and Engineering, Kyoto University, Kyoto daigaku-Katsura, Nishikyo-ku, Kyoto 615-8140, Japan
Naohisa Takagaki
Affiliation:
Department of Mechanical Engineering and Science and Advanced Research Institute of Fluid Science and Engineering, Kyoto University, Kyoto daigaku-Katsura, Nishikyo-ku, Kyoto 615-8140, Japan
Atsushi Kimura
Affiliation:
Department of Mechanical Engineering and Science and Advanced Research Institute of Fluid Science and Engineering, Kyoto University, Kyoto daigaku-Katsura, Nishikyo-ku, Kyoto 615-8140, Japan
Satoru Komori
Affiliation:
Department of Mechanical Engineering and Science and Advanced Research Institute of Fluid Science and Engineering, Kyoto University, Kyoto daigaku-Katsura, Nishikyo-ku, Kyoto 615-8140, Japan
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Turbulent heat transfer across a sheared wind-driven gas–liquid interface is investigated by means of a direct numerical simulation of gas–liquid two-phase turbulent flows under non-breaking wave conditions. The wind-driven wavy gas–liquid interface is captured using the arbitrary Lagrangian–Eulerian method with boundary-fitted coordinates on moving grids, and the temperature fields on both the gas and liquid sides, and the humidity field on the gas side are solved. The results show that although the distributions of the total, latent, sensible and radiative heat fluxes at the gas–liquid interface exhibit streak features such that low-heat-flux regions correspond to both low-streamwise-velocity regions on the gas side and high-streamwise-velocity regions on the liquid side, the similarity between the heat-flux streak and velocity streak on the gas side is more significant than that on the liquid side. This means that, under the condition of a fully developed wind-driven turbulent field on both the gas and liquid sides, the heat transfer across the sheared wind-driven gas–liquid interface is strongly affected by the turbulent eddies on the gas side, rather than by the turbulent eddies and Langmuir circulations on the liquid side. This trend is quite different from that of the mass transfer (i.e. 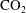$\text{CO}_{2}$ gas). This is because the resistance to heat transfer is normally lower than the resistance to mass transfer on the liquid side, and therefore the heat transfer is controlled by the turbulent eddies on the gas side. It is also verified that the predicted total heat, latent heat, sensible heat and enthalpy transfer coefficients agree well with previously measured values in both laboratory and field experiments. To estimate the heat transfer coefficients on both the gas and liquid sides, the surface divergence could be a useful parameter, even when Langmuir circulations exist.

JFM classification

Geophysical and Geological Flows: Air/sea interactions Multiphase and Particle-laden Flows: Gas/liquid flow Geophysical and Geological Flows: Geophysical and Geological Flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 804 , 10 October 2016 , pp. 646 - 687
Check for updates
Copyright
© 2016 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Assaf, G., Gerard, R. & Gordon, A. L. 1971 Some mechanisms of oceanic mixing revealed in aerial photographs. J. Geophys. Res. 76, 6550–6572.Google Scholar
Banerjee, S., Lakehal, D. & Fulgosi, M. 2004 Surface divergence models for scalar exchange between turbulent streams. Intl J. Multiphase Flow 30, 963–977.Google Scholar
Bird, R. B., Stewart, W. E. & Lightfoot, E. N. 1960 Transport Phenomena. Wiley.Google Scholar
Craik, A. D. D. & Leibovich, R. 1976 A rational model for Langmuir circulations. J. Fluid Mech. 73, 401–426.CrossRefGoogle Scholar
DeCosmo, J., Katsaros, K. B., Smith, S. D., Anderson, R. J., Oost, W. A., Bumke, K. & Chadwick, H. 1996 Air–sea exchange of water vapor and sensible heat: the humidity exchange over the sea (HEXOS) results. J. Geophys. Res. Oceans 101 (C5), 12001–12016.Google Scholar
Donelan, M. A. 1990 Air–sea interaction. In The Sea, Ocean Engineering Science (ed. LeMehaute, B. & Hanes, D. M.), vol. 9, pp. 239–292. Wiley.Google Scholar
Drennan, W. M., Zhang, J. A., French, J. R., Mccormick, C. & Black, P. G. 2007 Turbulent fluxes in the hurricane boundary layer. Part II: Latent heat fluxes. J. Atmos. Sci. 64, 1103–1115.CrossRefGoogle Scholar
Emanuel, K. A. 1986 An air–sea interaction theory for tropical cyclones. Part I: Steady-state maintenance. J. Atmos. Sci. 43, 585–604.Google Scholar
Francey, R. J. & Garratt, J. R. 1978 Eddy flux measurements over the ocean and related transfer coefficients. Boundary-Layer Meteorol. 14, 153–166.CrossRefGoogle Scholar
Friehe, C. A. & Schmitt, K. F. 1976 Parameterization of air–sea interface fluxes of sensible heat and moisture by the bulk aerodynamic formulas. J. Phys. Oceanogr. 6, 801–809.Google Scholar
Fulgosi, M., Lakehal, D., Banerjee, S. & De Angelis, V. 2003 Direct numerical simulation of turbulence in a sheared air–water flow with a deformable interface. J. Fluid Mech. 482, 319–345.Google Scholar
Garratt, J. R. & Hyson, P. 1975 Vertical fluxes of momentum, sensible heat and water vapour during the air mass transformation experiment (AMTEX) 1974. J. Met. Soc. Japan 53, 149–160.Google Scholar
Guo, X. & Shen, L. 2014 Numerical study of the effect of surface wave on turbulence underneath. Part 2. Eulerian and Lagrangian properties of turbulence kinetic energy. J. Fluid Mech. 744, 250–272.Google Scholar
Harlow, F. H. & Welch, J. E. 1965 Numerical calculation of time-dependent viscous incompressible flow of fluid with free surface. Phys. Fluids 8, 2182–2189.CrossRefGoogle Scholar
Hasse, L., Grunewald, M., Wucknitz, J., Dunckel, M. & Schriever, D. 1978 Profile derived turbulent fluxes in the surface layer under disturbed and undisturbed conditions during GATE. Meteor-Forschungsergebnisse B 13, 24–40.Google Scholar
Huang, N. E. 1979 On surface drift currents in the ocean. J. Fluid Mech. 91 (1), 191–208.Google Scholar
Ichiye, T. 1967 Upper ocean boundary-layer flow determined by dye diffusion. Phys. Fluids Suppl. 10, S270–S277.Google Scholar
Iwano, K.2014 Study on momentum, heat and mass transfer across the wind-sheared air–water interface at extremely high wind speeds. PhD thesis, Kyoto University (in Japanese).Google Scholar
Jahne, B. & Haußecker, H. 1998 Air–water gas exchange. Annu. Rev. Fluid Mech. 30, 443–468.Google Scholar
Johnson, H. K., Hojstrup, J., Vested, H. J. & Larsen, S. E. 1998 On the dependence of sea surface roughness on wind waves. J. Phys. Oceanogr. 28, 1702–1716.Google Scholar
Jones, I. S. F. & Toba, Y.(Eds) 2001 Wind Stress over The Ocean. p. 307. Cambridge University Press.CrossRefGoogle Scholar
Kermani, A., Khakpour, H. R., Shen, L. & Igusa, T. 2011 Statics of surface renewal of passive scalar in free-surface turbulence. J. Fluid Mech. 678, 379–416.Google Scholar
Kline, S. J., Reynolds, W. C., Schraub, F. A. & Runstadler, P. W. 1967 The structure of turbulent boundary layers. J. Fluid Mech. 30, 741–773.Google Scholar
Komori, S., Kurose, R., Iwano, K., Ukai, T. & Suzuki, N. 2010 Direct numerical simulation of wind-driven turbulence and scalar transfer at sheared gas–liquid interfaces. J. Turbul. 11, 1–20.CrossRefGoogle Scholar
Komori, S., Kurose, R., Takagaki, N., Ohtsubo, S., Iwano, K., Handa, K. & Shimada, S. 2011 Sensible and latent heat transfer across the air–water interface wind-driven turbulence. In Gas Transfer at Water Surfaces 2010 (ed. Komori, S., McGillis, W. & Kurose, K.), pp. 78–89. Kyoto University Press.Google Scholar
Komori, S., Nagaosa, R. & Murakami, Y. 1993b Turbulence structure and mass transfer across a sheared air–water interface in wind-driven turbulence. J. Fluid Mech. 249, 161–183.Google Scholar
Komori, S., Nagaosa, R., Murakami, Y., Chiba, S., Ishii, K. & Kuwahara, K. 1993a Direct numerical simulation of three-dimensional open-channel flow with zero-shear gas–liquid interface. Phys. Fluids A5, 115–125.Google Scholar
Kunugi, T. 1997 Direct numerical algorithm for multiphase flow with free surfaces and interfaces. Trans. JSME B 63 (609), 1576–1584.CrossRefGoogle Scholar
Lakehal, D., Fulgosi, M. & Yadigaroglu, G. 2008a Turbulence and heat exchange in condensing vapor–liquid flow. Phys. Fluids 20, 065101.Google Scholar
Lakehal, D., Fulgosi, M. & Yadigaroglu, G. 2008b Direct numerical simulation of condensing stratified flow. Trans. ASME J. Heat Transfer 130, 021501.Google Scholar
Lakehal, D., Fulgosi, M., Yadigaroglu, G. & Banerjee, S. 2003 Direct numerical simulation of turbulent heat transfer across a mobile, sheared gas–liquid interface. Trans. ASME J. Heat Transfer 125, 1129–1139.CrossRefGoogle Scholar
Lamb, H. 1932 Hydrodynamics, 6th edn. Cambridge University Press.Google Scholar
Langmuir, I. 1938 Surface motion of water induced by wind. Science 87, 119–123.CrossRefGoogle ScholarPubMed
Large, G. W. & Pond, S. 1982 Sensible and latent heat flux measurements over the ocean. J. Phys. Oceanogr. 12, 464–481.Google Scholar
Leibovich, S. 1983 The form and dynamics of Langmuir circulations. Annu. Rev. Fluid Mech. 15, 391–427.Google Scholar
Leibovich, S. & Paolucci, S. 1981 The instability of the ocean to Langmuir circulations. J. Fluid Mech. 102, 141–167.CrossRefGoogle Scholar
Lewis, W. K. & Whitman, W. G. 1924 Principles of gas absorption. Ind. Engng Chem. 16, 1215–1220.CrossRefGoogle Scholar
Lin, M.-Y., Moeng, C.-H., Tsai, W.-T., Sullivan, P. P. & Belcher, S. E. 2008 Direct numerical simulation of wind-wave generation processes. J. Fluid Mech. 616, 1–30.Google Scholar
McCready, M. J., Vassilliadou, E. & Hanratty, T. J. 1986 Computer simulation of turbulent mass transfer at a mobile interface. AIChE J. 32, 1108–1115.CrossRefGoogle Scholar
Melville, W. K., Shear, R. & Veron, F. 1998 Laboratory measurements of the generation and evolution of Langmuir circulations. J. Fluid Mech. 364, 31–58.Google Scholar
Mitsuyasu, H. & Nakayama, R. 1969 Measurements of waves and wind at Hakata Bay. Rep. Res. Inst. Appl. Mech. 33, 33–66.Google Scholar
Munz, C. & Roberts, P. V. 1984 The ratio of gas phase to liquid phase mass transfer coefficients in gas–liquid contacting processes. In Gas Transfer at Water Surfaces (ed. Brutsaert, W. & Jirka, G. H.), pp. 35–46. Reidal.Google Scholar
Ocampo-Torres, F. J., Donelan, M. A., Merzi, N. & Jia, A. 1994 Laboratory measurements of mass transfer of carbon dioxide and water vapour for smooth and rough flow conditions. Tellus 46B, 16–32.CrossRefGoogle Scholar
Pedreros, R., Dardier, G., Dupuis, H., Graber, H. C., Drennan, W. M., Weill, A. & Nacass, P. 2003 Momentum and heat fluxes via the eddy correlation method on the R/V L’Atalante and an ASIS buoy. J. Geophys. Res. Oceans 108 (C11), 3339.Google Scholar
Richter, D. H. & Stern, D. P. 2014 Evidence of spray-mediated air–sea enthalpy flux within tropical cyclones. Geophys. Res. Lett. 41, 2997–3003.Google Scholar
Schnieders, J., Garbe, S., Peirson, W. L., Smith, G. B. & Zappa, C. J. 2013 Analyzing the footprints of near surface aqueous turbulence: an image processing-based approach. J. Geophys. Res. Oceans 118, 1272–1286.Google Scholar
Smith, C. R. & Metzler, S. P. 1983 The characteristics of low-speed streaks in the near-wall region of a turbulent boundary layer. J. Fluid Mech. 129, 27–54.Google Scholar
Sullivan, P. P., McWilliams, J. C. & Moeng, C.-H. 2000 Simulation of turbulent flow over idealized water waves. J. Fluid Mech. 404, 47–85.Google Scholar
Takagaki, N.2009 Effects of rainfall on mass transfer across the air–water interface. PhD thesis, Kyoto University (in Japanese).Google Scholar
Takagaki, N., Komori, S. & Suzuki, N. 2016 Estimation of friction velocity from the wind-wave spectrum at extremely high wind speeds. IOP Conf. Series: Earth and Environmental Science 35 (1), 012009.Google Scholar
Takagaki, N., Komori, S., Suzuki, N., Iwano, K., Kuramoto, T., Shimada, S., Kurose, R. & Takahashi, K. 2012 Strong correlation between the drag coefficient and the shape of the wind sea spectrum over a broad range of wind. Geophys. Res. Lett. 39, L23604.Google Scholar
Takagaki, N., Kurose, R., Tsujimoto, Y., Komori, S. & Takahashi, K. 2015 Effects of turbulent eddies and Langmuir circulations on scalar transfer in a sheared wind-driven liquid flow. Phys. Fluids 27, 016603.Google Scholar
Tetens, O. 1930 Über einige meteorologiche Begriffe. Z. Geophys. 6, 297–309.Google Scholar
Thorpe, S. A. 2004 Langmuir circulation. Annu. Rev. Fluid Mech. 36, 55–79.CrossRefGoogle Scholar
Toba, Y. 1972 Local balance in the air–sea boundary processes. Part I: On the growth processes of wind waves. J. Oceanogr. 28, 109–121.Google Scholar
Tsai, W. T., Chen, S. M., Lu, G. H. & Garbe, C. S. 2013 Characteristics of interfacial signatures on a wind-driven gravity–capillary wave. J. Geophys. Res. Oceans 118, 1715–1735.CrossRefGoogle Scholar
Turney, D. E. & Banerjee, S. 2013 Air–water gas transfer and near-surface motions. J. Fluid Mech. 733, 588–624.Google Scholar
Yamamoto, Y., Kunugi, T., Satake, S. & Serizawa, A. 2004 Turbulent structures and heat transfer across the air–liquid interface in the wind-driven turbulent flow. Trans. JSME B 70 (692), 1006–1012.CrossRefGoogle Scholar
Zappa, C. J., Asher, W. E. & Jessup, A. T. 2004 Microbreaking and the enhancement of air–water transfer velocity. J. Geophys. Res. 109, C08S16.Google Scholar
Zhang, J. A, Black, P. G., French, J. R. & Drennan, W. M. 2008 First direct measurements of enthalpy flux in the hurricane boundary layer: the CBLAST results. Geophys. Res. Lett. 35, L14813.Google Scholar