Hostname: page-component-586b7cd67f-dlnhk Total loading time: 0 Render date: 2024-11-28T18:46:15.943Z Has data issue: false hasContentIssue false
  • English
  • Français

Direct numerical simulation of turbulence modulation by particles in compressible isotropic turbulence

Published online by Cambridge University Press:  26 October 2017

Qi Dai ,
Kun Luo Open the ORCID record for Kun Luo [Opens in a new window] ,
Tai Jin  and
Jianren Fan Open the ORCID record for Jianren Fan [Opens in a new window]
Qi Dai
Affiliation:
State Key Laboratory of Clean Energy Utilization, Zhejiang University, Hangzhou 310027, PR China
Kun Luo*
Affiliation:
State Key Laboratory of Clean Energy Utilization, Zhejiang University, Hangzhou 310027, PR China
Tai Jin
Affiliation:
State Key Laboratory of Clean Energy Utilization, Zhejiang University, Hangzhou 310027, PR China
Jianren Fan
Affiliation:
State Key Laboratory of Clean Energy Utilization, Zhejiang University, Hangzhou 310027, PR China
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

In this paper, a systematic investigation of turbulence modulation by particles and its underlying physical mechanisms in decaying compressible isotropic turbulence is performed by using direct numerical simulations with the Eulerian–Lagrangian point-source approach. Particles interact with turbulence through two-way coupling and the initial turbulent Mach number is 1.2. 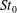Five simulations with different particle diameters (or initial Stokes numbers, $St_{0}$) are conducted while fixing both their volume fraction and particle densities. The underlying physical mechanisms responsible for turbulence modulation are analysed through investigating the particle motion in the different cases and the transport equations of turbulent kinetic energy, vorticity and dilatation, especially the two-way coupling terms. Our results show that microparticles (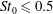$St_{0}\leqslant 0.5$) augment turbulent kinetic energy and the rotational motion of fluid, critical particles (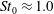$St_{0}\approx 1.0$) enhance the rotational motion of fluid, and large particles (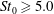$St_{0}\geqslant 5.0$) attenuate turbulent kinetic energy and the rotational motion of fluid. The compressibility of the turbulence field is suppressed for all the cases, and the suppression is more significant if the Stokes number of particles is close to 1. The modifications of turbulent kinetic energy, the rotational motion and the compressibility are all related with the particle inertia and distributions, and the suppression of the compressibility is attributed to the preferential concentration and the inertia of particles.

JFM classification

Turbulent Flows: Compressible turbulence Turbulent Flows: Isotropic turbulence Multiphase and Particle-laden Flows: Multiphase and Particle-laden Flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 832 , 10 December 2017 , pp. 438 - 482
Check for updates
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abdelsamie, A. H. & Lee, C. 2012 Decaying versus stationary turbulence in particle-laden isotropic turbulence: turbulence modulation mechanism. Phys. Fluids 24, 15106.Google Scholar
Abdelsamie, A. H. & Lee, C. 2013 Decaying versus stationary turbulence in particle-laden isotropic turbulence: heavy particle statistics modifications. Phys. Fluids 25, 33303.Google Scholar
Balachandar, S. & Eaton, J. K. 2010 Turbulent dispersed multiphase flow. Annu. Rev. Fluid Mech. 42, 111–133.Google Scholar
Boivin, M., Simonin, O. & Squires, K. D. 1998 Direct numerical simulation of turbulence modulation by particles in isotropic turbulence. J. Fluid Mech. 375, 235–263.Google Scholar
Crowe, C. T., Sommerfeld, M. & Tsuji, Y. 1998 Multiphase Flows with Droplets and Particles. CRC Press.Google Scholar
Davis, S., Dittmann, T., Jacobs, G. B. & Don, W. S. 2011 High-fidelity Eulerian–Lagrangian methods for simulation of three dimensional, unsteady, high-speed, two-phase flows in high-speed combustors. In 9th Annual International Energy Conversion Engineering Conference, p. 5744.Google Scholar
Donzis, D. A. & Jagannathan, S. 2013 Fluctuations of thermodynamic variables in stationary compressible turbulence. J. Fluid Mech. 733, 221–244.CrossRefGoogle Scholar
Elghobashi, S. 1994 On predicting particle-laden turbulent flows. Appl. Sci. Res. 52, 309–329.CrossRefGoogle Scholar
Ferrante, A. & Elghobashi, S. 2003 On the physical mechanisms of two-way coupling in particle-laden isotropic turbulence. Phys. Fluids 15, 315–329.Google Scholar
Goto, S. & Vassilicos, J. C. 2008 Sweep-stick mechanism of heavy particle clustering in fluid turbulence. Phys. Rev. Lett. 100, 054503.Google Scholar
He, Z.2004 Direct numerical simulation of non-isothermal gas-particle homogeneous isotropic turbulence. PhD thesis, Huazhong University of Science and Technology.Google Scholar
Hunt, J. C. R., Wray, A. A. & Moin, P. 1988 Eddies, streams, and convergence zones in turbulent flows. In Proceedings of Summer Program, pp. 9–14. Centre for Turbulence Research.Google Scholar
Jin, T., Luo, K., Dai, Q. & Fan, J. 2016a Simulations of cellular detonation interaction with turbulent flows. AIAA J 54, 419–433.Google Scholar
Jin, T., Luo, K., Dai, Q. & Fan, J. 2016b Direct numerical simulation on supersonic turbulent reacting and non-reacting spray jet in heated coflow. Fuel 164, 267–276.Google Scholar
Kennedy, C. A. & Carpenter, M. H. 1994 Several new numerical methods for compressible shear-layer simulations. Appl. Numer. Maths 14, 397–433.Google Scholar
Libby, P. A. 1996 Introduction to Turbulence. Taylor & Francis.Google Scholar
Lucci, F., Ferrante, A. & Elghobashi, S. 2010 Modulation of isotropic turbulence by particles of Taylor length-scale size. J. Fluid Mech. 650, 5–55.Google Scholar
Martín, M. P., Taylor, E. M., Wu, M. & Weirs, V. G. 2006 A bandwidth-optimized WENO scheme for the effective direct numerical simulation of compressible turbulence. J. Comput. Phys. 220, 270–289.Google Scholar
Mashayek, F. 1998 Droplet turbulence interactions in low-Mach-number homogeneous shear two-phase flows. J. Fluid Mech. 367, 163–203.Google Scholar
Maxey, M. R. 1987 The gravitational settling of aerosol particles in homogeneous turbulence and random flow fields. J. Fluid Mech. 174, 441–465.Google Scholar
Parmar, M., Haselbacher, A. & Balachandar, S. 2012 Equation of motion for a sphere in non-uniform compressible flows. J. Fluid Mech. 699, 352–375.Google Scholar
Passot, T. & Pouquet, A. 1987 Numerical simulation of compressible homogeneous flows in the turbulent regime. J. Fluid Mech. 181, 441–466.Google Scholar
Picano, F., Battista, F., Troiani, G. & Casciola, C. M. 2011 Dynamics of PIV seeding particles in turbulent premixed flames. Exp. Fluids 50, 75–88.Google Scholar
Pope, S. B. 2000 Turbulent Flows. Cambridge University Press.Google Scholar
Samtaney, R., Pullin, D. I. & Kosović, B. 2001 Direct numerical simulation of decaying compressible turbulence and shocklet statistics. Phys. Fluids 13, 1415.Google Scholar
Shu, C. W. & Osher, S. 1988 Efficient implementation of essentially non-oscillatory shock-capturing schemes. J. Comput. Phys. 77, 439–471.CrossRefGoogle Scholar
Sutherland, W. 1893 The viscosity of gases and molecular force. Phil. Mag. S5 36, 507–531.Google Scholar
Wang, J., Shi, Y., Wang, L., Xiao, Z., He, X. & Chen, S. 2011 Effect of shocklets on the velocity gradients in highly compressible isotropic turbulence. Phys. Fluids 23, 125103.Google Scholar
Wang, J., Shi, Y., Wang, L., Xiao, Z., He, X. T. & Chen, S. 2012a Effect of compressibility on the small-scale structures in isotropic turbulence. J. Fluid Mech. 713, 588–631.Google Scholar
Wang, J., Shi, Y., Wang, L. P., Xiao, Z. & He, X. T. 2012b Scaling and statistics in three-dimensional compressible turbulence. Phys. Rev. Lett. 108, 214505.CrossRefGoogle ScholarPubMed
Wang, J., Yang, Y., Shi, Y., Xiao, Z., He, X. T. & Chen, S. 2013 Cascade of kinetic energy in three-dimensional compressible turbulence. Phys. Rev. Lett. 110, 214505.Google Scholar
Wang, J., Gotoh, T. & Watanabe, T. 2017a Shocklet statistics in compressible isotropic turbulence. Phys. Rev. Fluids 2, 023401.Google Scholar
Wang, J., Gotoh, T. & Watanabe, T. 2017b Spectra and statistics in compressible isotropic turbulence. Phys. Rev. Fluids 2, 013403.Google Scholar
Xia, Z., Shi, Y., Zhang, Q. & Chen, S. 2016 Modulation to compressible homogenous turbulence by heavy point particles. I. Effect of particles’ density. Phys. Fluids 28, 16103.Google Scholar
Yang, Y., Wang, J., Shi, Y., Xiao, Z., He, X. T. & Chen, S. 2014 Interactions between inertial particles and shocklets in compressible turbulent flow. Phys. Fluids 26, 91702.CrossRefGoogle Scholar
Zhang, Q., Liu, H., Ma, Z. & Xiao, Z. 2016 Preferential concentration of heavy particles in compressible isotropic turbulence. Phys. Fluids 28, 55104.Google Scholar