Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Cheng, T.S.
and
Liu, W.-H.
2014.
Effects of cavity inclination on mixed convection heat transfer in lid-driven cavity flows.
Computers & Fluids,
Vol. 100,
Issue. ,
p.
108.
Qiu, Xiang
Liu, Yu-Lu
and
Zhou, Quan
2014.
Local dissipation scales in two-dimensional Rayleigh-Taylor turbulence.
Physical Review E,
Vol. 90,
Issue. 4,
van der Poel, Erwin P.
Ostilla-Mónico, Rodolfo
Verzicco, Roberto
and
Lohse, Detlef
2014.
Effect of velocity boundary conditions on the heat transfer and flow topology in two-dimensional Rayleigh-Bénard convection.
Physical Review E,
Vol. 90,
Issue. 1,
Bao, Yun
Chen, Jun
Liu, Bo-Fang
She, Zhen-Su
Zhang, Jun
and
Zhou, Quan
2015.
Enhanced heat transport in partitioned thermal convection.
Journal of Fluid Mechanics,
Vol. 784,
Issue. ,
Xia, Shu-Ning
Wan, Zhen-Hua
Liu, Shuang
Wang, Qi
and
Sun, De-Jun
2016.
Flow reversals in Rayleigh–Bénard convection with non-Oberbeck–Boussinesq effects.
Journal of Fluid Mechanics,
Vol. 798,
Issue. ,
p.
628.
Zhou, Quan
Huang, Yong-Xiang
Lu, Zhi-Ming
Liu, Yu-Lu
and
Ni, Rui
2016.
Scale-to-scale energy and enstrophy transport in two-dimensional Rayleigh–Taylor turbulence.
Journal of Fluid Mechanics,
Vol. 786,
Issue. ,
p.
294.
Zhou, Quan
and
Jiang, Lin-Feng
2016.
Kinetic and thermal energy dissipation rates in two-dimensional Rayleigh-Taylor turbulence.
Physics of Fluids,
Vol. 28,
Issue. 4,
Huang, Shi-Di
and
Xia, Ke-Qing
2016.
Effects of geometric confinement in quasi-2-D turbulent Rayleigh–Bénard convection.
Journal of Fluid Mechanics,
Vol. 794,
Issue. ,
p.
639.
Zhang, Yang
Huang, Yong-Xiang
Jiang, Nan
Liu, Yu-Lu
Lu, Zhi-Ming
Qiu, Xiang
and
Zhou, Quan
2017.
Statistics of velocity and temperature fluctuations in two-dimensional Rayleigh-Bénard convection.
Physical Review E,
Vol. 96,
Issue. 2,
Zhang, Yang
Zhou, Quan
and
Sun, Chao
2017.
Statistics of kinetic and thermal energy dissipation rates in two-dimensional turbulent Rayleigh–Bénard convection.
Journal of Fluid Mechanics,
Vol. 814,
Issue. ,
p.
165.
Qiang, Wei
Cao, Hui
Li, Weimin
and
Zhang, Fansiqi
2017.
Counter-gradient heat transport by large-scale circulation in two-dimensional turbulent convection.
EPL (Europhysics Letters),
Vol. 118,
Issue. 6,
p.
64001.
Dabbagh, F.
Trias, F. X.
Gorobets, A.
and
Oliva, A.
2017.
A priori study of subgrid-scale features in turbulent Rayleigh-Bénard convection.
Physics of Fluids,
Vol. 29,
Issue. 10,
Huang, Xiao-Jie
Zhang, Li
Hu, Yu-Peng
and
Li, You-Rong
2018.
Large eddy simulation on Rayleigh–Bénard convection of cold water in the neighborhood of the maximum density.
Fluid Dynamics Research,
Vol. 50,
Issue. 3,
p.
035503.
Wang, Qi
Wan, Zhen-Hua
Yan, Rui
and
Sun, De-Jun
2018.
Multiple states and heat transfer in two-dimensional tilted convection with large aspect ratios.
Physical Review Fluids,
Vol. 3,
Issue. 11,
Gao, Zhen-Yuan
Luo, Jia-Hui
and
Bao, Yun
2018.
Numerical study of heat-transfer in two- and quasi-two-dimensional Rayleigh–Bénard convection.
Chinese Physics B,
Vol. 27,
Issue. 10,
p.
104702.
Wang, Qi
Xia, Shu-Ning
Wang, Bo-Fu
Sun, De-Jun
Zhou, Quan
and
Wan, Zhen-Hua
2018.
Flow reversals in two-dimensional thermal convection in tilted cells.
Journal of Fluid Mechanics,
Vol. 849,
Issue. ,
p.
355.
Xu, Bo-Lun
Wang, Qi
Wan, Zhen-Hua
Yan, Rui
and
Sun, De-Jun
2018.
Heat transport enhancement and scaling law transition in two-dimensional Rayleigh-Bénard convection with rectangular-type roughness.
International Journal of Heat and Mass Transfer,
Vol. 121,
Issue. ,
p.
872.
Zhu, Xiaojue
Mathai, Varghese
Stevens, Richard J. A. M.
Verzicco, Roberto
and
Lohse, Detlef
2018.
Transition to the Ultimate Regime in Two-Dimensional Rayleigh-Bénard Convection.
Physical Review Letters,
Vol. 120,
Issue. 14,
Wang, Qi
Zhou, Quan
Wan, Zhen-Hua
and
Sun, De-Jun
2019.
Penetrative turbulent Rayleigh–Bénard convection in two and three dimensions.
Journal of Fluid Mechanics,
Vol. 870,
Issue. ,
p.
718.
Zhu, Xu
Jiang, Lin-Feng
Zhou, Quan
and
Sun, Chao
2019.
Turbulent Rayleigh–Bénard convection in an annular cell.
Journal of Fluid Mechanics,
Vol. 869,
Issue. ,
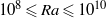 $1{0}^{8} \leqslant Ra\leqslant 1{0}^{10} $ and the Prandtl number range
$1{0}^{8} \leqslant Ra\leqslant 1{0}^{10} $ and the Prandtl number range 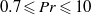 $0. 7\leqslant Pr\leqslant 10$. We find that there exists strong counter-gradient local heat flux with magnitude much larger than the global Nusselt number
$0. 7\leqslant Pr\leqslant 10$. We find that there exists strong counter-gradient local heat flux with magnitude much larger than the global Nusselt number  $Nu$ of the system. Two mechanisms for generating counter-gradient heat transport are identified: one is due to the bulk dynamics and the other is due to the competition between the corner-flow rolls and the large-scale circulation (LSC). While the magnitude of the former is found to increase with increasing Prandtl number, that of the latter maximizes at medium
$Nu$ of the system. Two mechanisms for generating counter-gradient heat transport are identified: one is due to the bulk dynamics and the other is due to the competition between the corner-flow rolls and the large-scale circulation (LSC). While the magnitude of the former is found to increase with increasing Prandtl number, that of the latter maximizes at medium 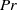 $Pr$. We further reveal that the corner–LSC competition leads to the anomalous
$Pr$. We further reveal that the corner–LSC competition leads to the anomalous 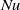 $Nu$–
$Nu$–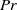 $Pr$ relation in 2D RB convection, i.e.
$Pr$ relation in 2D RB convection, i.e.  $Nu(Pr)$ minimizes, rather than maximizes as in the three-dimensional cylindrical case, at
$Nu(Pr)$ minimizes, rather than maximizes as in the three-dimensional cylindrical case, at 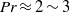 $Pr\approx 2\sim 3$ for moderate
$Pr\approx 2\sim 3$ for moderate 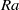 $Ra$.
$Ra$.

