Hostname: page-component-cd9895bd7-dzt6s Total loading time: 0 Render date: 2024-12-19T04:08:41.918Z Has data issue: false hasContentIssue false
  • English
  • Français

Converging gravity currents of power-law fluid

Published online by Cambridge University Press:  05 May 2021

S. Longo Open the ORCID record for S. Longo [Opens in a new window] ,
L. Chiapponi Open the ORCID record for L. Chiapponi [Opens in a new window] ,
D. Petrolo Open the ORCID record for D. Petrolo [Opens in a new window] ,
A. Lenci Open the ORCID record for A. Lenci [Opens in a new window]  and
V. Di Federico Open the ORCID record for V. Di Federico [Opens in a new window]
S. Longo
Affiliation:
Department of Engineering and Architecture, Università degli Studi di Parma, Parco Area delle Scienze 181/A, 43124Parma, Italy
L. Chiapponi*
Affiliation:
Department of Engineering and Architecture, Università degli Studi di Parma, Parco Area delle Scienze 181/A, 43124Parma, Italy
D. Petrolo
Affiliation:
Department of Engineering and Architecture, Università degli Studi di Parma, Parco Area delle Scienze 181/A, 43124Parma, Italy
A. Lenci
Affiliation:
Department of Civil, Chemical, Environmental, and Materials Engineering, Alma Mater Studiorum Università di Bologna, Viale Risorgimento 2, 40136Bologna, Italy
V. Di Federico
Affiliation:
Department of Civil, Chemical, Environmental, and Materials Engineering, Alma Mater Studiorum Università di Bologna, Viale Risorgimento 2, 40136Bologna, Italy
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We study non-Newtonian effects associated with power-law rheology of behaviour index $n$ on the propagation of horizontal gravity currents. Two different set-ups are examined: (i) converging flow toward the origin in a channel of gap thickness $b(x) \propto x^k$ and $k<1$ and (ii) converging flow along $r$ toward the centre in a cylinder. The front of the current propagates in the negative $x$ or $r$ direction reaching the origin in a finite touch-down time $t_c$ during the pre-closure phase; in the post-closure phase, the current flows back in the positive direction and progressively levels out. Under the classical viscous-buoyancy balance, the current propagation is described by a differential problem amenable to a self-similar solution of the second-kind coupling space and reduced time $t_r=t_c-t$. The problem formulation in the phase plane leads to an autonomous system of differential equations which requires numerical integration and yields the shape of the current and its front as $\xi _f\propto t_r^{\delta _c}$, $\xi _f$ being the self-similar variable value at the front and $\delta _c$ being the critical eigenvalue. 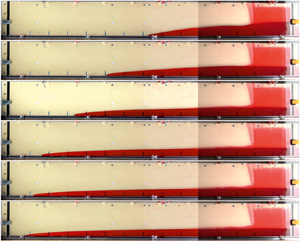The latter is a function of fluid rheology $n$ and of channel geometry $k$ for the first set-up; it is a function only of $n$ for the second set-up. The dependency on $n$ is modest. The theoretical formulation is validated through experiments conducted during both pre- and post-closure phases and aimed at measuring the front position and the profile of the current. Experimental results are in fairly good agreement with theory and allow quantitative determination of the time interval of validity of the intermediate asymptotics regime, when self-similarity is achieved and when it is lost.

JFM classification

Geophysical and Geological Flows: Gravity currents Low-Reynolds-number flows: Hele-Shaw flows
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 918 , 10 July 2021 , A5
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Al-Housseiny, T.T. & Stone, H.A. 2013 Controlling viscous fingering in tapered hele-shaw cells. Phys. Fluids 25 (9), 092102.CrossRefGoogle Scholar
Al-Housseiny, T.T., Tsai, P.A. & Stone, H.A. 2012 Control of interfacial instabilities using flow geometry. Nat. Phys. 8 (10), 747–750.CrossRefGoogle Scholar
Angenent, S.B. & Aronson, D.G. 1995 a The focusing problem for the radially symmetric porous medium equation. Commun. Part. Diff. Eq. 20 (7–8), 1217–1240.CrossRefGoogle Scholar
Angenent, S.B. & Aronson, D.G. 1995 b Intermediate asymptotics for convergent viscous gravity currents. Phys. Fluids 7 (1), 223–225.CrossRefGoogle Scholar
Angenent, S.B., Aronson, D.G., Betelu, S.I. & Lowengrub, J.S. 2001 Focusing of an elongated hole in porous medium flow. Physica D 151 (2), 228–252.CrossRefGoogle Scholar
Aronson, D.G. & Graveleau, J. 1993 A selfsimilar solution to the focusing problem for the porous medium equation. Eur. J. Appl. Maths 4 (1), 65–81.CrossRefGoogle Scholar
Aronson, D.G., Van Den Berg, J.B. & Hulshof, J. 2003 Parametric dependence of exponents and eigenvalues in focusing porous media flows. Eur. J. Appl. Maths 14 (4), 485–512.CrossRefGoogle Scholar
Aronsson, G. & Janfalk, U. 1992 On Hele-Shaw flow of power-law fluids. Eur. J. Appl. Maths 3 (4), 343–366.CrossRefGoogle Scholar
Ball, T.V. & Huppert, H.E. 2019 Similarity solutions and viscous gravity current adjustment times. J. Fluid Mech. 874, 285–298.CrossRefGoogle Scholar
Ball, T.V., Huppert, H.E., Lister, J. & Neufeld, J. 2017 The relaxation time for viscous and porous gravity currents following a change in flux. J. Fluid Mech. 821, 330–342.CrossRefGoogle Scholar
Barenblatt, G.I. 1996 Scaling, Self-Similarity, and Intermediate Asymptotics. Cambridge University Press.CrossRefGoogle Scholar
Barenblatt, G.I. 2003 Scaling, vol. 14. Cambridge University Press.CrossRefGoogle Scholar
Barenblatt, G.I. & Zel'Dovich, Y.B. 1972 Self-similar solutions as intermediate asymptotics. Annu. Rev. Fluid Mech. 4 (1), 285–312.CrossRefGoogle Scholar
Bongrand, G. & Tsai, P.A. 2018 Manipulation of viscous fingering in a radially tapered cell geometry. Phys. Rev. E 97 (6), 061101.CrossRefGoogle Scholar
Carpenter, H.J., Gholipour, A., Ghayesh, M.H., Zander, A.C. & Psaltis, P.J. 2020 A review on the biomechanics of coronary arteries. Intl J. Engng Sci. 147, 103201.CrossRefGoogle Scholar
Ciriello, V., Longo, S., Chiapponi, L. & Di Federico, V. 2016 Porous gravity currents: a survey to determine the joint influence of fluid rheology and variations of medium properties. Adv. Water Resour. 92, 105–115.CrossRefGoogle Scholar
Ciriello, V., Longo, S. & Di Federico, V. 2013 On shear thinning fluid flow induced by continuous mass injection in porous media with variable conductivity. Mech. Res. Commun. 52, 101–107.CrossRefGoogle Scholar
Cole, J.D. & Wagner, B.A. 1996 On self-similar solutions of Barenblatt's nonlinear filtration equation. Eur. J. Appl. Maths 7 (2), 151–167.CrossRefGoogle Scholar
Coussot, P. & Meunier, M. 1996 Recognition, classification and mechanical description of debris flows. Earth-Sci. Rev. 40 (3), 209–227.CrossRefGoogle Scholar
Daly, E. & Porporato, A. 2004 Similarity solutions of nonlinear diffusion problems related to mathematical hydraulics and the Fokker–Planck equation. Phys. Rev. E 70 (5), 056303.CrossRefGoogle ScholarPubMed
Di Federico, V. 1998 Non-Newtonian flow in a variable aperture fracture. Transp. Porous Media 30 (1), 75–86.CrossRefGoogle Scholar
Di Federico, V., Archetti, R. & Longo, S. 2012 Similarity solutions for spreading of a two-dimensional non-Newtonian gravity current. J. Non-Newtonian Fluid Mech. 177–178, 46–53.CrossRefGoogle Scholar
Di Federico, V., Longo, S., Chiapponi, L., Archetti, R. & Ciriello, V. 2014 Radial gravity currents in vertically graded porous media: theory and experiments for Newtonian and power-law fluids. Adv. Water Resour. 70, 65–76.CrossRefGoogle Scholar
Dias, E.O. & Miranda, J.A. 2013 Taper-induced control of viscous fingering in variable-gap Hele-Shaw flows. Phys. Rev. E 87 (5), 053015.CrossRefGoogle ScholarPubMed
Didden, N. & Maxworthy, T. 1982 The viscous spreading of plane and axisymmetric gravity currents. J. Fluid Mech. 121, 27–42.CrossRefGoogle Scholar
Diez, J.A., Gratton, J. & Minotti, F. 1992 a Self-similar solutions of the second kind of nonlinear diffusion-type equations. Q. Appl. Maths 50 (3), 401–414.CrossRefGoogle Scholar
Diez, J.A., Gratton, R. & Gratton, J. 1992 b Self-similar solution of the second kind for a convergent viscous gravity current. Phys. Fluids A 4 (6), 1148–1155.CrossRefGoogle Scholar
Diez, J.A., Thomas, L.P., Betelú, S., Gratton, R., Marino, B., Gratton, J., Aronson, D.G. & Angenent, S.B. 1998 Noncircular converging flows in viscous gravity currents. Phys. Rev. E 58 (5), 6182.CrossRefGoogle Scholar
Eggers, J. & Fontelos, M.A. 2015 Singularities: Formation, Structure, and Propagation, vol. 53. Cambridge University Press.CrossRefGoogle Scholar
Epelle, E.I. & Gerogiorgis, D.I. 2020 A review of technological advances and open challenges for oil and gas drilling systems engineering. AIChE J. 66 (4), e16842.CrossRefGoogle Scholar
Eshtiaghi, N., Markis, F., Yap, S.D., Baudez, J.-C. & Slatter, P. 2013 Rheological characterisation of municipal sludge: a review. Water Res. 47 (15), 5493–5510.CrossRefGoogle ScholarPubMed
Ghodgaonkar, A.A. 2019 Numerical methods for studying self-similar propagation ov viscous gravity currents. Master's thesis, Purdue University, West Lafayette, Indiana.Google Scholar
Gratton, J. & Minotti, F. 1990 Self-similar viscous gravity currents: phase-plane formalism. J. Fluid Mech. 210, 155–182.CrossRefGoogle Scholar
Gratton, J., Minotti, F. & Mahajan, S.M. 1999 Theory of creeping gravity currents of a non-Newtonian liquid. Phys. Rev. E 60 (6), 6960–6967.CrossRefGoogle ScholarPubMed
Gratton, J. & Perazzo, C.A. 2010 Self-similar collapse of a circular cavity of a power-law liquid. J. non-Newtonian Fluid Mech. 165 (3–4), 158–162.CrossRefGoogle Scholar
Huppert, H.E. 1982 The propagation of two-dimensional and axisymmetric viscous gravity currents over a rigid horizontal surface. J. Fluid Mech. 121, 43–58.CrossRefGoogle Scholar
Huppert, H.E. 2006 Gravity currents: a personal perspective. J. Fluid Mech. 554, 299–322.CrossRefGoogle Scholar
Huppert, H.E. & Woods, A.W. 1995 Gravity-driven flows in porous layers. J. Fluid Mech. 292, 55–69.CrossRefGoogle Scholar
Kondic, L., Palffy-Muhoray, P. & Shelley, M.J. 1996 Models of non-Newtonian Hele-Shaw flow. Phys. Rev. E 54 (5), R4536.CrossRefGoogle ScholarPubMed
Kondic, L., Shelley, M.J. & Palffy-Muhoray, P. 1998 Non-Newtonian Hele-Shaw flow and the Saffman–Taylor instability. Phys. Rev. Lett. 80 (7), 1433.CrossRefGoogle Scholar
Lareo, C., Fryer, P.J. & Barigou, M. 1997 The fluid mechanics of two-phase solid-liquid food flows: a review. Food Bioprod. Process. 75 (C2), 73–105.CrossRefGoogle Scholar
Lauriola, I., Felisa, G., Petrolo, D., Di Federico, V. & Longo, S. 2018 Porous gravity currents: axisymmetric propagation in horizontally graded medium and a review of similarity solutions. Adv. Water Resour. 115, 136–150.CrossRefGoogle Scholar
Livescu, S. 2012 Mathematical modeling of thixotropic drilling mud and crude oil flow in wells and pipelines – a review. J. Petrol. Sci. Engng 98–99, 174–184.CrossRefGoogle Scholar
Longo, S., Ciriello, V., Chiapponi, L. & Di Federico, V. 2015 a Combined effect of rheology and confining boundaries on spreading of porous gravity currents. Adv. Water Resour. 79, 140–152.CrossRefGoogle Scholar
Longo, S. & Di Federico, V. 2015 Unsteady flow of shear-thinning fluids in porous media with pressure-dependent properties. Transp. Porous Media 110 (3), 429–447.CrossRefGoogle Scholar
Longo, S., Di Federico, V. & Chiapponi, L. 2015 b Non-Newtonian power-law gravity currents propagating in confining boundaries. Environ. Fluid Mech. 15 (3), 515–535.CrossRefGoogle Scholar
Lyle, S., Huppert, H.E., Hallworth, M., Bickle, M. & Chadwick, A. 2005 Axisymmetric gravity currents in a porous medium. J. Fluid Mech. 543, 293–302.CrossRefGoogle Scholar
McCue, S.W., Jin, W., Moroney, T.J., Lo, K.-Y., Chou, S.-E. & Simpson, M.J. 2019 Hole-closing model reveals exponents for nonlinear degenerate diffusivity functions in cell biology. Physica D 398, 130–140.CrossRefGoogle Scholar
McCue, S.W. & King, J.R. 2011 Contracting bubbles in Hele-Shaw cells with a power-law fluid. Nonlinearity 24 (2), 613.CrossRefGoogle Scholar
Morrell, R.S. & De Waele, A. 1920 Rubber, Resins, Paints and Varnishes. Nostrand.Google Scholar
Morrow, L.C., Moroney, T.J. & McCue, S.W. 2019 Numerical investigation of controlling interfacial instabilities in non-standard Hele-Shaw configurations. arXiv:1901.00288.CrossRefGoogle Scholar
Ostwald, W. 1929 Ueber die rechnerische darstellung des strukturgebietes der viskosität. Colloid Polym. Sci. 47 (2), 176–187.Google Scholar
Perazzo, C.A. & Gratton, J. 2003 Thin film of non-Newtonian fluid on an incline. Phys. Rev. E 67 (1), 016307.CrossRefGoogle Scholar
Perazzo, C.A. & Gratton, J. 2005 Exact solutions for two-dimensional steady flows of a power-law liquid on an incline. Phys. Fluids 17 (1), 013102.CrossRefGoogle Scholar
Simpson, J.E. 1982 Gravity currents in the laboratory, atmosphere, and ocean. Annu. Rev. Fluid Mech. 14, 213–234.CrossRefGoogle Scholar
Slim, A.C. & Huppert, H.E. 2004 Self-similar solutions of the axisymmetric shallow-water equations governing converging inviscid gravity currents. J. Fluid Mech. 506, 331–355.CrossRefGoogle Scholar
Stokes, G.G. 1880 On the Theory of Oscillatory Waves. Transactions of the Cambridge Philosophical Society.Google Scholar
Wagner, B. 2005 An asymptotic approach to second-kind similarity solutions of the modified porous-medium equation. J. Engng Maths 53 (3–4), 201–220.CrossRefGoogle Scholar
Wolfram Research, Inc. 2017 Mathematica, Version 11.1. Champaign, IL.Google Scholar
Zheng, Z., Christov, I.C. & Stone, H.A. 2014 Influence of heterogeneity on second-kind self-similar solutions for viscous gravity currents. J. Fluid Mech. 747, 218–246.CrossRefGoogle Scholar
Zheng, Z., Shin, S. & Stone, H.A. 2015 Converging gravity currents over a permeable substrate. J. Fluid Mech. 778, 669–690.CrossRefGoogle Scholar