Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Liang, Yu
and
Luo, Xisheng
2021.
Shock-induced dual-layer evolution.
Journal of Fluid Mechanics,
Vol. 929,
Issue. ,
Feng, Lili
Xu, Jinru
Zhai, Zhigang
and
Luo, Xisheng
2021.
Evolution of shock-accelerated double-layer gas cylinder.
Physics of Fluids,
Vol. 33,
Issue. 8,
Ding, Juchun
Deng, Xiaoming
and
Luo, Xisheng
2021.
Convergent Richtmyer–Meshkov instability on a light gas layer with perturbed inner and outer surfaces.
Physics of Fluids,
Vol. 33,
Issue. 10,
Zhou, Ye
Williams, Robin J.R.
Ramaprabhu, Praveen
Groom, Michael
Thornber, Ben
Hillier, Andrew
Mostert, Wouter
Rollin, Bertrand
Balachandar, S.
Powell, Phillip D.
Mahalov, Alex
and
Attal, N.
2021.
Rayleigh–Taylor and Richtmyer–Meshkov instabilities: A journey through scales.
Physica D: Nonlinear Phenomena,
Vol. 423,
Issue. ,
p.
132838.
Li, Xinliang
Fu, Yaowei
Yu, Changping
and
Li, Li
2021.
Statistical characteristics of turbulent mixing in spherical and cylindrical converging Richtmyer–Meshkov instabilities.
Journal of Fluid Mechanics,
Vol. 928,
Issue. ,
Liang, Yu
and
Luo, Xisheng
2021.
On shock-induced heavy-fluid-layer evolution.
Journal of Fluid Mechanics,
Vol. 920,
Issue. ,
Xu, Jinru
Wang, He
Feng, Lili
Zhai, Zhigang
and
Luo, Xisheng
2021.
Interaction of a shock with two concentric/eccentric cylinders.
Experiments in Fluids,
Vol. 62,
Issue. 10,
Dang, Zi-Han
Zheng, Chun
Zhang, Huan-Hao
and
Chen, Zhi-Hua
2022.
Evolution mechanism of double-layer heavy gas column interface with sinusoidal disturbance induced by convergent shock wave.
Acta Physica Sinica,
Vol. 71,
Issue. 21,
p.
214703.
Liang, Yu
2022.
Fundamental Studies of Shock-Driven Hydrodynamic Instabilities.
p.
1.
Li, Jianming
Ding, Juchun
Luo, Xisheng
and
Zou, Liyong
2022.
Instability of a heavy gas layer induced by a cylindrical convergent shock.
Physics of Fluids,
Vol. 34,
Issue. 4,
Liang, Yu
and
Luo, Xisheng
2022.
On shock-induced light-fluid-layer evolution.
Journal of Fluid Mechanics,
Vol. 933,
Issue. ,
Liang, Yu
2022.
The phase effect on the Richtmyer–Meshkov instability of a fluid layer.
Physics of Fluids,
Vol. 34,
Issue. 3,
Zhang, Yibo
Zhou, Zhangbo
Ding, Juchun
and
Luo, Xisheng
2022.
Interaction of a planar shock wave with two heavy/light interfaces.
Acta Mechanica Sinica,
Vol. 38,
Issue. 9,
Chen, Feng
Xu, Aiguo
Zhang, Yudong
Gan, Yanbiao
Liu, Bingbing
and
Wang, Shuang
2022.
Effects of the initial perturbations on the Rayleigh—Taylor—Kelvin—Helmholtz instability system.
Frontiers of Physics,
Vol. 17,
Issue. 3,
Zheng, Chun
He, Yong
Zhang, Huanhao
and
Chen, Zhihua
2022.
Effects of the parameters of inner air cylinder on evolution of annular SF6 cylinder accelerated by a planar shock wave.
Physics of Fluids,
Vol. 34,
Issue. 12,
Liang, Yu
and
Luo, Xisheng
2022.
On shock-induced evolution of a gas layer with two fast/slow interfaces.
Journal of Fluid Mechanics,
Vol. 939,
Issue. ,
Zhang, Duo
Ding, Juchun
Li, Ming
Zou, Liyong
and
Luo, Xisheng
2023.
Effects of reverberating waves and interface coupling on a divergent Richtmyer–Meshkov instability.
Journal of Fluid Mechanics,
Vol. 975,
Issue. ,
Liang, Yu
Liu, Lili
Luo, Xisheng
and
Wen, Chih-Yung
2023.
Hydrodynamic instabilities of a dual-mode air–SF6 interface induced by a cylindrically convergent shock.
Journal of Fluid Mechanics,
Vol. 963,
Issue. ,
Wang, Tao
Zhong, Min
Wang, Bing
Li, Ping
and
Bai, Jingsong
2023.
Evolution of turbulent mixing driven by implosion in spherical geometry.
Journal of Turbulence,
Vol. 24,
Issue. 9-10,
p.
419.
2023.
Heat transfer effects on multiphase Richtmyer–Meshkov instability of dense gas–particle flow.
Physics of Fluids,
Vol. 35,
Issue. 5,
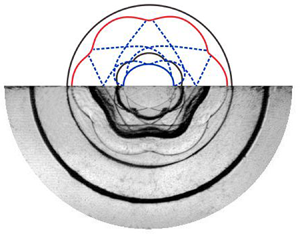
 $\textrm {SF}_6$ layer with a uniform outer surface and a sinusoidal inner surface surrounded by air (generated by a novel soap film technique) is studied in a semiannular convergent shock tube using high-speed schlieren photography. The outer interface initially suffers only a slight deformation over a long period of time, but distorts quickly at late stages when the inner interface is close to it and produces strong coupling effects. The development of the inner interface can be divided into three stages. At stage I, the interface amplitude first drops suddenly to a lower value due to shock compression, then decreases gradually to zero (phase reversal) and later increases sustainedly in the negative direction. After the reshock (stage II), the perturbation amplitude exhibits long-term quasi-linear growth with time. The quasi-linear growth rate depends weakly on the pre-reshock amplitude and wavelength, but strongly on the pre-reshock growth rate. An empirical model for the growth of convergent RMI under reshock is proposed, which reasonably predicts the present results and those in the literature. At stage III, the perturbation growth is promoted by the Rayleigh–Taylor instability caused by a rarefaction wave reflected from the outer interface. It is found that both the Rayleigh–Taylor effect and the interface coupling depend heavily on the layer thickness. Therefore, controlling the layer thickness is an effective way to modulate the late-stage instability growth, which may be useful for the target design.
$\textrm {SF}_6$ layer with a uniform outer surface and a sinusoidal inner surface surrounded by air (generated by a novel soap film technique) is studied in a semiannular convergent shock tube using high-speed schlieren photography. The outer interface initially suffers only a slight deformation over a long period of time, but distorts quickly at late stages when the inner interface is close to it and produces strong coupling effects. The development of the inner interface can be divided into three stages. At stage I, the interface amplitude first drops suddenly to a lower value due to shock compression, then decreases gradually to zero (phase reversal) and later increases sustainedly in the negative direction. After the reshock (stage II), the perturbation amplitude exhibits long-term quasi-linear growth with time. The quasi-linear growth rate depends weakly on the pre-reshock amplitude and wavelength, but strongly on the pre-reshock growth rate. An empirical model for the growth of convergent RMI under reshock is proposed, which reasonably predicts the present results and those in the literature. At stage III, the perturbation growth is promoted by the Rayleigh–Taylor instability caused by a rarefaction wave reflected from the outer interface. It is found that both the Rayleigh–Taylor effect and the interface coupling depend heavily on the layer thickness. Therefore, controlling the layer thickness is an effective way to modulate the late-stage instability growth, which may be useful for the target design.